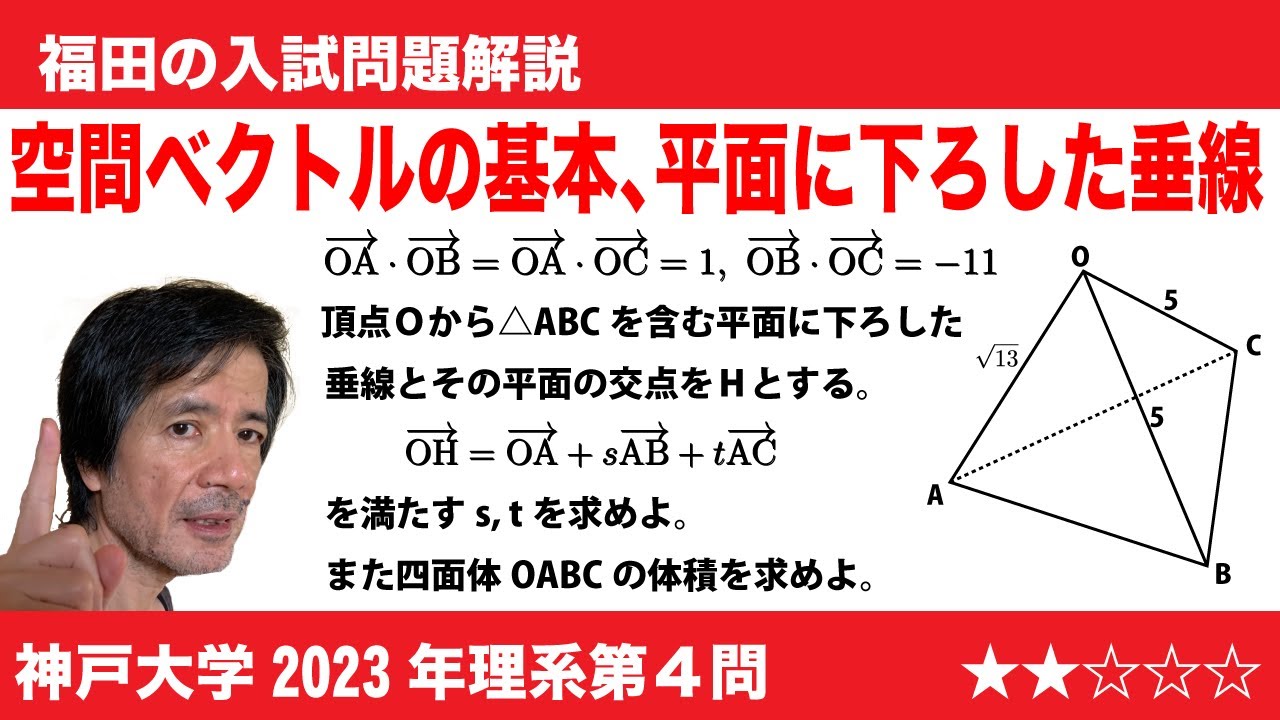
問題文全文(内容文):
$\Large{\boxed{1}}$ (4)四面体OABCにおいて、辺OAを1:3に内分する点をD、辺ABを1:2に内分する点をE、辺OCを1:2に内分する点をFとすると、
$\overrightarrow{DE}$=$\frac{\boxed{\ \ ノ\ \ }}{\boxed{\ \ ハヒ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ フ\ \ }}{\boxed{\ \ ヘ\ \ }}\overrightarrow{OB}$, $\overrightarrow{DF}$=$-\frac{\boxed{\ \ ホ\ \ }}{\boxed{\ \ マ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ ミ\ \ }}{\boxed{\ \ ム\ \ }}\overrightarrow{OC}$
である。さらに、3点D,E,Fを通る平面と辺BCの交点をGとすると、
$\overrightarrow{DF}$=$\frac{\boxed{\ \ メ\ \ }}{\boxed{\ \ モ\ \ }}\overrightarrow{DE}$+$\frac{\boxed{\ \ ヤ\ \ }}{\boxed{\ \ ユ\ \ }}\overrightarrow{DF}$
である。したがって、$\overrightarrow{BG}$=$\frac{\boxed{\ \ ヨ\ \ }}{\boxed{\ \ ラ\ \ }}\overrightarrow{BC}$ となる。
$\Large{\boxed{1}}$ (4)四面体OABCにおいて、辺OAを1:3に内分する点をD、辺ABを1:2に内分する点をE、辺OCを1:2に内分する点をFとすると、
$\overrightarrow{DE}$=$\frac{\boxed{\ \ ノ\ \ }}{\boxed{\ \ ハヒ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ フ\ \ }}{\boxed{\ \ ヘ\ \ }}\overrightarrow{OB}$, $\overrightarrow{DF}$=$-\frac{\boxed{\ \ ホ\ \ }}{\boxed{\ \ マ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ ミ\ \ }}{\boxed{\ \ ム\ \ }}\overrightarrow{OC}$
である。さらに、3点D,E,Fを通る平面と辺BCの交点をGとすると、
$\overrightarrow{DF}$=$\frac{\boxed{\ \ メ\ \ }}{\boxed{\ \ モ\ \ }}\overrightarrow{DE}$+$\frac{\boxed{\ \ ヤ\ \ }}{\boxed{\ \ ユ\ \ }}\overrightarrow{DF}$
である。したがって、$\overrightarrow{BG}$=$\frac{\boxed{\ \ ヨ\ \ }}{\boxed{\ \ ラ\ \ }}\overrightarrow{BC}$ となる。
単元:
#大学入試過去問(数学)#空間ベクトル#空間ベクトル#学校別大学入試過去問解説(数学)#明治大学#数学(高校生)#数C
指導講師:
福田次郎
問題文全文(内容文):
$\Large{\boxed{1}}$ (4)四面体OABCにおいて、辺OAを1:3に内分する点をD、辺ABを1:2に内分する点をE、辺OCを1:2に内分する点をFとすると、
$\overrightarrow{DE}$=$\frac{\boxed{\ \ ノ\ \ }}{\boxed{\ \ ハヒ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ フ\ \ }}{\boxed{\ \ ヘ\ \ }}\overrightarrow{OB}$, $\overrightarrow{DF}$=$-\frac{\boxed{\ \ ホ\ \ }}{\boxed{\ \ マ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ ミ\ \ }}{\boxed{\ \ ム\ \ }}\overrightarrow{OC}$
である。さらに、3点D,E,Fを通る平面と辺BCの交点をGとすると、
$\overrightarrow{DF}$=$\frac{\boxed{\ \ メ\ \ }}{\boxed{\ \ モ\ \ }}\overrightarrow{DE}$+$\frac{\boxed{\ \ ヤ\ \ }}{\boxed{\ \ ユ\ \ }}\overrightarrow{DF}$
である。したがって、$\overrightarrow{BG}$=$\frac{\boxed{\ \ ヨ\ \ }}{\boxed{\ \ ラ\ \ }}\overrightarrow{BC}$ となる。
$\Large{\boxed{1}}$ (4)四面体OABCにおいて、辺OAを1:3に内分する点をD、辺ABを1:2に内分する点をE、辺OCを1:2に内分する点をFとすると、
$\overrightarrow{DE}$=$\frac{\boxed{\ \ ノ\ \ }}{\boxed{\ \ ハヒ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ フ\ \ }}{\boxed{\ \ ヘ\ \ }}\overrightarrow{OB}$, $\overrightarrow{DF}$=$-\frac{\boxed{\ \ ホ\ \ }}{\boxed{\ \ マ\ \ }}\overrightarrow{OA}$+$\frac{\boxed{\ \ ミ\ \ }}{\boxed{\ \ ム\ \ }}\overrightarrow{OC}$
である。さらに、3点D,E,Fを通る平面と辺BCの交点をGとすると、
$\overrightarrow{DF}$=$\frac{\boxed{\ \ メ\ \ }}{\boxed{\ \ モ\ \ }}\overrightarrow{DE}$+$\frac{\boxed{\ \ ヤ\ \ }}{\boxed{\ \ ユ\ \ }}\overrightarrow{DF}$
である。したがって、$\overrightarrow{BG}$=$\frac{\boxed{\ \ ヨ\ \ }}{\boxed{\ \ ラ\ \ }}\overrightarrow{BC}$ となる。
投稿日:2023.11.14