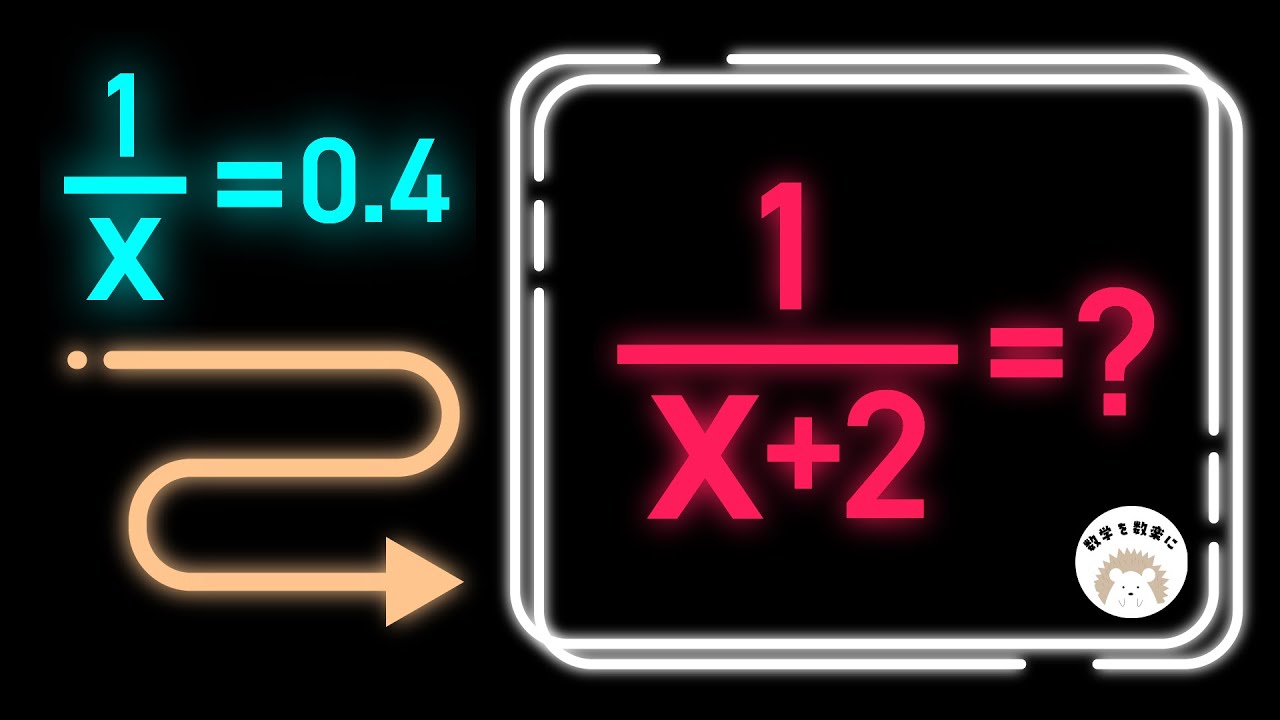問題文全文(内容文)：
$
(1)
\left\{
\begin{array}{l}
0.2x-0.3y=0.7x+0.4y-0.6 \\
6(5x+2y)=3x-2
\end{array}
\right.
$
$
(2)
\left\{
\begin{array}{l}
\displaystyle \frac{4}{3}x-\frac{3}{4}y=-14\\
0.3x-0.7y=-7.4
\end{array}
\right.
$
$
(3)
\left\{
\begin{array}{l}
\displaystyle \frac{5x+3y}{4}=\frac{x+5}{2}\\
\displaystyle \frac{4x-7y+3}{11}=2
\end{array}
\right.
$
$
(4)x-3y=5x+3y=4x-y+5
$
$(5)
\left\{
\begin{array}{l}
ax-by=1\\
bx-ay=8
\end{array}
\right.
$
の解が$(x,y)=(3,2)$のとき、定数$a,b$の値を求めよ
$
(1)
\left\{
\begin{array}{l}
0.2x-0.3y=0.7x+0.4y-0.6 \\
6(5x+2y)=3x-2
\end{array}
\right.
$
$
(2)
\left\{
\begin{array}{l}
\displaystyle \frac{4}{3}x-\frac{3}{4}y=-14\\
0.3x-0.7y=-7.4
\end{array}
\right.
$
$
(3)
\left\{
\begin{array}{l}
\displaystyle \frac{5x+3y}{4}=\frac{x+5}{2}\\
\displaystyle \frac{4x-7y+3}{11}=2
\end{array}
\right.
$
$
(4)x-3y=5x+3y=4x-y+5
$
$(5)
\left\{
\begin{array}{l}
ax-by=1\\
bx-ay=8
\end{array}
\right.
$
の解が$(x,y)=(3,2)$のとき、定数$a,b$の値を求めよ
単元：
#数学(中学生)#中２数学#連立方程式
指導講師：
【楽しい授業動画】あきとんとん
問題文全文(内容文)：
$
(1)
\left\{
\begin{array}{l}
0.2x-0.3y=0.7x+0.4y-0.6 \\
6(5x+2y)=3x-2
\end{array}
\right.
$
$
(2)
\left\{
\begin{array}{l}
\displaystyle \frac{4}{3}x-\frac{3}{4}y=-14\\
0.3x-0.7y=-7.4
\end{array}
\right.
$
$
(3)
\left\{
\begin{array}{l}
\displaystyle \frac{5x+3y}{4}=\frac{x+5}{2}\\
\displaystyle \frac{4x-7y+3}{11}=2
\end{array}
\right.
$
$
(4)x-3y=5x+3y=4x-y+5
$
$(5)
\left\{
\begin{array}{l}
ax-by=1\\
bx-ay=8
\end{array}
\right.
$
の解が$(x,y)=(3,2)$のとき、定数$a,b$の値を求めよ
$
(1)
\left\{
\begin{array}{l}
0.2x-0.3y=0.7x+0.4y-0.6 \\
6(5x+2y)=3x-2
\end{array}
\right.
$
$
(2)
\left\{
\begin{array}{l}
\displaystyle \frac{4}{3}x-\frac{3}{4}y=-14\\
0.3x-0.7y=-7.4
\end{array}
\right.
$
$
(3)
\left\{
\begin{array}{l}
\displaystyle \frac{5x+3y}{4}=\frac{x+5}{2}\\
\displaystyle \frac{4x-7y+3}{11}=2
\end{array}
\right.
$
$
(4)x-3y=5x+3y=4x-y+5
$
$(5)
\left\{
\begin{array}{l}
ax-by=1\\
bx-ay=8
\end{array}
\right.
$
の解が$(x,y)=(3,2)$のとき、定数$a,b$の値を求めよ
投稿日：2022.08.13
