18神奈川県教員採用試験（数学：11番 区分求積法） - 質問解決D.B.（データベース）
Generic selectors
完全一致検索
タイトルから検索
記事本文から検索
Post Type Selectors
all_unit_post
質問解決D.B.（データベース） > 動画投稿 > 数学(高校生) > 数Ⅲ > 積分とその応用 > 定積分 > 18神奈川県教員採用試験（数学：11番　区分求積法）

18神奈川県教員採用試験（数学：11番　区分求積法）

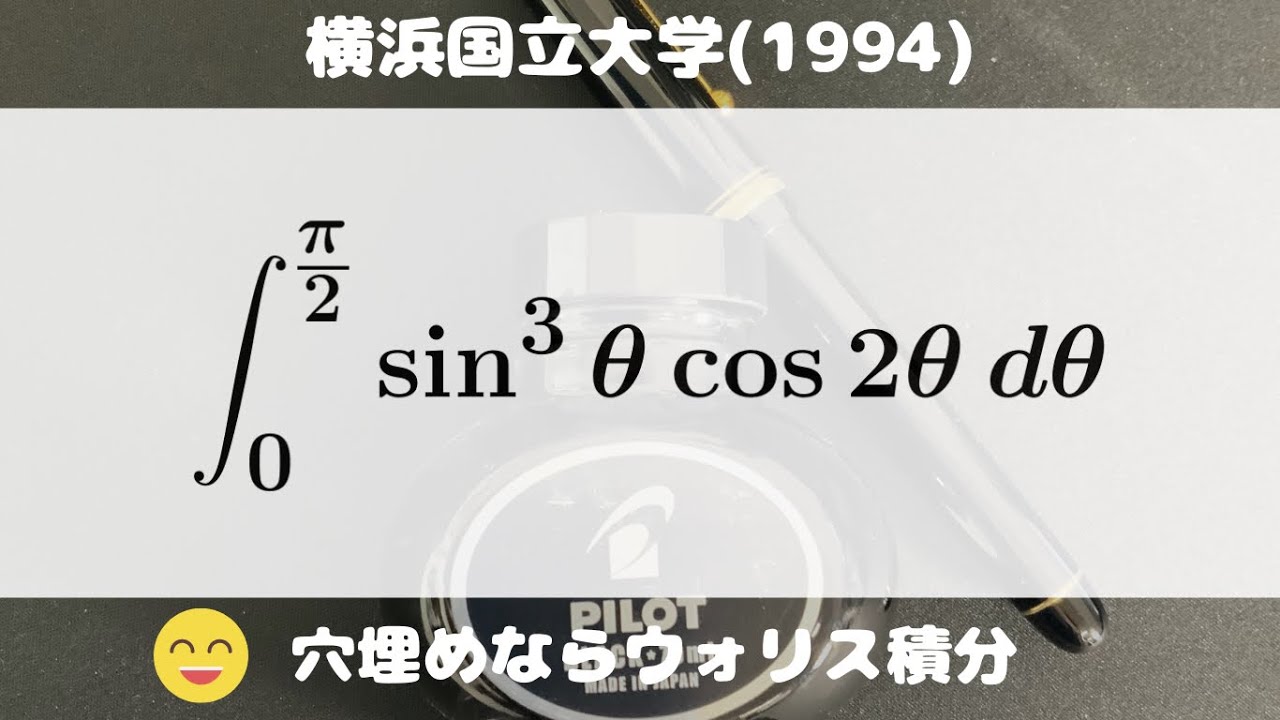
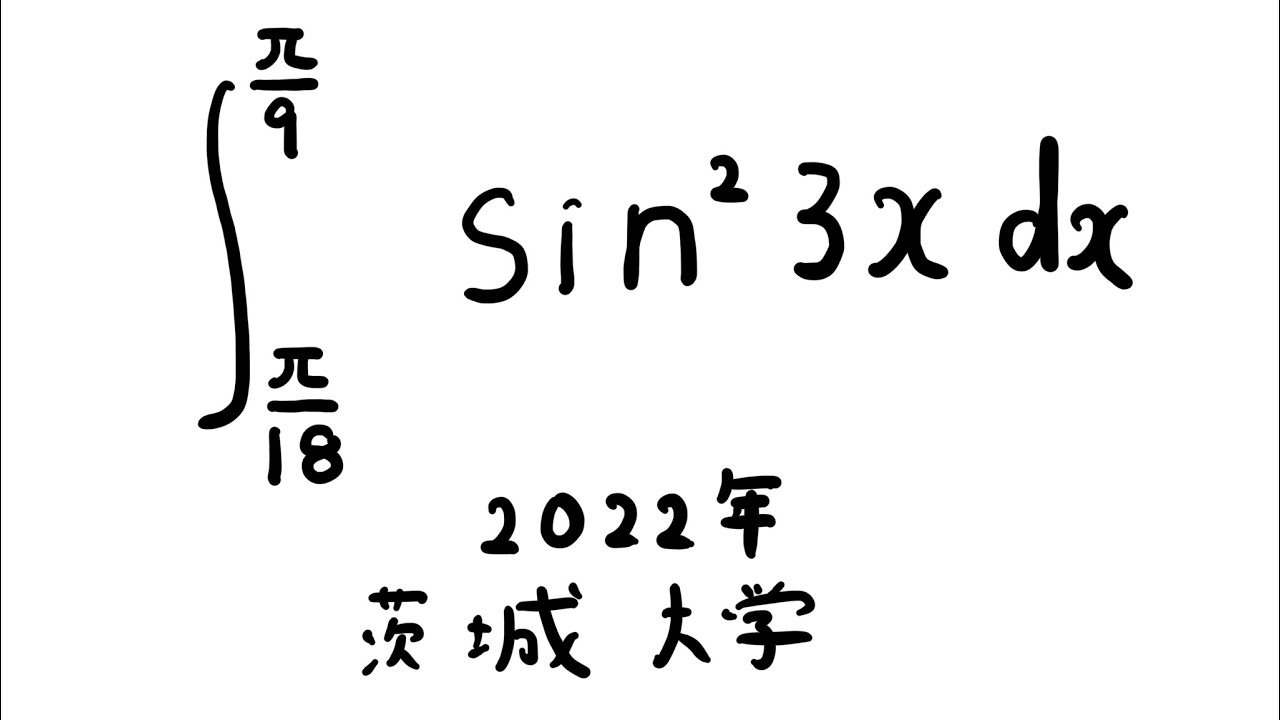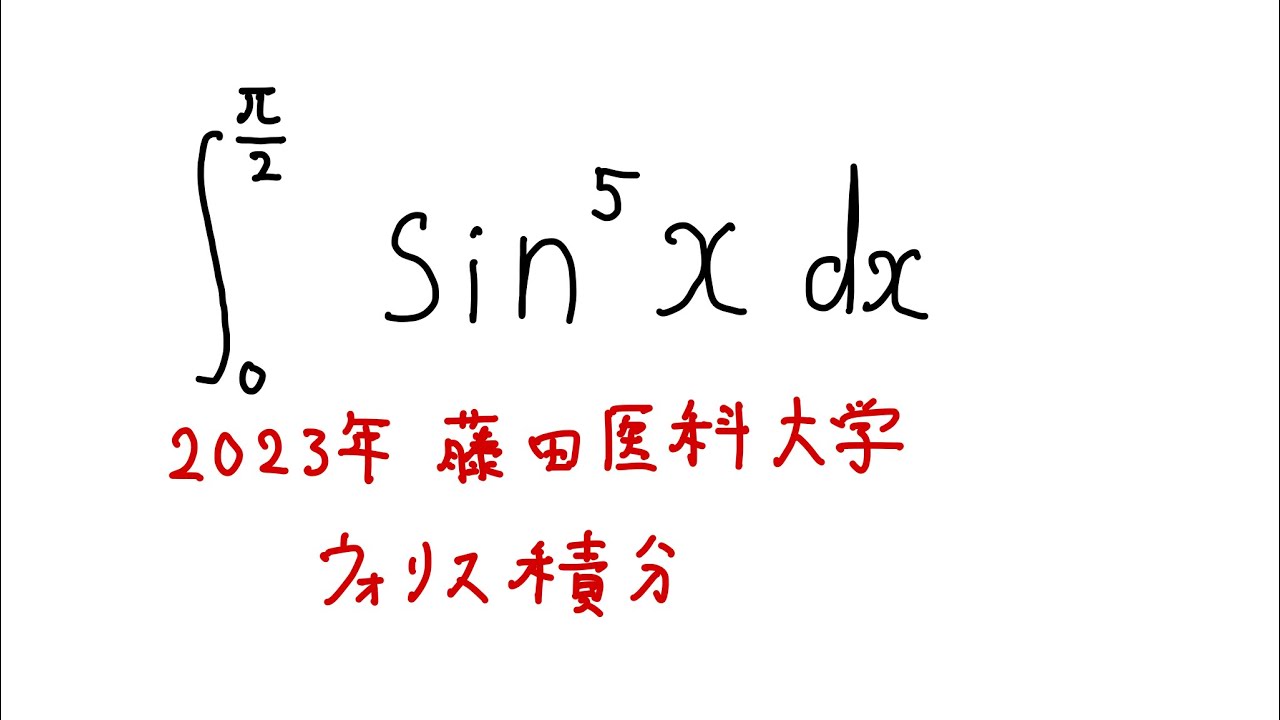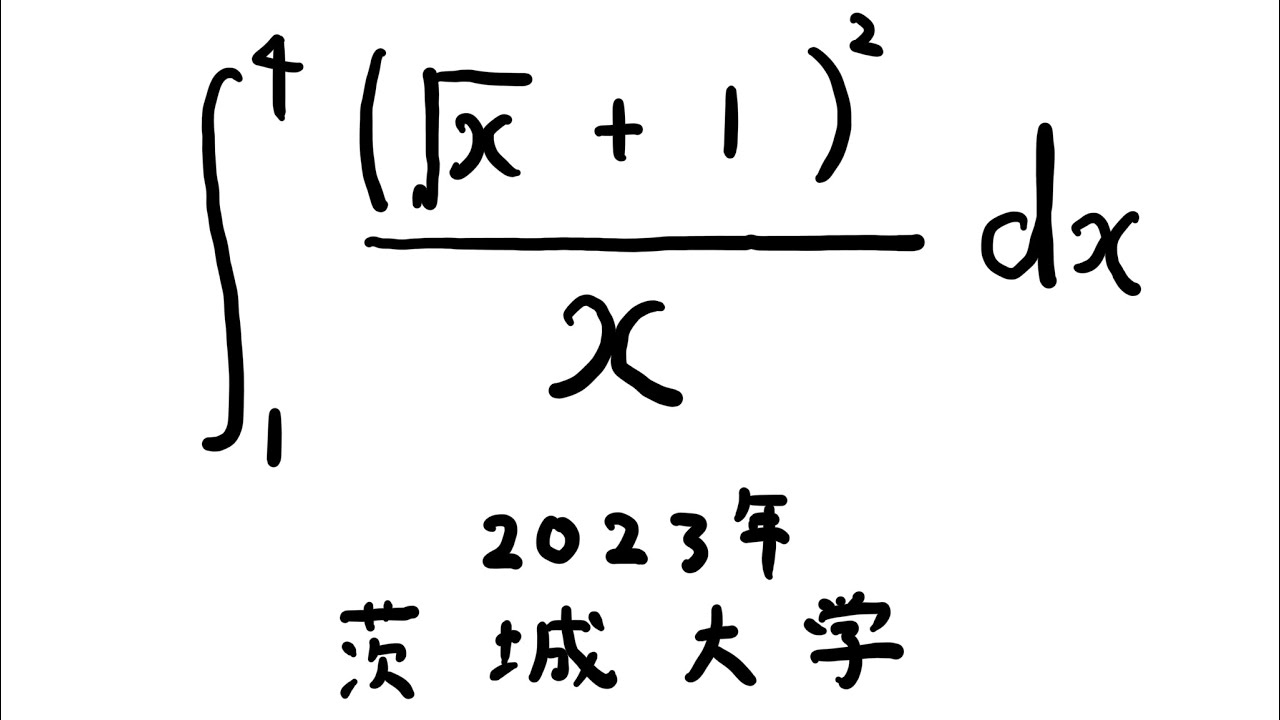問題文全文(内容文)：
$\boxed{11}$
$\displaystyle \lim_{ n \to m } \frac{1}{n} ( \sqrt{\frac{n+1}{n}} + \sqrt{\frac{n+2}{n}} + \cdots +\sqrt{\frac{n+n}{n}})$
単元： #積分とその応用#定積分#数Ⅲ
指導講師： ますただ
問題文全文(内容文)：
$\boxed{11}$
$\displaystyle \lim_{ n \to m } \frac{1}{n} ( \sqrt{\frac{n+1}{n}} + \sqrt{\frac{n+2}{n}} + \cdots +\sqrt{\frac{n+n}{n}})$
投稿日：2020.09.03

＜関連動画＞

福田の数学〜北里大学2022年医学部第２問〜定積分と不等式

アイキャッチ画像
単元： #大学入試過去問(数学)#微分とその応用#積分とその応用#関数の変化（グラフ・最大最小・方程式・不等式）#定積分#学校別大学入試過去問解説(数学)#北里大学#数学(高校生)#数Ⅲ
指導講師： 福田次郎
問題文全文(内容文)：
次の各問いに答えよ。
(1)定積分\int^1_0\frac{1}{1+x^2}dxを求めよ。
(2)$x≠0$を満たすすべての実数xに対して、$e^x \gt 1+x$と$e^{-x^2} \lt \frac{1}{1+x^2}$が
成り立つことを証明せよ。
(3)$\frac{2}{3} \lt \int^1_0e^{-x^2}dx \lt \frac{\pi}{4}$が成り立つことを証明せよ。

2022北里大学医学部過去問
この動画を見る　

大学入試問題#559「解法色々」　筑波大学(2020)　#定積分

アイキャッチ画像
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学#数Ⅲ
指導講師： ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{4}} \sin^2x\ \cos2x\ dx$

出典：2020年筑波大学　入試問題
この動画を見る　

東邦大学医学部(2011) #Shorts #King_property #キングプロパティ

アイキャッチ画像
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東邦大学
指導講師： ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{2}} \displaystyle \frac{\cos\ x}{\sin\ x+\cos\ x} dx$

出典：2011年東邦大学医学部　入試問題
この動画を見る　

【高校数学】毎日積分64日目~47都道府県制覇への道~【⑧福岡】【毎日17時投稿】

アイキャッチ画像
単元： #積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師： 理数個別チャンネル
問題文全文(内容文)：
$xy$平面上の曲線$C$を、媒介変数tを用いて次のように定める。
$x=t+2\sin^{2t}, y=t+\sin t (0\lt t\lt \pi)$
以下の問いに答えよ。
(1)曲線$C$に接する直線のうち$y$軸と平行なものがいくつあるか求めよ。
(2)曲線$C$のうち$y≦x$の領域にある部分と直線$y=x$で囲まれた図形の面積を求めよ。
【九州大学 2023】
この動画を見る　

#高専数学_12#定積分#元高専教員

アイキャッチ画像
単元： #数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師： ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1} \displaystyle \frac{1}{3x^2+1} dx$
この動画を見る　
PAGE TOP