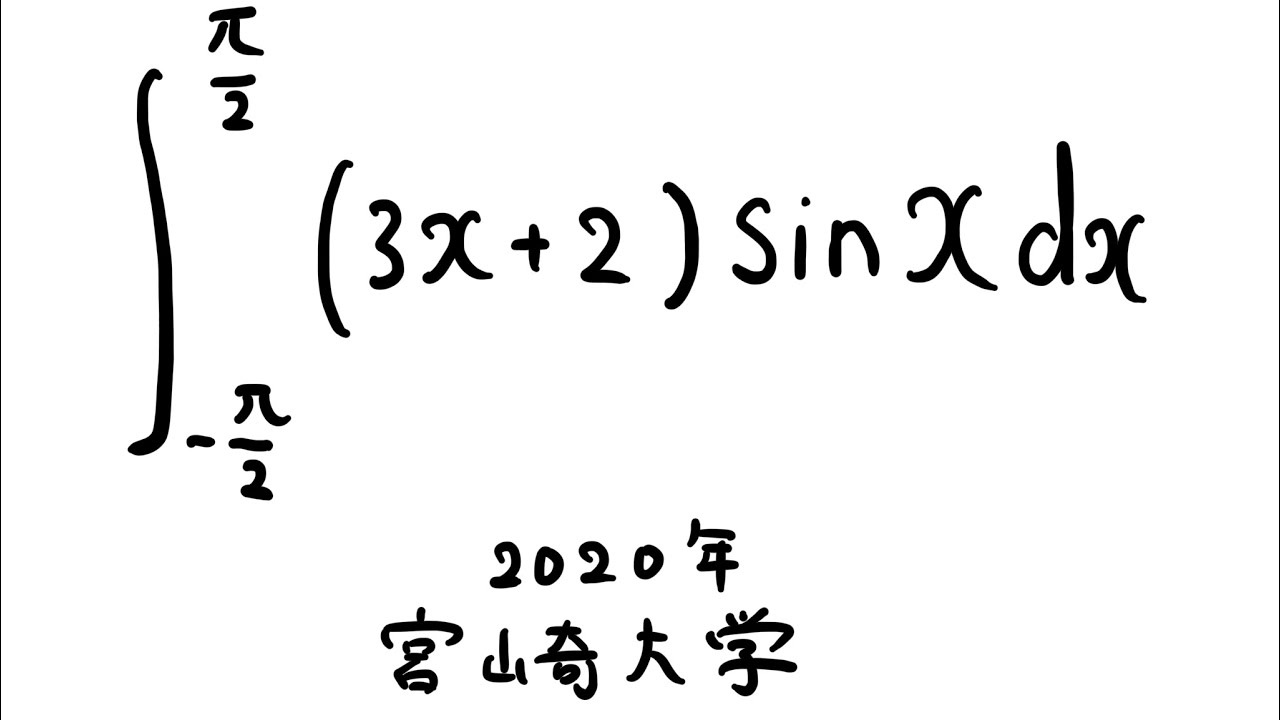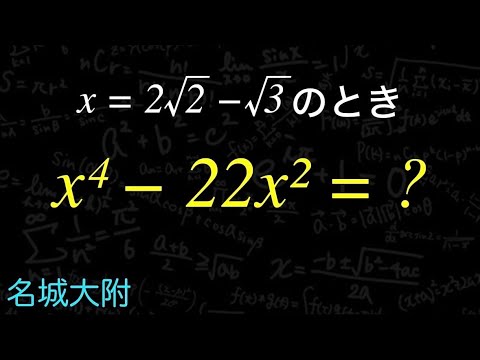問題文全文(内容文):
等式$f(x)=12x^2+6x\displaystyle \int_{0}^{1} f(t) dt+2\displaystyle \int_{0}^{1} tf(t)dt$を満たす関数$f(x)$を求めよ。
出典:2024年慶應義塾大学商学部 入試問題
等式$f(x)=12x^2+6x\displaystyle \int_{0}^{1} f(t) dt+2\displaystyle \int_{0}^{1} tf(t)dt$を満たす関数$f(x)$を求めよ。
出典:2024年慶應義塾大学商学部 入試問題
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
等式$f(x)=12x^2+6x\displaystyle \int_{0}^{1} f(t) dt+2\displaystyle \int_{0}^{1} tf(t)dt$を満たす関数$f(x)$を求めよ。
出典:2024年慶應義塾大学商学部 入試問題
等式$f(x)=12x^2+6x\displaystyle \int_{0}^{1} f(t) dt+2\displaystyle \int_{0}^{1} tf(t)dt$を満たす関数$f(x)$を求めよ。
出典:2024年慶應義塾大学商学部 入試問題
投稿日:2024.04.07