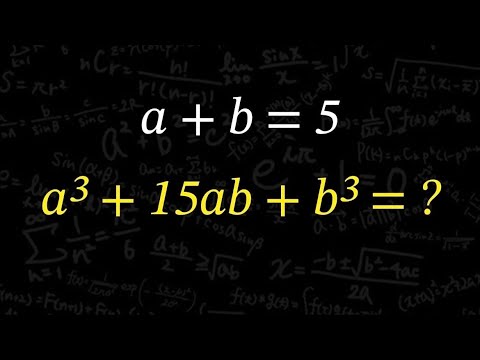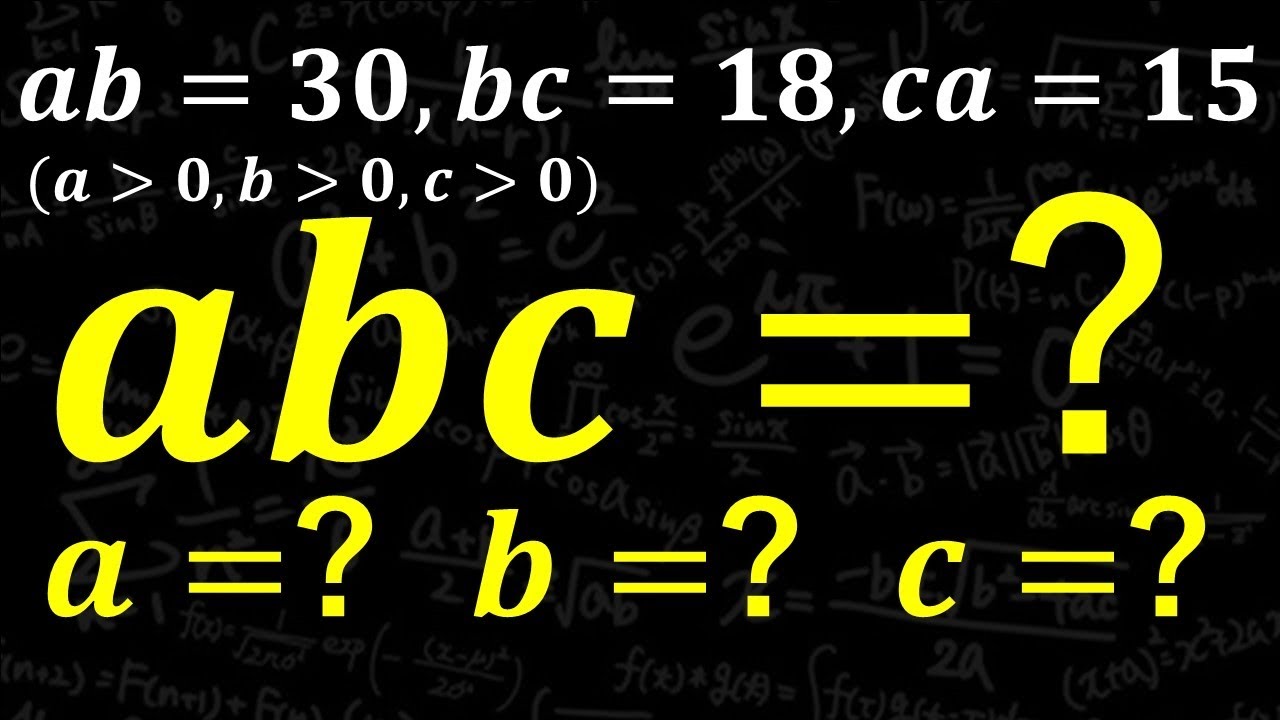問題文全文(内容文)：
◎$0° \leqq \theta \leqq 180°$のとき、次の等式を満たす$\theta$を求めよう。
①$\cos \theta=\displaystyle \frac{1}{\sqrt{ 2 }}$
②$\sin \theta=\sqrt{ 3 }$
③$\sqrt{ 3 } \tan \theta+1=0$
④$0° \leqq \theta \leqq 180°$とする。
$\sin \theta=\displaystyle \frac{4}{5}$のとき、$\cos \theta,\tan \theta$の値を求めよう。
◎$0° \leqq \theta \leqq 180°$のとき、次の等式を満たす$\theta$を求めよう。
①$\cos \theta=\displaystyle \frac{1}{\sqrt{ 2 }}$
②$\sin \theta=\sqrt{ 3 }$
③$\sqrt{ 3 } \tan \theta+1=0$
④$0° \leqq \theta \leqq 180°$とする。
$\sin \theta=\displaystyle \frac{4}{5}$のとき、$\cos \theta,\tan \theta$の値を求めよう。
単元：
#数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)
指導講師：
とある男が授業をしてみた
問題文全文(内容文)：
◎$0° \leqq \theta \leqq 180°$のとき、次の等式を満たす$\theta$を求めよう。
①$\cos \theta=\displaystyle \frac{1}{\sqrt{ 2 }}$
②$\sin \theta=\sqrt{ 3 }$
③$\sqrt{ 3 } \tan \theta+1=0$
④$0° \leqq \theta \leqq 180°$とする。
$\sin \theta=\displaystyle \frac{4}{5}$のとき、$\cos \theta,\tan \theta$の値を求めよう。
◎$0° \leqq \theta \leqq 180°$のとき、次の等式を満たす$\theta$を求めよう。
①$\cos \theta=\displaystyle \frac{1}{\sqrt{ 2 }}$
②$\sin \theta=\sqrt{ 3 }$
③$\sqrt{ 3 } \tan \theta+1=0$
④$0° \leqq \theta \leqq 180°$とする。
$\sin \theta=\displaystyle \frac{4}{5}$のとき、$\cos \theta,\tan \theta$の値を求めよう。
投稿日：2014.10.18
