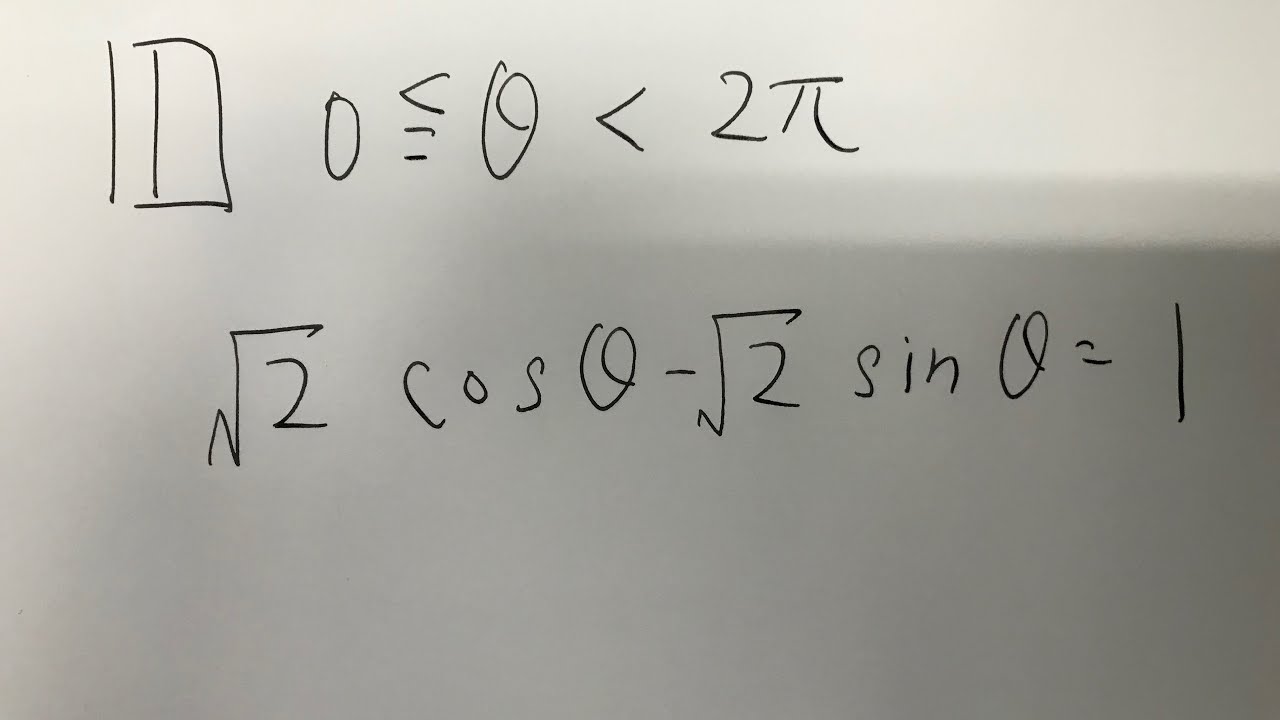問題文全文(内容文):
[1] (1)
(2)太郎さんと花子さんは、
以下では、
太郎:
花子:
着目してみようよ。
を満たす。よって、
太郎:
整数部分にだけ着目してもわからないな。
花子:
正の整数Nに着目してみたらどうかな。
が成り立つので、
[2]座標平面上の原点を中心とする半径1の円周上に3点
とする。このとき、
(1)
考察
この場合、
であり、加法定理により
である。同様に、
これらのことから、
⓪
①
②
③
④
⑤
②
③
考察2:
例えば、点
であるときを考える。このとき、
である。
ここで、三角関数の合成により
である。したがって
のとき、
(2)次に、
考察
この場合、
である。
同様に、
であるから、
という関係が得られる。
(3)これまでの考察を振り返ると、次の⓪~③のうち、
正しいものは
⓪
①
あっても
②
③
[1] (1)
(2)太郎さんと花子さんは、
以下では、
太郎:
花子:
着目してみようよ。
を満たす。よって、
太郎:
整数部分にだけ着目してもわからないな。
花子:
正の整数Nに着目してみたらどうかな。
が成り立つので、
[2]座標平面上の原点を中心とする半径1の円周上に3点
とする。このとき、
(1)
考察
この場合、
であり、加法定理により
である。同様に、
これらのことから、
⓪
①
②
③
④
⑤
②
③
考察2:
例えば、点
であるときを考える。このとき、
である。
ここで、三角関数の合成により
である。したがって
のとき、
(2)次に、
考察
この場合、
である。
同様に、
であるから、
という関係が得られる。
(3)これまでの考察を振り返ると、次の⓪~③のうち、
正しいものは
⓪
①
あっても
②
③
単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#指数関数と対数関数#三角関数とグラフ#加法定理とその応用#指数関数#対数関数#センター試験・共通テスト関連#共通テスト#数学(高校生)
指導講師:
福田次郎
問題文全文(内容文):
[1] (1)
(2)太郎さんと花子さんは、
以下では、
太郎:
花子:
着目してみようよ。
を満たす。よって、
太郎:
整数部分にだけ着目してもわからないな。
花子:
正の整数Nに着目してみたらどうかな。
が成り立つので、
[2]座標平面上の原点を中心とする半径1の円周上に3点
とする。このとき、
(1)
考察
この場合、
であり、加法定理により
である。同様に、
これらのことから、
⓪
①
②
③
④
⑤
②
③
考察2:
例えば、点
であるときを考える。このとき、
である。
ここで、三角関数の合成により
である。したがって
のとき、
(2)次に、
考察
この場合、
である。
同様に、
であるから、
という関係が得られる。
(3)これまでの考察を振り返ると、次の⓪~③のうち、
正しいものは
⓪
①
あっても
②
③
[1] (1)
(2)太郎さんと花子さんは、
以下では、
太郎:
花子:
着目してみようよ。
を満たす。よって、
太郎:
整数部分にだけ着目してもわからないな。
花子:
正の整数Nに着目してみたらどうかな。
が成り立つので、
[2]座標平面上の原点を中心とする半径1の円周上に3点
とする。このとき、
(1)
考察
この場合、
であり、加法定理により
である。同様に、
これらのことから、
⓪
①
②
③
④
⑤
②
③
考察2:
例えば、点
であるときを考える。このとき、
である。
ここで、三角関数の合成により
である。したがって
のとき、
(2)次に、
考察
この場合、
である。
同様に、
であるから、
という関係が得られる。
(3)これまでの考察を振り返ると、次の⓪~③のうち、
正しいものは
⓪
①
あっても
②
③
投稿日:2021.02.01