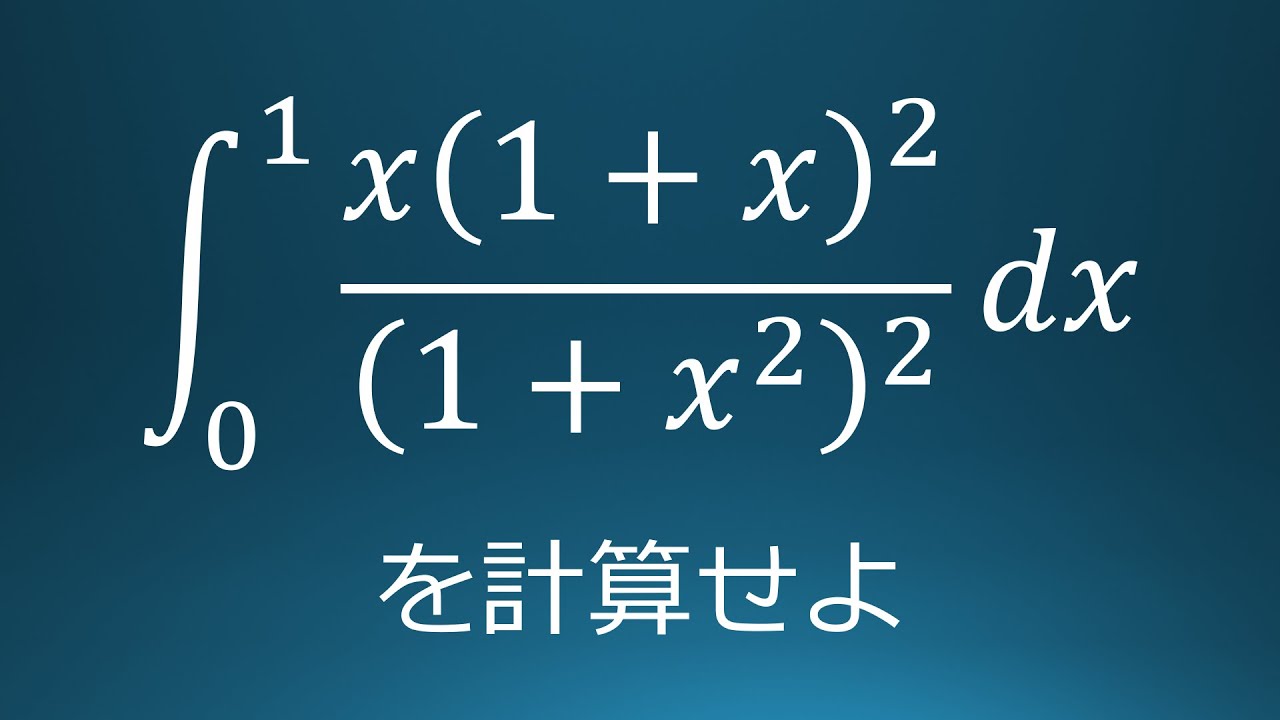大学入試問題#343「計算のクセが強すぎる」防衛大学校2013 #定積分

大学入試問題#343「計算のクセが強すぎる」　防衛大学校2013　#定積分

問題文全文(内容文)：

^{\forall} t \in R,

\sin 3 t = f (\sin t)

\int_{0}^{1} {f (x)}^{2} \sqrt{1 - x^{2}} d x

出典：2013年防衛大学校　入試問題

チャプター：

00:00 問題紹介
00:14 本編スタート
05:35 作成した解答①
05:46 作成した解答②
05:58 エンディング（音楽提供：兄いえてぃさん）

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#防衛大学校#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

^{\forall} t \in R,

\sin 3 t = f (\sin t)

\int_{0}^{1} {f (x)}^{2} \sqrt{1 - x^{2}} d x

出典：2013年防衛大学校　入試問題

投稿日：2022.10.21

＜関連動画＞

大学入試問題#796「解法は、ほぼ1択か」　#横浜国立大学(2024) #定積分

単元： #数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#横浜国立大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{l o g \sqrt{3}} \frac{e^{3 x} + 4 e^{2 x} + e^{x}}{e^{4 x} + 2 e^{2 x} + 1} d x

出典：2024年横浜国立大学

この動画を見る　

【高校数学】毎日積分35日目【バウムクーヘン積分】【毎日17時投稿】

単元： #積分とその応用#定積分#数学(高校生)#数Ⅲ

指導講師：理数個別チャンネル

問題文全文(内容文)：
今回は共通テスト直後ということで、バウムクーヘン積分について解説します.

この動画を見る　

大学入試問題#616「これは理系が解くと逆にはまるかも」　名古屋大学(1963)

単元： #数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#名古屋大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

x > y

とする

x + y = 6, x y = 4

のとき

\frac{\sqrt{x} - \sqrt{y}}{\sqrt{x} + \sqrt{y}}

の値を求めよ。

出典：1963年名古屋大学　入試問題

この動画を見る　

#福島大学2024#定積分_4#元高校教員

単元： #数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#福島大学

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{1} x \sqrt{1 - x}

d x

出典：2024年福島大学

この動画を見る　

大学入試問題#130　東海大学医学部(2016)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東海大学

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{1} \frac{x (1 + x)^{2}}{(1 + x^{2})^{2}} d x

を計算せよ。

出典：2016年東海大学医学部　入試問題

この動画を見る　

<img src="https://kaiketsu-db.net/wp-content/uploads/2021/11/112-book-morph-outline.gif" alt="" data-eio="l"> 大学入試問題#343「計算のクセが強すぎる」 防衛大学校2013 #定積分

＜関連動画＞

大学入試問題#796「解法は、ほぼ1択か」 #横浜国立大学(2024) #定積分

【高校数学】毎日積分35日目【バウムクーヘン積分】【毎日17時投稿】

大学入試問題#616「これは理系が解くと逆にはまるかも」 名古屋大学(1963)

#福島大学2024#定積分_4#元高校教員

大学入試問題#130 東海大学医学部(2016) 定積分