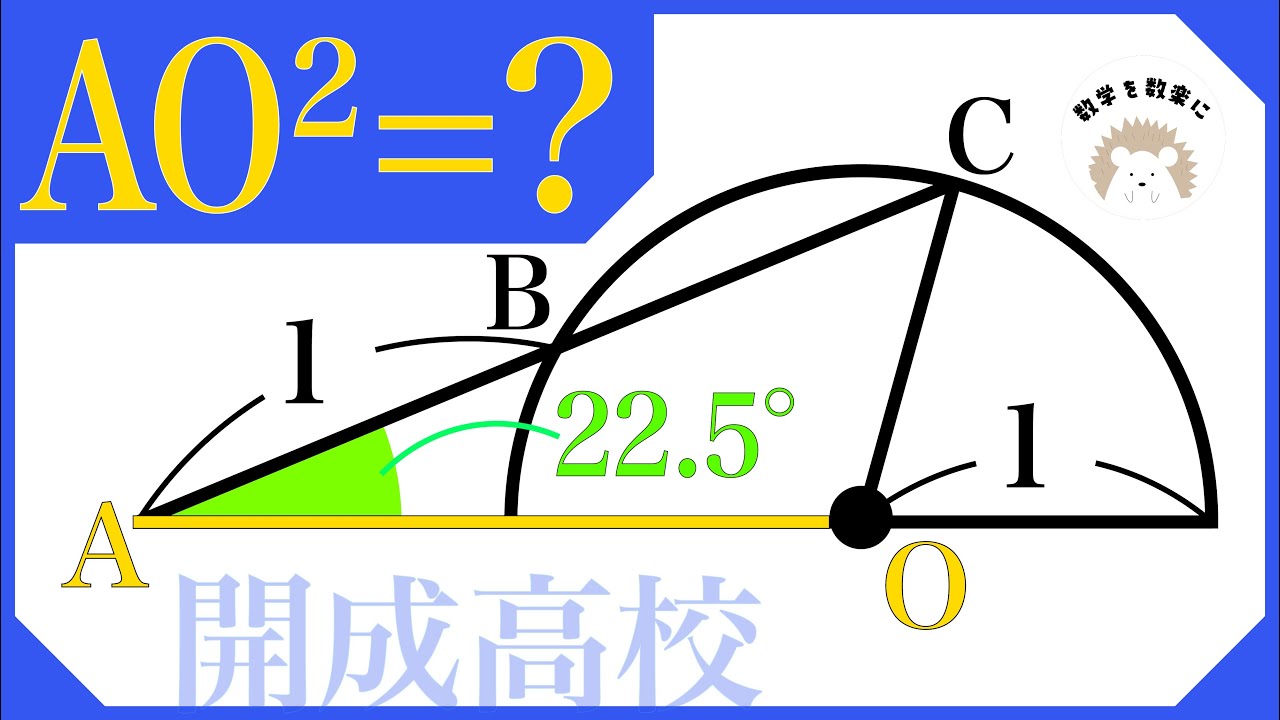
問題文全文(内容文)：
$y=2 \sqrt{ 3 }(x- \cos \theta)^2+ \sin \theta$
$y=-2 \sqrt{ 3 }(x+ \cos \theta)^2- \sin \theta$
この2つの放物線が相違となる2点で交わるような$\theta$の範囲
出典：2002年東京大学 過去問
$y=2 \sqrt{ 3 }(x- \cos \theta)^2+ \sin \theta$
$y=-2 \sqrt{ 3 }(x+ \cos \theta)^2- \sin \theta$
この2つの放物線が相違となる2点で交わるような$\theta$の範囲
出典：2002年東京大学 過去問
単元：
#数Ⅰ#数Ⅱ#大学入試過去問(数学)#２次関数#図形と計量#2次関数とグラフ#三角比（三角比・拡張・相互関係・単位円）#三角比への応用（正弦・余弦・面積）#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)
指導講師：
鈴木貫太郎
問題文全文(内容文)：
$y=2 \sqrt{ 3 }(x- \cos \theta)^2+ \sin \theta$
$y=-2 \sqrt{ 3 }(x+ \cos \theta)^2- \sin \theta$
この2つの放物線が相違となる2点で交わるような$\theta$の範囲
出典：2002年東京大学 過去問
$y=2 \sqrt{ 3 }(x- \cos \theta)^2+ \sin \theta$
$y=-2 \sqrt{ 3 }(x+ \cos \theta)^2- \sin \theta$
この2つの放物線が相違となる2点で交わるような$\theta$の範囲
出典：2002年東京大学 過去問
投稿日：2019.02.01
