問題文全文(内容文):

(1)f(x)の最大値
(2)

(1)f(x)の最大値
(2)
単元:
#関数と極限#微分とその応用#積分とその応用#関数の極限#関数の変化(グラフ・最大最小・方程式・不等式)#定積分#その他#数学(高校生)#数Ⅲ#教員採用試験
指導講師:
ますただ
問題文全文(内容文):

(1)f(x)の最大値
(2)

(1)f(x)の最大値
(2)
投稿日:2020.12.08


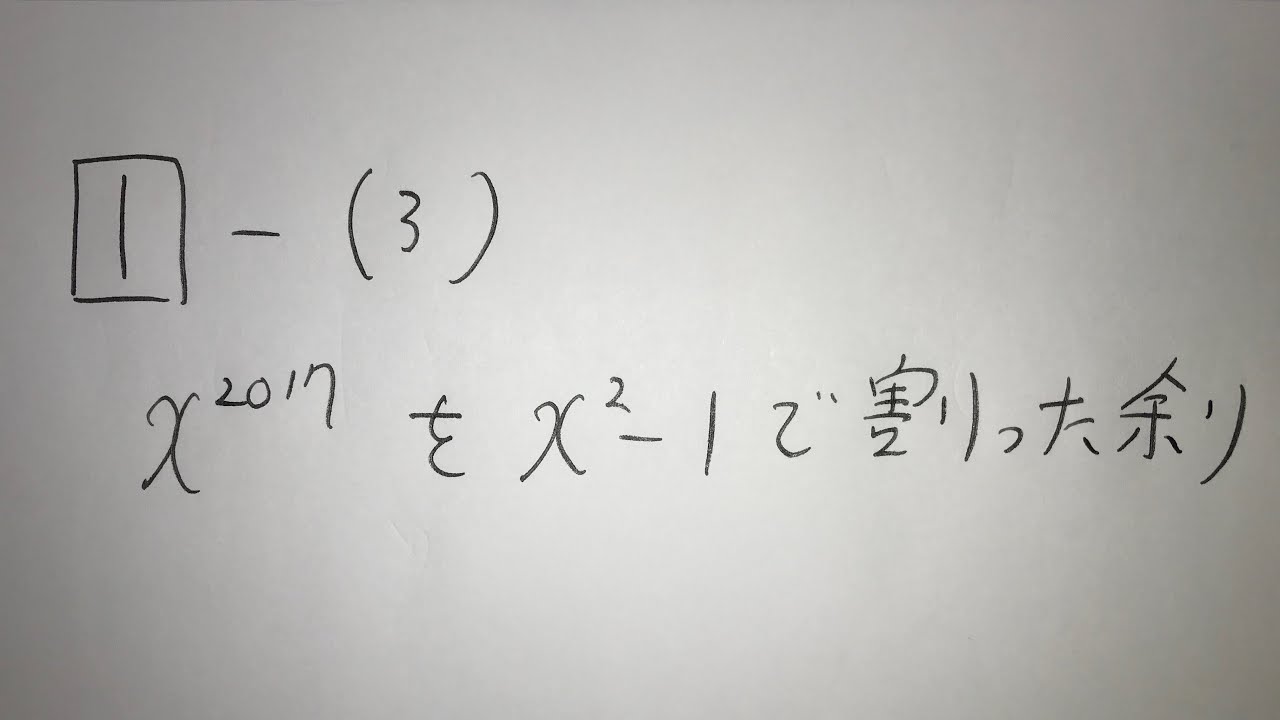
 -(3)
-(3)



