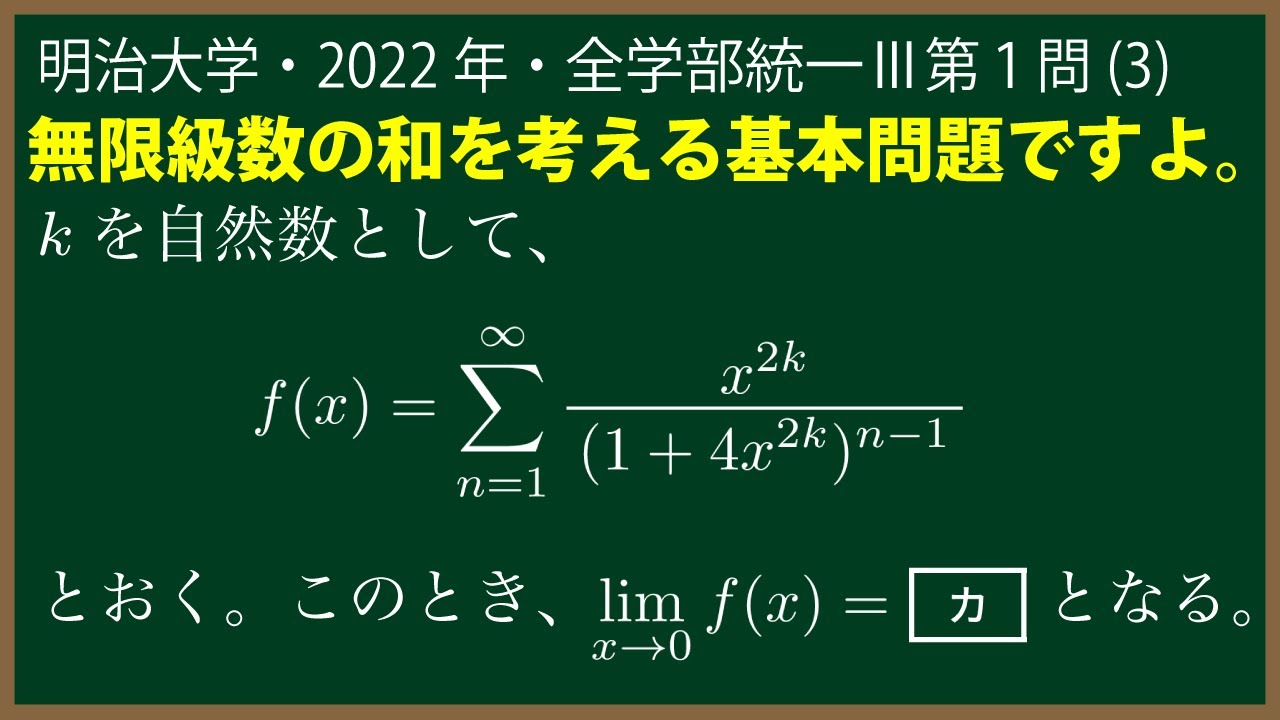
問題文全文(内容文):
出典:2019年九州大学 過去問
出典:2019年九州大学 過去問
単元:
#大学入試過去問(数学)#関数と極限#積分とその応用#関数の極限#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#九州大学#数Ⅲ
指導講師:
鈴木貫太郎
問題文全文(内容文):
出典:2019年九州大学 過去問
出典:2019年九州大学 過去問
投稿日:2019.09.02