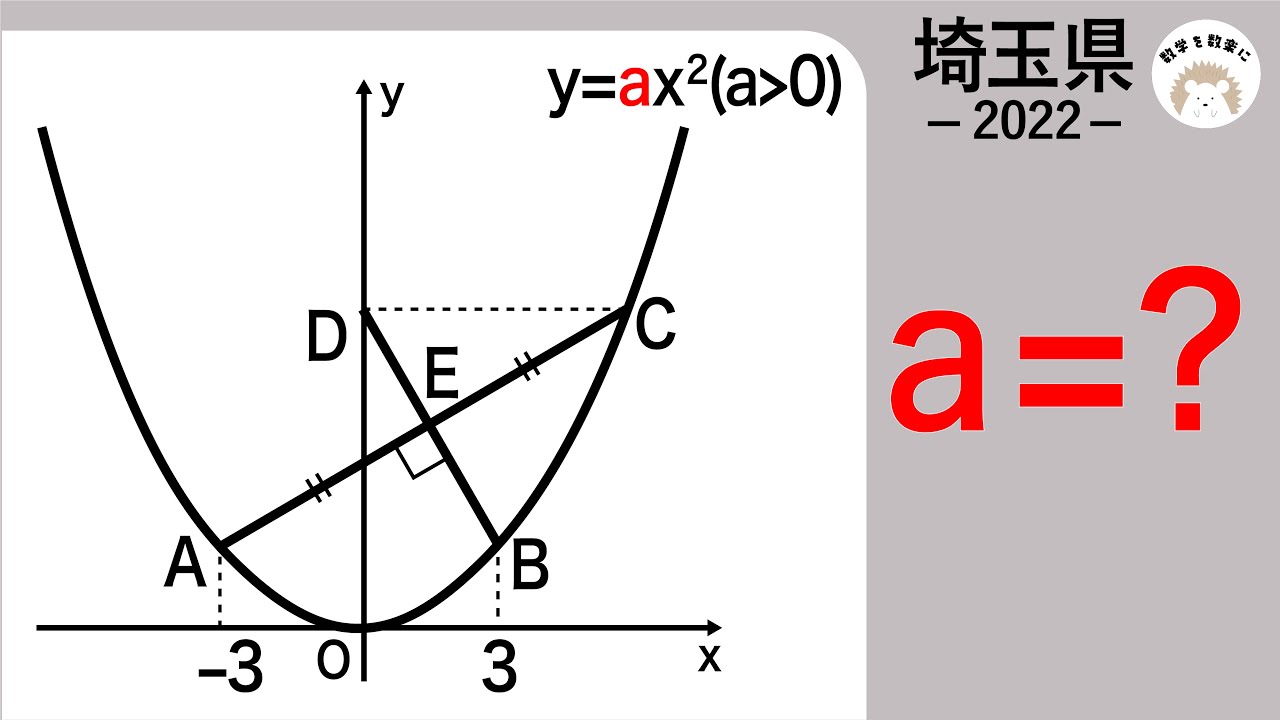
問題文全文(内容文)：
（1）
$0^{ \circ } \lt \theta \lt 180^{ \circ }$
$\tan \theta =-2$
$\sin \theta,\cos \theta$は？
（2）
$0 \leqq \theta \lt 2 \pi$
$\cos \theta \lt \displaystyle \frac{\sqrt{ 3 }}{2}$を解け
（3）
$0 \lt \theta \leqq 2 \pi$
$\sin \theta \geqq \displaystyle \frac{1}{2}$を解け
（4）
$0 \leqq \theta \lt 2 \pi$
$\sin \theta + \sqrt{ 3 } \cos \theta =\sqrt{ 2 }$を解け
（5）
$0 \leqq x \leqq \pi$とする
$y=2 \sin 2x-2(\sin x- \cos x)+1$
のとり得る値の範囲は？
（6）
$f(x)=\sin x - \cos 2x$の
$0 \leqq x \leqq \pi$における
max、minを求めよ
（1）
$0^{ \circ } \lt \theta \lt 180^{ \circ }$
$\tan \theta =-2$
$\sin \theta,\cos \theta$は？
（2）
$0 \leqq \theta \lt 2 \pi$
$\cos \theta \lt \displaystyle \frac{\sqrt{ 3 }}{2}$を解け
（3）
$0 \lt \theta \leqq 2 \pi$
$\sin \theta \geqq \displaystyle \frac{1}{2}$を解け
（4）
$0 \leqq \theta \lt 2 \pi$
$\sin \theta + \sqrt{ 3 } \cos \theta =\sqrt{ 2 }$を解け
（5）
$0 \leqq x \leqq \pi$とする
$y=2 \sin 2x-2(\sin x- \cos x)+1$
のとり得る値の範囲は？
（6）
$f(x)=\sin x - \cos 2x$の
$0 \leqq x \leqq \pi$における
max、minを求めよ
単元：
#三角関数#三角関数とグラフ#加法定理とその応用#数学(高校生)
指導講師：
カサニマロ【べんとう・ふきのとうの授業動画】
問題文全文(内容文)：
（1）
$0^{ \circ } \lt \theta \lt 180^{ \circ }$
$\tan \theta =-2$
$\sin \theta,\cos \theta$は？
（2）
$0 \leqq \theta \lt 2 \pi$
$\cos \theta \lt \displaystyle \frac{\sqrt{ 3 }}{2}$を解け
（3）
$0 \lt \theta \leqq 2 \pi$
$\sin \theta \geqq \displaystyle \frac{1}{2}$を解け
（4）
$0 \leqq \theta \lt 2 \pi$
$\sin \theta + \sqrt{ 3 } \cos \theta =\sqrt{ 2 }$を解け
（5）
$0 \leqq x \leqq \pi$とする
$y=2 \sin 2x-2(\sin x- \cos x)+1$
のとり得る値の範囲は？
（6）
$f(x)=\sin x - \cos 2x$の
$0 \leqq x \leqq \pi$における
max、minを求めよ
（1）
$0^{ \circ } \lt \theta \lt 180^{ \circ }$
$\tan \theta =-2$
$\sin \theta,\cos \theta$は？
（2）
$0 \leqq \theta \lt 2 \pi$
$\cos \theta \lt \displaystyle \frac{\sqrt{ 3 }}{2}$を解け
（3）
$0 \lt \theta \leqq 2 \pi$
$\sin \theta \geqq \displaystyle \frac{1}{2}$を解け
（4）
$0 \leqq \theta \lt 2 \pi$
$\sin \theta + \sqrt{ 3 } \cos \theta =\sqrt{ 2 }$を解け
（5）
$0 \leqq x \leqq \pi$とする
$y=2 \sin 2x-2(\sin x- \cos x)+1$
のとり得る値の範囲は？
（6）
$f(x)=\sin x - \cos 2x$の
$0 \leqq x \leqq \pi$における
max、minを求めよ
投稿日：2022.09.03
