 三角比(三角比・拡張・相互関係・単位円)
三角比(三角比・拡張・相互関係・単位円)
 三角比(三角比・拡張・相互関係・単位円)
三角比(三角比・拡張・相互関係・単位円)
【高校数学】三角比①~三角比とは横顔??~ 3-1【数学Ⅰ】

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
【楽しい授業動画】あきとんとん
問題文全文(内容文):
木の根もとから水平に10m離れた地点で木の先端の仰角を測ったところ、
28°であった。
目の高さを1.6mとして、木の高さを求めよ。
この動画を見る
木の根もとから水平に10m離れた地点で木の先端の仰角を測ったところ、
28°であった。
目の高さを1.6mとして、木の高さを求めよ。
名古屋大 円の方程式 2円と直線に接する円 高校数学 Japanese university entrance exam questions

単元:
#数Ⅰ#数A#大学入試過去問(数学)#図形の性質#図形と計量#三角比(三角比・拡張・相互関係・単位円)#周角と円に内接する四角形・円と接線・接弦定理#数学(高校生)#名古屋大学
指導講師:
鈴木貫太郎
問題文全文(内容文):
'08名古屋大学過去問題
2つの円、$x^2+(y-2)^2=9$と$(x-4)^2+(y+4)^2=1$に外接し、x=6と接する円を求めよ。
この動画を見る
'08名古屋大学過去問題
2つの円、$x^2+(y-2)^2=9$と$(x-4)^2+(y+4)^2=1$に外接し、x=6と接する円を求めよ。
和歌山大 三項間漸化式 半角の公式 高校数学 Japanese university entrance exam questions
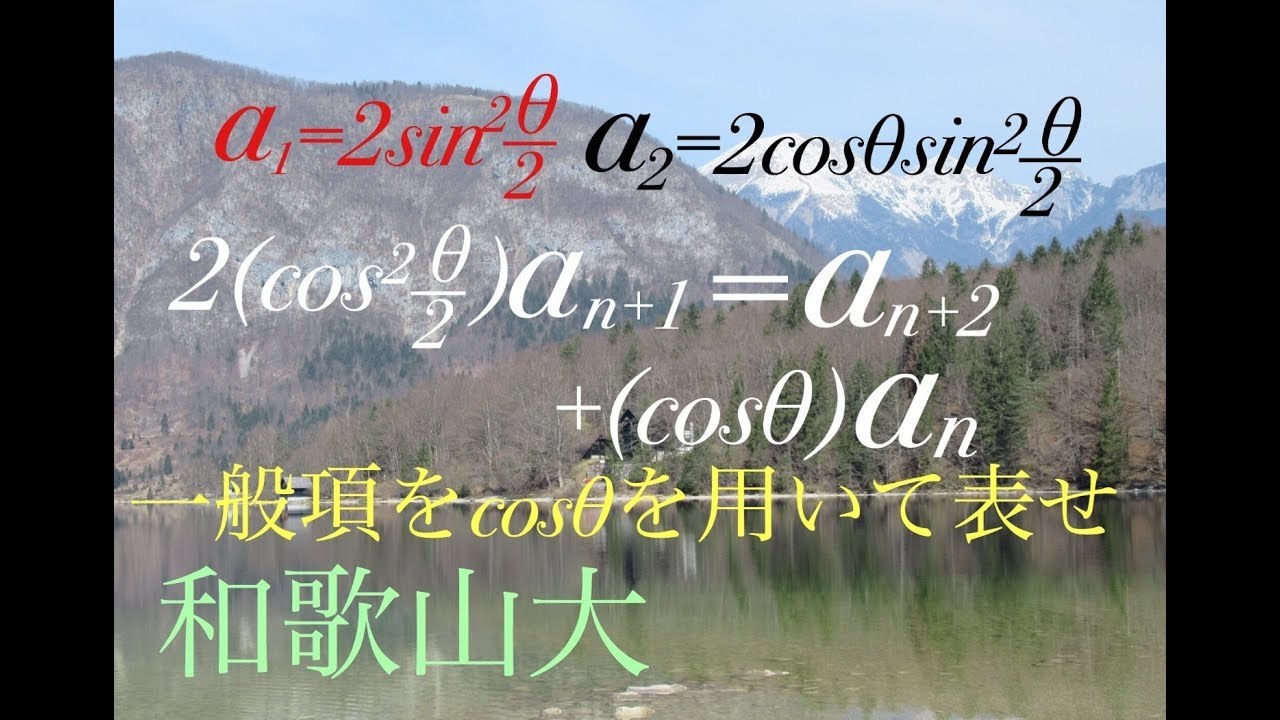
単元:
#数Ⅰ#数Ⅱ#大学入試過去問(数学)#図形と計量#三角比(三角比・拡張・相互関係・単位円)#三角比への応用(正弦・余弦・面積)#三角関数#三角関数とグラフ#数列#数列とその和(等差・等比・階差・Σ)#数学(高校生)#和歌山大学#数B
指導講師:
鈴木貫太郎
問題文全文(内容文):
和歌山大学過去問題
$a_1=2\sin^2\frac{θ}{2}$,$a_2=2\cosθ\sin^2\frac{θ}{2}$
$2(cos^2\frac{θ}{2})a_{n+1}=a_{n+2}+(\cosθ)a_n$
$a_n$を$\cosθ$を用いて表せ。
この動画を見る
和歌山大学過去問題
$a_1=2\sin^2\frac{θ}{2}$,$a_2=2\cosθ\sin^2\frac{θ}{2}$
$2(cos^2\frac{θ}{2})a_{n+1}=a_{n+2}+(\cosθ)a_n$
$a_n$を$\cosθ$を用いて表せ。
【高校数学】 数Ⅰ-92 三角形となる条件

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎3辺の長さが、5,3,xである三角形が鈍角三角形となるように、xの範囲を定めよう。
この動画を見る
◎3辺の長さが、5,3,xである三角形が鈍角三角形となるように、xの範囲を定めよう。
【高校数学】 数Ⅰ-85 三角比⑩
単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
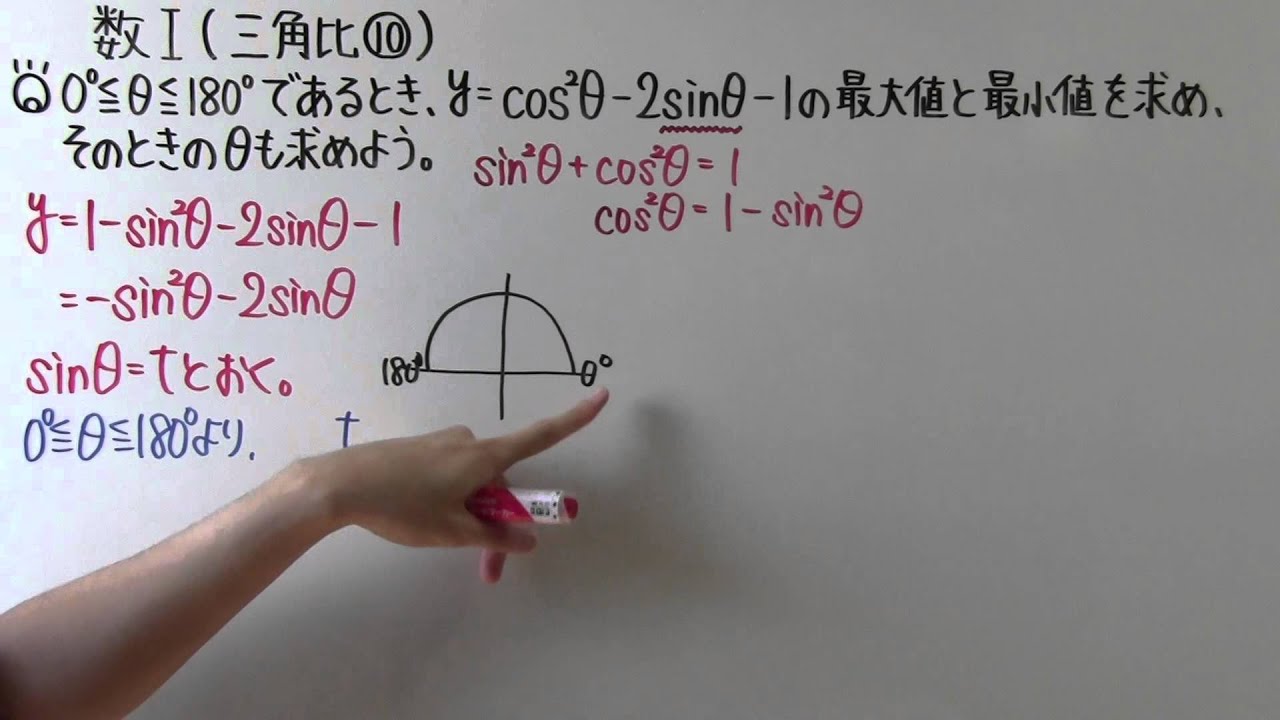
$0° \leqq \theta \leqq 180°$であるとき、$y=\cos^2\theta-2\sin\theta-1$の最大値と最小値を求め、そのときの$\theta$も求めよう。
この動画を見る
$0° \leqq \theta \leqq 180°$であるとき、$y=\cos^2\theta-2\sin\theta-1$の最大値と最小値を求め、そのときの$\theta$も求めよう。
【高校数学】 数Ⅰ-84 三角比⑨

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
$0° \leqq \theta \leqq 180°$とする。次の不等式を満たす
$\theta $の範囲を求めよう。
①$\sin \theta \gt \displaystyle \frac{\sqrt{ 3 }}{2}$
②$\cos \theta \lt \displaystyle \frac{1}{2}$
③$\tan \theta \geqq \sqrt{ 3 }$
④$2\sin \theta-1\leqq0$
⑤$2\cos \theta+ \sqrt{ 3 } \gt 0$
⑥$\tan \theta +1 \geqq 0$
この動画を見る
$0° \leqq \theta \leqq 180°$とする。次の不等式を満たす
$\theta $の範囲を求めよう。
①$\sin \theta \gt \displaystyle \frac{\sqrt{ 3 }}{2}$
②$\cos \theta \lt \displaystyle \frac{1}{2}$
③$\tan \theta \geqq \sqrt{ 3 }$
④$2\sin \theta-1\leqq0$
⑤$2\cos \theta+ \sqrt{ 3 } \gt 0$
⑥$\tan \theta +1 \geqq 0$
【高校数学】 数Ⅰ-83 三角比⑧

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎$0° \leqq \theta \leqq 180°,\sin \theta+\cos \theta=\displaystyle \frac{1}{2}$のとき、次の式の値を求めよう。
①$\sin \theta\cos \theta$
②$\sin^3 \theta+\cos^3 \theta$
③$\sin \theta-\cos \theta$
この動画を見る
◎$0° \leqq \theta \leqq 180°,\sin \theta+\cos \theta=\displaystyle \frac{1}{2}$のとき、次の式の値を求めよう。
①$\sin \theta\cos \theta$
②$\sin^3 \theta+\cos^3 \theta$
③$\sin \theta-\cos \theta$
【高校数学】 数Ⅰ-82 三角比⑦

単元:
#数Ⅰ#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎次の式のとりうる値の範囲を求めよう。
①$\cos \theta+2(0° \leqq \theta \leqq 180°)$
②$3\sin \theta-1(0° \leqq \theta \leqq 180°)$
③$\sqrt{ 2 }\sin \theta+3(45° \leqq \theta \leqq 120°)$
④$\sqrt{ 3 }\tan \theta-3(30° \leqq \theta \lt 60°)$
この動画を見る
◎次の式のとりうる値の範囲を求めよう。
①$\cos \theta+2(0° \leqq \theta \leqq 180°)$
②$3\sin \theta-1(0° \leqq \theta \leqq 180°)$
③$\sqrt{ 2 }\sin \theta+3(45° \leqq \theta \leqq 120°)$
④$\sqrt{ 3 }\tan \theta-3(30° \leqq \theta \lt 60°)$
【高校数学】 数Ⅰ-81 三角比⑥

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎$0° \leqq \theta \leqq 180°$のとき、次の等式を満たす$\theta$を求めよう。
①$\cos \theta=\displaystyle \frac{1}{\sqrt{ 2 }}$
②$\sin \theta=\sqrt{ 3 }$
③$\sqrt{ 3 } \tan \theta+1=0$
④$0° \leqq \theta \leqq 180°$とする。
$\sin \theta=\displaystyle \frac{4}{5}$のとき、$\cos \theta,\tan \theta$の値を求めよう。
この動画を見る
◎$0° \leqq \theta \leqq 180°$のとき、次の等式を満たす$\theta$を求めよう。
①$\cos \theta=\displaystyle \frac{1}{\sqrt{ 2 }}$
②$\sin \theta=\sqrt{ 3 }$
③$\sqrt{ 3 } \tan \theta+1=0$
④$0° \leqq \theta \leqq 180°$とする。
$\sin \theta=\displaystyle \frac{4}{5}$のとき、$\cos \theta,\tan \theta$の値を求めよう。
【高校数学】 数Ⅰ-78 三角比③
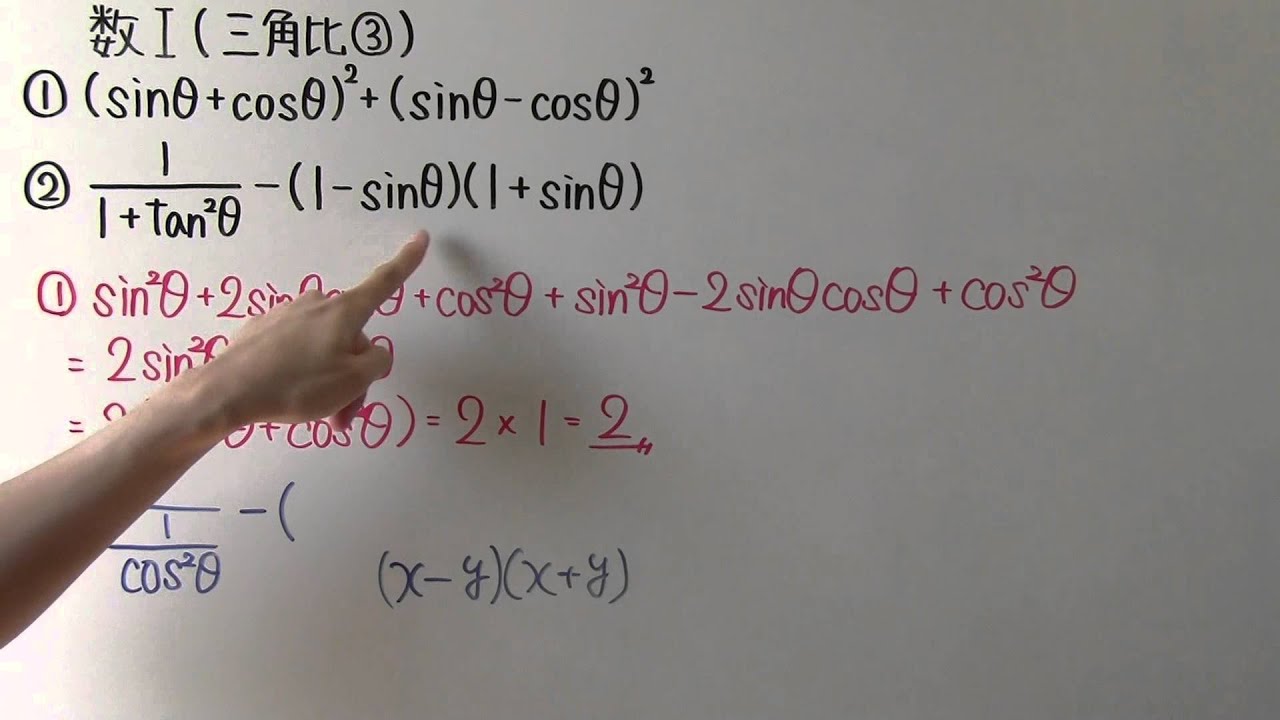
単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
計算してみよう。
①$(\sin \theta+\cos \theta)^2+(\sin \theta-\cos \theta)^2$
②$\displaystyle \frac{1}{1+\tan^2 \theta}-(1-\sin \theta)(1+\sin \theta)$
この動画を見る
計算してみよう。
①$(\sin \theta+\cos \theta)^2+(\sin \theta-\cos \theta)^2$
②$\displaystyle \frac{1}{1+\tan^2 \theta}-(1-\sin \theta)(1+\sin \theta)$
【高校数学】 数Ⅰ-77 三角比② ・ 公式編

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
$0° \lt \theta \lt 90°$のとき
$\sin (90°-\theta)=$①____
$\cos(90°-\theta)=$②____
$\tan(90°-\theta)=$③____
$\tan \theta=$④____
$\sin^2 \theta+\cos^2 \theta=$⑤____
$1+\tan^2 \theta=$⑥____
◎次の三角比を45°以下の角の三角比で表そう。
⑦$\sin56°=$
⑧$\cos79°=$
⑨$\tan62°=$
⑩$\sin \theta=\displaystyle \frac{1}{\sqrt{ 5 }}$のとき、$\cos \theta,\tan \theta$の値を求めよう。ただし、$\theta$は鋭角とする。
この動画を見る
$0° \lt \theta \lt 90°$のとき
$\sin (90°-\theta)=$①____
$\cos(90°-\theta)=$②____
$\tan(90°-\theta)=$③____
$\tan \theta=$④____
$\sin^2 \theta+\cos^2 \theta=$⑤____
$1+\tan^2 \theta=$⑥____
◎次の三角比を45°以下の角の三角比で表そう。
⑦$\sin56°=$
⑧$\cos79°=$
⑨$\tan62°=$
⑩$\sin \theta=\displaystyle \frac{1}{\sqrt{ 5 }}$のとき、$\cos \theta,\tan \theta$の値を求めよう。ただし、$\theta$は鋭角とする。
