ますただ

※下の画像部分をクリックすると、先生の紹介ページにリンクします。

担当科目：数学

大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！

職業：数学者
職歴：高校非常勤（院生時代）、高専、大学　准教授
趣味：
①将棋　棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀　アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス　中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。

大学入試問題#156　昭和大学(2019)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#昭和大学

指導講師：ますただ

問題文全文(内容文)：

\int_{1}^{2} \frac{d x}{x^{2} - 2 x + 2}

を求めよ。

出典：2019年昭和大学　入試問題

この動画を見る　

大学入試問題#155　琉球大学(1987)　極限

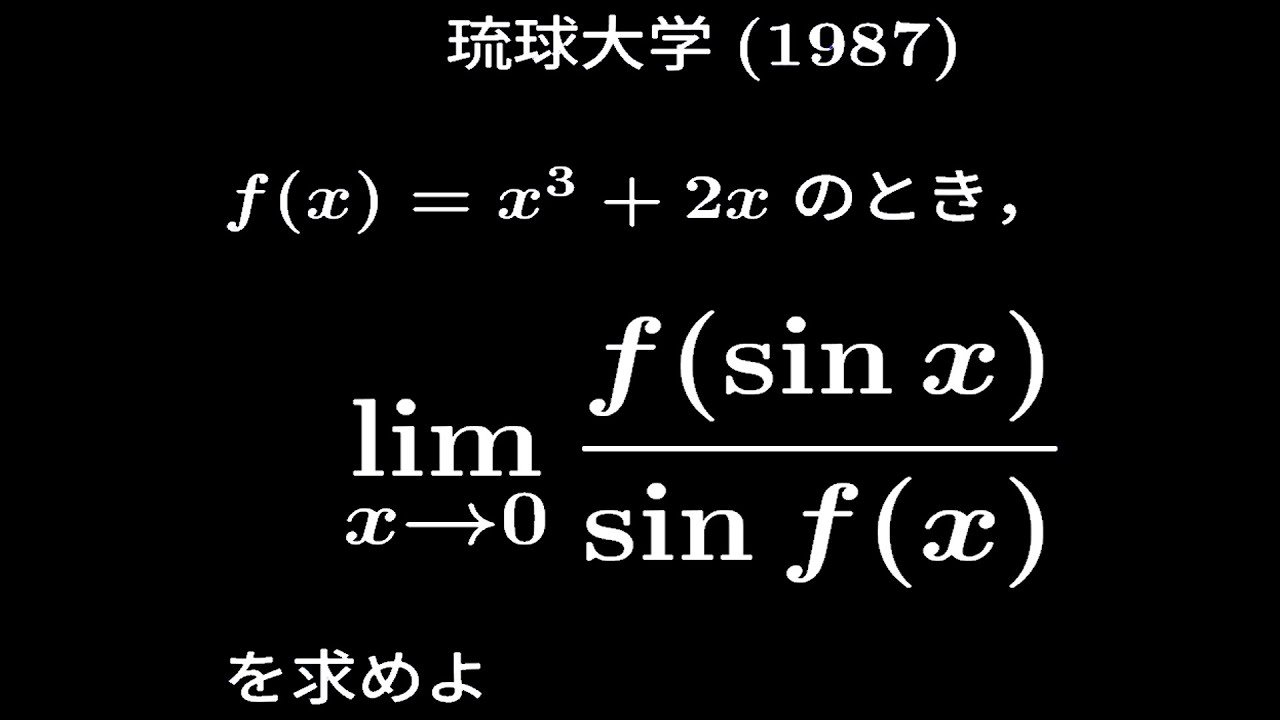
単元： #大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#琉球大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

f (x) = x^{3} + 2 x

のとき

lim_{x \to 0} \frac{f (\sin x)}{\sin f (x)}

を求めよ。

出典：1987年琉球大学　入試問題

この動画を見る　

大学入試問題#154　横浜市立大学医学部(2017)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#横浜市立大学

指導講師：ますただ

問題文全文(内容文)：

\int_{\frac{π}{3}}^{\frac{π}{2}} \frac{\sin x}{\cos 2 x} d x

を求めよ。

出典：2017年横浜市立大学医学部　入試問題

この動画を見る　

大学入試問題#153　東京医科大学(2017)　微積の応用

単元： #大学入試過去問(数学)#微分とその応用#積分とその応用#微分法#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学#東京医科大学

指導講師：ますただ

問題文全文(内容文)：

x > 0

f (x) = \int_{1}^{x} \frac{x + 4 t}{\sqrt{3 x^{4} + t^{4}}} d t

において

f^{'} (x)

を求めよ。

出典：2017年東京医科大学　入試問題

この動画を見る　

大学入試問題#152　東京工業大学(2002)　極限

単元： #大学入試過去問(数学)#関数と極限#数列の極限#学校別大学入試過去問解説(数学)#東京工業大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

lim_{n \to \infty} \frac{1}{l o g n} (1 + \frac{1}{2} + ・ ・ ・ + \frac{1}{n})

を求めよ。

出典：2002年東京工業大学　入試問題

この動画を見る　

大学入試問題#151　東北大学2020　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{1} \frac{d x}{(1 + x^{2})^{3}}

を計算せよ。

出典：2020年東北大学　入試問題

この動画を見る　

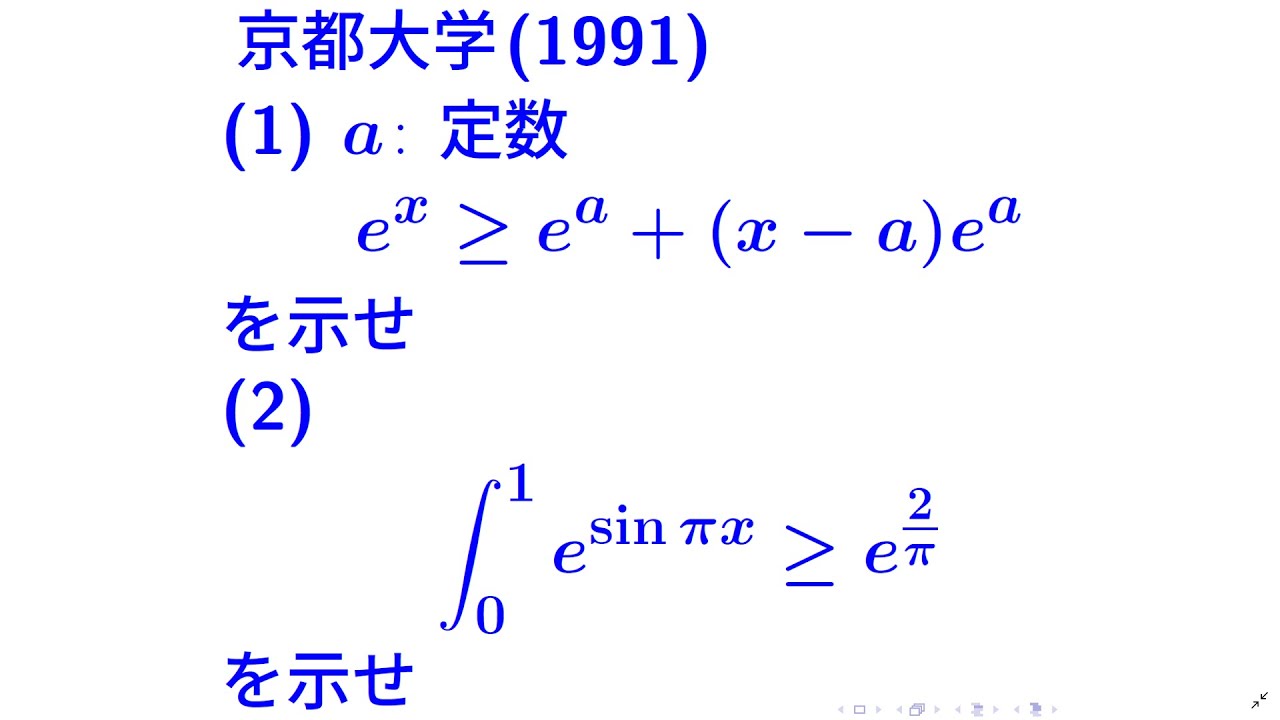
大学入試問題#150　京都大学(1991)　積分の応用

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：
（1）

a

実数

e^{x} ≧ e^{a} + (x - 1) e^{a}

を示せ

（2）

\int_{0}^{1} e^{\sin π x} d x ≧ e^{\frac{2}{x}}

を示せ

出典：1991年京都大学　入試問題

この動画を見る　

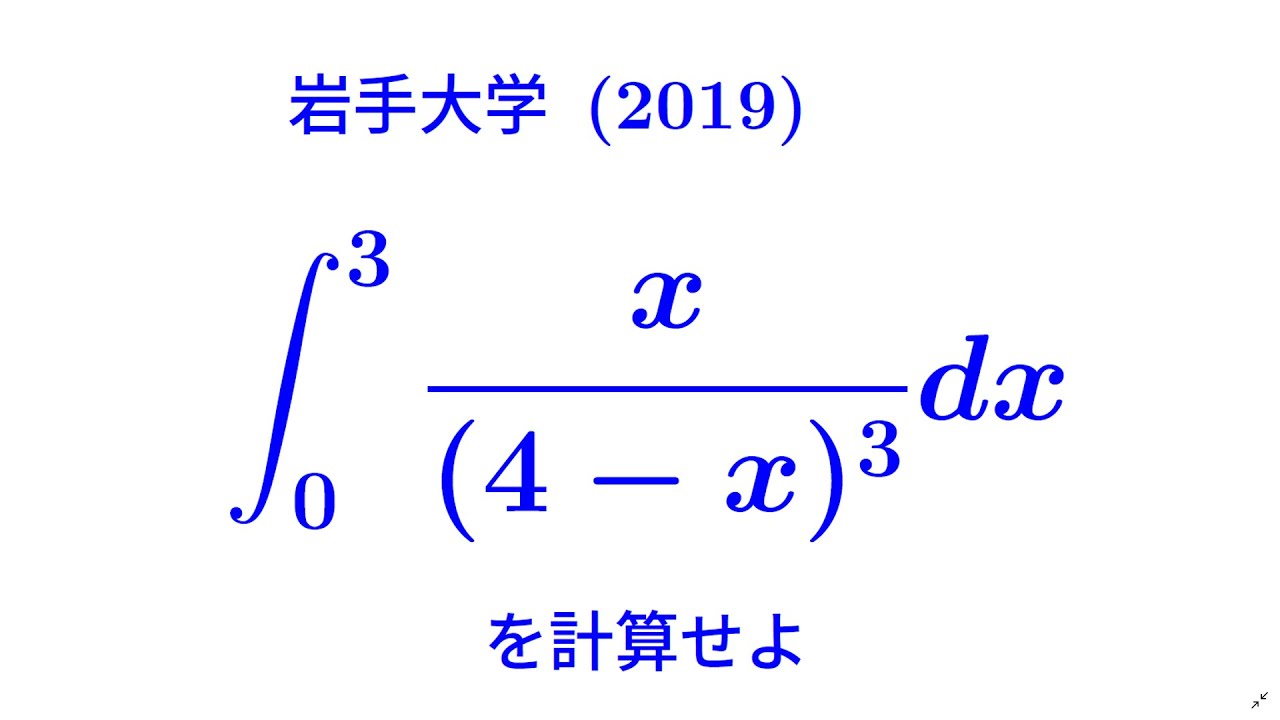
大学入試問題#149　岩手大学(2019)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{3} \frac{x}{(4 - x)^{3}} d x

を計算せよ。

出典：2019年岩手大学　入試問題

この動画を見る　

大学入試問題#148　京都大学(1972)　積分と極限

単元： #大学入試過去問(数学)#関数と極限#積分とその応用#関数の極限#定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

x > 0

F (x) = \int_{0}^{x} \frac{t}{(t + 1) (t + 3)} d t

のとき

lim_{x \to \infty} (F (x) - l o g x)

を求めよ。

出典：1972年京都大学　入試問題

この動画を見る　

大学入試問題#147　三重大学(2020)　積分の応用

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#三重大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：
（1）

x ≧ 1

のとき

x ≧ 1 + l o g x

を示せ

（2）

\int_{1}^{e} \frac{l o g x}{1 + l o g x} d x ≧ \frac{1}{2}

を示せ

出典：2020年三重大学　入試問題

この動画を見る　

大学入試問題#146　東京工業大学(1966)　定積分

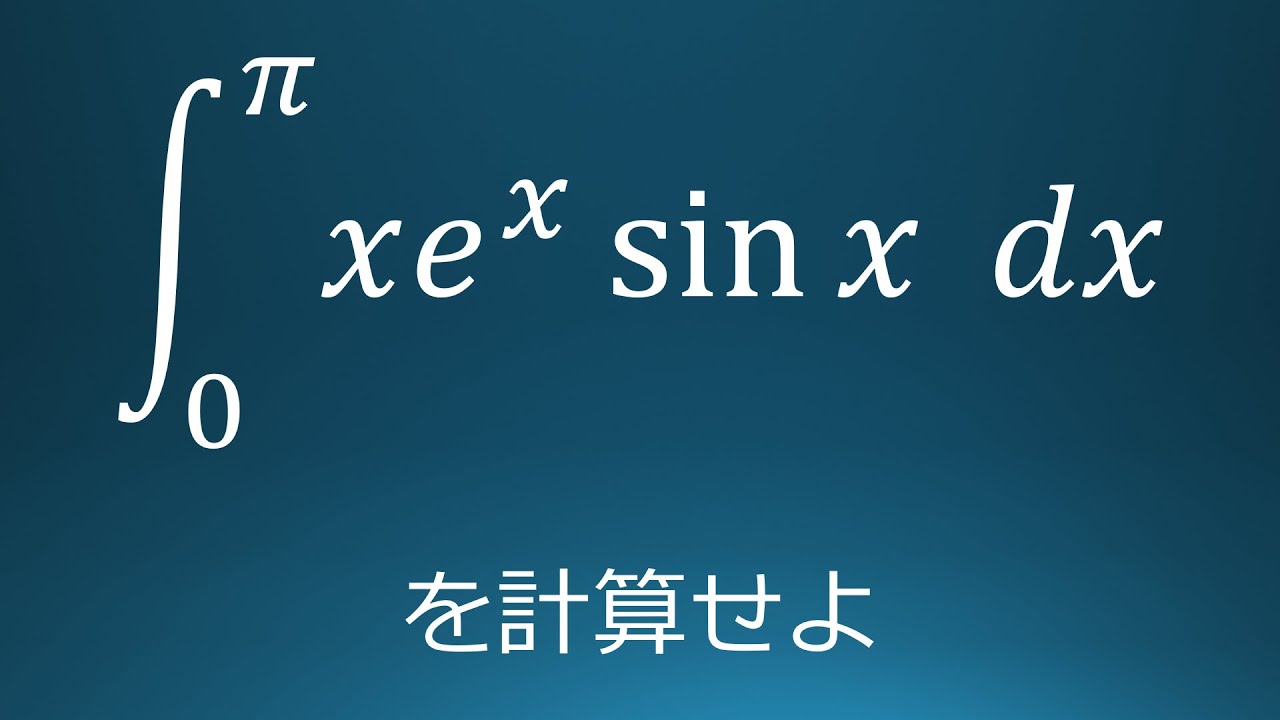
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京工業大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{π} x e^{x} \sin x d x

を計算せよ。

出典：1966年東京工業大学　入試問題

この動画を見る　

大学入試問題#145　自治医科大(2004)　整数問題

単元： #数A#大学入試過去問(数学)#整数の性質#約数・倍数・整数の割り算と余り・合同式#学校別大学入試過去問解説(数学)#数学(高校生)#自治医科大学

指導講師：ますただ

問題文全文(内容文)：

x^{2} - 3 a x + 2 a - 3 = 0

が2つの整数解をもつように

a

が定まっている。

a^{2} + 3

の値を求めよ。

出典：2004年自治医科大学　入試問題

この動画を見る　

大学入試問題#144　東京理科大学(2006)　定積分

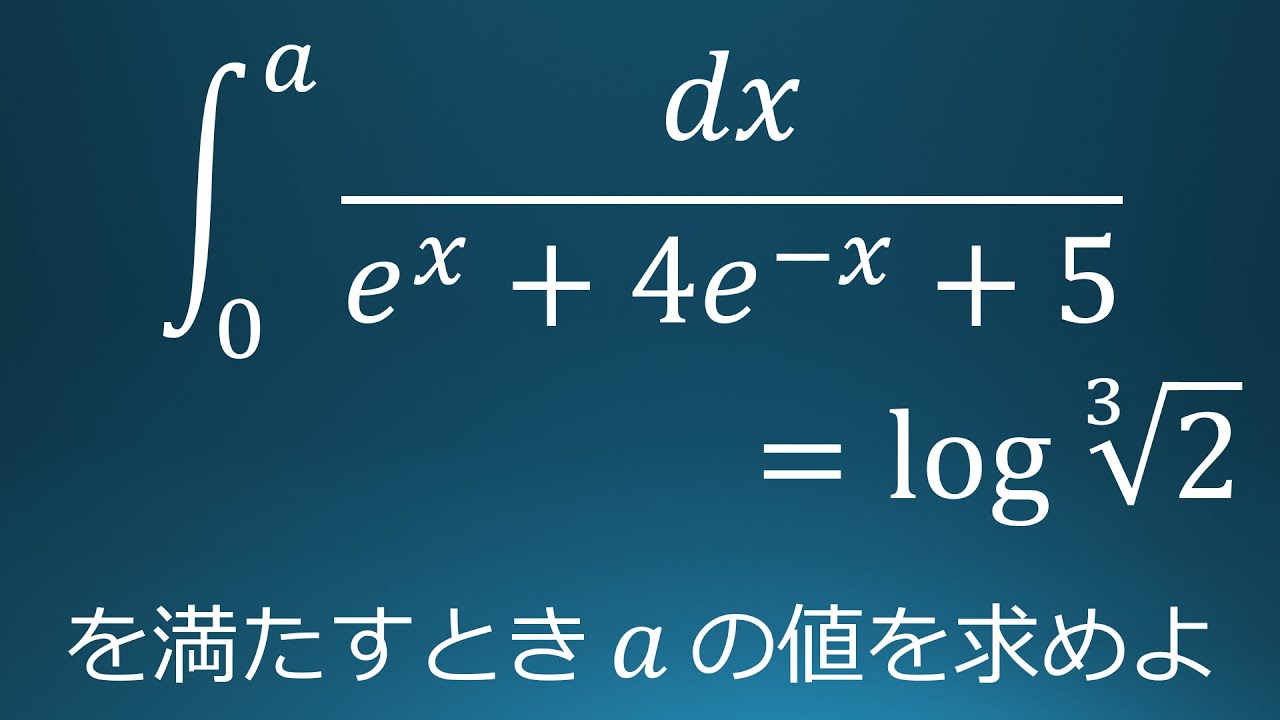
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{a} \frac{d x}{e^{x} + 4 e^{- x} + 5} = l o g \sqrt[3]{2}

が成り立つとき

a

の値を求めよ。

出典：2006年東京理科大学　入試問題

この動画を見る　

大学入試問題#143　東海大学医学部(2020)　因数分解

単元： #数学(中学生)#中３数学#式の計算（展開、因数分解）#数Ⅰ#大学入試過去問(数学)#数と式#式の計算（整式・展開・因数分解）#学校別大学入試過去問解説(数学)#数学(高校生)#東海大学

指導講師：ますただ

問題文全文(内容文)：

(a + b + c)^{3} - a^{3} - b^{3} - c^{3}

を因数分解せよ。

出典：2020年東海大学医学部　入試問題

この動画を見る　

大学入試問題#142　広島市立大学(2014)　不定積分

単元： #大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学

指導講師：ますただ

問題文全文(内容文)：

\int \cos \sqrt{x} d x

を計算せよ。

出典：2014年広島市立大学　入試問題

この動画を見る　

大学入試問題#141　島根大学(2020)　数列

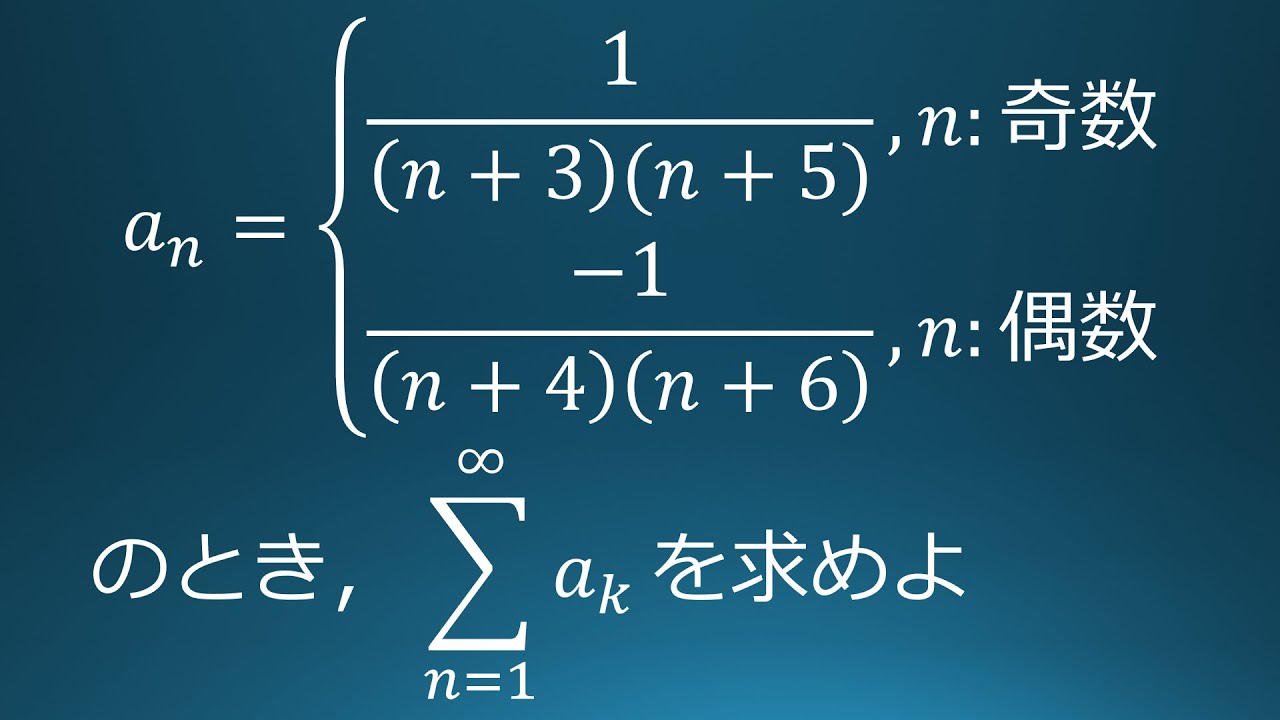
単元： #大学入試過去問(数学)#数列#数列とその和（等差・等比・階差・Σ）#学校別大学入試過去問解説(数学)#数学(高校生)#島根大学#数B

指導講師：ますただ

問題文全文(内容文)：

a_{n} = \begin{array}{r} {\begin{cases} \frac{1}{(n + 3) (n + 5))}, n ： 奇 数 \\ \frac{1}{(n + 4) (n + 6)}, n ： 偶 数 \end{cases} \end{array}

\sum_{n = 1}^{\infty} a_{k}

を求めよ。

出典：2020年島根大学　入試問題

この動画を見る　

大学入試問題#140　横浜市立大学医学部(2008)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#横浜市立大学

指導講師：ますただ

問題文全文(内容文)：

\int_{- 2}^{2} x^{4} \sqrt{4 - x^{2}} d x

を計算せよ。

出典：2008年横浜市立大学医学部　入試問題

この動画を見る　

大学入試問題#139　佐賀大学(2014)　定積分

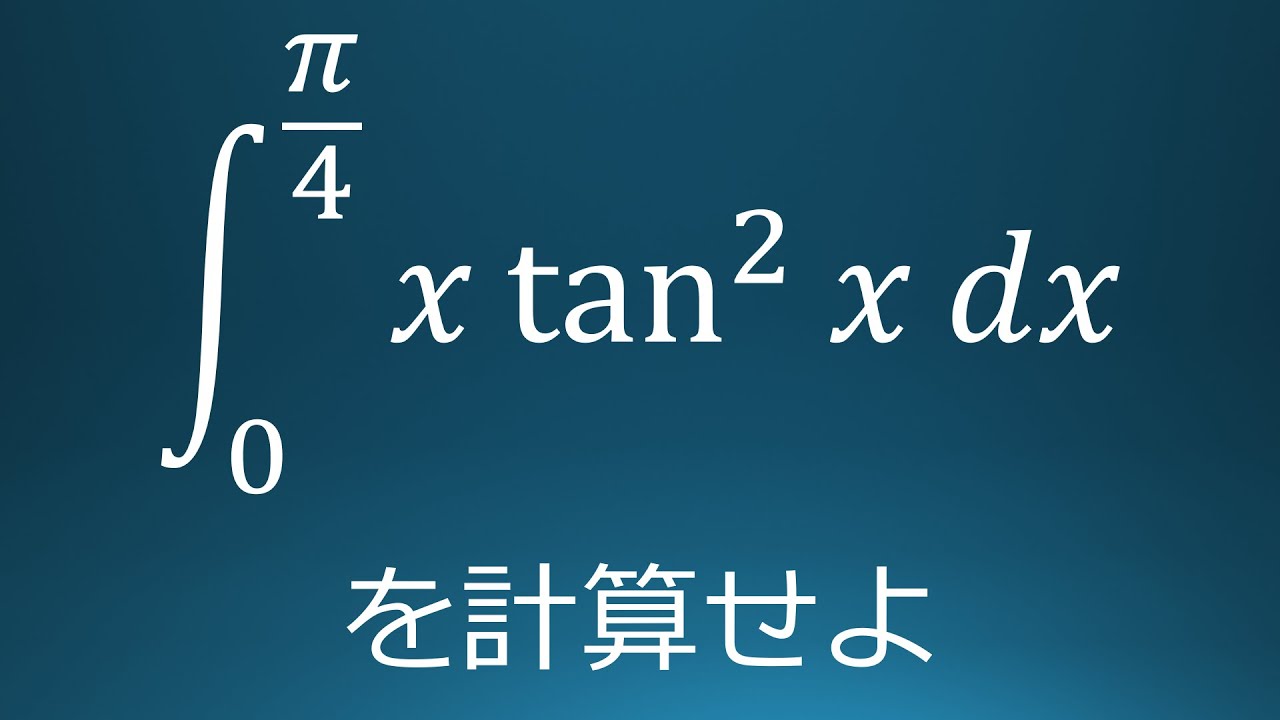
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#佐賀大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{\frac{π}{4}} x \tan^{2} x d x

を計算せよ。

出典：2014年佐賀大学　入試問題

この動画を見る　

大学入試問題#138　静岡県立大学(2021)　定積分

単元： #積分とその応用#定積分#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{\frac{1}{2}}^{2} \frac{(l o g x)^{2}}{1 + x} d x

を計算せよ。

出典：2019年静岡県立大学　入試問題

この動画を見る　

どっかの都道府県の教採の問題　数列　個人的に数列では過去一の難問

単元： #数列#数列とその和（等差・等比・階差・Σ）#数学(高校生)#数B

指導講師：ますただ

問題文全文(内容文)：

\sum_{k = 1}^{n} 5^{- k} k (k + 1) a_{k} = 2 (n + \frac{1}{4})^{2}

（1）

a_{n}

を求めよ。
（2）

\sum_{k = 1}^{n} a_{k}

を求めよ。

この動画を見る　

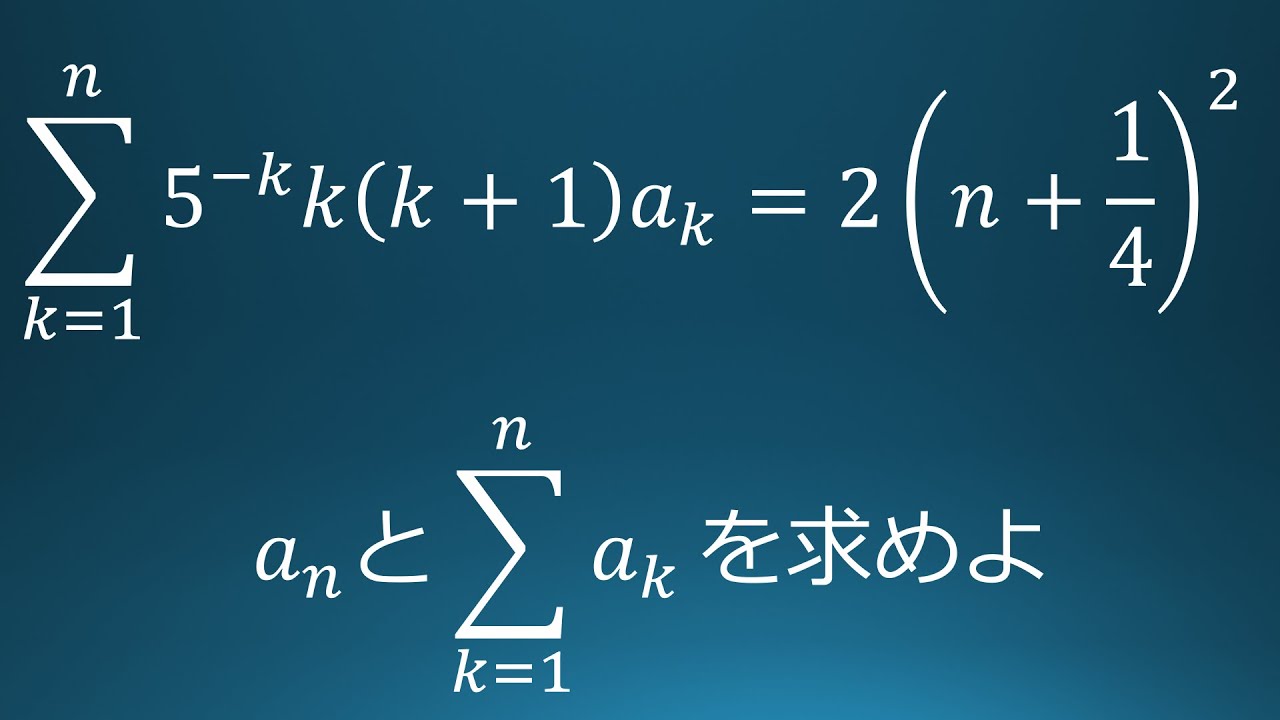
大学入試問題#137　京都大学(2021)　曲線の長さ

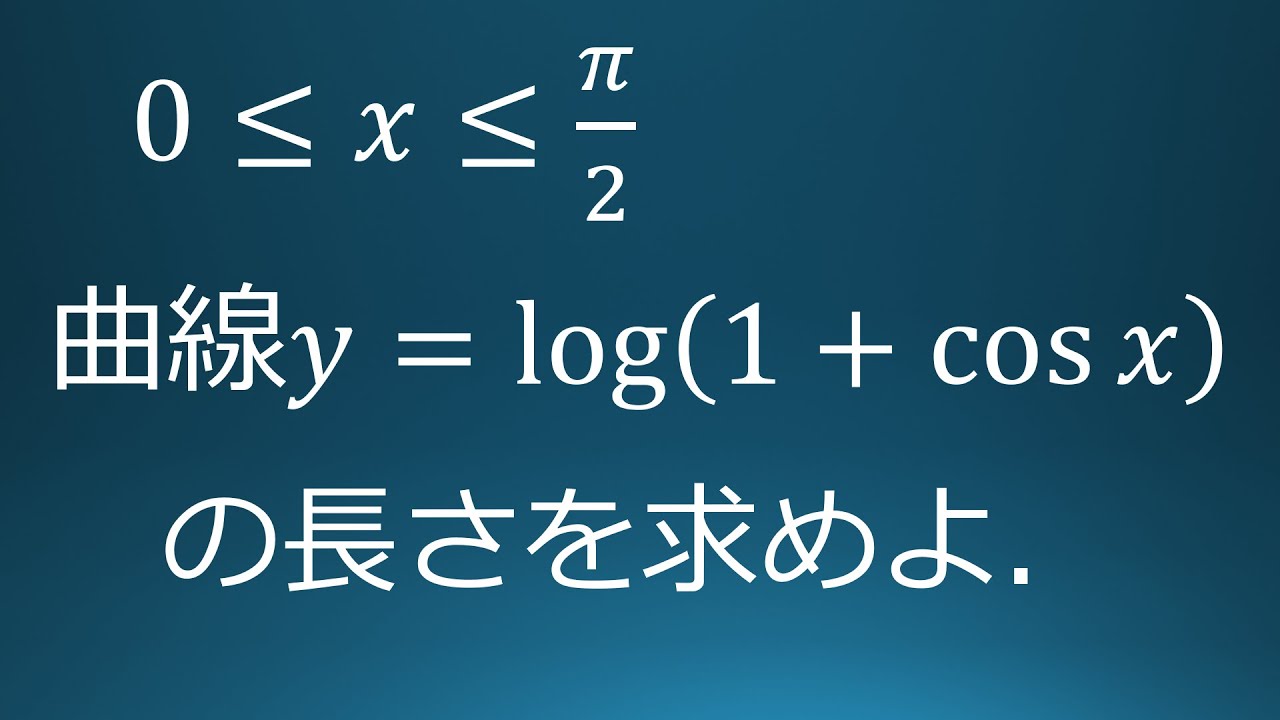
単元：
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269

Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270

指導講師：ますただ

問題文全文(内容文)：

0 ≦ x ≦ \frac{π}{2}

曲線

y = l o g (1 + \cos x)

の長さ

l

を求めよ。

出典：2021年京都大学　入試問題

この動画を見る　

大学入試問題#137　秋田大学(2020)　三角関数

単元： #大学入試過去問(数学)#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#数学(高校生)#秋田大学

指導講師：ますただ

問題文全文(内容文)：

y = \frac{6 + 4 \sin θ + 4 \cos θ + \sin 2 θ}{2 + \sin θ + \cos θ}

の最小値を求めよ。

出典：2020年秋田大学　入試問題

この動画を見る　

大学入試問題#136　南山大学(2021)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#南山大学

指導講師：ますただ

問題文全文(内容文)：

\int_{- 1}^{1} | 2 x (1 - x^{2}) e^{- x^{2}} | d x

を計算せよ。

出典：2021年南山大学　入試問題

この動画を見る　

#51　数検1級1次　過去問　逆三角関数

単元： #数Ⅱ#数学検定・数学甲子園・数学オリンピック等#三角関数#三角関数とグラフ#数学検定#数学検定1級

指導講師：ますただ

問題文全文(内容文)：

\sin (\sin^{- 1} (- \frac{5}{13}) + \cos^{- 1} (\frac{4}{5}))

の値を求めよ。

出典：数検1級1次　過去問

この動画を見る　

大学入試問題#135　横浜市立大学(2020)　定積分　個人的には難

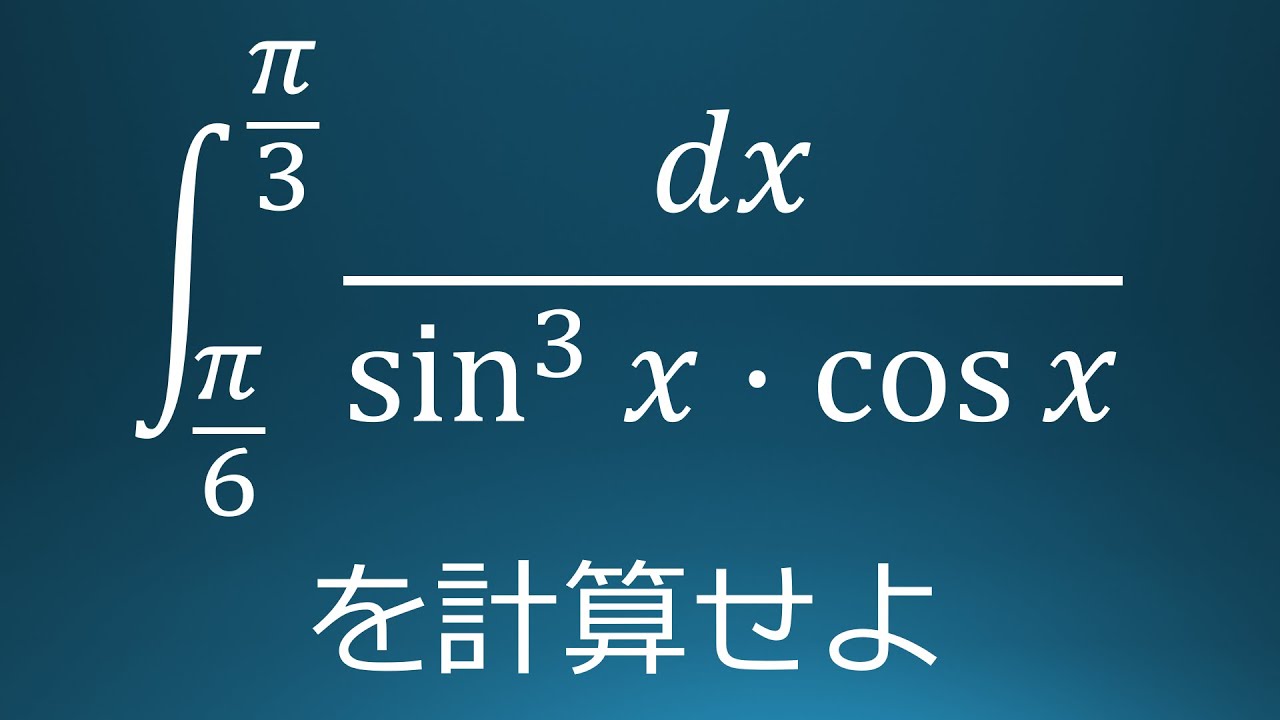
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#横浜市立大学

指導講師：ますただ

問題文全文(内容文)：

\int_{\frac{π}{6}}^{\frac{π}{3}} \frac{d x}{\sin^{3} x \cos x}

出典：2020年横浜市立大学　入試問題

この動画を見る　

大学入試問題#134　京都工芸繊維大学(2018)　不定積分

単元： #積分とその応用#不定積分#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int {l o g (3 + \cos^{2} θ)} \cos θ d θ

を計算せよ。

出典：2018年京都工芸繊維大学　入試問題

この動画を見る　

大学入試問題#133　京都大学(2009)　極方程式の曲線の長さ

単元： #大学入試過去問(数学)#平面上の曲線#2次曲線#学校別大学入試過去問解説(数学)#媒介変数表示と極座標#京都大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：
極方程式

r = 1 + \cos θ

(0 ≦ θ ≦ π)

で表される曲線の長さ

l

を求めよ。

出典：2009年京都大学　入試問題

この動画を見る　

大学入試問題#132　横浜国立大学(2007)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{\frac{4}{3}}^{2} \frac{1}{x^{2} \sqrt{x - 1}} d x

を計算せよ。

出典：2007年横浜国立大学　入試問題

この動画を見る　

【誘導あり：概要欄】大学入試問題#131　浜松医科大学(2020)　三角比

単元： #数Ⅱ#大学入試過去問(数学)#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#浜松医科大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：
（1）

x > 0

のとき

x > \sin x

を示せ

（2）

\frac{1}{6} < \sin 10^{\circ} < \frac{π}{18}

を示せ

出典：2020年浜松医科大学　入試問題

この動画を見る　

大学入試問題#130　東海大学医学部(2016)　定積分

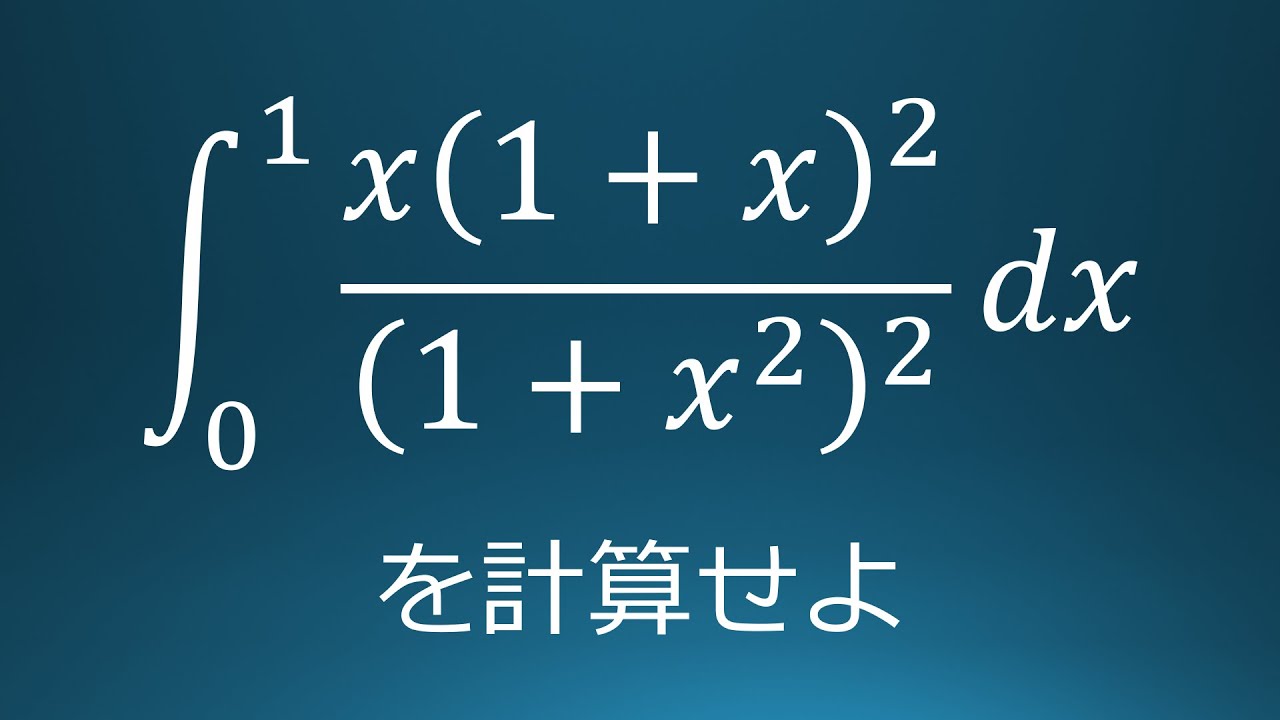
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東海大学

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{1} \frac{x (1 + x)^{2}}{(1 + x^{2})^{2}} d x

を計算せよ。

出典：2016年東海大学医学部　入試問題

この動画を見る　

<img src="https://kaiketsu-db.net/wp-content/uploads/2021/11/112-book-morph-outline.gif" alt="" data-eio="l"> ますただ

大学入試問題#156 昭和大学(2019) 定積分

大学入試問題#155 琉球大学(1987) 極限

大学入試問題#154 横浜市立大学医学部(2017) 定積分

大学入試問題#153 東京医科大学(2017) 微積の応用

大学入試問題#152 東京工業大学(2002) 極限

大学入試問題#151 東北大学2020 定積分

大学入試問題#150 京都大学(1991) 積分の応用

大学入試問題#149 岩手大学(2019) 定積分

大学入試問題#148 京都大学(1972) 積分と極限

大学入試問題#147 三重大学(2020) 積分の応用

大学入試問題#146 東京工業大学(1966) 定積分

大学入試問題#145 自治医科大(2004) 整数問題

大学入試問題#144 東京理科大学(2006) 定積分

大学入試問題#143 東海大学医学部(2020) 因数分解

大学入試問題#142 広島市立大学(2014) 不定積分

大学入試問題#141 島根大学(2020) 数列

大学入試問題#140 横浜市立大学医学部(2008) 定積分

大学入試問題#139 佐賀大学(2014) 定積分

大学入試問題#138 静岡県立大学(2021) 定積分

どっかの都道府県の教採の問題 数列 個人的に数列では過去一の難問

大学入試問題#137 京都大学(2021) 曲線の長さ

大学入試問題#137 秋田大学(2020) 三角関数

大学入試問題#136 南山大学(2021) 定積分

#51 数検1級1次 過去問 逆三角関数

大学入試問題#135 横浜市立大学(2020) 定積分 個人的には難

大学入試問題#134 京都工芸繊維大学(2018) 不定積分

大学入試問題#133 京都大学(2009) 極方程式の曲線の長さ

大学入試問題#132 横浜国立大学(2007) 定積分

【誘導あり：概要欄】大学入試問題#131 浜松医科大学(2020) 三角比

大学入試問題#130 東海大学医学部(2016) 定積分

ますただ

大学入試問題#156　昭和大学(2019)　定積分

大学入試問題#155　琉球大学(1987)　極限

大学入試問題#154　横浜市立大学医学部(2017)　定積分

大学入試問題#153　東京医科大学(2017)　微積の応用

大学入試問題#152　東京工業大学(2002)　極限

大学入試問題#151　東北大学2020　定積分

大学入試問題#150　京都大学(1991)　積分の応用

大学入試問題#149　岩手大学(2019)　定積分

大学入試問題#148　京都大学(1972)　積分と極限

大学入試問題#147　三重大学(2020)　積分の応用

大学入試問題#146　東京工業大学(1966)　定積分

大学入試問題#145　自治医科大(2004)　整数問題

大学入試問題#144　東京理科大学(2006)　定積分

大学入試問題#143　東海大学医学部(2020)　因数分解

大学入試問題#142　広島市立大学(2014)　不定積分

大学入試問題#141　島根大学(2020)　数列

大学入試問題#140　横浜市立大学医学部(2008)　定積分

大学入試問題#139　佐賀大学(2014)　定積分

大学入試問題#138　静岡県立大学(2021)　定積分

どっかの都道府県の教採の問題　数列　個人的に数列では過去一の難問

大学入試問題#137　京都大学(2021)　曲線の長さ

大学入試問題#137　秋田大学(2020)　三角関数

大学入試問題#136　南山大学(2021)　定積分

#51　数検1級1次　過去問　逆三角関数

大学入試問題#135　横浜市立大学(2020)　定積分　個人的には難

大学入試問題#134　京都工芸繊維大学(2018)　不定積分

大学入試問題#133　京都大学(2009)　極方程式の曲線の長さ

大学入試問題#132　横浜国立大学(2007)　定積分

【誘導あり：概要欄】大学入試問題#131　浜松医科大学(2020)　三角比

大学入試問題#130　東海大学医学部(2016)　定積分