 数Ⅱ
数Ⅱ
 数Ⅱ
数Ⅱ
大学入試問題#913「作成者サイコ−!」 #広島市立大学後期2024

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{2}^{8} \displaystyle \frac{1}{x(x+1)}log \displaystyle \frac{x}{x+1} dx$
出典:2024年 広島市立大学後期試験
この動画を見る
$\displaystyle \int_{2}^{8} \displaystyle \frac{1}{x(x+1)}log \displaystyle \frac{x}{x+1} dx$
出典:2024年 広島市立大学後期試験
#宮崎大学2024#不定積分_19#元高校教員
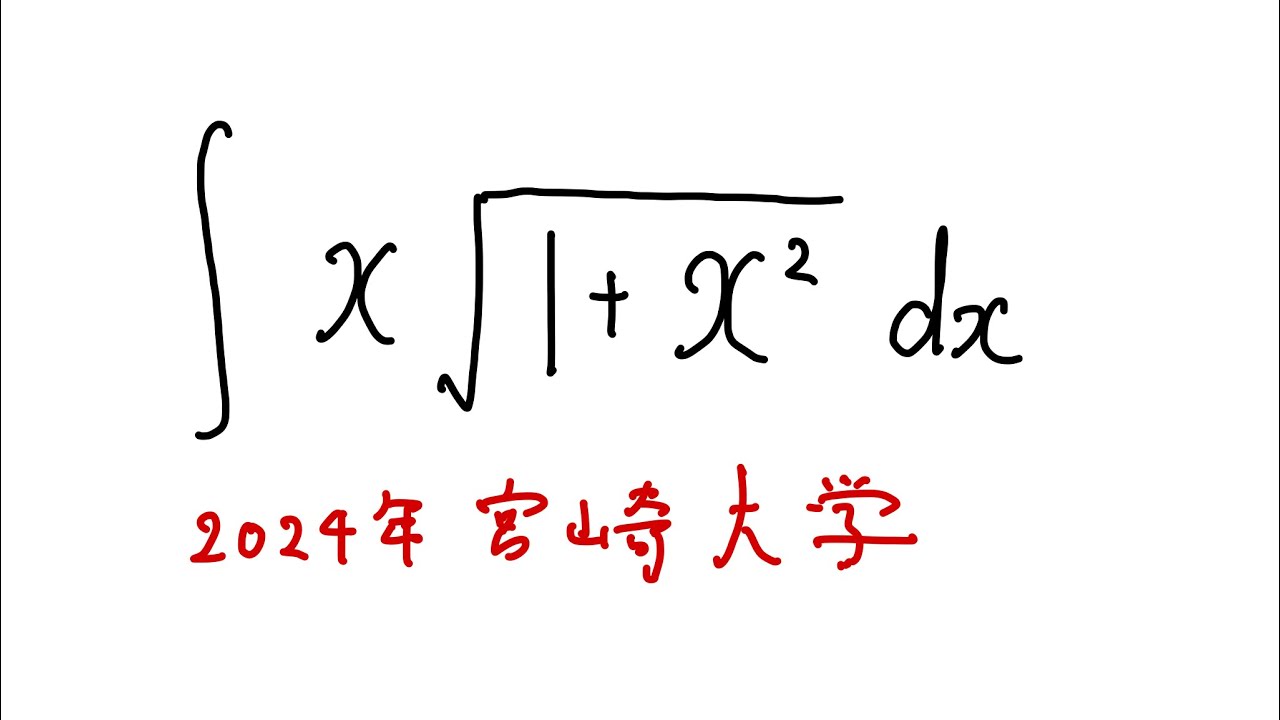
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#宮崎大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int x \sqrt{ 1+x^2 }dx$
出典:2024年宮崎大学
この動画を見る
$\displaystyle \int x \sqrt{ 1+x^2 }dx$
出典:2024年宮崎大学
#名古屋工業大学2024#不定積分_18#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#名古屋大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int\sqrt{ 2 }$ $logx$ $dx$
出典:2024年 名古屋工業大学
この動画を見る
$\displaystyle \int\sqrt{ 2 }$ $logx$ $dx$
出典:2024年 名古屋工業大学
#宮崎大学2024#定積分_17#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#宮崎大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{3}} \cos^2\displaystyle \frac{x}{4} dx$
出典:2024年宮崎大学
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{3}} \cos^2\displaystyle \frac{x}{4} dx$
出典:2024年宮崎大学
#前橋工科大学2017#定積分_16#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{2\pi} t\sin^2t$ $dt$
出典:2017年前橋工科大学
この動画を見る
$\displaystyle \int_{0}^{2\pi} t\sin^2t$ $dt$
出典:2017年前橋工科大学
#名古屋工業大学2020#定積分_15#元高校教員
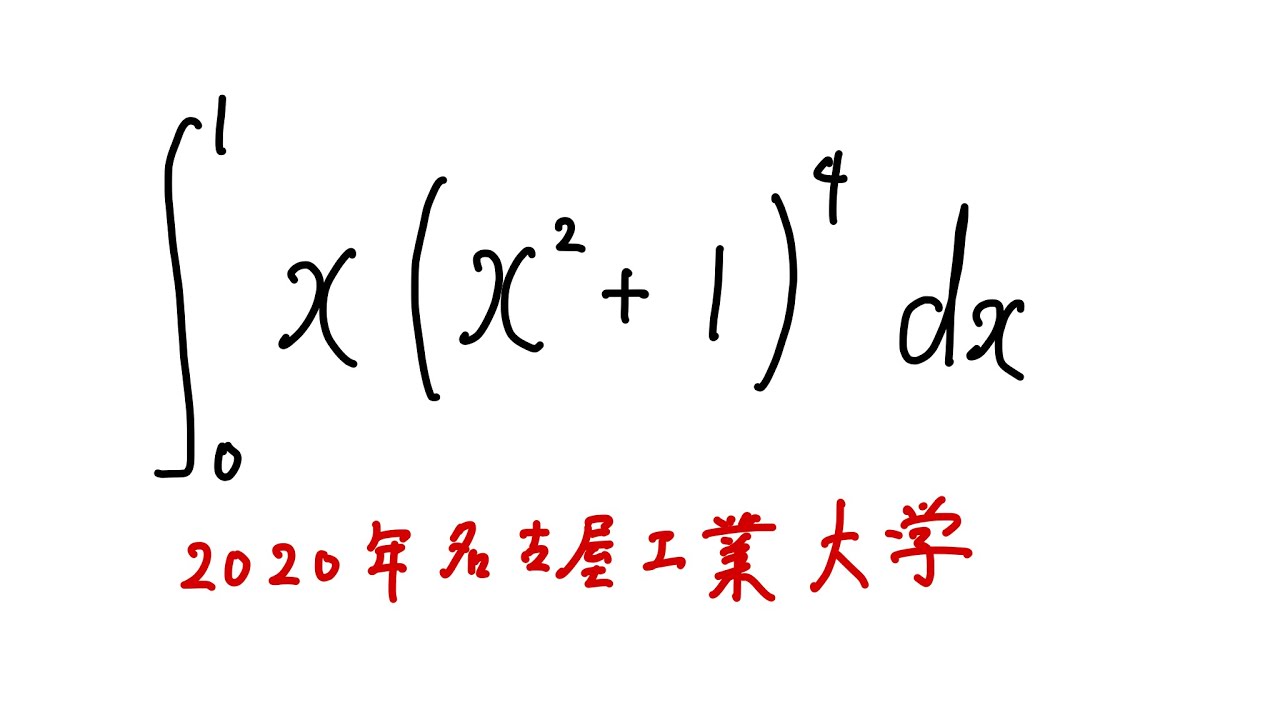
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} x(x^2+1)^4 dx$
出典:2020年名古屋工業大学
この動画を見る
$\displaystyle \int_{0}^{1} x(x^2+1)^4 dx$
出典:2020年名古屋工業大学
#前橋工科大学2021#定積分_14#元高校教員
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
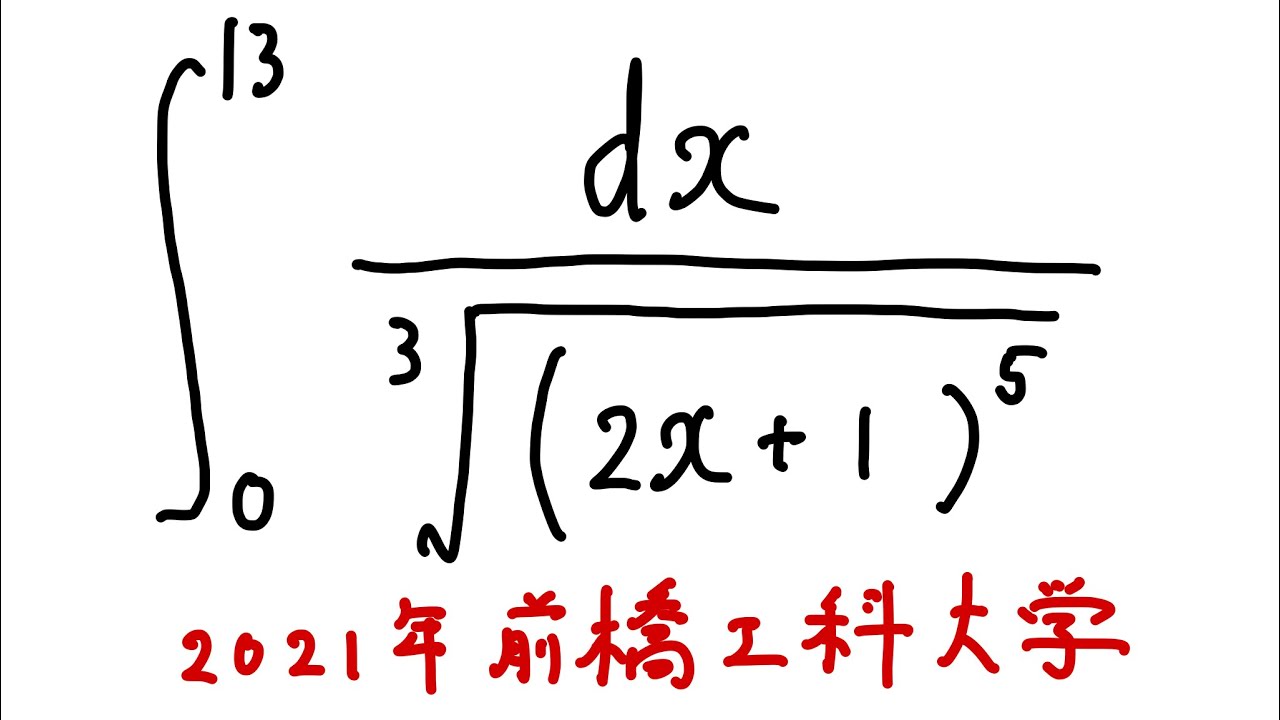
$\displaystyle \int_{0}^{13} \displaystyle \frac{dx}{\sqrt[ 3 ]{ (2x+1)^5 }}$
出典:2021年前橋工科大学
この動画を見る
$\displaystyle \int_{0}^{13} \displaystyle \frac{dx}{\sqrt[ 3 ]{ (2x+1)^5 }}$
出典:2021年前橋工科大学
大学入試問題#910「いやーいかにもミスりそう」 #琉球大学2021

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#琉球大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\pi} |3\sin x+\cos x| dx$
出典:2021年琉球大学後期
この動画を見る
$\displaystyle \int_{0}^{\pi} |3\sin x+\cos x| dx$
出典:2021年琉球大学後期
#前橋工科大学2024#定積分_13#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
問題文全文(内容文):
$\displaystyle \int_{0}^{\pi} \displaystyle \frac{1}{2}(1-\cos x)^2 dx$
出典:2024年前橋工科大学
この動画を見る
$\displaystyle \int_{0}^{\pi} \displaystyle \frac{1}{2}(1-\cos x)^2 dx$
出典:2024年前橋工科大学
#群馬大学推薦2023#定積分_12#元高校教員
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#群馬大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
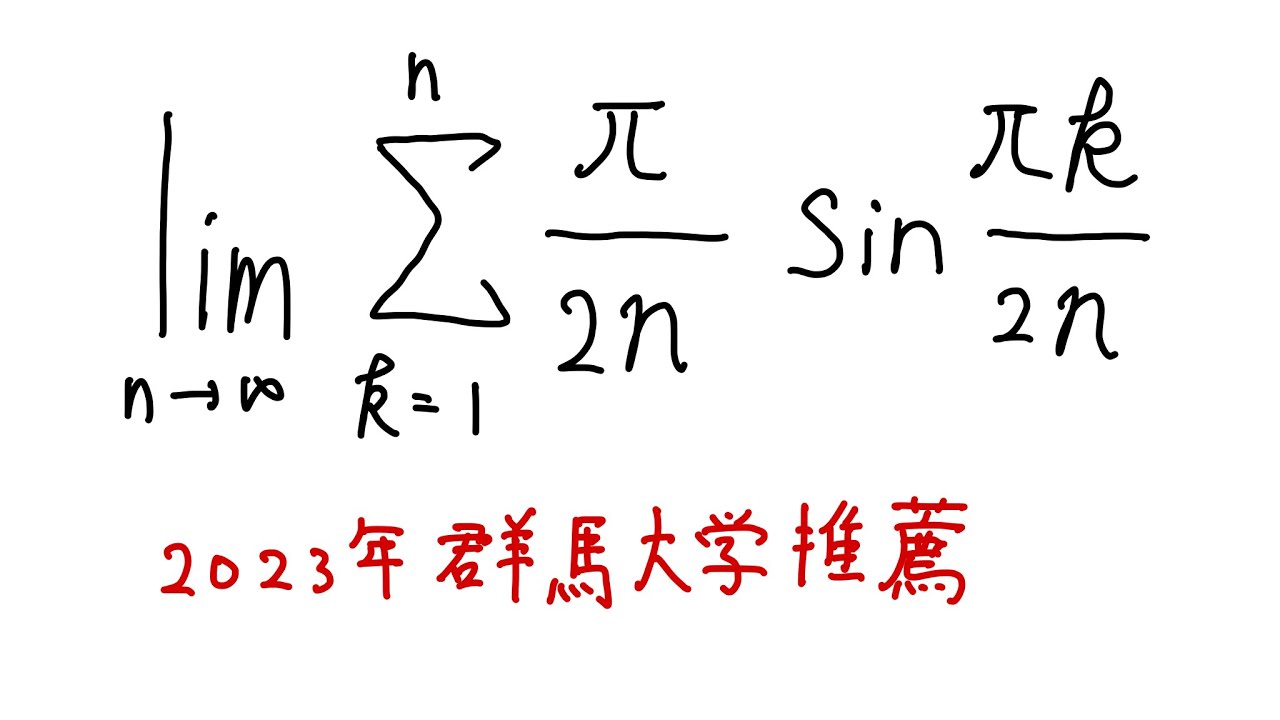
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=1}^n \displaystyle \frac{\pi}{2n}\sin\displaystyle \frac{k \pi }{2n}$
出典:2023年群馬大学推薦
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=1}^n \displaystyle \frac{\pi}{2n}\sin\displaystyle \frac{k \pi }{2n}$
出典:2023年群馬大学推薦
#茨城大学2024#定積分_11#元高校教員
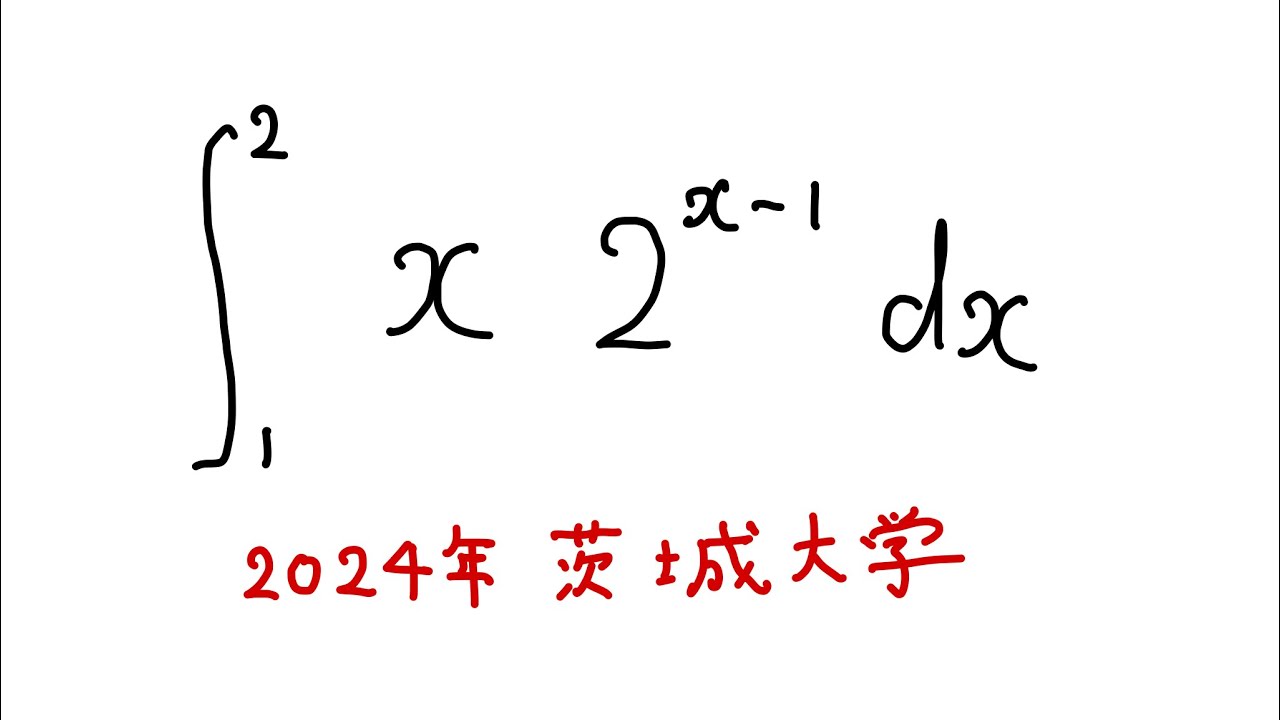
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{2} x 2^{x-1}$ $dx$
出典:2024年茨城大学
この動画を見る
$\displaystyle \int_{1}^{2} x 2^{x-1}$ $dx$
出典:2024年茨城大学
#茨城大学2022#極限_10#元高校教員
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#数学(高校生)#琉球大学
指導講師:
ますただ
問題文全文(内容文):
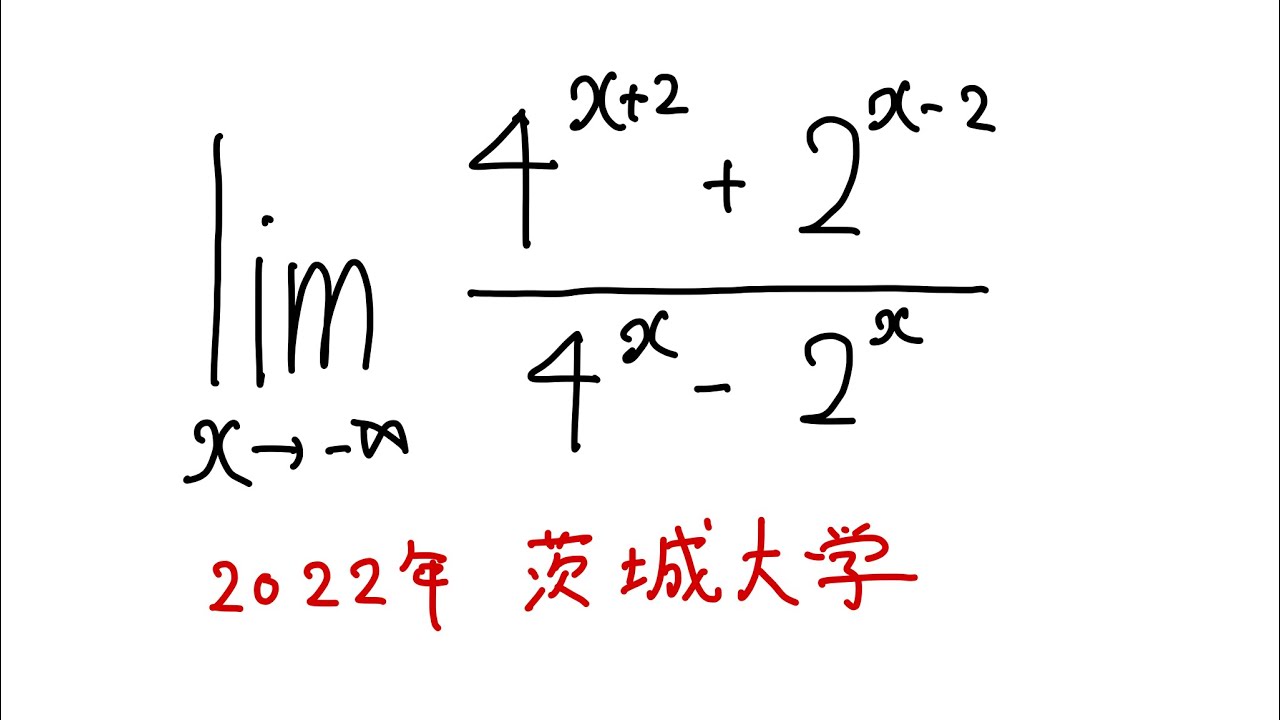
$\displaystyle \lim_{ x \to -\infty } \displaystyle \frac{4^{x+2}+2^{x-2}}{4^x-2^x}$
出典:2022年茨城大学
この動画を見る
$\displaystyle \lim_{ x \to -\infty } \displaystyle \frac{4^{x+2}+2^{x-2}}{4^x-2^x}$
出典:2022年茨城大学
大学入試問題#909「基本に忠実に」 前橋工科大学(2023)

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\sqrt{ 3 }} (x^7-3x^3)e-\displaystyle \frac{x^4}{4}$ $dx$
出典:2023年前橋工科大学
この動画を見る
$\displaystyle \int_{0}^{\sqrt{ 3 }} (x^7-3x^3)e-\displaystyle \frac{x^4}{4}$ $dx$
出典:2023年前橋工科大学
#会津大学2023#定積分_9#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\pi} \sin3x\cos2x$ $dx$
出典:2023年会津大学
この動画を見る
$\displaystyle \int_{0}^{\pi} \sin3x\cos2x$ $dx$
出典:2023年会津大学
#茨城大学2024#定積分_8#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}} \cos\theta\sin 2 \theta d \theta$
出典:2024年茨城大学後期
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} \cos\theta\sin 2 \theta d \theta$
出典:2024年茨城大学後期
大学入試問題#908「正確に対応するだけ」 #信州大学理学部(2024) #積分方程式

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#信州大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
次の等式を満たす関数$f(x)$を求めよ
$f(x)=x+\displaystyle \int_{0}^{\pi} f(t) \cos(x+t) dt$
出典:2024年信州大学理学部
この動画を見る
次の等式を満たす関数$f(x)$を求めよ
$f(x)=x+\displaystyle \int_{0}^{\pi} f(t) \cos(x+t) dt$
出典:2024年信州大学理学部
#茨城大学2024#定積分_7#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} e^x(e^{2x}+\frac{1}{e^{2x}}) dx$
出典:2024年茨城大学
この動画を見る
$\displaystyle \int_{0}^{1} e^x(e^{2x}+\frac{1}{e^{2x}}) dx$
出典:2024年茨城大学
#茨城大学後期2024#定積分_6#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{2}^{4} \displaystyle \frac{2}{x^2-1} dx$
出典:2024年茨城大学後期
この動画を見る
$\displaystyle \int_{2}^{4} \displaystyle \frac{2}{x^2-1} dx$
出典:2024年茨城大学後期
大学入試問題#907「チャートに掲載されてる?」 #信州大学理学部(2024) #極限

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ x \to a } \displaystyle \frac{x^3-x^2+(2a-3)x+b}{x^2-(a-1)x-a}=3$
が成り立つように定数$a$と$b$の値を求めよ。
出典:2024年信州大学理学部
この動画を見る
$\displaystyle \lim_{ x \to a } \displaystyle \frac{x^3-x^2+(2a-3)x+b}{x^2-(a-1)x-a}=3$
が成り立つように定数$a$と$b$の値を求めよ。
出典:2024年信州大学理学部
#茨城大学2024#区分求積法_5#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ n \to \infty } \displaystyle \frac{1}{n^{3}}\displaystyle \sum_{k=1}^n (n-k)^2$
出典:2024年茨城大学
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \frac{1}{n^{3}}\displaystyle \sum_{k=1}^n (n-k)^2$
出典:2024年茨城大学
#福島大学2024#定積分_4#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#福島大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} x\sqrt{ 1-x }$ $dx$
出典:2024年福島大学
この動画を見る
$\displaystyle \int_{0}^{1} x\sqrt{ 1-x }$ $dx$
出典:2024年福島大学
#高専数学#不定積分_12#元高専教員
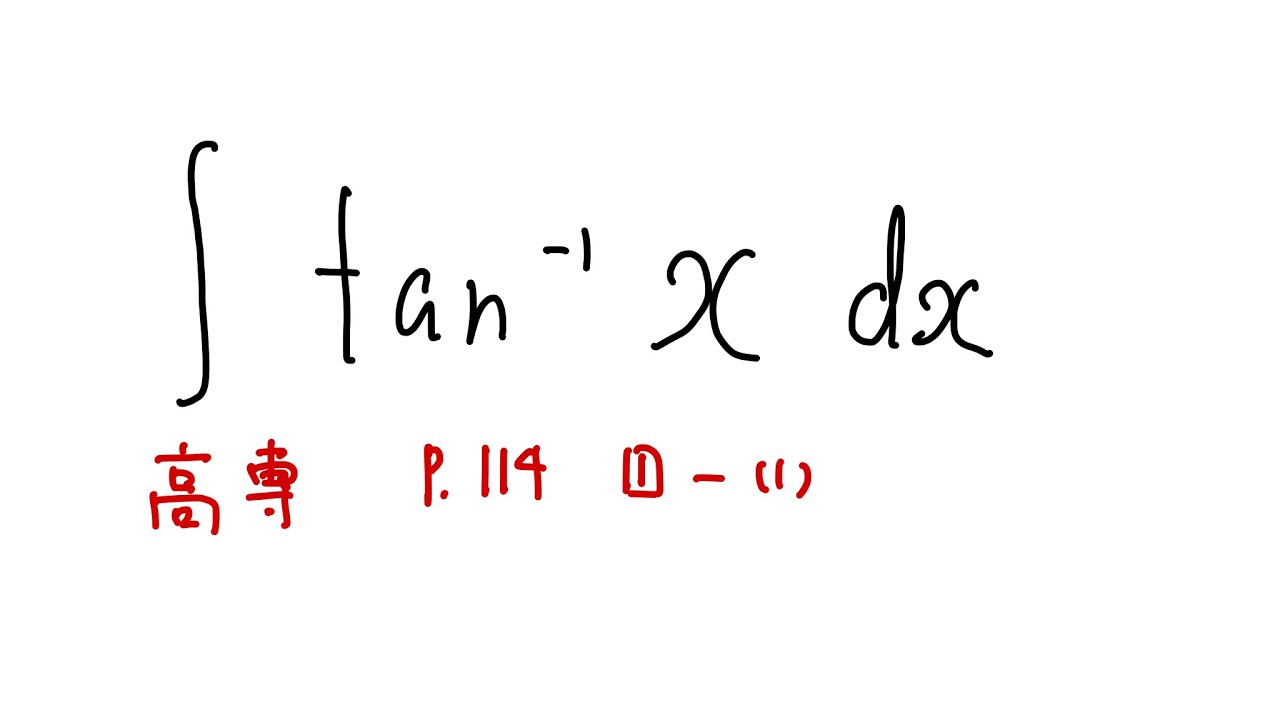
単元:
#数Ⅱ#微分法と積分法#積分とその応用#不定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int fan^{-1}x$ $dx$
この動画を見る
$\displaystyle \int fan^{-1}x$ $dx$
#会津大学2024#定積分_3#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
問題文全文(内容文):
$\displaystyle \int_{e^2}^{e^3} \displaystyle \frac{1}{x log x} dx$
出典:2024年会津大学
この動画を見る
$\displaystyle \int_{e^2}^{e^3} \displaystyle \frac{1}{x log x} dx$
出典:2024年会津大学
大学入試問題#905「基本変形の王道」 #信州大学教育学部(2024) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#信州大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} (\cos x\cos 2x-\cos3x\sin4x) dx$
出典:2024年信州大学教育学部
この動画を見る
$\displaystyle \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} (\cos x\cos 2x-\cos3x\sin4x) dx$
出典:2024年信州大学教育学部
#高専数学_12#定積分#元高専教員
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
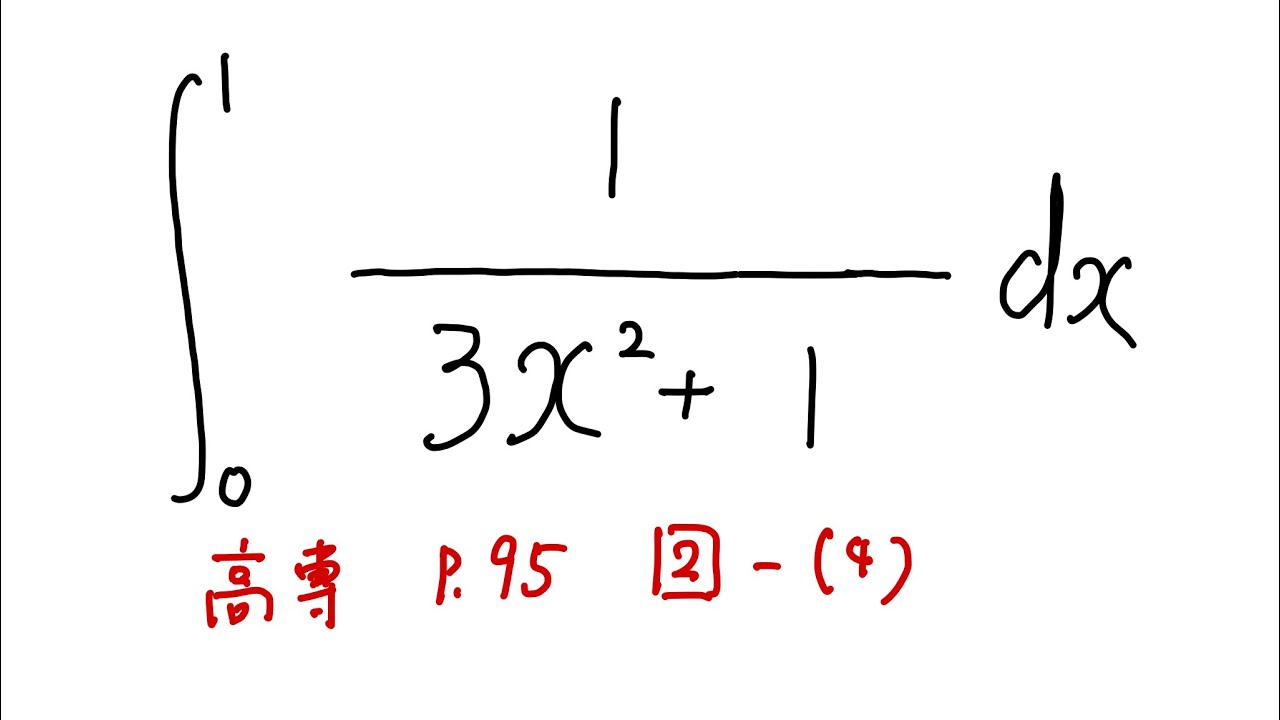
$\displaystyle \int_{0}^{1} \displaystyle \frac{1}{3x^2+1} dx$
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{1}{3x^2+1} dx$
#茨城大学2024#定積分_2#元高校教員
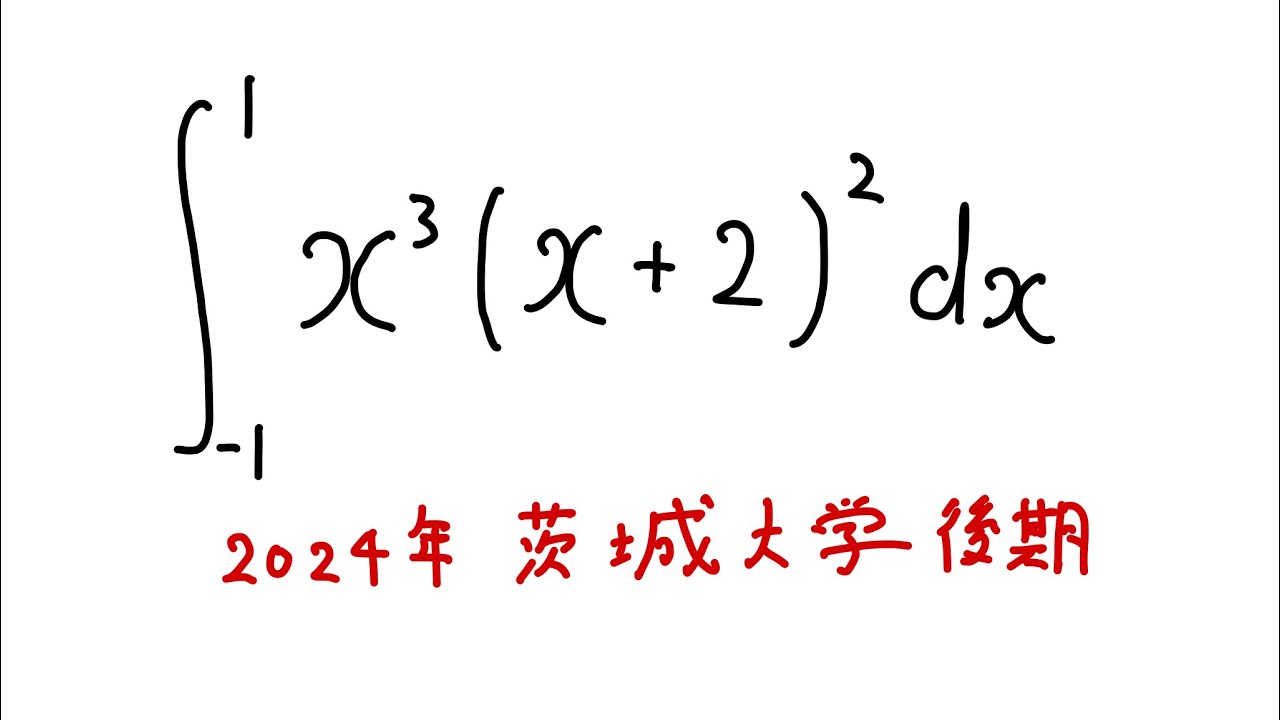
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-1}^{1} x^3(x+2)^2 dx$
出典:2024年茨城大学後期
この動画を見る
$\displaystyle \int_{-1}^{1} x^3(x+2)^2 dx$
出典:2024年茨城大学後期
大学入試問題#904「解き方いろいろ」 #お茶の水女子大学(2013) #積分方程式

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#お茶の水女子大学
指導講師:
ますただ
問題文全文(内容文):
$x \gt 0$で
$f(x)+\displaystyle \int_{1}^{x} \displaystyle \frac{f(t)}{t}dt=3x^2-2x$を満たす多項式$f(x)$を求めよ。
出典:2013年お茶の水女子大学
この動画を見る
$x \gt 0$で
$f(x)+\displaystyle \int_{1}^{x} \displaystyle \frac{f(t)}{t}dt=3x^2-2x$を満たす多項式$f(x)$を求めよ。
出典:2013年お茶の水女子大学
大学入試問題#616「これは理系が解くと逆にはまるかも」 名古屋大学(1963)

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#名古屋大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$x \gt y$とする
$x+y=6,\ xy=4$のとき
$\displaystyle \frac{\sqrt{ x }-\sqrt{ y }}{\sqrt{ x }+\sqrt{ y }}$の値を求めよ。
出典:1963年名古屋大学 入試問題
この動画を見る
$x \gt y$とする
$x+y=6,\ xy=4$のとき
$\displaystyle \frac{\sqrt{ x }-\sqrt{ y }}{\sqrt{ x }+\sqrt{ y }}$の値を求めよ。
出典:1963年名古屋大学 入試問題
#高専数学_11#定積分#元高専教員

単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{4} \displaystyle \frac{dx}{x^2-4x+8}$
この動画を見る
$\displaystyle \int_{0}^{4} \displaystyle \frac{dx}{x^2-4x+8}$
#茨城大学2024_1#定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{4}} \sin^5x\cos x$ $dx$
出典:2024年茨城大学
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}} \sin^5x\cos x$ $dx$
出典:2024年茨城大学
