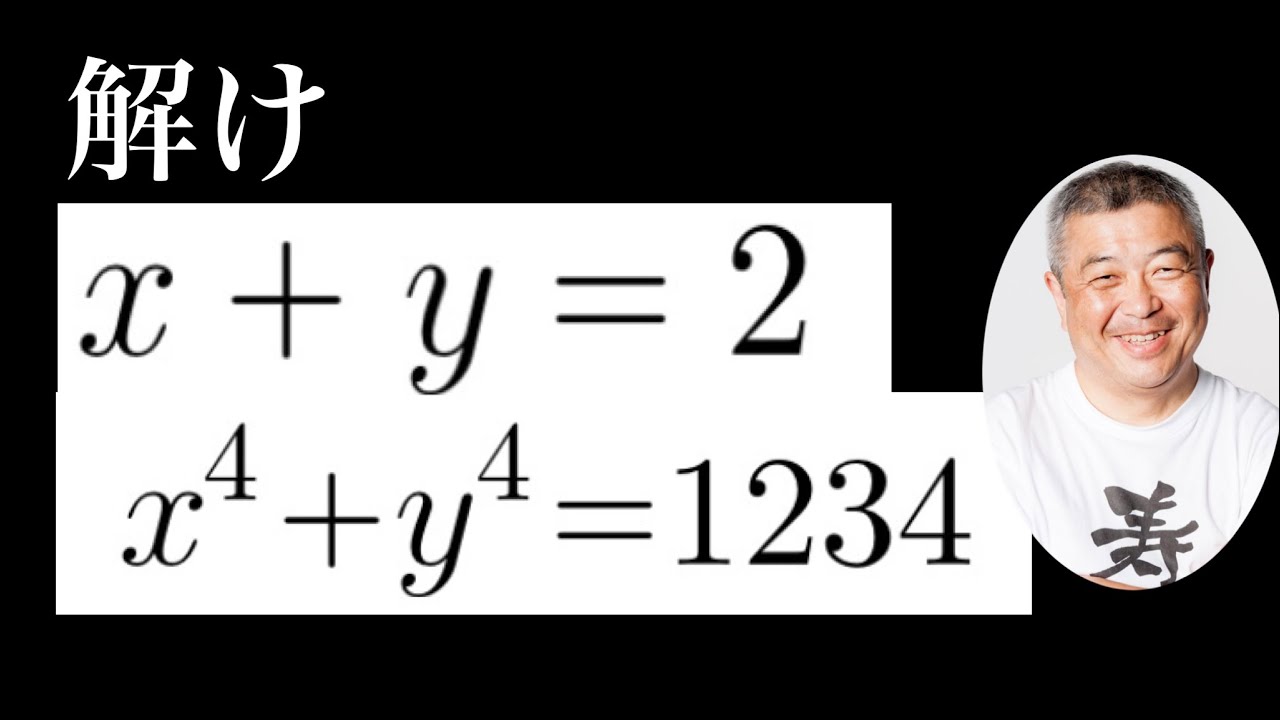問題文全文(内容文):
$-90^{ \circ } \lt \theta \lt 90^{ \circ }$
$(\sin \theta)x^2+2(\cos2\theta)x+cos2\theta=0$が少なくとも1つの実数解をもつような$\theta$の範囲を求めよ
出典:2001年早稲田大学 政治経済学部 過去問
$-90^{ \circ } \lt \theta \lt 90^{ \circ }$
$(\sin \theta)x^2+2(\cos2\theta)x+cos2\theta=0$が少なくとも1つの実数解をもつような$\theta$の範囲を求めよ
出典:2001年早稲田大学 政治経済学部 過去問
単元:
#数Ⅱ#大学入試過去問(数学)#複素数と方程式#剰余の定理・因数定理・組み立て除法と高次方程式#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$-90^{ \circ } \lt \theta \lt 90^{ \circ }$
$(\sin \theta)x^2+2(\cos2\theta)x+cos2\theta=0$が少なくとも1つの実数解をもつような$\theta$の範囲を求めよ
出典:2001年早稲田大学 政治経済学部 過去問
$-90^{ \circ } \lt \theta \lt 90^{ \circ }$
$(\sin \theta)x^2+2(\cos2\theta)x+cos2\theta=0$が少なくとも1つの実数解をもつような$\theta$の範囲を求めよ
出典:2001年早稲田大学 政治経済学部 過去問
投稿日:2020.02.01