ますただ

※下の画像部分をクリックすると、先生の紹介ページにリンクします。

担当科目：数学

大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！

職業：数学者
職歴：高校非常勤（院生時代）、高専、大学　准教授
趣味：
①将棋　棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀　アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス　中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。

大学入試問題#129　関西学院大学(1991)　二項定理の応用

単元： #数Ⅱ#大学入試過去問(数学)#式と証明#整式の除法・分数式・二項定理#学校別大学入試過去問解説(数学)#関西学院大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

(a + b + \frac{1}{a} + \frac{1}{b})^{7}

を展開した時の

a b^{2}

の係数を求めよ。

出典：1991年関西学院大学　入試問題

この動画を見る　

大学入試問題#128　東京理科大学(2020)　定積分

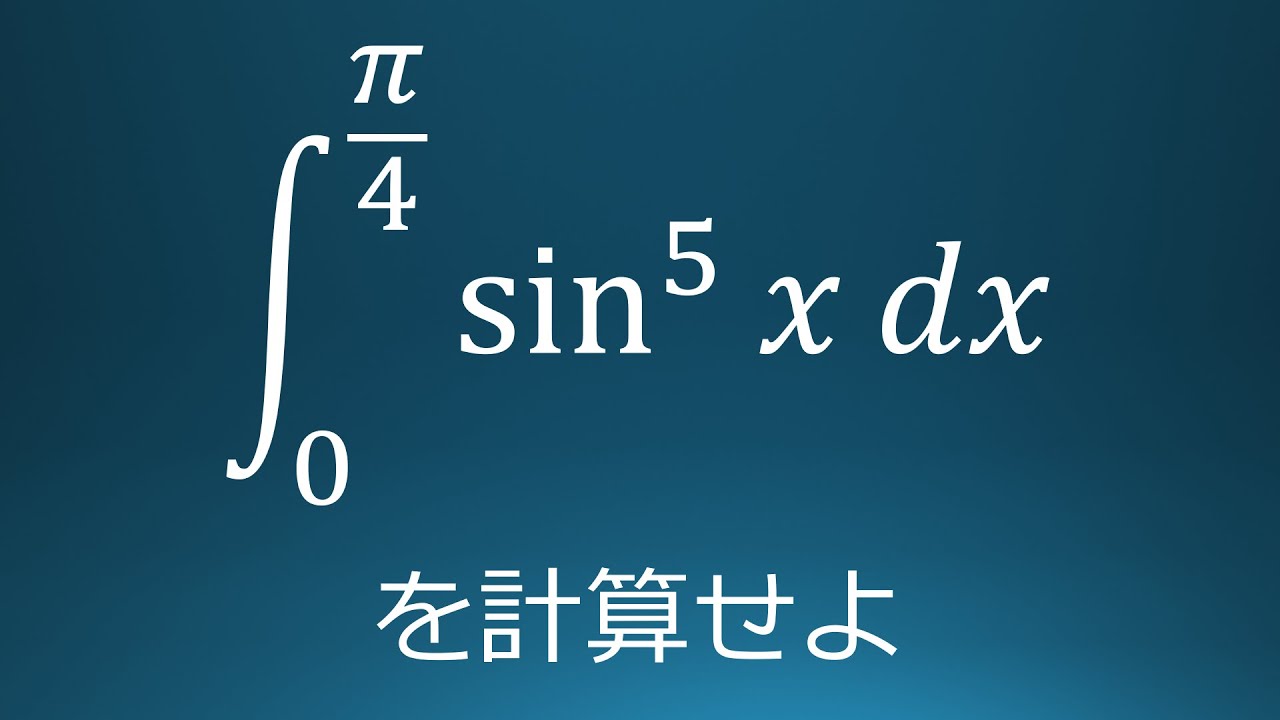
単元： #数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#東京理科大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{\frac{π}{4}} \sin^{5} x d x

を計算せよ。

出典：2020年東京理科大学　入試問題

この動画を見る　

大学入試問題#127　関西大学(1991)　整数問題

単元： #数A#大学入試過去問(数学)#整数の性質#約数・倍数・整数の割り算と余り・合同式#学校別大学入試過去問解説(数学)#数学(高校生)#関西大学

指導講師：ますただ

問題文全文(内容文)：

l, m, n

：正の整数

l^{2} m n = 64

を満たす組（

l, m, n

）の個数を求めよ。

出典：1991年関西大学　入試問題

この動画を見る　

大学入試問題#126　慶應大学医学部(2005)　不定積分

単元： #大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int (\frac{2}{x^{3}} + \frac{1}{x}) \sin x d x

を計算せよ。

出典：2005年慶應義塾大学　入試問題

この動画を見る　

大学入試問題#125　広島修道大学(2015)　整数問題

単元： #数A#大学入試過去問(数学)#整数の性質#学校別大学入試過去問解説(数学)#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

n

:自然数

2 n - 1

と

2 n + 1

は互いに素であることを示せ

出典：2015年広島修道大学　入試問題

この動画を見る　

大学入試問題#124　高知大学(2020)　定積分

単元： #大学入試過去問(数学)#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#高知大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{- \frac{7}{2}}^{\frac{9}{2}} \frac{2^{x}}{2^{x} + \sqrt{2}} d x

を計算せよ。

出典：2020年高知大学　入試問題

この動画を見る　

大学入試問題#123　鳥取大学　改　(2020)　Σの計算

単元： #大学入試過去問(数学)#数列#数列とその和（等差・等比・階差・Σ）#学校別大学入試過去問解説(数学)#数学(高校生)#鳥取大学#数B

指導講師：ますただ

問題文全文(内容文)：

\sum_{k = 1}^{n} k^{2} 2^{k}

を計算せよ。

出典：2020年鳥取大学　入試問題

この動画を見る　

大学入試問題#122　愛知県立大学(2020)　極限

単元： #大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

a > 0

lim_{x \to \infty} \frac{1}{x^{x}} (x - a)^{x}

を求めよ。

出典：2020年愛知県立大学　入試問題

この動画を見る　

大学入試問題#121　横浜国立大学(2004)　定積分

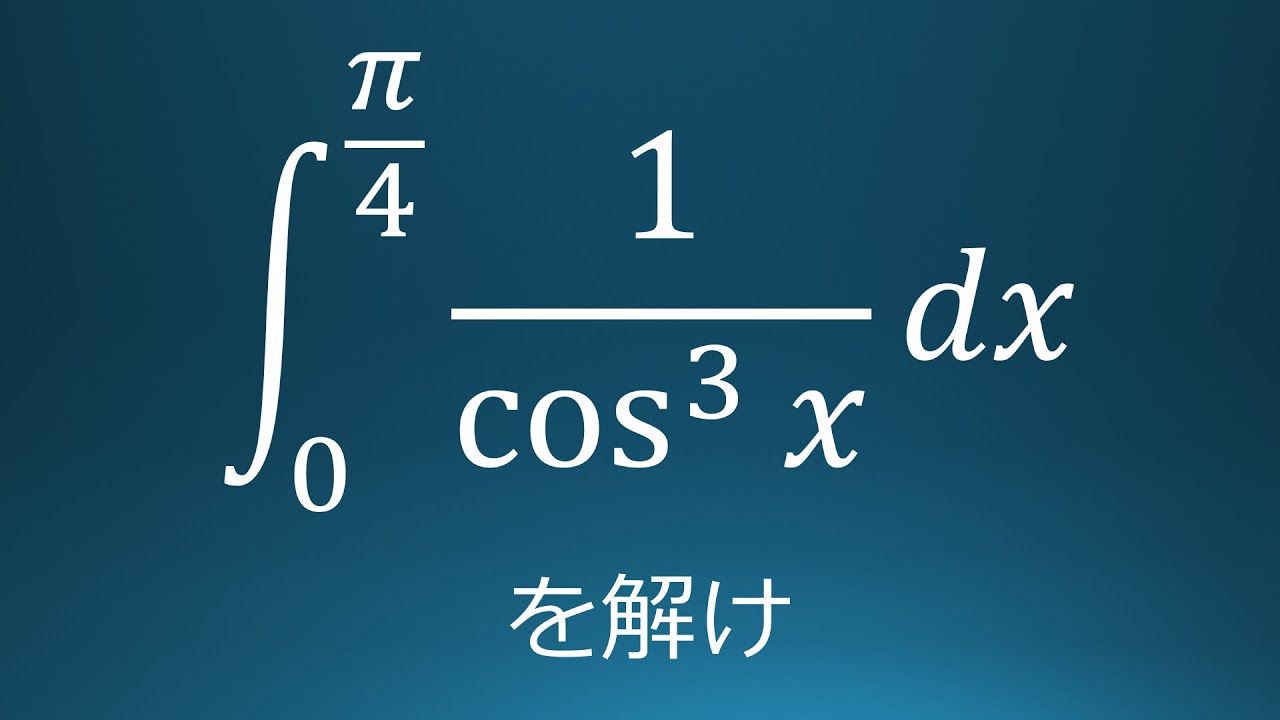
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{\frac{π}{4}} \frac{1}{\cos^{3} x} d x

を計算せよ。

出典：2004年横浜国立大学　入試問題

この動画を見る　

大学入試問題#120　早稲田大学(2003)　対数の不等式

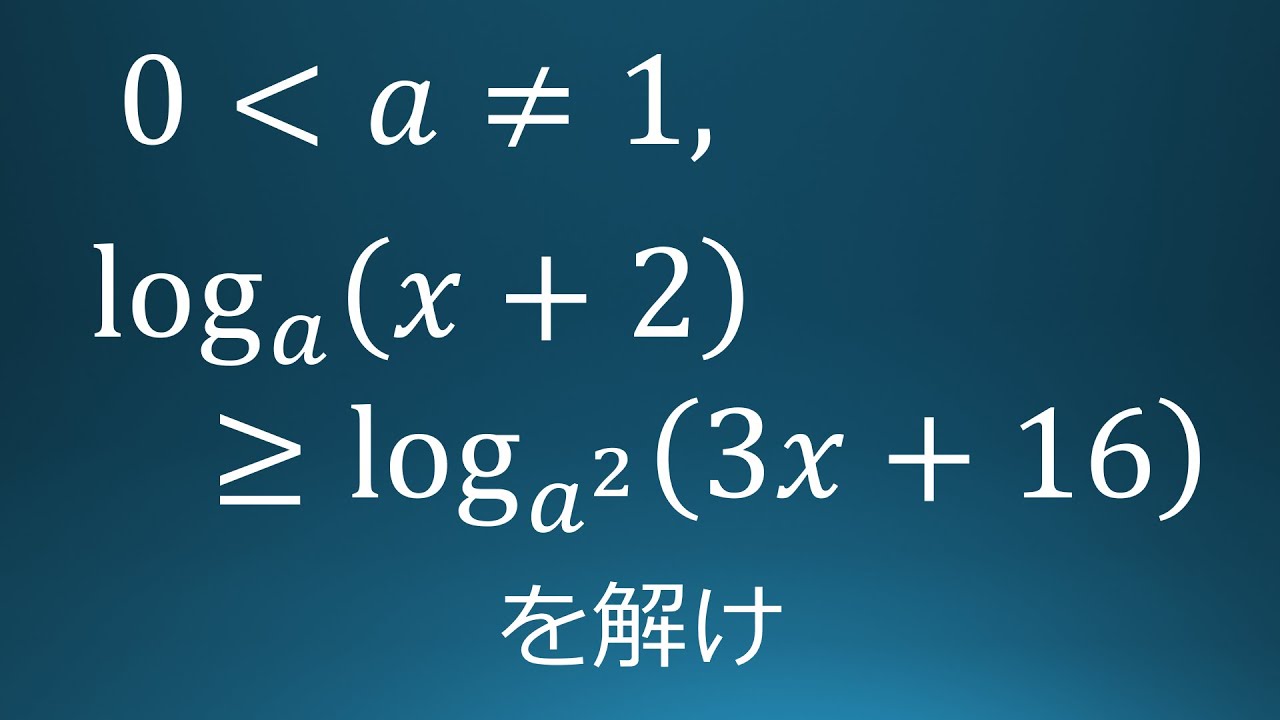
単元： #数Ⅱ#大学入試過去問(数学)#指数関数と対数関数#対数関数#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

a > 0, a \neq 1

l o g a (x + 2) ≧ l o g a^{2} (3 x + 16)

を解け

出典：2003年早稲田大学　入試問題

この動画を見る　

大学入試問題#119　横浜国立大学(2020)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

\int_{\frac{π}{6}}^{\frac{π}{4}} \frac{l o g (\sin x)}{\tan x} d x

を計算せよ。

出典：2020年横浜国立大学　入試問題

この動画を見る　

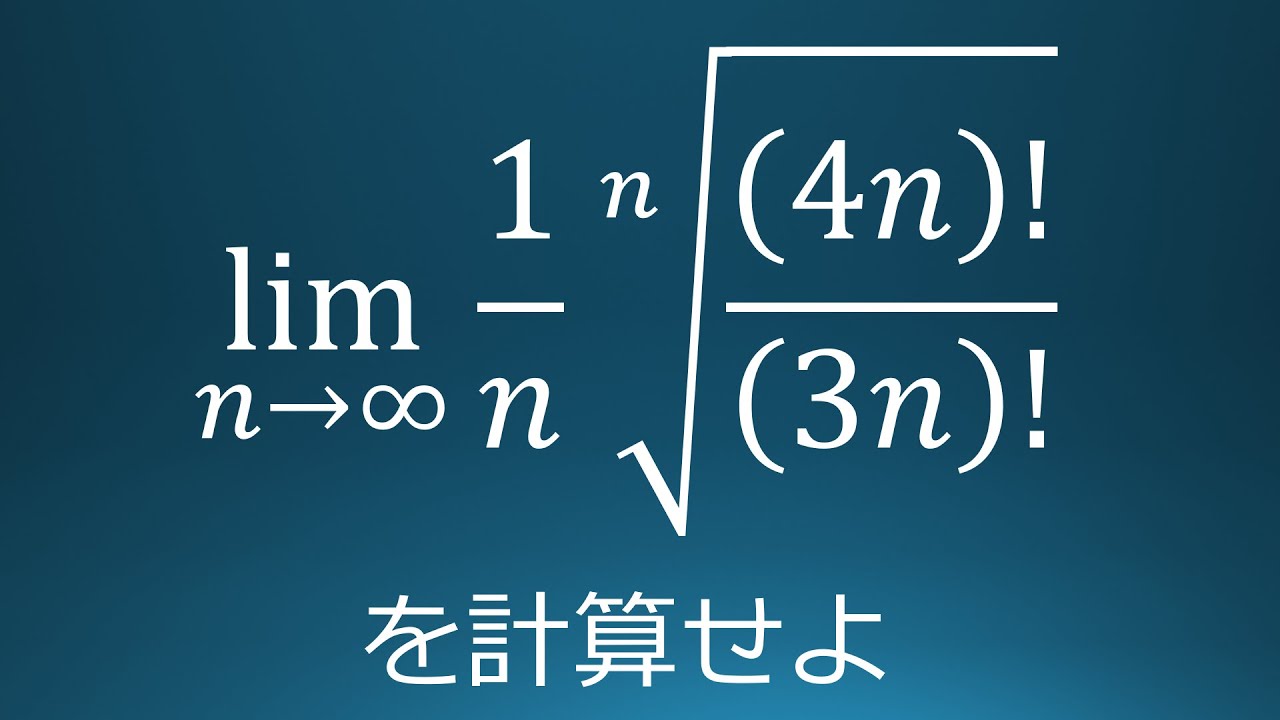
大学入試問題#118　防衛医科大学(2012)　区分求積法

単元： #大学入試過去問(数学)#関数と極限#積分とその応用#数列の極限#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#防衛医科大学

指導講師：ますただ

問題文全文(内容文)：

lim_{n \to \infty} \frac{1}{n} \sqrt[n]{\frac{(4 n)!}{(3 n)!}}

を求めよ。

出典：2012年防衛医科大学　入試問題

この動画を見る　

#50数検1級１次　過去問　重積分の積分順序の変更

単元： #数学検定・数学甲子園・数学オリンピック等#数学検定#数学検定1級

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{2} d y \int_{y}^{2} x \sqrt{x^{3} + 1} d x

を計算せよ。

この動画を見る　

大学入試問題#117　大分大学(2007)　整数問題

単元： #数A#大学入試過去問(数学)#整数の性質#学校別大学入試過去問解説(数学)#大分大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

x, y, z

：自然数

x ≦ y ≦ z

7 (x + y + z) = 2 (x y + y z + z x)

をみたす組

(x, y, z)

をすべて求めよ。

出典：2007年大分大学　入試問題

この動画を見る　

大学入試問題#116　岡山県立大学(2009)　定積分②

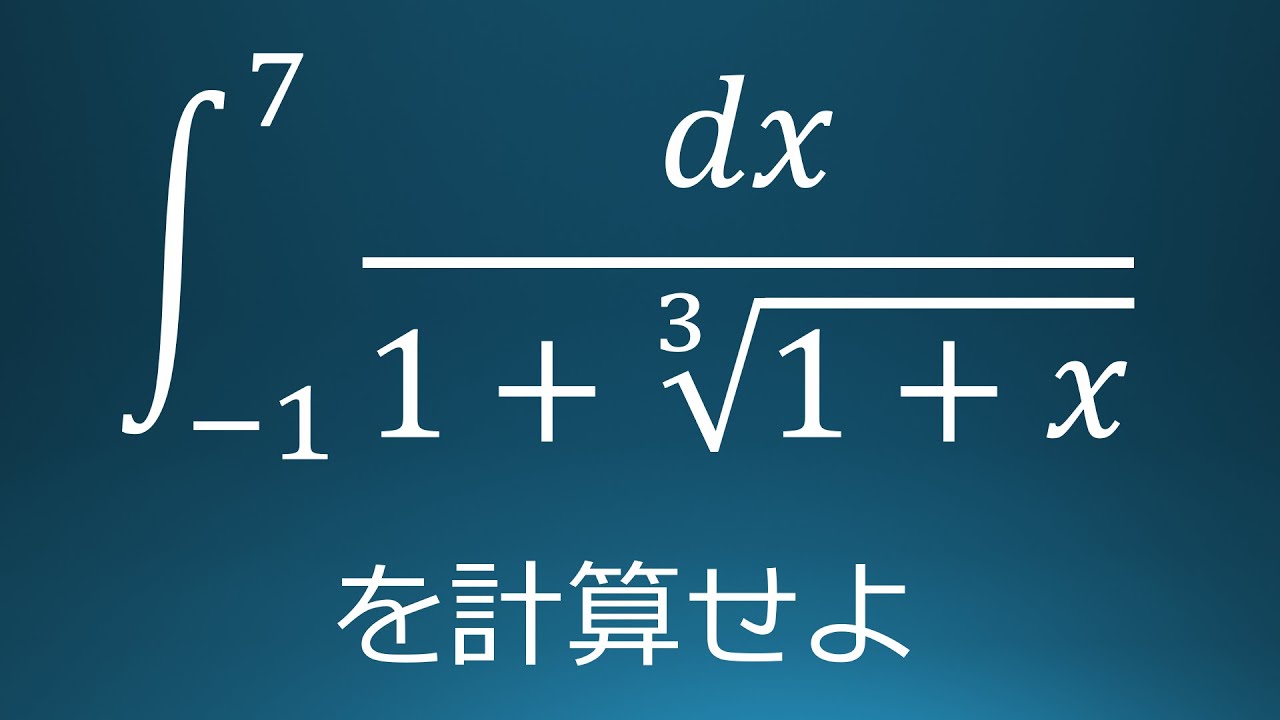
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#岡山県立大学

指導講師：ますただ

問題文全文(内容文)：

\int_{- 1}^{7} \frac{d x}{1 + \sqrt[3]{1 + x}}

を計算せよ。

出典：2009年岡山県立大学　入試問題

この動画を見る　

【概要欄に誘導あり】大学入試問題#115　京都大学(2002)　曲線の長さ（極方程式）

単元： #大学入試過去問(数学)#平面上の曲線#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数C

指導講師：ますただ

問題文全文(内容文)：
（1）

x ≧ 0

f (x) = l o g (x + \sqrt{1 + x^{2}})

を微分せよ。

（2）
極方程式

r = θ (0 ≦ θ ≦ π)

で定まる曲線の長さ

L

を求めよ。

出典：2002年京都大学　入試問題

この動画を見る　

数学ゴールデン#2【漫画】で紹介された数オリの問題の解答がなかったから作成してみた。

単元： #数学検定・数学甲子園・数学オリンピック等#数学オリンピック

指導講師：ますただ

問題文全文(内容文)：

\frac{\sqrt{10 + \sqrt{1}} + \sqrt{10 + \sqrt{2}} + ・ ・ ・ + \sqrt{10 + \sqrt{99}}}{\sqrt{10 - \sqrt{1}} + \sqrt{10 - \sqrt{2}} + ・ ・ ・ + \sqrt{10 - \sqrt{99}}}

を計算せよ。

出典：数学ゴールデン　数学オリンピック

この動画を見る　

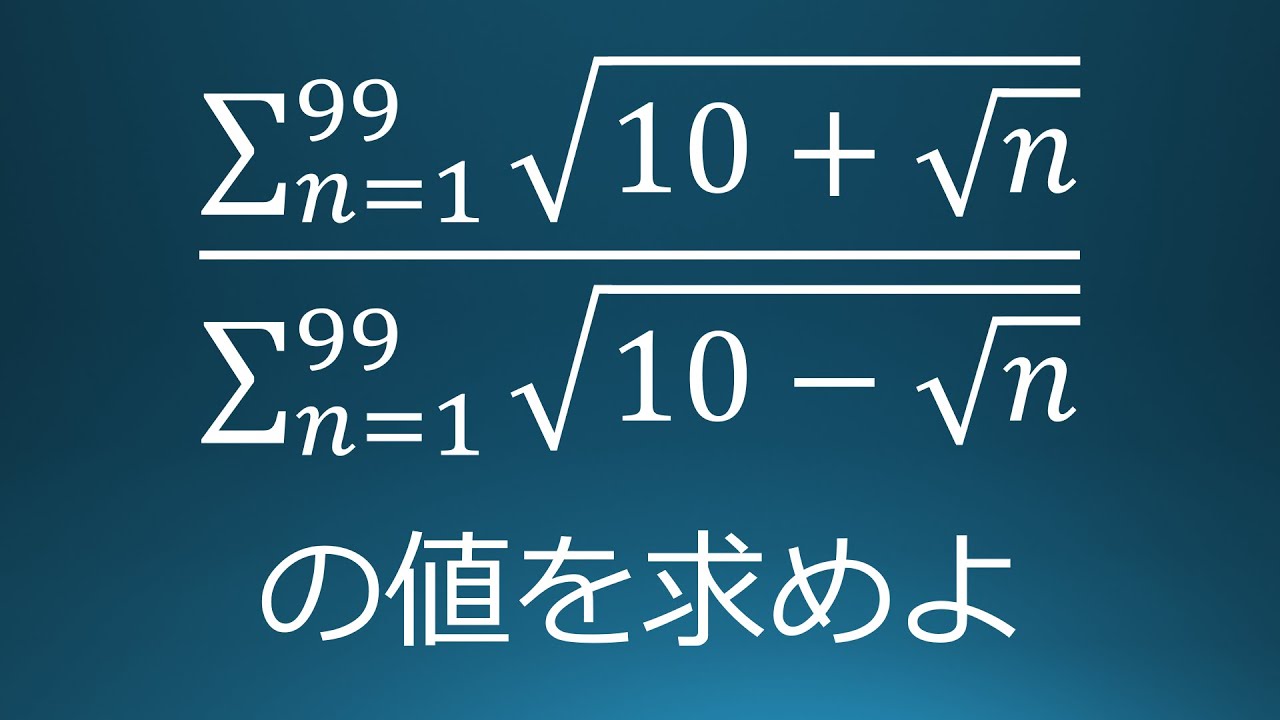
大学入試問題#114　岡山県立大学(2009)　定積分

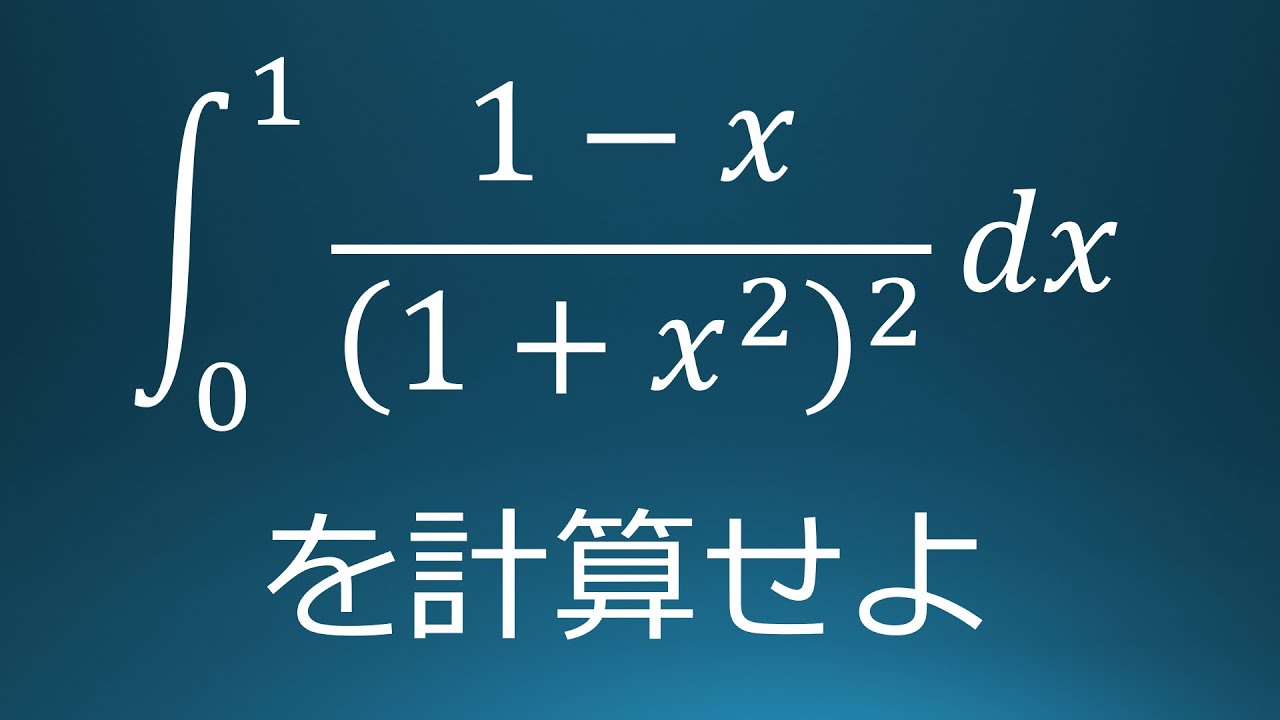
単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#岡山県立大学

指導講師：ますただ

問題文全文(内容文)：

\int_{0}^{1} \frac{1 - x}{(1 + x^{2})^{2}} d x

を計算せよ。

出典：2009年岡山県立大学　入試問題

この動画を見る　

大学入試問題#113　一橋大学(2011)　整数問題

単元： #数A#大学入試過去問(数学)#整数の性質#約数・倍数・整数の割り算と余り・合同式#学校別大学入試過去問解説(数学)#一橋大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

1 < x < y

(1 + \frac{1}{x}) (1 + \frac{1}{y}) = \frac{5}{3}

を満たす自然数の組

(x, y)

をすべて求めよ。

出典：2011年一橋大学　入試問題

この動画を見る　

大学入試問題#112　琉球大学(1989)　不定積分

単元： #大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#琉球大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

- 1 \neq α

：定数

\int \frac{(l o g x)^{α}}{x} l o g (\log x) d x

を計算せよ。

出典：1989年琉球大学　入試問題

この動画を見る　

√xは一様連続であることをε-δ論法で示す

単元：
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269

Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270

指導講師：ますただ

問題文全文(内容文)：

f : [0, \infty] \to R

f (x) = \sqrt{x}

で定めると

f

は一様連続であることを示す

この動画を見る　

大学入試問題#111　早稲田大学(2021)　次数下げ

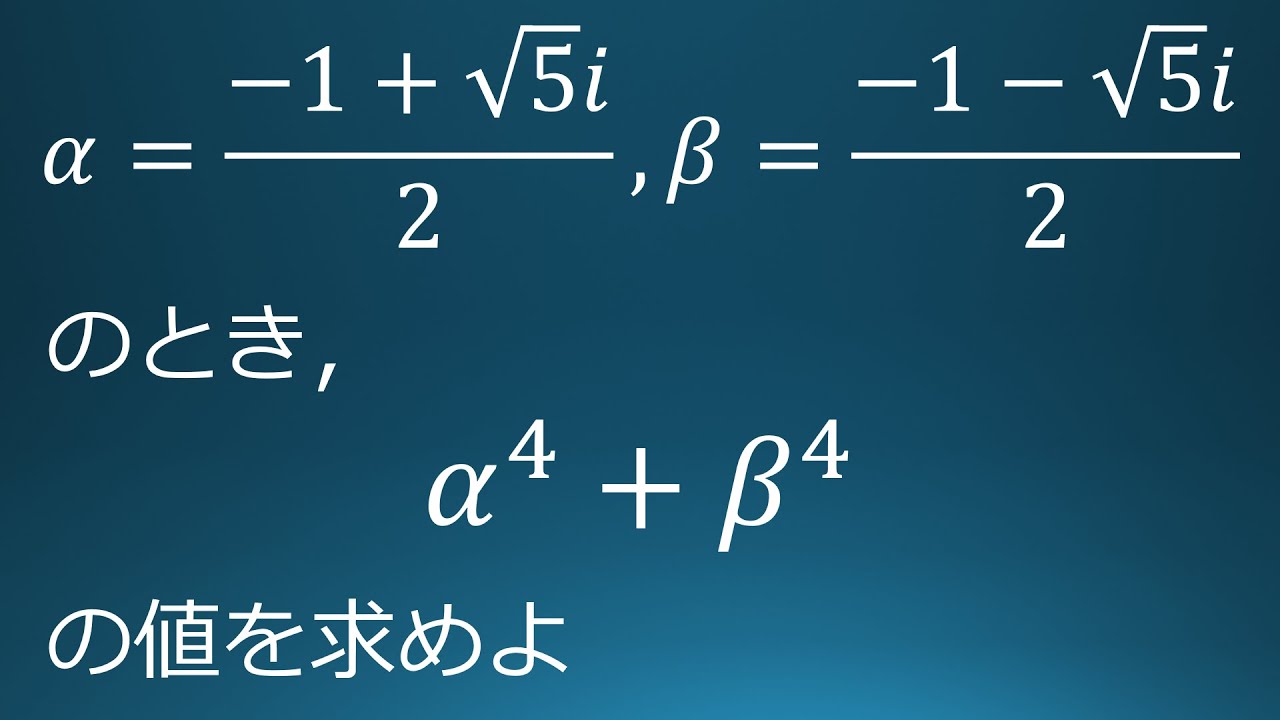
単元： #数Ⅱ#大学入試過去問(数学)#複素数と方程式#複素数#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

α = \frac{- 1 + \sqrt{5} i}{2}

β = \frac{- 1 - \sqrt{5} i}{2}

のとき

α^{4} + β^{4}

の値を求めよ。

出典：2021年早稲田大学　入試問題

この動画を見る　

大学入試問題#110　産業医科大学(2019)　定積分②

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学

指導講師：ますただ

問題文全文(内容文)：

\int_{- \frac{π}{4}}^{\frac{π}{4}} \tan^{4} x d x

を計算せよ。

出典：2019年産業医科大学　入試問題

この動画を見る　

大学入試問題#109　大阪府立大学(2010)　無限級数

単元： #大学入試過去問(数学)#関数と極限#数列の極限#学校別大学入試過去問解説(数学)#大阪府立大学#数学(高校生)#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

a_{1} = 1

a_{n + 1} = \frac{n}{n + 5} a_{n}

のとき

\sum_{n = 1}^{\infty} a_{n}

を求めよ

出典：2010年大阪府立大学　入試問題

この動画を見る　

大学入試問題#108　弘前大学(2018)　定積分

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#弘前大学#数Ⅲ

指導講師：ますただ

問題文全文(内容文)：

a > 0

\int_{0}^{1} \frac{d x}{(e^{2 x} + a) (e^{- 2 x} + a)}

を計算せよ。

出典：2018年弘前大学　入試問題

この動画を見る　

大学入試問題#107　産業医科大学(2019)　定積分①

単元： #大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学

指導講師：ますただ

問題文全文(内容文)：

\int_{99}^{101} \sqrt{(x - 99) (101 - x)} d x

を計算せよ。

出典：2019年産業医科大学　入試問題

この動画を見る　

大学入試問題#106　明治薬科大学(2004)　整数問題

単元： #数A#整数の性質#約数・倍数・整数の割り算と余り・合同式#数学(高校生)

指導講師：ますただ

問題文全文(内容文)：

l, m, n

：自然数

l ≦ m ≦ n

\frac{1}{l} + \frac{1}{m} + \frac{1}{n} = \frac{3}{2}

をみたす組

(l, m, n)

をすべて求めよ。

出典：2004年明治薬科大学　入試問題

この動画を見る　

大学入試問題#105　京都大学(2003)　数列

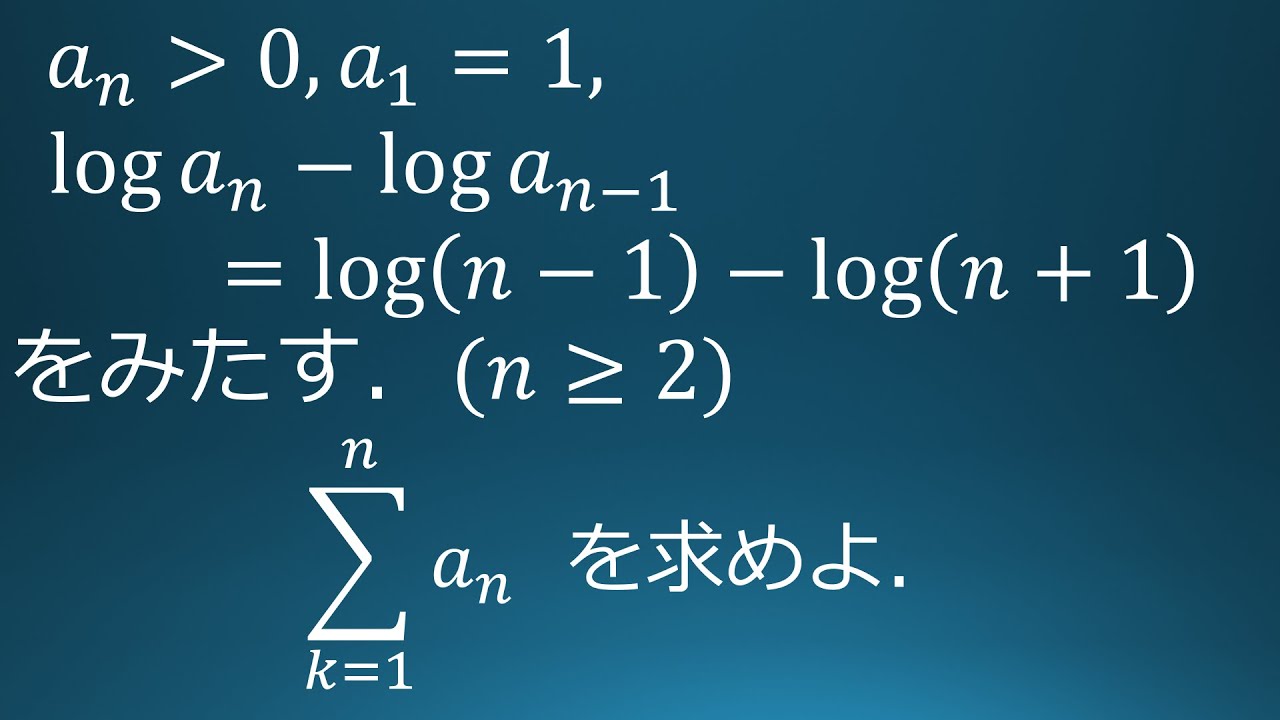
単元： #大学入試過去問(数学)#数列#漸化式#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数B

指導講師：ますただ

問題文全文(内容文)：

a_{n} > 0, a_{1} = 1

n ≧ 2

のとき

l o g a_{n} - l o g a_{n - 1} = l o g (n - 1) - l o g (n + 1)

である。

\sum_{k = 1}^{n} a_{k}

を求めよ

出典：2003年京都大学　入試問題

この動画を見る　

大学入試問題#104　一橋大学(2006)　ベクトル

単元： #大学入試過去問(数学)#平面上のベクトル#平面上のベクトルと内積#学校別大学入試過去問解説(数学)#一橋大学#数学(高校生)#数C

指導講師：ますただ

問題文全文(内容文)：

| \vec{a} | = 5, | \vec{b} | = 3, | \vec{c} | = 1

\vec{Z} = \vec{a} + \vec{b} + \vec{c}

（1）

| \vec{Z} |

の最大値、最小値
（2）

\vec{a} ・ \vec{Z} = 20

をみたすとき

| \vec{Z} |

の最大値、最小値を求めよ

出典：2006年一橋大学　入試問題

この動画を見る　

大学入試問題#103　東海大学医学部(2017)　二項定理

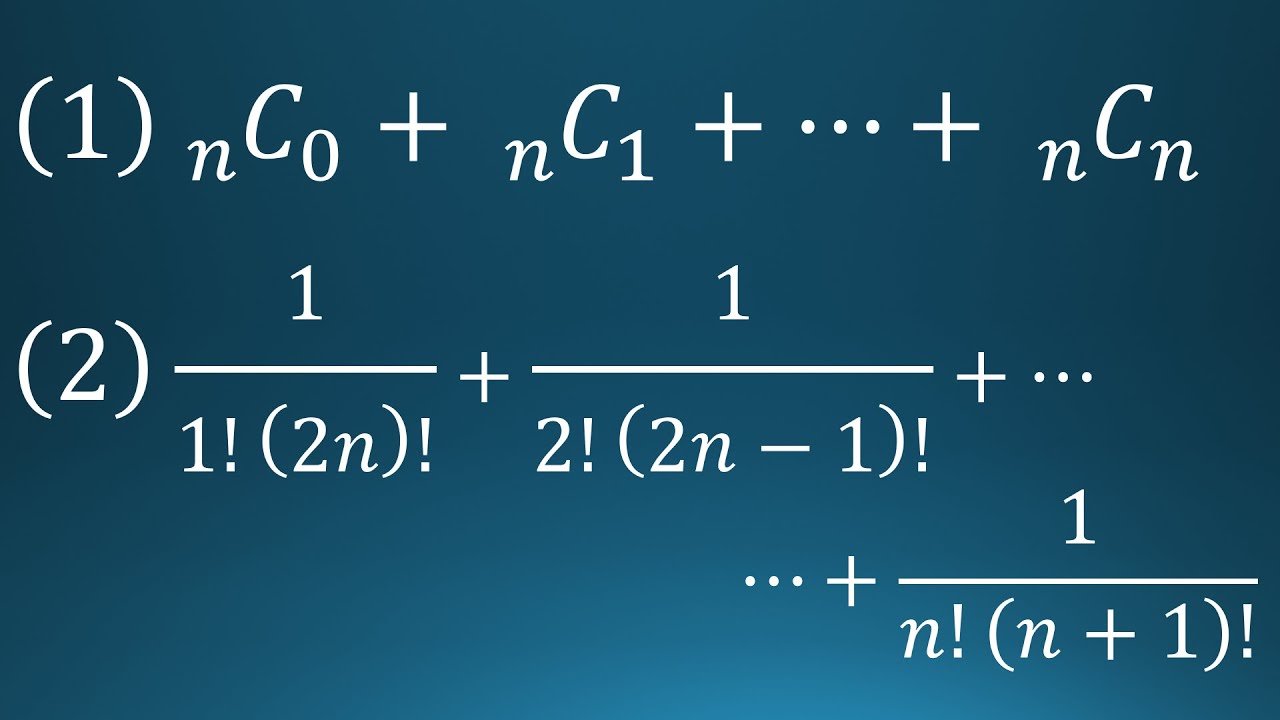
単元： #数Ⅱ#大学入試過去問(数学)#式と証明#整式の除法・分数式・二項定理#学校別大学入試過去問解説(数学)#数学(高校生)#東海大学

指導講師：ますただ

問題文全文(内容文)：
次の和を求めよ。
（1）

_{n} C_{0} +_{n} C_{1} + ・ ・ ・ +_{n} C_{n}

（2）

\frac{1}{1! (2 n)!} + \frac{1}{2 (2 n - 1)!} + ・ ・ ・ + \frac{1}{n! (n + 1)!}

出典：2017年東海大学医学部　入試問題

この動画を見る　

<img src="https://kaiketsu-db.net/wp-content/uploads/2021/11/112-book-morph-outline.gif" alt="" data-eio="l"> ますただ

大学入試問題#129 関西学院大学(1991) 二項定理の応用

大学入試問題#128 東京理科大学(2020) 定積分

大学入試問題#127 関西大学(1991) 整数問題

大学入試問題#126 慶應大学医学部(2005) 不定積分

大学入試問題#125 広島修道大学(2015) 整数問題

大学入試問題#124 高知大学(2020) 定積分

大学入試問題#123 鳥取大学 改 (2020) Σの計算

大学入試問題#122 愛知県立大学(2020) 極限

大学入試問題#121 横浜国立大学(2004) 定積分

大学入試問題#120 早稲田大学(2003) 対数の不等式

大学入試問題#119 横浜国立大学(2020) 定積分

大学入試問題#118 防衛医科大学(2012) 区分求積法

#50数検1級１次 過去問 重積分の積分順序の変更

大学入試問題#117 大分大学(2007) 整数問題

大学入試問題#116 岡山県立大学(2009) 定積分②

【概要欄に誘導あり】大学入試問題#115 京都大学(2002) 曲線の長さ（極方程式）

数学ゴールデン#2【漫画】で紹介された数オリの問題の解答がなかったから作成してみた。

大学入試問題#114 岡山県立大学(2009) 定積分

大学入試問題#113 一橋大学(2011) 整数問題

大学入試問題#112 琉球大学(1989) 不定積分

√xは一様連続であることをε-δ論法で示す

大学入試問題#111 早稲田大学(2021) 次数下げ

大学入試問題#110 産業医科大学(2019) 定積分②

大学入試問題#109 大阪府立大学(2010) 無限級数

大学入試問題#108 弘前大学(2018) 定積分

大学入試問題#107 産業医科大学(2019) 定積分①

大学入試問題#106 明治薬科大学(2004) 整数問題

大学入試問題#105 京都大学(2003) 数列

大学入試問題#104 一橋大学(2006) ベクトル

大学入試問題#103 東海大学医学部(2017) 二項定理

ますただ

大学入試問題#129　関西学院大学(1991)　二項定理の応用

大学入試問題#128　東京理科大学(2020)　定積分

大学入試問題#127　関西大学(1991)　整数問題

大学入試問題#126　慶應大学医学部(2005)　不定積分

大学入試問題#125　広島修道大学(2015)　整数問題

大学入試問題#124　高知大学(2020)　定積分

大学入試問題#123　鳥取大学　改　(2020)　Σの計算

大学入試問題#122　愛知県立大学(2020)　極限

大学入試問題#121　横浜国立大学(2004)　定積分

大学入試問題#120　早稲田大学(2003)　対数の不等式

大学入試問題#119　横浜国立大学(2020)　定積分

大学入試問題#118　防衛医科大学(2012)　区分求積法

#50数検1級１次　過去問　重積分の積分順序の変更

大学入試問題#117　大分大学(2007)　整数問題

大学入試問題#116　岡山県立大学(2009)　定積分②

【概要欄に誘導あり】大学入試問題#115　京都大学(2002)　曲線の長さ（極方程式）

大学入試問題#114　岡山県立大学(2009)　定積分

大学入試問題#113　一橋大学(2011)　整数問題

大学入試問題#112　琉球大学(1989)　不定積分

大学入試問題#111　早稲田大学(2021)　次数下げ

大学入試問題#110　産業医科大学(2019)　定積分②

大学入試問題#109　大阪府立大学(2010)　無限級数

大学入試問題#108　弘前大学(2018)　定積分

大学入試問題#107　産業医科大学(2019)　定積分①

大学入試問題#106　明治薬科大学(2004)　整数問題

大学入試問題#105　京都大学(2003)　数列

大学入試問題#104　一橋大学(2006)　ベクトル

大学入試問題#103　東海大学医学部(2017)　二項定理