東京医科大学
東京医科大学
大学入試問題#816「ほぼ直感通り！」 #東京医科大学(2011)
単元：
#数Ⅰ#大学入試過去問(数学)#数と式#一次不等式（不等式・絶対値のある方程式・不等式）#学校別大学入試過去問解説(数学)#数学(高校生)#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
すべての正の数$x,y$に対して、不等式
$\displaystyle \frac{K}{x+y} \leq \displaystyle \frac{1}{x}+\displaystyle \frac{49}{y}$
が成り立つような定数$K$の最大値を求めよ。
出典：2011年東京医科大学
この動画を見る
すべての正の数$x,y$に対して、不等式
$\displaystyle \frac{K}{x+y} \leq \displaystyle \frac{1}{x}+\displaystyle \frac{49}{y}$
が成り立つような定数$K$の最大値を求めよ。
出典：2011年東京医科大学
大学入試問題#808「難しすぎない良問」 #東京医科大学(2009) #整数問題
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
不等式$\sqrt{ n+1 }-\sqrt{ n } \gt \displaystyle \frac{1}{100}$を満たす正の整数$n$の最大値を求めよ。
出典：2009年東京医科大学 入試問題
この動画を見る
不等式$\sqrt{ n+1 }-\sqrt{ n } \gt \displaystyle \frac{1}{100}$を満たす正の整数$n$の最大値を求めよ。
出典：2009年東京医科大学 入試問題
大学入試問題#740「解き方色々」 東京医科大学(2024) 定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-2}^{1} (x+2)(x-1)^7 dx$
出典：2024年東京医科大学 入試問題
この動画を見る
$\displaystyle \int_{-2}^{1} (x+2)(x-1)^7 dx$
出典：2024年東京医科大学 入試問題
大学入試問題#671「方針が見えやすい良問」 東京医科大学(2001)
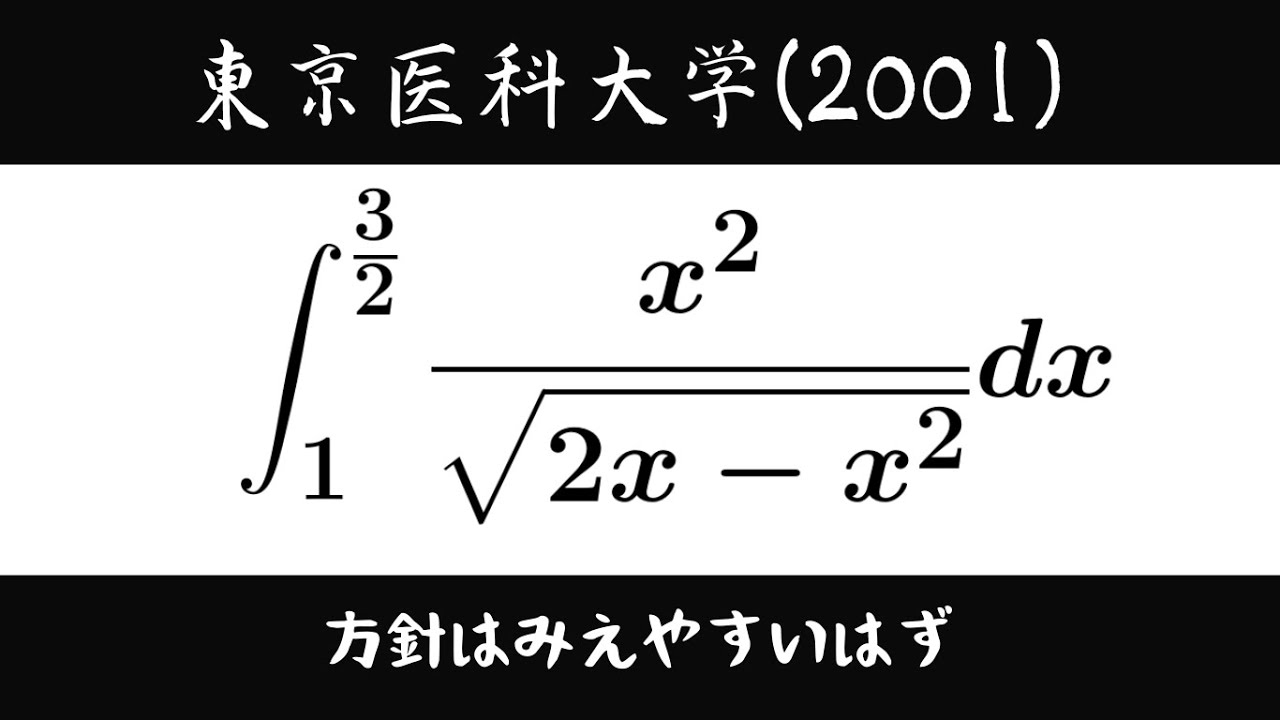
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#東京医科大学#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{\frac{3}{2}} \displaystyle \frac{x^2}{\sqrt{ 2x-x^2 }} dx$
出典：2001年東京医科大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{\frac{3}{2}} \displaystyle \frac{x^2}{\sqrt{ 2x-x^2 }} dx$
出典：2001年東京医科大学 入試問題
東京医科大 楽ちん問題
単元：
#数Ⅰ#数A#大学入試過去問(数学)#数と式#実数と平方根（循環小数・有理数・無理数・絶対値・平方根計算・2重根号）#整数の性質#学校別大学入試過去問解説(数学)#数学(高校生)#東京医科大学#東京医科大学
指導講師：
鈴木貫太郎
問題文全文(内容文)：
$a,b$は自然数であり、$\sqrt{ab}$は整数でないとき、
$\sqrt[3]{301\sqrt{a}-319\sqrt{b}}=\sqrt{a}-\sqrt{b}$
をみたす$a,b$を求めよ。
この動画を見る
$a,b$は自然数であり、$\sqrt{ab}$は整数でないとき、
$\sqrt[3]{301\sqrt{a}-319\sqrt{b}}=\sqrt{a}-\sqrt{b}$
をみたす$a,b$を求めよ。
東京医科大 見掛け倒しな問題
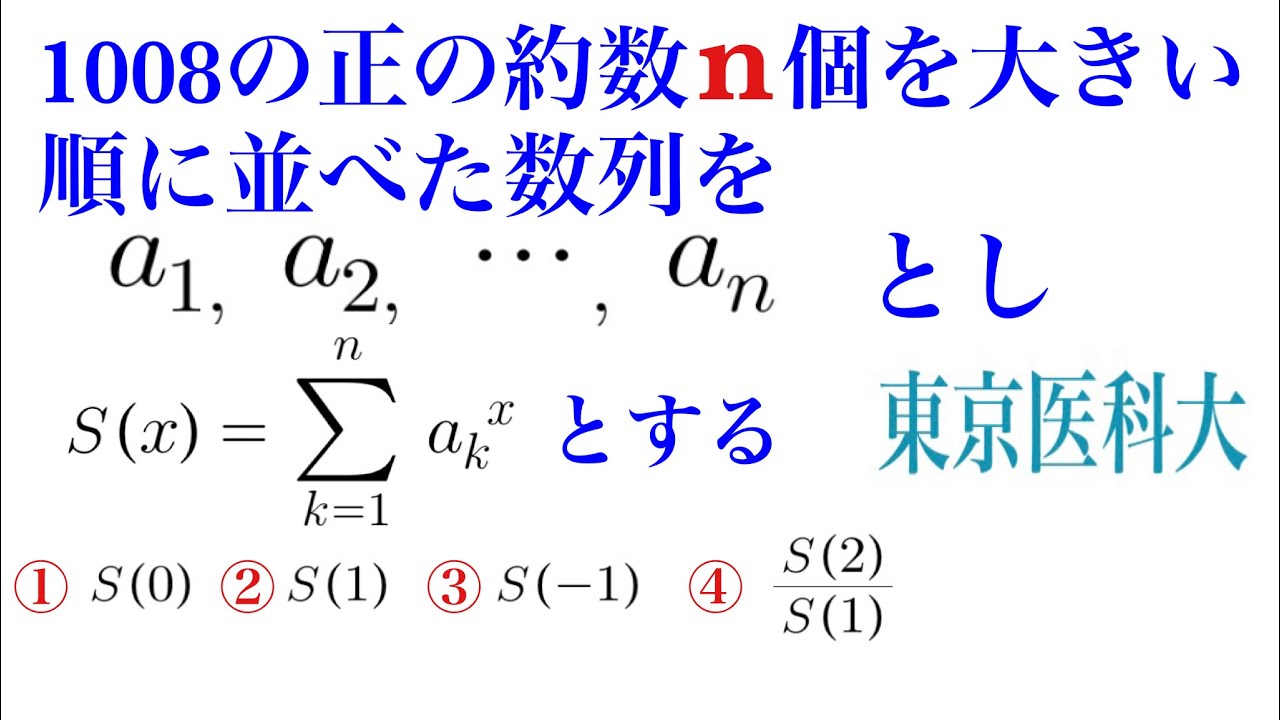
単元：
#大学入試過去問(数学)#数列#数列とその和（等差・等比・階差・Σ）#学校別大学入試過去問解説(数学)#数学(高校生)#数B#東京医科大学#東京医科大学
指導講師：
鈴木貫太郎
問題文全文(内容文)：
$1008$の正の約数$n$個を大きい順に並べた数列を
$a_1,a_2･･････,a_n$とし、$S(x)$を$S(x)=\displaystyle \sum_{k=1}^n a_k^x $とする。
①$S(0)$ ②$S(1)$ ③$S(-1)$ ④$\dfrac{S(2)}{S(1)}$
この動画を見る
$1008$の正の約数$n$個を大きい順に並べた数列を
$a_1,a_2･･････,a_n$とし、$S(x)$を$S(x)=\displaystyle \sum_{k=1}^n a_k^x $とする。
①$S(0)$ ②$S(1)$ ③$S(-1)$ ④$\dfrac{S(2)}{S(1)}$
大学入試問題#551「もはやオリジナル越えの芸術点高め！」 東京医科大学類題 By 英語orドイツ語シはBかHか さん #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} \displaystyle \frac{e^{\tan^2x+\sqrt{ \tan|x| }e^{\tan|x|}}}{1-\sin\ x} dx$
出典：2022年東京医科大学
この動画を見る
$\displaystyle \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} \displaystyle \frac{e^{\tan^2x+\sqrt{ \tan|x| }e^{\tan|x|}}}{1-\sin\ x} dx$
出典：2022年東京医科大学
東京医科大学 対数の極限
単元：
#大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学
指導講師：
鈴木貫太郎
問題文全文(内容文)：
$f(x)=(1+x)\log(3+x)-(1+x)\log(5+x)$
$\displaystyle \lim_{ n \to \infty } f(x) =?$
東京医科大過去問
この動画を見る
$f(x)=(1+x)\log(3+x)-(1+x)\log(5+x)$
$\displaystyle \lim_{ n \to \infty } f(x) =?$
東京医科大過去問
大学入試問題#153 東京医科大学(2017) 微積の応用
単元：
#大学入試過去問(数学)#微分とその応用#積分とその応用#微分法#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
$x \gt 0$
$f(x)=\displaystyle \int_{1}^{x}\displaystyle \frac{x+4t}{\sqrt{ 3x^4+t^4 }}\ dt$において$f'(x)$を求めよ。
出典：2017年東京医科大学 入試問題
この動画を見る
$x \gt 0$
$f(x)=\displaystyle \int_{1}^{x}\displaystyle \frac{x+4t}{\sqrt{ 3x^4+t^4 }}\ dt$において$f'(x)$を求めよ。
出典：2017年東京医科大学 入試問題
大学入試問題#92 東京医科大学(2015) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1}(x\sqrt{ 1-x^2 })^3 dx$を計算せよ。
出典：2015年東京医科大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}(x\sqrt{ 1-x^2 })^3 dx$を計算せよ。
出典：2015年東京医科大学 入試問題
数学「大学入試良問集」【17−1 隣接三項間漸化式と極限】を宇宙一わかりやすく
単元：
#大学入試過去問(数学)#関数と極限#数列の極限#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学#東京医科大学
指導講師：
ハクシ高校【数学科】良問演習チャンネル
問題文全文(内容文)：
$a_1=1,a_2=2,n \geqq 3$のとき$a_n=\displaystyle \frac{1}{5}(3a_{n-1}+2a_{n-2})$で定義される数列$\{a_n\}$の極限値を求めよ。
この動画を見る
$a_1=1,a_2=2,n \geqq 3$のとき$a_n=\displaystyle \frac{1}{5}(3a_{n-1}+2a_{n-2})$で定義される数列$\{a_n\}$の極限値を求めよ。
東京医科大 極限値
単元：
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#数学(高校生)#東京医科大学
指導講師：
鈴木貫太郎
問題文全文(内容文)：
$a_n=7n^2+n(n$自然数$)$
$\displaystyle \lim_{ n \to \infty } log(\displaystyle \frac{a_{n+1}-6}{a_n})^{9n}$
出典：東京医科大学 過去問
この動画を見る
$a_n=7n^2+n(n$自然数$)$
$\displaystyle \lim_{ n \to \infty } log(\displaystyle \frac{a_{n+1}-6}{a_n})^{9n}$
出典：東京医科大学 過去問
東京医科大 不等式
単元：
#数Ⅰ#大学入試過去問(数学)#数と式#一次不等式（不等式・絶対値のある方程式・不等式）#学校別大学入試過去問解説(数学)#数学(高校生)#東京医科大学
指導講師：
鈴木貫太郎
問題文全文(内容文)：
$\sqrt{ n+1 }-\sqrt{ n } \gt \displaystyle \frac{1}{100}$を満たす最大の自然数$n$を求めよ
出典：2009年東京医科大学 過去問
この動画を見る
$\sqrt{ n+1 }-\sqrt{ n } \gt \displaystyle \frac{1}{100}$を満たす最大の自然数$n$を求めよ
出典：2009年東京医科大学 過去問