 不定積分・定積分
不定積分・定積分
 不定積分・定積分
不定積分・定積分
#日本工業大学(2021) #定積分 #Shorts
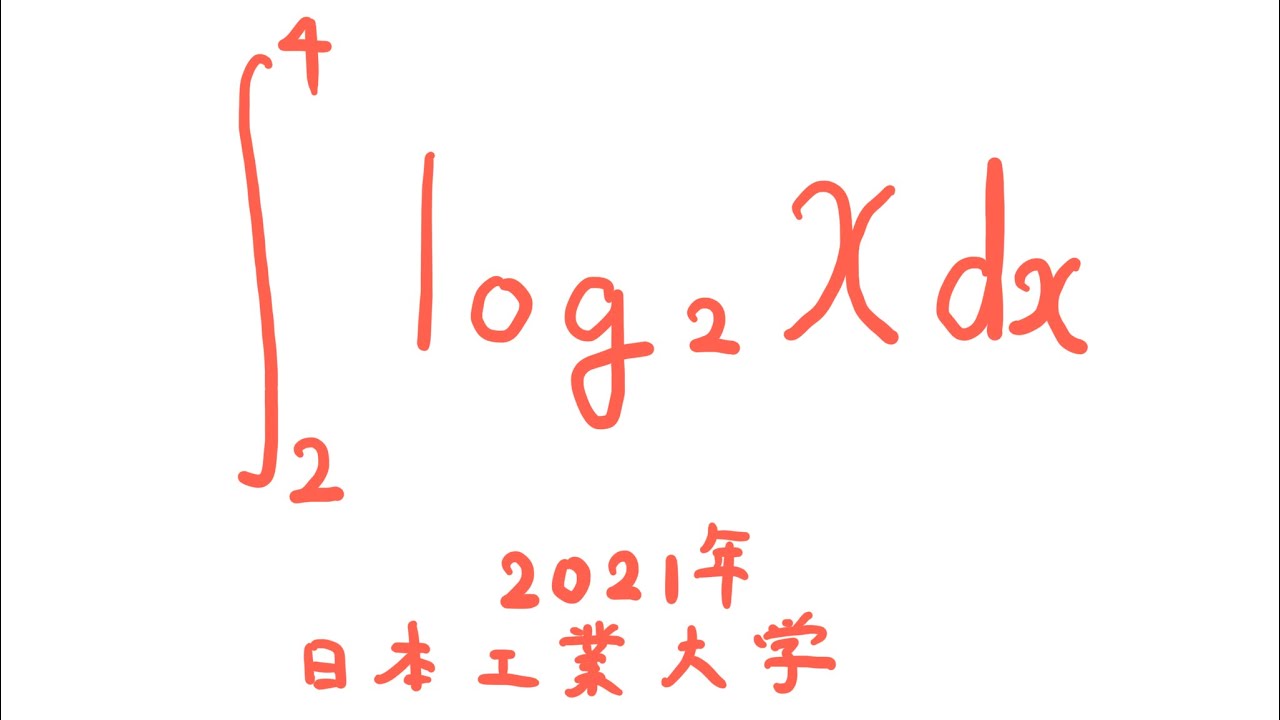
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{2}^{4} log_2\ x\ dx$
出典:2021年日本工業大学
この動画を見る
$\displaystyle \int_{2}^{4} log_2\ x\ dx$
出典:2021年日本工業大学
#61数検1級1次「よくできた問題」

単元:
#数Ⅱ#微分法と積分法#積分とその応用#不定積分#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$(x-1)^7-(x^7-1)$を実数係数の範囲で因数分解せよ
出典:数検1級1次
この動画を見る
$(x-1)^7-(x^7-1)$を実数係数の範囲で因数分解せよ
出典:数検1級1次
#会津大学(2009) #定積分 #Shorts
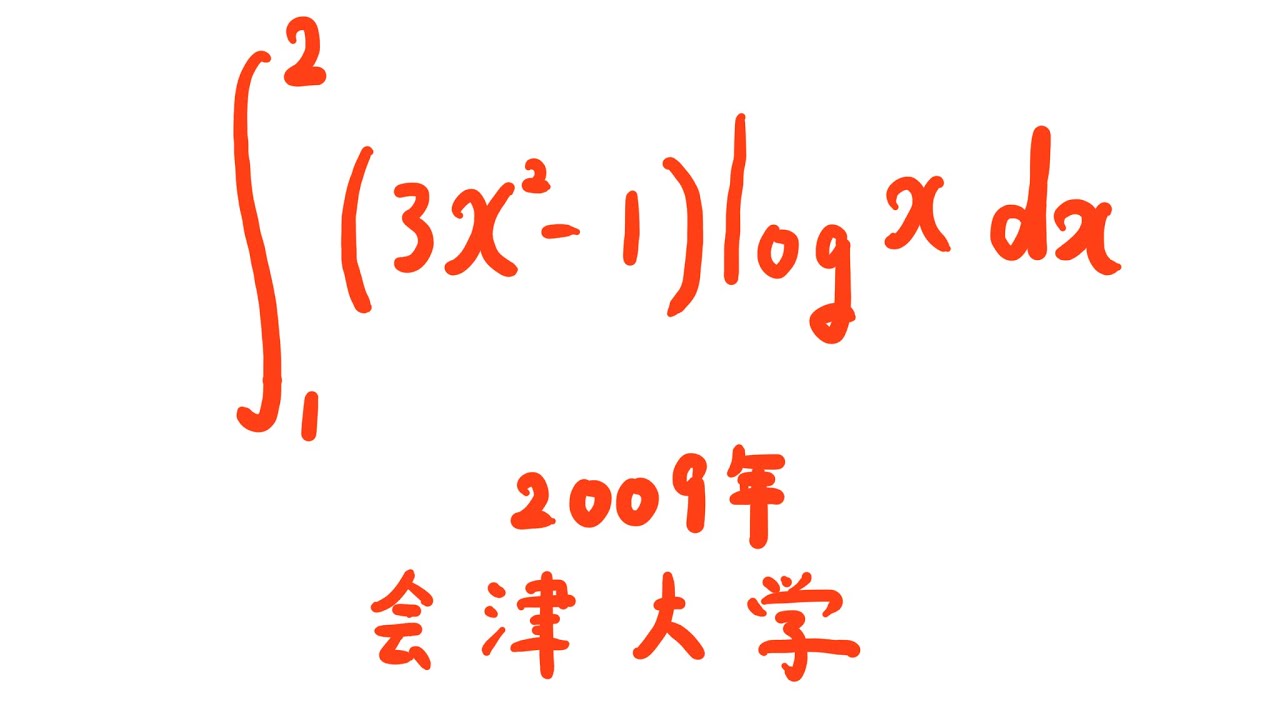
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{2} (3x^3-1)log\ x\ dx$
出典:2009年会津大学
この動画を見る
$\displaystyle \int_{1}^{2} (3x^3-1)log\ x\ dx$
出典:2009年会津大学
#59数検1級1次「国立大の入試問題の代表的な題材」

単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$n$を正の整数とするとき定積分
$\displaystyle \int_{0}^{1} (log_e\ x)^n\ dx$の値を$n$に関する式で表せ。
出典:数検1級1次
この動画を見る
$n$を正の整数とするとき定積分
$\displaystyle \int_{0}^{1} (log_e\ x)^n\ dx$の値を$n$に関する式で表せ。
出典:数検1級1次
大学入試問題#783「おもろいタイプ」 岡山県立大学中期(2011) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#岡山県立大学
指導講師:
ますただ
問題文全文(内容文):
$f(x)=\displaystyle \int_{0}^{x} \displaystyle \frac{1}{\sqrt{ 1-t^2 }}\ dt(0 \leq x \leq 1)$において
$\displaystyle \int_{0}^{\frac{1}{2}} f(x)\ dx$を求めよ
出典:2011年青山県立大学中期 入試問題
この動画を見る
$f(x)=\displaystyle \int_{0}^{x} \displaystyle \frac{1}{\sqrt{ 1-t^2 }}\ dt(0 \leq x \leq 1)$において
$\displaystyle \int_{0}^{\frac{1}{2}} f(x)\ dx$を求めよ
出典:2011年青山県立大学中期 入試問題
大学入試問題#782「もう何回目だろうか」 横浜市立大学(2004) #区分求積法

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#横浜市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ n \to \infty } \{\displaystyle \frac{(2n+1)(2n+2)・・・(2n+n)}{(n+1)(n+2)・・・(n+n)}\}^\frac{1}{n}$
出典:2004年横浜市立大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty } \{\displaystyle \frac{(2n+1)(2n+2)・・・(2n+n)}{(n+1)(n+2)・・・(n+n)}\}^\frac{1}{n}$
出典:2004年横浜市立大学 入試問題
#宮崎大学(2017) #定積分 #Shorts
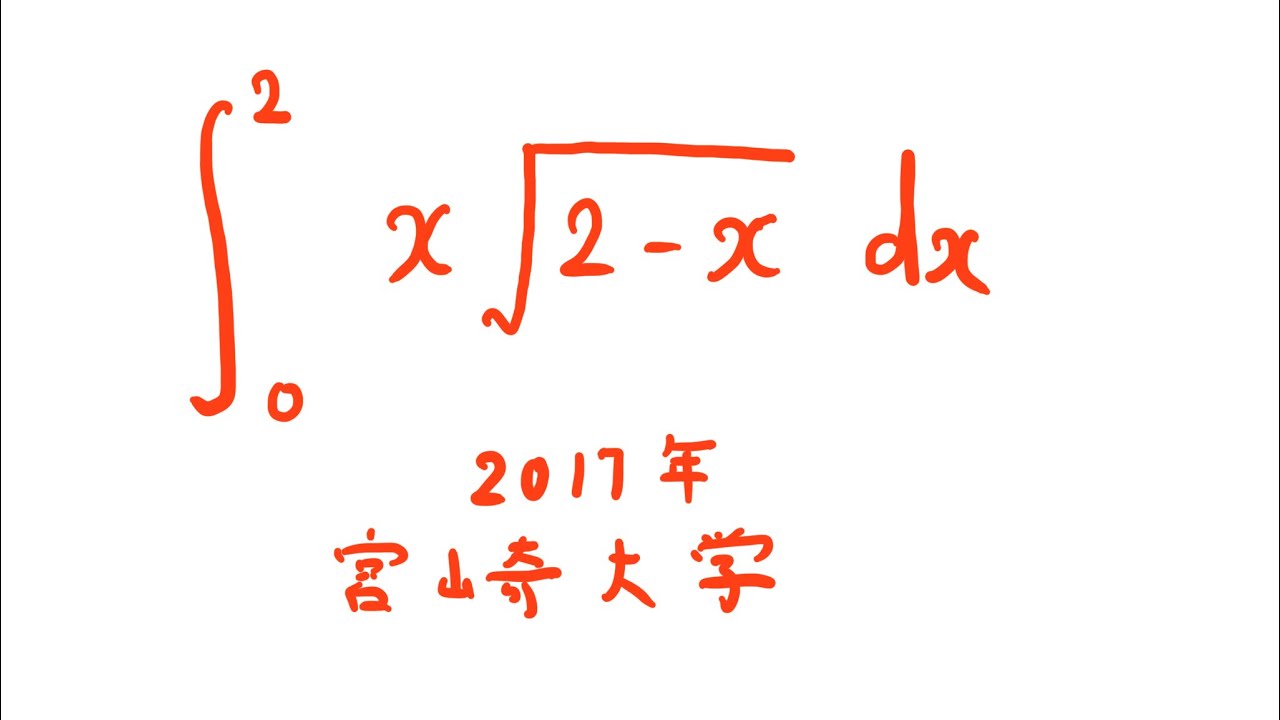
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{2} x\sqrt{ 2-x }\ dx$
出典:2017年宮崎大学
この動画を見る
$\displaystyle \int_{0}^{2} x\sqrt{ 2-x }\ dx$
出典:2017年宮崎大学
大学入試問題#781「絶対値付きの積分は、なんか苦手!」 久留米大学医学部(2005) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#久留米大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}} |\sin\ x-2\sin\ 2x|\ dx$
出典:2005年久留米大学医学部 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} |\sin\ x-2\sin\ 2x|\ dx$
出典:2005年久留米大学医学部 入試問題
大学入試問題#780「この当て方は、凄すぎ!横浜市立の先生は視聴者かな!?w」 横浜市立大学(2024) #定積分
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#横浜市立大学
指導講師:
ますただ
問題文全文(内容文):
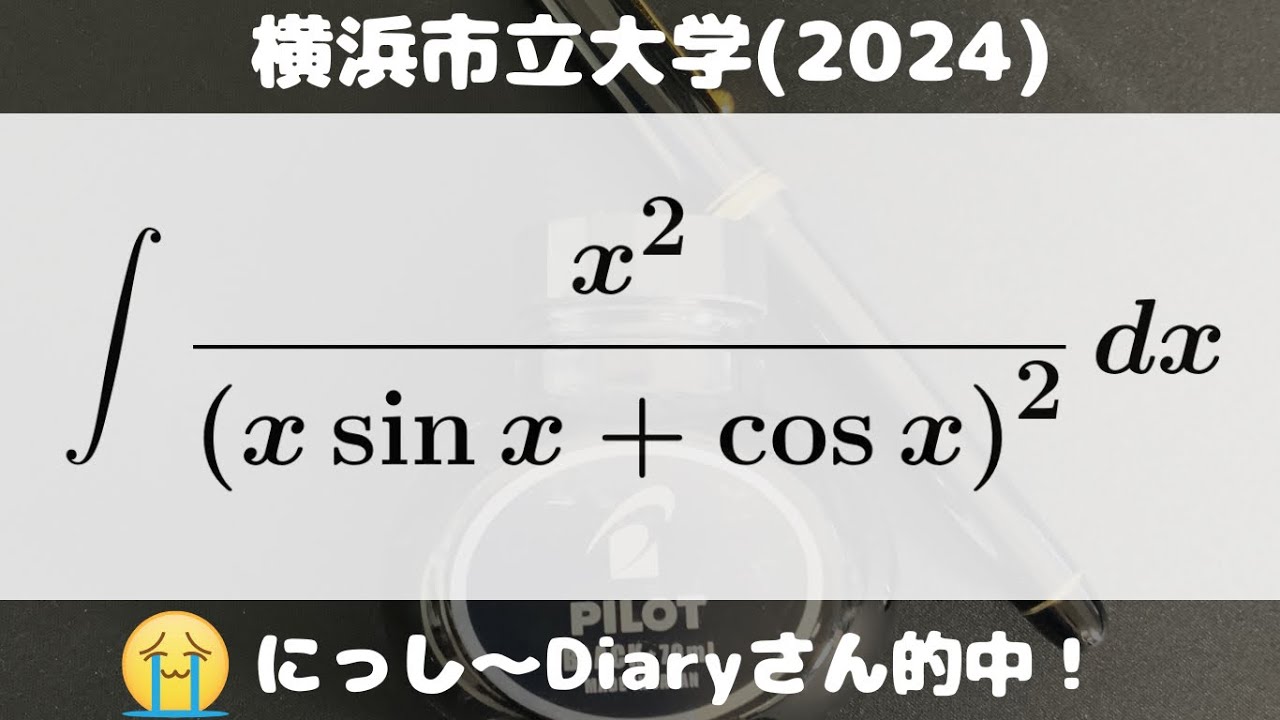
$\displaystyle \int \displaystyle \frac{x^2}{(x\ \sin\ x+\cos\ x)^2} dx$
出典:2024年横浜市立大学
この動画を見る
$\displaystyle \int \displaystyle \frac{x^2}{(x\ \sin\ x+\cos\ x)^2} dx$
出典:2024年横浜市立大学
#広島市立大学(2011) #定積分 #Shorts
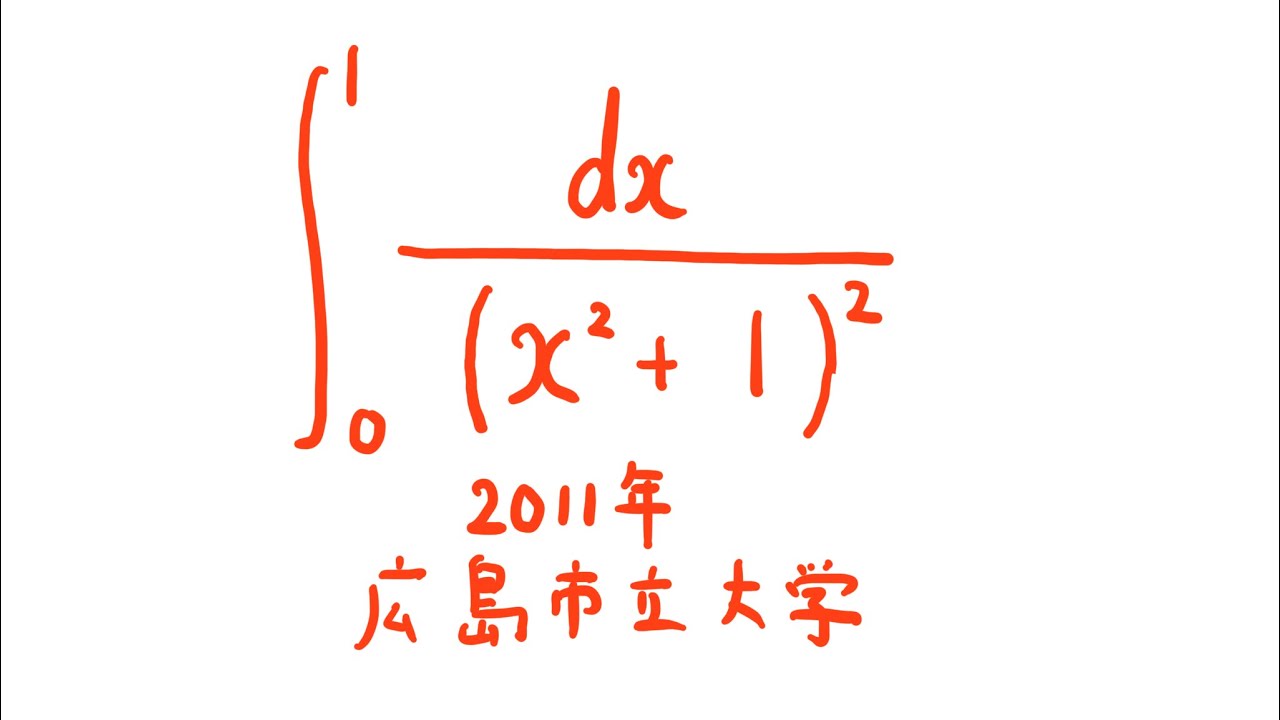
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} \displaystyle \frac{dx}{(x^2+1)^2}$
出典:2011年広島市立大学
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{dx}{(x^2+1)^2}$
出典:2011年広島市立大学
大学入試問題#778「ウォリス積分なら一撃」 横浜国立大学(1994) #定積分
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
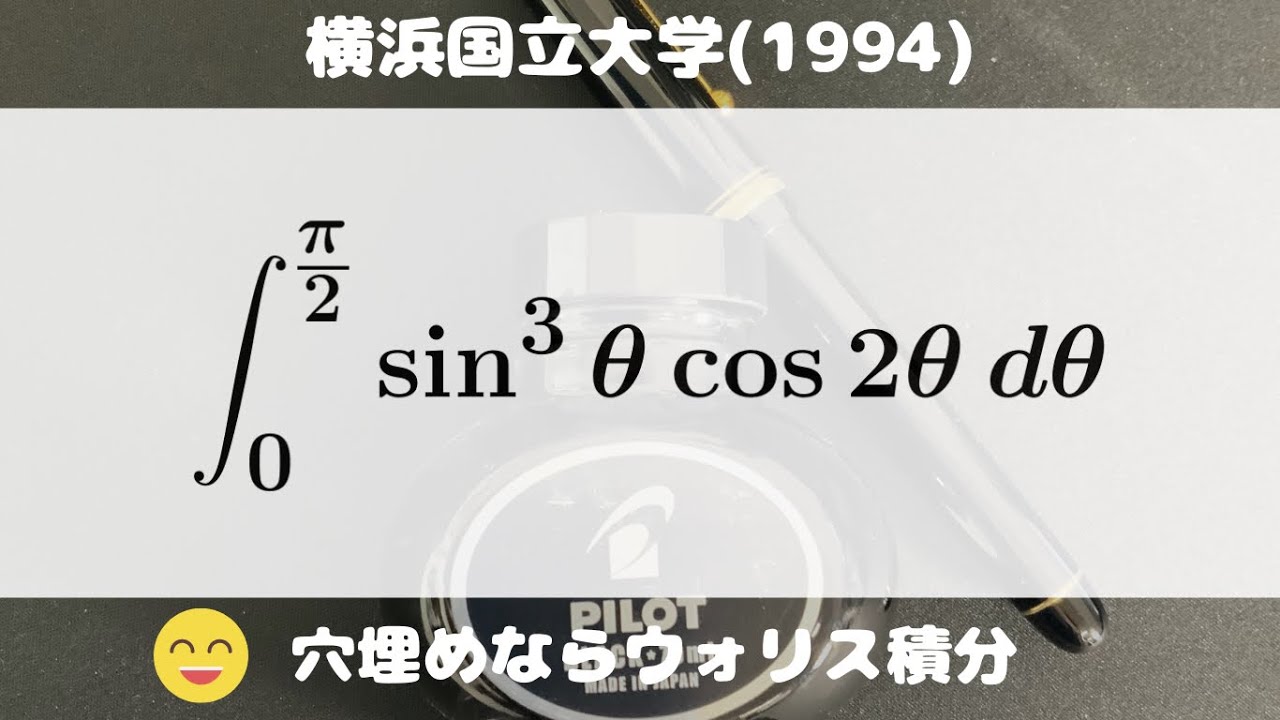
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sin^3\theta\ \cos2\theta\ d\theta$
出典:1994年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sin^3\theta\ \cos2\theta\ d\theta$
出典:1994年横浜国立大学 入試問題
大学入試問題#776「シグマの気持ち」 横浜国立大学(1996)
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
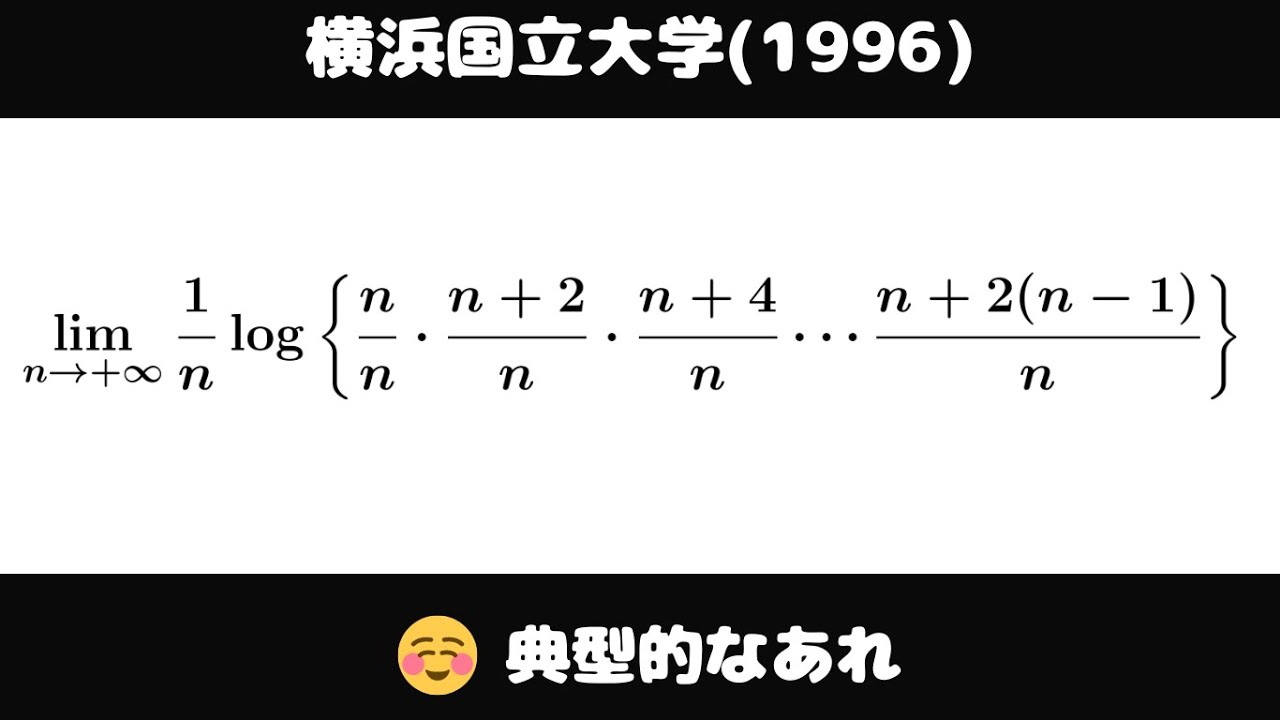
$\displaystyle \lim_{ n \to +\infty } \displaystyle \frac{1}{n}log\{\displaystyle \frac{n}{n}・\displaystyle \frac{n+2}{n}・\displaystyle \frac{n+4}{n}・・・\displaystyle \frac{n+2(n-1)}{n}\}$
出典:1996年横浜国立大学
この動画を見る
$\displaystyle \lim_{ n \to +\infty } \displaystyle \frac{1}{n}log\{\displaystyle \frac{n}{n}・\displaystyle \frac{n+2}{n}・\displaystyle \frac{n+4}{n}・・・\displaystyle \frac{n+2(n-1)}{n}\}$
出典:1996年横浜国立大学
#広島市立大学(2016) #定積分 #Shorts
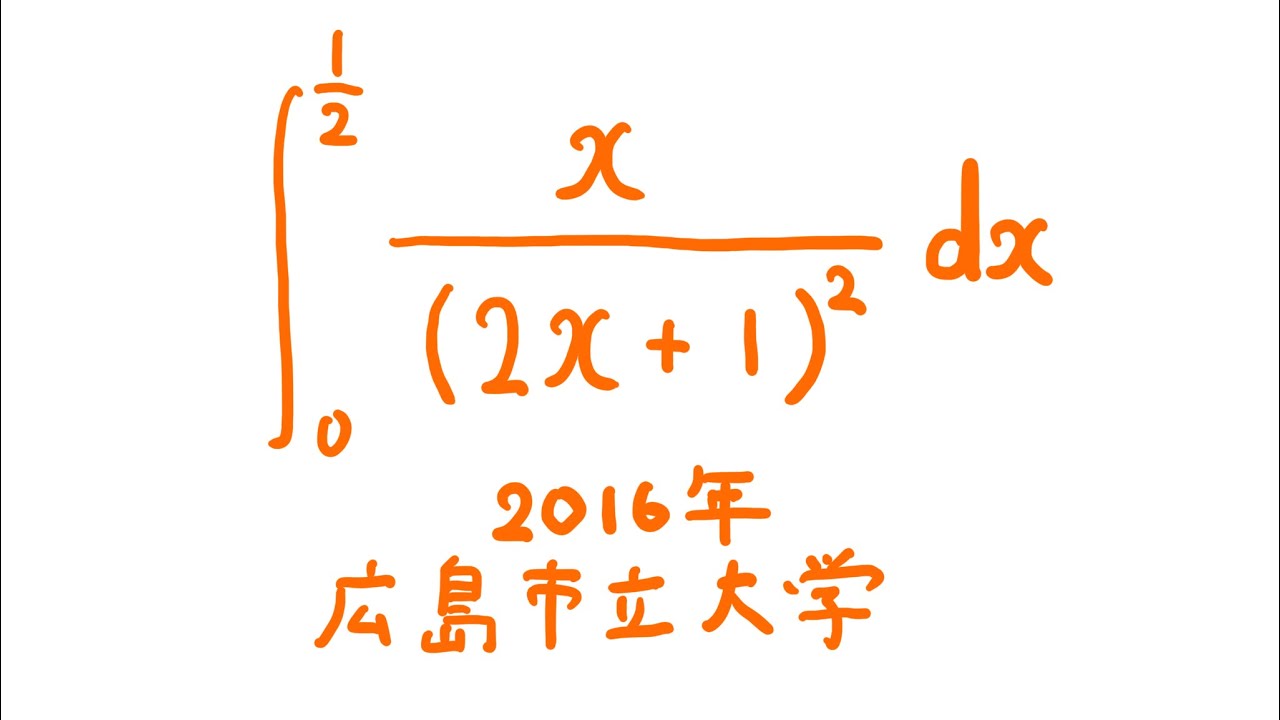
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{1}{2}} \displaystyle \frac{x}{(2x+1)^2} dx$
出典:2016年広島市立大学
この動画を見る
$\displaystyle \int_{0}^{\frac{1}{2}} \displaystyle \frac{x}{(2x+1)^2} dx$
出典:2016年広島市立大学
大学入試問題#775「ほぼ、詰んでる」 横浜国立大学(1998) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{2\pi} x^2|\sin\ x|\ dx$
出典:1998年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{2\pi} x^2|\sin\ x|\ dx$
出典:1998年横浜国立大学 入試問題
大学入試問題#774「基本的な良問」 横浜国立大学(1998) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{e-1} \displaystyle \frac{log(log(x+1))}{x+1} dx$
出典:1998年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{e-1} \displaystyle \frac{log(log(x+1))}{x+1} dx$
出典:1998年横浜国立大学 入試問題
#広島市立大学(2016) #定積分 #Shorts
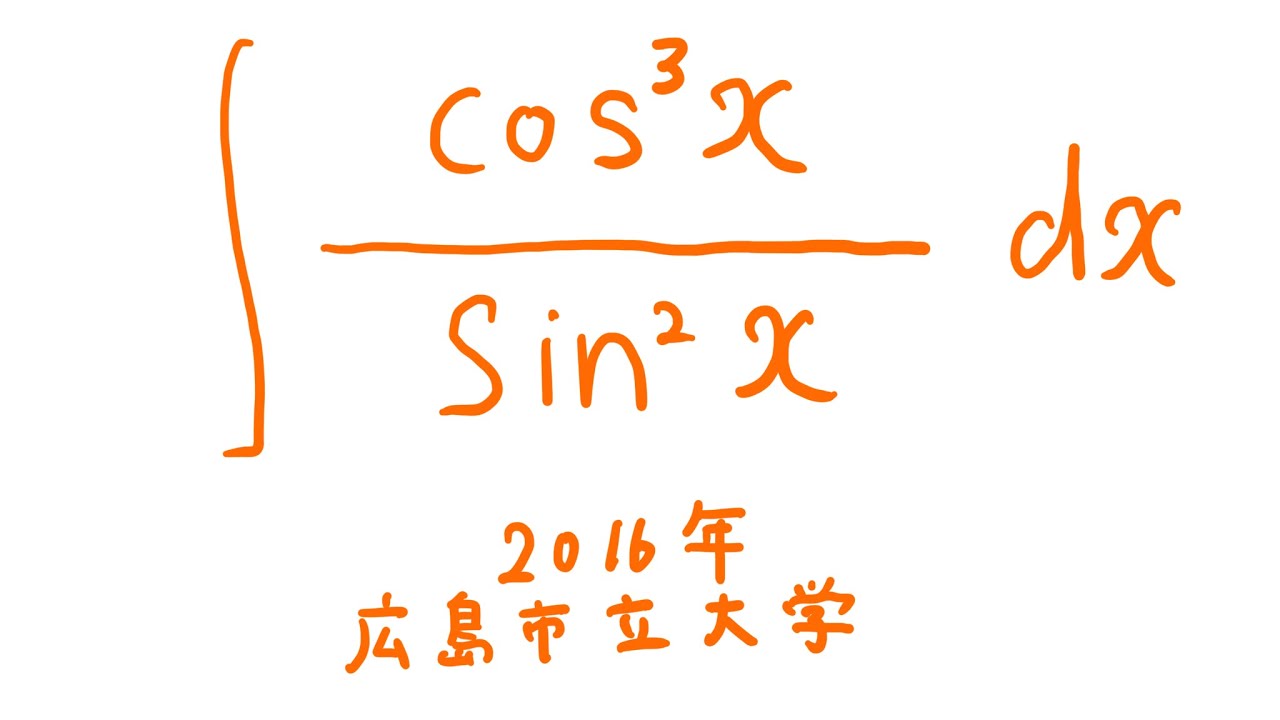
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{\cos^3\ x}{\sin^2\ x} dx$
出典:2016年広島市立大学
この動画を見る
$\displaystyle \int \displaystyle \frac{\cos^3\ x}{\sin^2\ x} dx$
出典:2016年広島市立大学
大学入試問題#772「初手は好みがでそう」 広島市立大学(2012) #不定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{log\ x}{\sqrt[ 3 ]{ x }} dx$
出典:2012年広島市立大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{log\ x}{\sqrt[ 3 ]{ x }} dx$
出典:2012年広島市立大学 入試問題
#会津大学(2023) #定積分 #Shorts
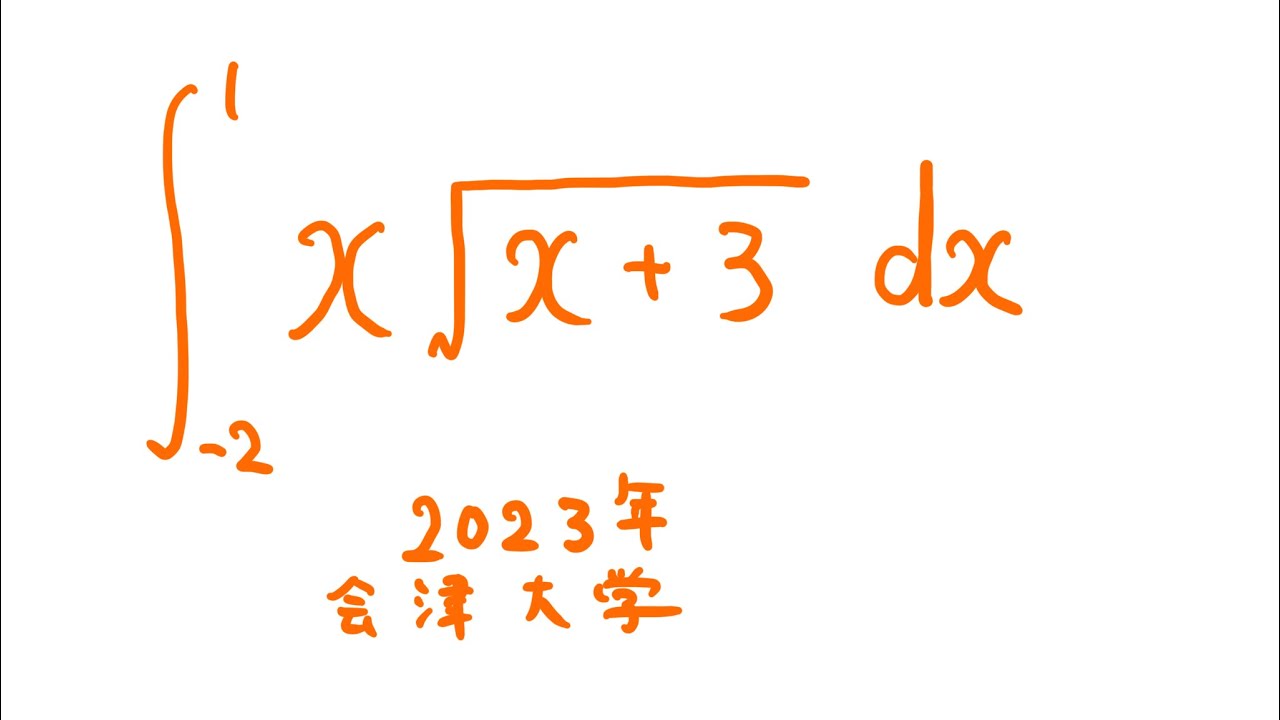
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-2}^{1} x\sqrt{ x+3 }\ dx$
出典:2023年会津大学
この動画を見る
$\displaystyle \int_{-2}^{1} x\sqrt{ x+3 }\ dx$
出典:2023年会津大学
大学入試問題#771「たぶん良問!」 島根大学後期(2023) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#島根大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} \displaystyle \frac{2x-2}{2x^2-2x+1} dx$
出典:2023年島根大学後期 入試問題
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{2x-2}{2x^2-2x+1} dx$
出典:2023年島根大学後期 入試問題
#会津大学 2020年 #定積分 #Shorts
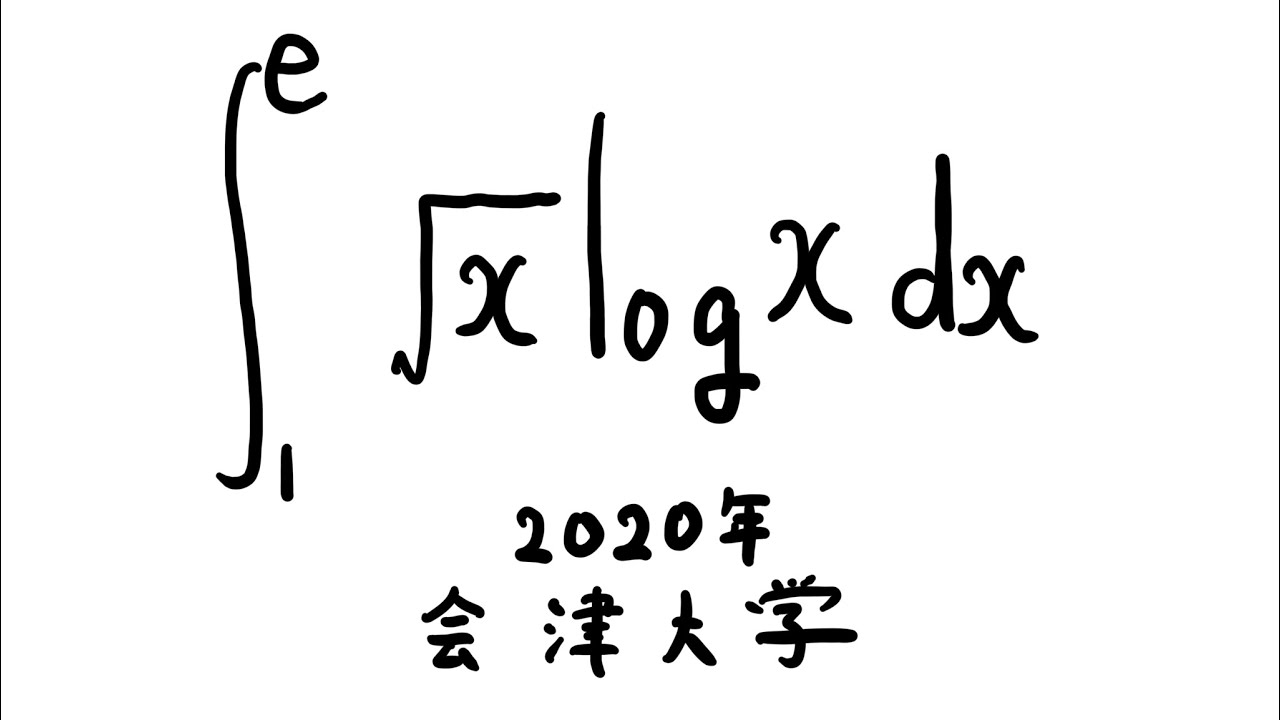
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{e} \sqrt{ x }\ log\ x\ dx$
出典:2020年会津大学
この動画を見る
$\displaystyle \int_{1}^{e} \sqrt{ x }\ log\ x\ dx$
出典:2020年会津大学
大学入試問題#770「減点注意!」 千葉大学(2003) #微積の応用

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$a$は定数とし、$n$は2以上の整数とする。
関数$f(x)=ax^n log\ x-ax(x \gt 0)$の最小値が-1のとき、定積分$\displaystyle \int_{1}^{e} f(x)\ dx$の値を$n$と$e$を用いて表せ。
出典:2003年千葉大学 入試問題
この動画を見る
$a$は定数とし、$n$は2以上の整数とする。
関数$f(x)=ax^n log\ x-ax(x \gt 0)$の最小値が-1のとき、定積分$\displaystyle \int_{1}^{e} f(x)\ dx$の値を$n$と$e$を用いて表せ。
出典:2003年千葉大学 入試問題
#会津大学 2023年 #定積分 #Shorts
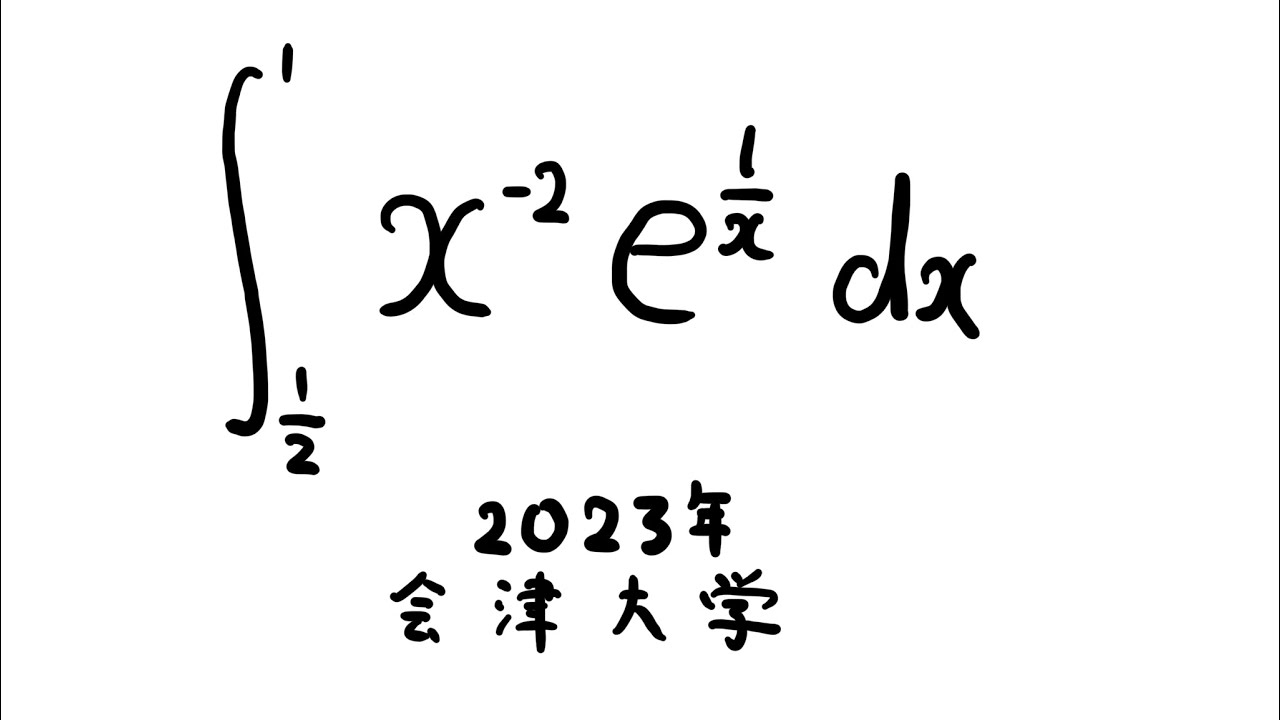
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{1}{2}}^{1} x^{-2}e^{\frac{1}{x}} dx$
出典:2023年会津大学
この動画を見る
$\displaystyle \int_{\frac{1}{2}}^{1} x^{-2}e^{\frac{1}{x}} dx$
出典:2023年会津大学
大阪大学2023年の積分に見えない積分難問にガチで挑んでみた!#shorts #高校数学 #大阪大学
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
大阪大学2023年の積分に見えない積分難問にガチで挑んでみた!
この動画を見る
大阪大学2023年の積分に見えない積分難問にガチで挑んでみた!
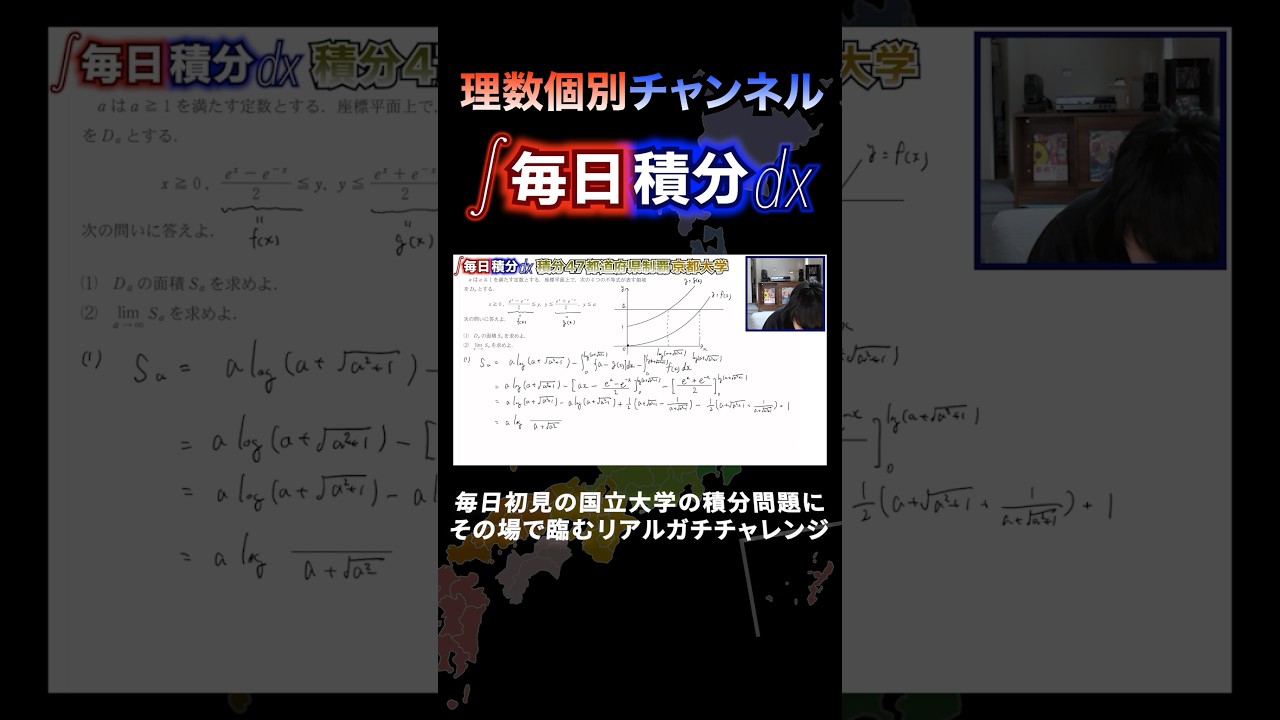
京都大学2024年の積分の問題をその場で解きながら解説してみた! #shorts #高校数学 #京都大学
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
京都大学2024年の積分の問題をその場で解きながら解説してみた!
この動画を見る
京都大学2024年の積分の問題をその場で解きながら解説してみた!
【短時間でポイントチェック!!】絶対値を含む定積分〔現役講師解説、数学〕

単元:
#数Ⅱ#数と式#実数と平方根(循環小数・有理数・無理数・絶対値・平方根計算・2重根号)#微分法と積分法#不定積分・定積分#数学(高校生)
指導講師:
3rd School
問題文全文(内容文):
$\int_1^3{|x^2-4|}dx$
この動画を見る
$\int_1^3{|x^2-4|}dx$
【短時間でポイントチェック!!】定積分 面積③ 曲線と曲線で囲まれた面積〔現役講師解説、数学〕

単元:
#数Ⅱ#微分法と積分法#不定積分・定積分#面積、体積#数学(高校生)
指導講師:
3rd School
問題文全文(内容文):
$y=x^2-2,y=-x^2-2x+2$で囲まれた部分の面積は?
この動画を見る
$y=x^2-2,y=-x^2-2x+2$で囲まれた部分の面積は?
【短時間でポイントチェック!!】定積分 面積② 直線と曲線で囲まれた面積〔現役講師解説、数学〕

単元:
#数Ⅱ#微分法と積分法#不定積分・定積分#面積、体積#数学(高校生)
指導講師:
3rd School
問題文全文(内容文):
$y=x^2-x-4,y=x-1$で囲まれた部分の面積
この動画を見る
$y=x^2-x-4,y=x-1$で囲まれた部分の面積
【高校数学】毎日積分76日目~47都道府県制覇への道~【⑲大阪】【毎日17時投稿】

単元:
#数Ⅱ#微分法と積分法#不定積分・定積分#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
■【大阪大学 2023】
$n$を2以上の自然数とする。
(1)$0\leqq x\leqq 1$の時、次の不等式が成り立つことを示せ。
$\dfrac{1}{2}x^n\leqq (-1)^n\left[\dfrac{1}{x+1}-1-\displaystyle \sum_{k=2}^n(-1)^{k-1}\right]\leqq x^n-\dfrac{1}{2}x^{n+1}$
(2)$a_n=\displaystyle \sum_{k=1}^n\dfrac{(-1)^{k-1}}{k}$とするとき、次の極限値を求めよ。
$\lim_{n\to\infty}(-1)^n n(a_n-\log 2)$
この動画を見る
■【大阪大学 2023】
$n$を2以上の自然数とする。
(1)$0\leqq x\leqq 1$の時、次の不等式が成り立つことを示せ。
$\dfrac{1}{2}x^n\leqq (-1)^n\left[\dfrac{1}{x+1}-1-\displaystyle \sum_{k=2}^n(-1)^{k-1}\right]\leqq x^n-\dfrac{1}{2}x^{n+1}$
(2)$a_n=\displaystyle \sum_{k=1}^n\dfrac{(-1)^{k-1}}{k}$とするとき、次の極限値を求めよ。
$\lim_{n\to\infty}(-1)^n n(a_n-\log 2)$
【高校数学】毎日積分74日目~47都道府県制覇への道~【九州~四国・中国地方総集編(テーマ別)】【毎日17時投稿】

【短時間でポイントチェック!!】定積分 面積①〔現役講師解説、数学〕

単元:
#数Ⅱ#微分法と積分法#不定積分・定積分#面積、体積#数学(高校生)
指導講師:
3rd School
問題文全文(内容文):
$y=x^2-3x$と$x$軸および$x=1,x=4$で囲まれた面積は?
この動画を見る
$y=x^2-3x$と$x$軸および$x=1,x=4$で囲まれた面積は?
