 積分とその応用
積分とその応用
 積分とその応用
積分とその応用
#東京理科大学2023#定積分#ますただ

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#東京理科大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\sqrt{ e }} \displaystyle \frac{e}{x^2+e} dx$
出典:2023年東京理科大学
この動画を見る
$\displaystyle \int_{0}^{\sqrt{ e }} \displaystyle \frac{e}{x^2+e} dx$
出典:2023年東京理科大学
#数検準1級-1#定積分#ますただ
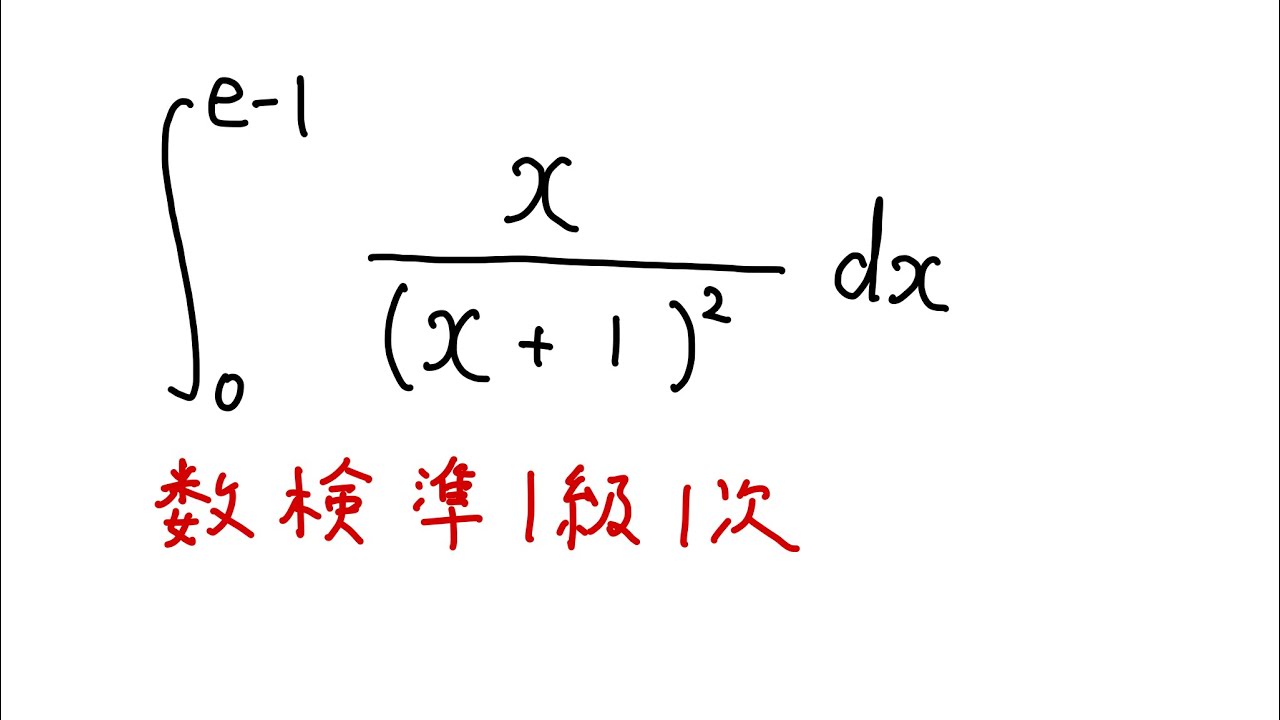
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{e-1} \displaystyle \frac{x}{(x+1)^2} dx$
出典:数検準1級1次
この動画を見る
$\displaystyle \int_{0}^{e-1} \displaystyle \frac{x}{(x+1)^2} dx$
出典:数検準1級1次
#数学検定準1級2次過去問#69「展開が最短かも」 #定積分

単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} x^4(1-x)^4$ $dx$
出典:数検準1級1次
この動画を見る
$\displaystyle \int_{0}^{1} x^4(1-x)^4$ $dx$
出典:数検準1級1次
#数検準1級1次#定積分#ますただ
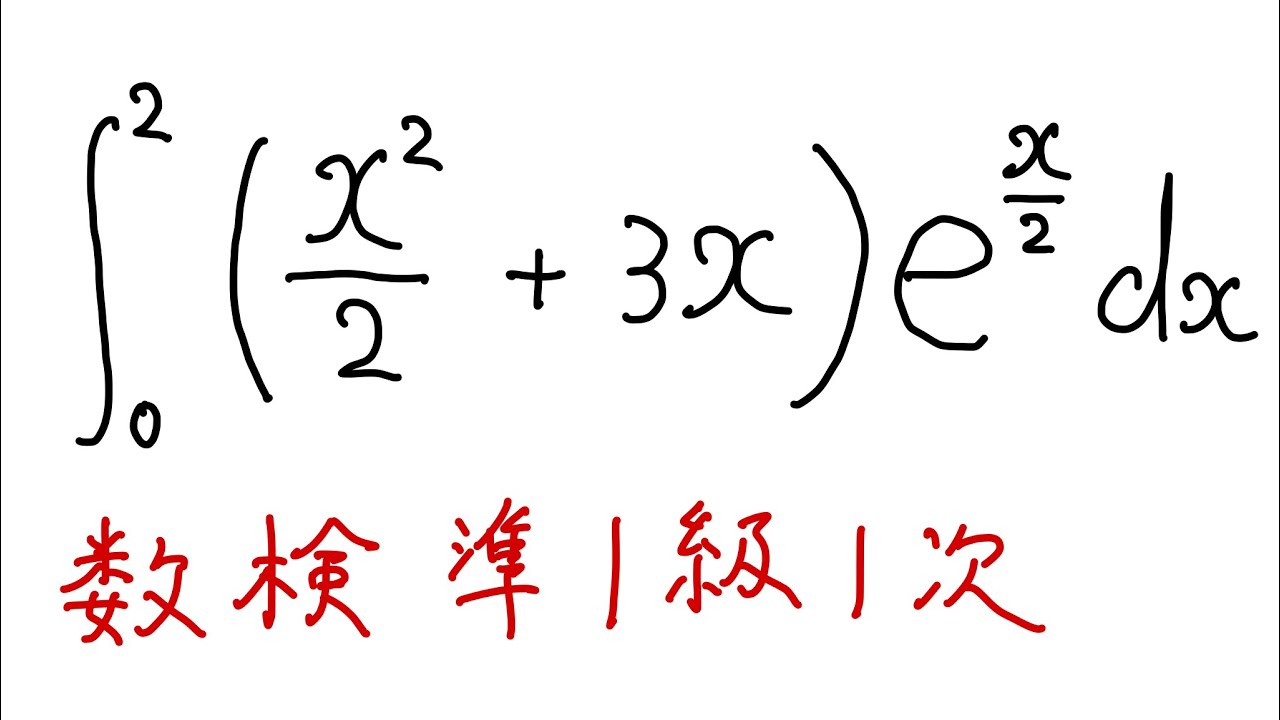
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{2} (\displaystyle \frac{x^2}{2}+3x)e^{\frac{x}{2}}dx$
出典:
この動画を見る
$\displaystyle \int_{0}^{2} (\displaystyle \frac{x^2}{2}+3x)e^{\frac{x}{2}}dx$
出典:
#山梨大学2013#定積分#ますただ

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#山梨大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-10}^{0} \displaystyle \frac{1}{(x+11)(x+12)}$ $dx$
出典:2013年山梨大学
この動画を見る
$\displaystyle \int_{-10}^{0} \displaystyle \frac{1}{(x+11)(x+12)}$ $dx$
出典:2013年山梨大学
#数検準1級1次過去問#定積分

単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{e^2-1} log(x+1)$ $dx$
出典:数検準1級1次
この動画を見る
$\displaystyle \int_{0}^{e^2-1} log(x+1)$ $dx$
出典:数検準1級1次
#福岡大学#不定積分#ますただ
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#福岡大学
指導講師:
ますただ
問題文全文(内容文):
以下の不定積分を解け
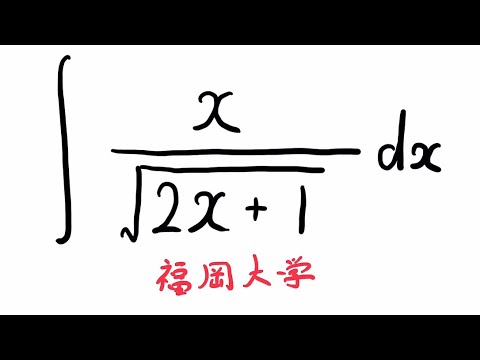
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+1 }}$ $dx$
出典:福岡大学
この動画を見る
以下の不定積分を解け
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+1 }}$ $dx$
出典:福岡大学
大学入試問題#884「ミスれん」 #東京理科大学(2022) #定積分
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#東京理科大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
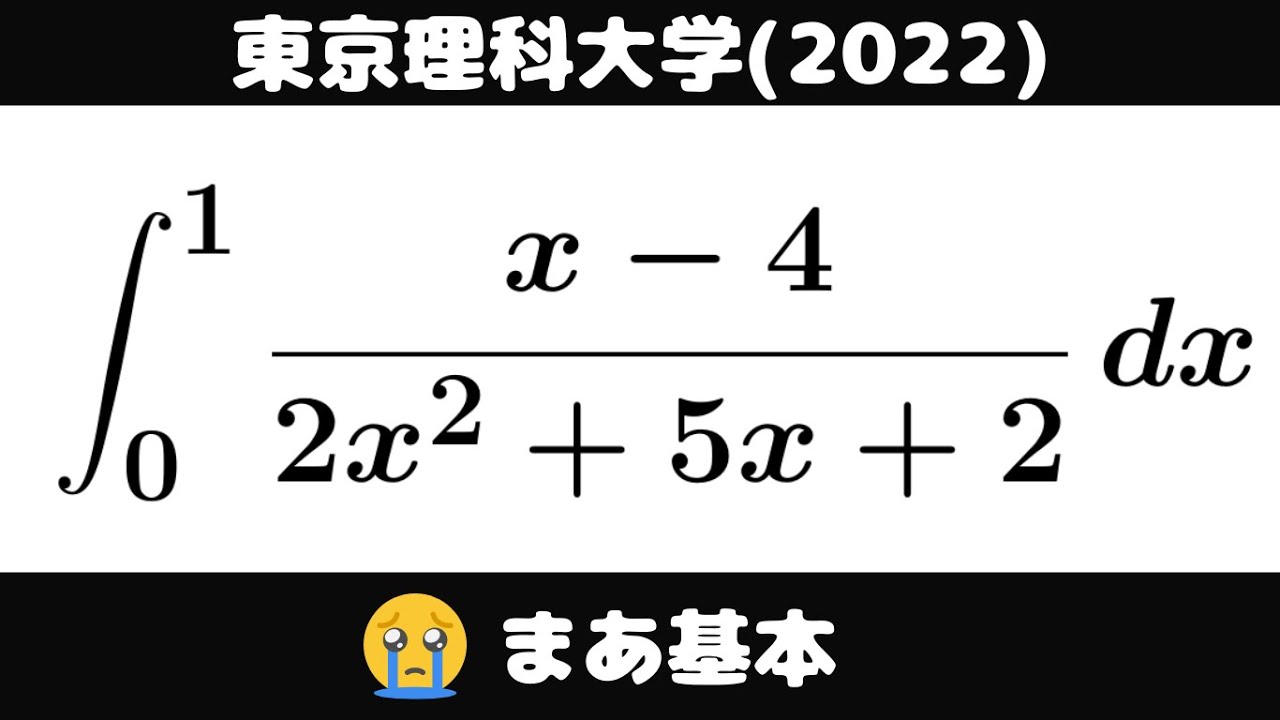
$\displaystyle \int_{0}^{1} \displaystyle \frac{x-4}{2x^2+5x+2}$ $dx$
出典:2022年東京理科大学
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{x-4}{2x^2+5x+2}$ $dx$
出典:2022年東京理科大学
#小樽商科大学#不定積分#ますただ

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#小樽商科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+2}-\sqrt{ 2 }}$ $dx$
出典:小樽商科大学
この動画を見る
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+2}-\sqrt{ 2 }}$ $dx$
出典:小樽商科大学
福田の数学〜慶應義塾大学2024年医学部第4問〜空間に浮かぶ四面体の平面による切り口の面積

単元:
#大学入試過去問(数学)#空間ベクトル#空間ベクトル#積分とその応用#面積・体積・長さ・速度#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)#数C#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
$\Large\boxed{4}$ 座標空間の4点O(0,0,0),A(-3,-1,1),B(2,-2,2),C(3,3,3)を頂点とする四面体OABCの、平面$z$=$t$による切り口を$S_t$とする。
(1)$S_t$は1<$t$<2のとき四角形となり、$t$=1および$t$=2のとき三角形となる。
1<$t$1 となるので、点Eはこの六面体の外にある。
(さ),(し),(す)の選択肢:ABC,ABD,ACD,BCD,OAD,OBD,OCD
(4)1<$t$<2に対して、(3)の六面体を平面$z$=$t$で切った切り口の面積を$U(t)$とすると、$U(t)$は$t$=$\boxed{\ \ (た)\ \ }$(ただし1<$\boxed{\ \ (た)\ \ }$<2)において最大値$\boxed{\ \ (ち)\ \ }$をとる。
この動画を見る
$\Large\boxed{4}$ 座標空間の4点O(0,0,0),A(-3,-1,1),B(2,-2,2),C(3,3,3)を頂点とする四面体OABCの、平面$z$=$t$による切り口を$S_t$とする。
(1)$S_t$は1<$t$<2のとき四角形となり、$t$=1および$t$=2のとき三角形となる。
1<$t$1 となるので、点Eはこの六面体の外にある。
(さ),(し),(す)の選択肢:ABC,ABD,ACD,BCD,OAD,OBD,OCD
(4)1<$t$<2に対して、(3)の六面体を平面$z$=$t$で切った切り口の面積を$U(t)$とすると、$U(t)$は$t$=$\boxed{\ \ (た)\ \ }$(ただし1<$\boxed{\ \ (た)\ \ }$<2)において最大値$\boxed{\ \ (ち)\ \ }$をとる。
大学入試問題#857「スッキリとした解答になるはず」 #大阪市立大学(1998) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#大阪市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{2} \displaystyle \frac{1}{\mathit{u}^{(\frac{3}{2})}}\{\sin(log\ \mathit{u})+\displaystyle \frac{1}{2}\cos(log\ \mathit{u})\}du$
出典:1998年大阪市立大学
この動画を見る
$\displaystyle \int_{1}^{2} \displaystyle \frac{1}{\mathit{u}^{(\frac{3}{2})}}\{\sin(log\ \mathit{u})+\displaystyle \frac{1}{2}\cos(log\ \mathit{u})\}du$
出典:1998年大阪市立大学
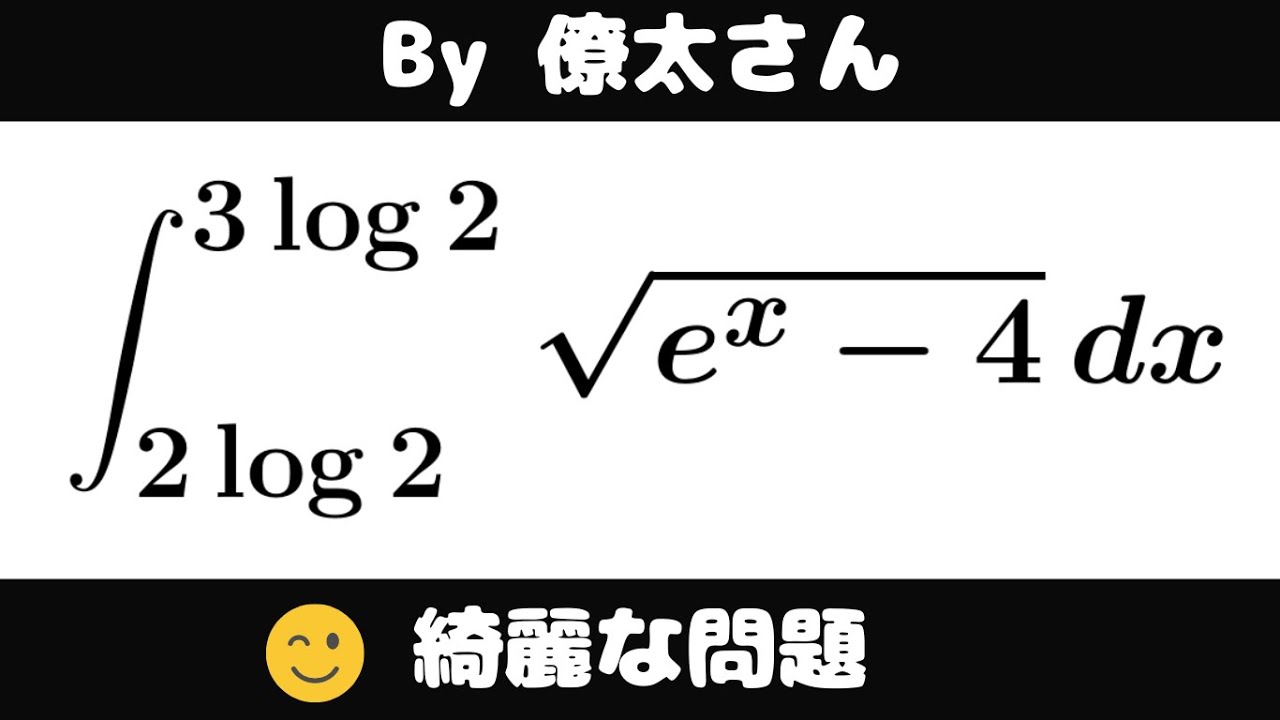
視聴者の僚太さんの積分「編集に5時間・・・・」
単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{2\ log\ 2}^{3\ log\ 2} \sqrt{ e^x-4 } \ dx$
この動画を見る
$\displaystyle \int_{2\ log\ 2}^{3\ log\ 2} \sqrt{ e^x-4 } \ dx$
福田の数学〜九州大学2024年理系第5問〜定積分で定義された数列の極限

単元:
#関数と極限#微分とその応用#積分とその応用#数列の極限#微分法#定積分#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
$\Large\boxed{5}$ 自然数$m$, $n$に対して
$I(m,n)$=$\displaystyle\int_1^ex^me^x(\log x)^ndx$
とする。以下の問いに答えよ。
(1)$I(m+1,n+1)$を$I(m,n+1)$, $I(m,n)$, $m$, $n$を用いて表せ。
(2)すべての自然数$m$に対して、$\displaystyle\lim_{n \to \infty}I(m,n)$=0 が成り立つことを示せ。
この動画を見る
$\Large\boxed{5}$ 自然数$m$, $n$に対して
$I(m,n)$=$\displaystyle\int_1^ex^me^x(\log x)^ndx$
とする。以下の問いに答えよ。
(1)$I(m+1,n+1)$を$I(m,n+1)$, $I(m,n)$, $m$, $n$を用いて表せ。
(2)すべての自然数$m$に対して、$\displaystyle\lim_{n \to \infty}I(m,n)$=0 が成り立つことを示せ。
大学入試問題#847「もうネタ切れ寸前」 #青山学院大学(2006) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数Ⅲ#青山学院大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} \{log(4-x^2)+2\} dx$
出典:2006年青山学院大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1} \{log(4-x^2)+2\} dx$
出典:2006年青山学院大学 入試問題
福田の数学〜神戸大学2024年理系第5問〜定積分で表された関数と不等式

単元:
#積分とその応用#定積分#神戸大学#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
$\Large\boxed{5}$ 0以上の実数$x$に対して、
$f(x)$=$\displaystyle\frac{1}{2}\int_{-x}^x\frac{1}{1+u^2}du$
と定める。以下の問いに答えよ。
(1)0≦$\alpha$<$\displaystyle\frac{\pi}{2}$ を満たす実数$\alpha$に対して、$f(\tan\alpha)$を求めよ。
(2)$xy$平面上で、次の連立不等式の表す領域を図示せよ。
0≦$x$≦1, 0≦$y$≦1, $f(x)$+$f(y)$≦$f(1)$
またその領域の面積を求めよ。
この動画を見る
$\Large\boxed{5}$ 0以上の実数$x$に対して、
$f(x)$=$\displaystyle\frac{1}{2}\int_{-x}^x\frac{1}{1+u^2}du$
と定める。以下の問いに答えよ。
(1)0≦$\alpha$<$\displaystyle\frac{\pi}{2}$ を満たす実数$\alpha$に対して、$f(\tan\alpha)$を求めよ。
(2)$xy$平面上で、次の連立不等式の表す領域を図示せよ。
0≦$x$≦1, 0≦$y$≦1, $f(x)$+$f(y)$≦$f(1)$
またその領域の面積を求めよ。
#広島市立大学(2013)

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \sin^9x \cos^3x\ dx$
出典:2013年広島市立大学
この動画を見る
$\displaystyle \int \sin^9x \cos^3x\ dx$
出典:2013年広島市立大学
大学入試問題#845「気持ち応用か!?」 #電気通信大学(2020) #区分求積法

単元:
#大学入試過去問(数学)#積分とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=n+1}^{2n} \displaystyle \frac{n}{k^2+3kn+2n^2}$
出典:2020年電気通信大学
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=n+1}^{2n} \displaystyle \frac{n}{k^2+3kn+2n^2}$
出典:2020年電気通信大学
福田の数学〜神戸大学2024年理系第4問〜回転体の体積

単元:
#積分とその応用#面積・体積・長さ・速度#神戸大学#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
$\Large\boxed{4}$ 1辺の長さが$\sqrt 2$の正方形ABCDを底面にもち、高さが1である直方体ABCD-EFGHを、頂点の座標がそれぞれ
A(1,0,0), B(0,1,0), C(-1,0,0), D(0,-1,0),
E(1,0,1), F(0,1,1), G(-1,0,1), H(0,-1,1)
になるように$xyz$空間におく。以下の問いに答えよ。
(1)直方体ABCD-EFGHを直線AEのまわりに1回転してできる回転体を$X_1$とし、また直線ABのまわりに1回転してできる回転体を$X_2$とする。$X_1$の体積$V_1$と$X_2$の体積$V_2$を求めよ。
(2)0≦$t$≦1 とする。平面$x$=$t$と線分EFの共有点の座標を求めよ。
(3)直方体ABCD-EFGHを$x$軸のまわりに1回転してできる回転体を$X_3$とする。
$X_3$の体積$V_3$を求めよ。
この動画を見る
$\Large\boxed{4}$ 1辺の長さが$\sqrt 2$の正方形ABCDを底面にもち、高さが1である直方体ABCD-EFGHを、頂点の座標がそれぞれ
A(1,0,0), B(0,1,0), C(-1,0,0), D(0,-1,0),
E(1,0,1), F(0,1,1), G(-1,0,1), H(0,-1,1)
になるように$xyz$空間におく。以下の問いに答えよ。
(1)直方体ABCD-EFGHを直線AEのまわりに1回転してできる回転体を$X_1$とし、また直線ABのまわりに1回転してできる回転体を$X_2$とする。$X_1$の体積$V_1$と$X_2$の体積$V_2$を求めよ。
(2)0≦$t$≦1 とする。平面$x$=$t$と線分EFの共有点の座標を求めよ。
(3)直方体ABCD-EFGHを$x$軸のまわりに1回転してできる回転体を$X_3$とする。
$X_3$の体積$V_3$を求めよ。
大学入試問題#844「まあ基本・・・」 #電気通信大学(2015) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}} (\sin x)(\sin 2x)(\sin 3x) dx$
出典:2015年電気通信大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} (\sin x)(\sin 2x)(\sin 3x) dx$
出典:2015年電気通信大学 入試問題
#大阪医科大学(2014) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#大阪医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-1}^{1} x \sin n \pi \ x\ dx$
$n$:自然数
出典:2014年大阪医科大学
この動画を見る
$\displaystyle \int_{-1}^{1} x \sin n \pi \ x\ dx$
$n$:自然数
出典:2014年大阪医科大学
大学入試問題#843「解き方色々ありそう」 #筑波大学(2013) #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{1}{\sin x \cos x} dx$
出典:2013年筑波大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{1}{\sin x \cos x} dx$
出典:2013年筑波大学 入試問題
#宮崎大学(2016)

単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{e} \displaystyle \frac{\sqrt{ 1+log\ x }}{x} dx$
出典:2016年宮崎大学
この動画を見る
$\displaystyle \int_{1}^{e} \displaystyle \frac{\sqrt{ 1+log\ x }}{x} dx$
出典:2016年宮崎大学
#福島大学(2021) #定積分 #Shorts
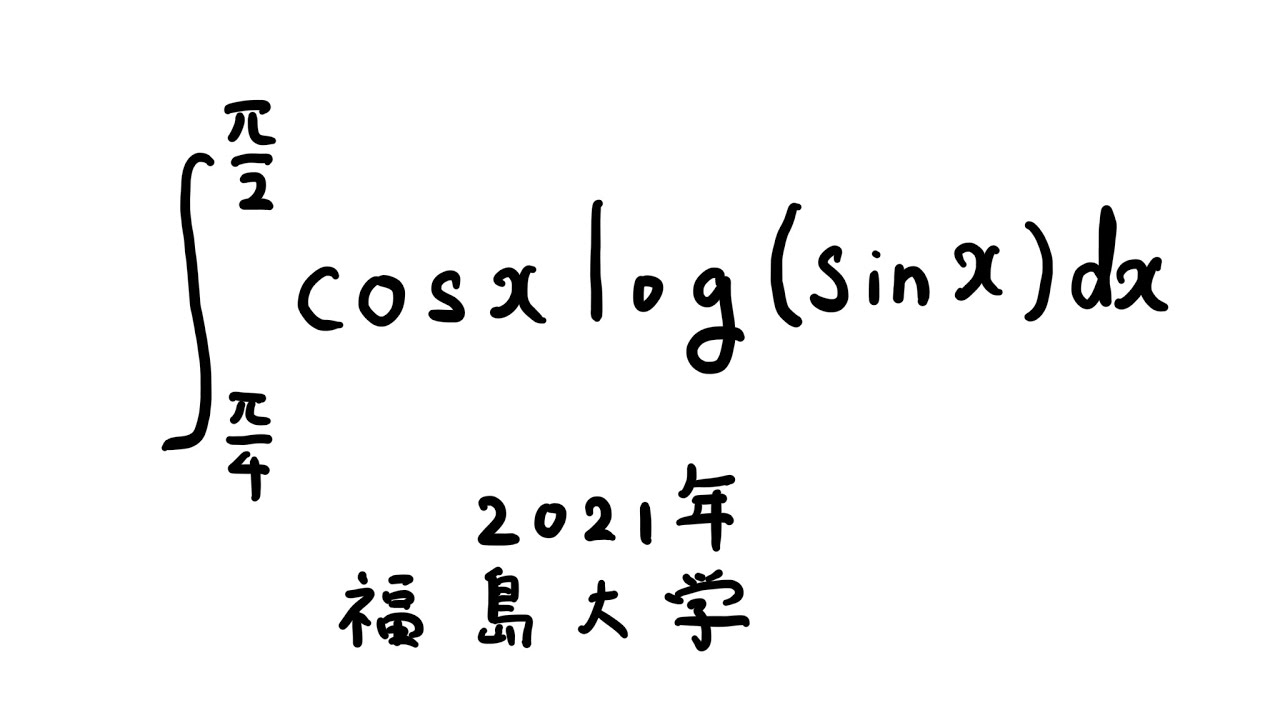
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#福島大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{4}}^{\frac{\pi}{2}} \cos\ x\ log(\sin\ x) dx$
出典:2021年福島大学
この動画を見る
$\displaystyle \int_{\frac{\pi}{4}}^{\frac{\pi}{2}} \cos\ x\ log(\sin\ x) dx$
出典:2021年福島大学
#筑波大学(2016) #定積分 #Shorts

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#筑波大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{1}{2}}^{2} |log\ x| dx$
出典:2016年筑波大学
この動画を見る
$\displaystyle \int_{\frac{1}{2}}^{2} |log\ x| dx$
出典:2016年筑波大学
大学入試問題#825「まあまあ良問」 #茨城大学(2022) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-7}^{1}(2-x) \sqrt[ 3 ]{ 1-x }\ dx$
出典:2022年茨城大学
この動画を見る
$\displaystyle \int_{-7}^{1}(2-x) \sqrt[ 3 ]{ 1-x }\ dx$
出典:2022年茨城大学
#秋田大学(2019) #定積分 #Shorts
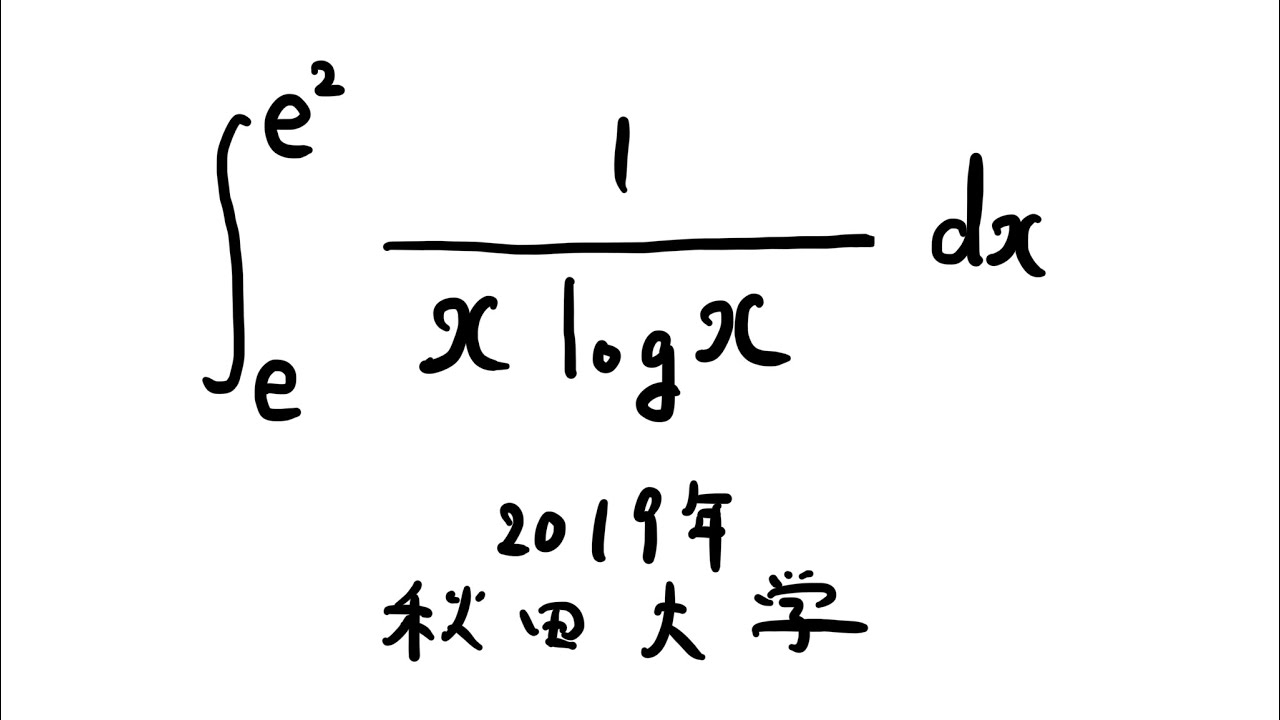
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#秋田大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{e}^{e^2} \displaystyle \frac{1}{x\ log\ x} dx$
出典:2019年秋田大学
この動画を見る
$\displaystyle \int_{e}^{e^2} \displaystyle \frac{1}{x\ log\ x} dx$
出典:2019年秋田大学
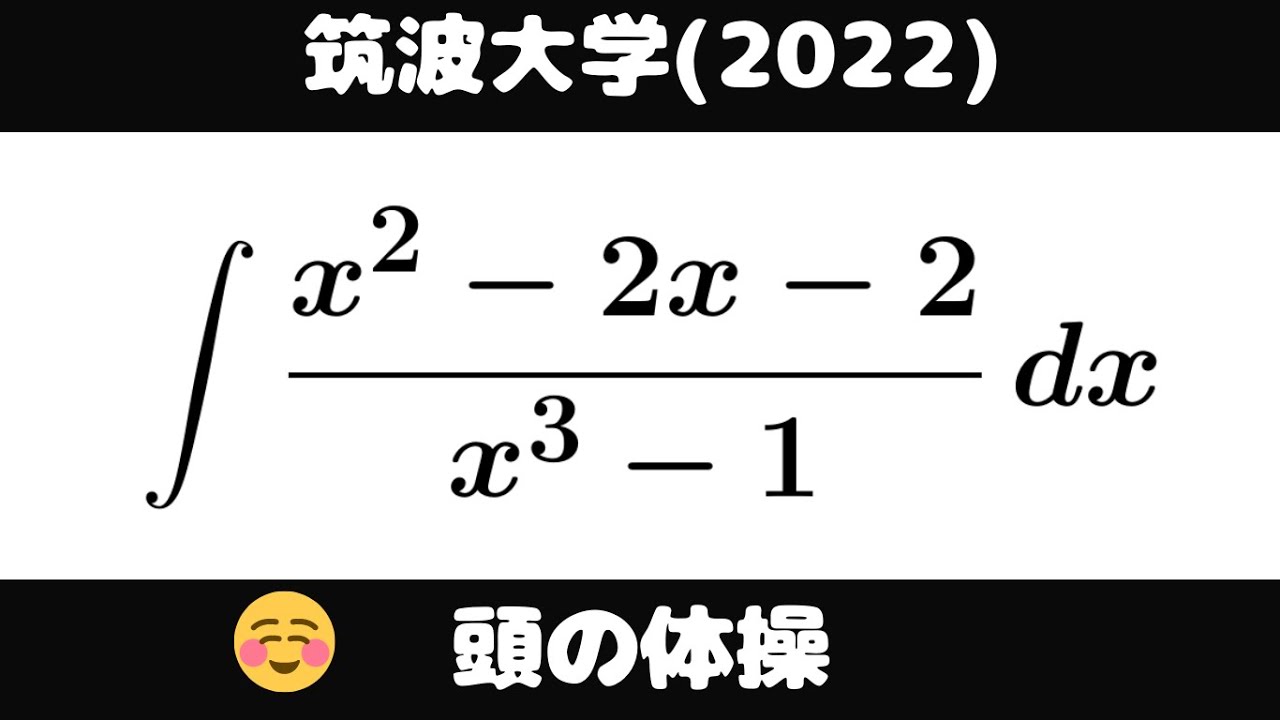
大学入試問題#824「たぶん良問」 #筑波大学(2022) #定積分
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#筑波大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{x^2-2x-2}{x^3-1} dx$
出典:2022年筑波大学
この動画を見る
$\displaystyle \int \displaystyle \frac{x^2-2x-2}{x^3-1} dx$
出典:2022年筑波大学
#茨城大学(2023) #定積分 #Shorts
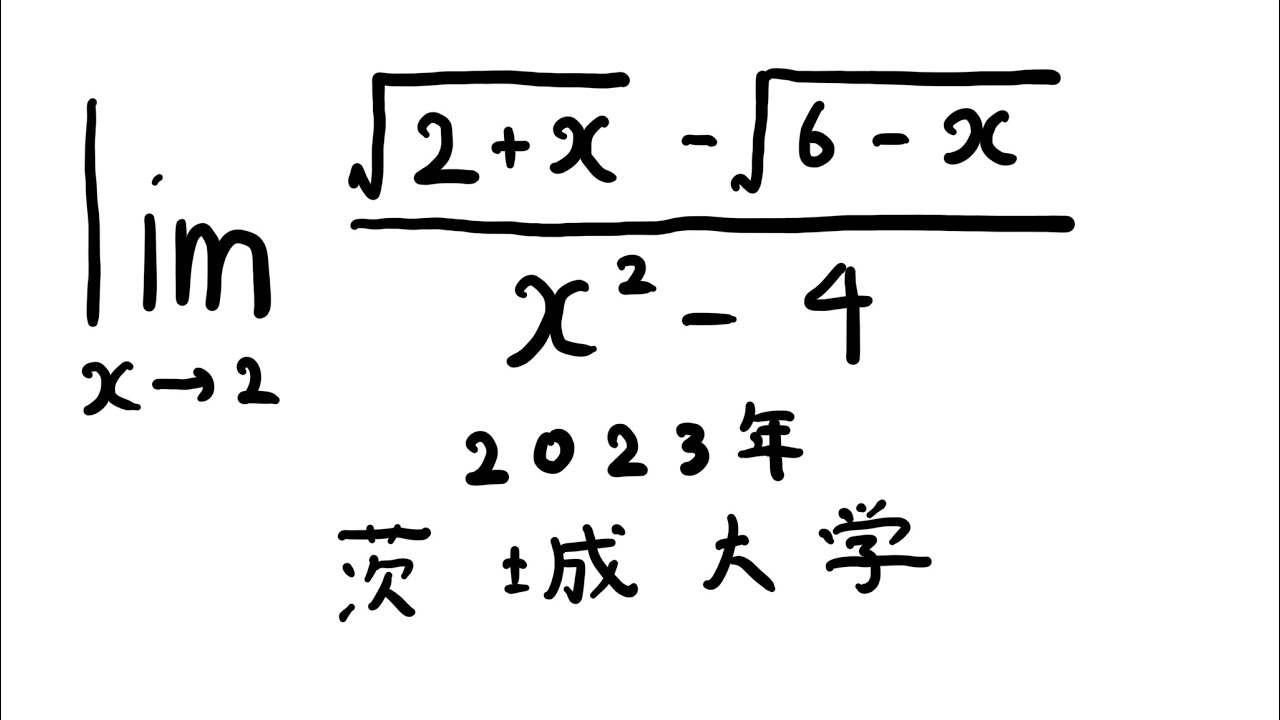
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ x \to 2 } \displaystyle \frac{\sqrt{ 2+x }-\sqrt{ 6-x }}{x^2-4}$
出典:2023年茨城大学
この動画を見る
$\displaystyle \lim_{ x \to 2 } \displaystyle \frac{\sqrt{ 2+x }-\sqrt{ 6-x }}{x^2-4}$
出典:2023年茨城大学
#茨城大学(2022) #定積分 #Shorts
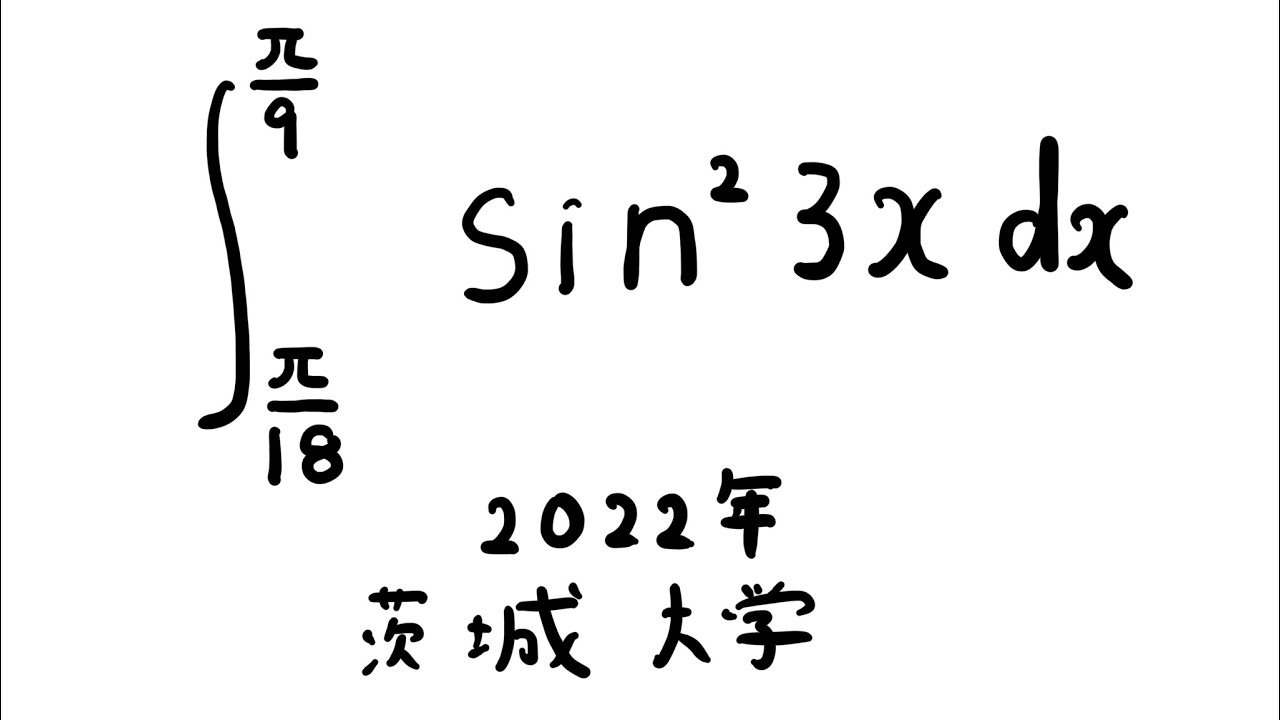
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{18}}^{\frac{\pi}{9}} \sin^23x\ dx$
出典:2022年茨城大学
この動画を見る
$\displaystyle \int_{\frac{\pi}{18}}^{\frac{\pi}{9}} \sin^23x\ dx$
出典:2022年茨城大学
大学入試問題#823「置換するかどうか」 #筑波大学(2019) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#筑波大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} (x+1)^2e-(x+1) dx$
出典:2019年筑波大学
この動画を見る
$\displaystyle \int_{0}^{1} (x+1)^2e-(x+1) dx$
出典:2019年筑波大学
