ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！
職業：数学者
職歴：高校非常勤（院生時代）、高専、大学 准教授
趣味：
①将棋 棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀 アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス 中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。
大学入試問題#516「ちょっとした公式で一撃！」 高知工科大学(2022) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#高知工科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{\frac{\pi}{6}}^{\frac{\pi}{4}} \displaystyle \frac{dx}{\tan^2x\ \cos^2x}$
出典：2022年高知工科大学 入試問題
この動画を見る
$\displaystyle \int_{\frac{\pi}{6}}^{\frac{\pi}{4}} \displaystyle \frac{dx}{\tan^2x\ \cos^2x}$
出典：2022年高知工科大学 入試問題
大学入試問題#515「この問題は結構有名？」 名古屋大学(2005) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#名古屋大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\pi} \displaystyle \frac{x\ \sin^3x}{4-\cos^2x} dx$
出典：2005年名古屋大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\pi} \displaystyle \frac{x\ \sin^3x}{4-\cos^2x} dx$
出典：2005年名古屋大学 入試問題
大学入試問題#514「困ったらz=x+yi？」 札幌医科大学(2022) #複素数
単元：
#数Ⅱ#大学入試過去問(数学)#複素数と方程式#学校別大学入試過去問解説(数学)#数学(高校生)#札幌医科大学
指導講師：
ますただ
問題文全文(内容文)：
$|z+3i|=2|z|$
$|z+4i|=|z|$
を満たす複素数$z$をすべて求めよ
出典：2022年札幌医科大学 入試問題
この動画を見る
$|z+3i|=2|z|$
$|z+4i|=|z|$
を満たす複素数$z$をすべて求めよ
出典：2022年札幌医科大学 入試問題
大学入試問題#513「このチャンネルでは初めての発想！！」 By Nissydarts さん #定積分
単元：
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$I=\displaystyle \int_{0}^{\frac{\pi}{6}} \displaystyle \frac{dx}{1-6\sin^2x+12\sin^4x-8\sin^6x}$
この動画を見る
$I=\displaystyle \int_{0}^{\frac{\pi}{6}} \displaystyle \frac{dx}{1-6\sin^2x+12\sin^4x-8\sin^6x}$
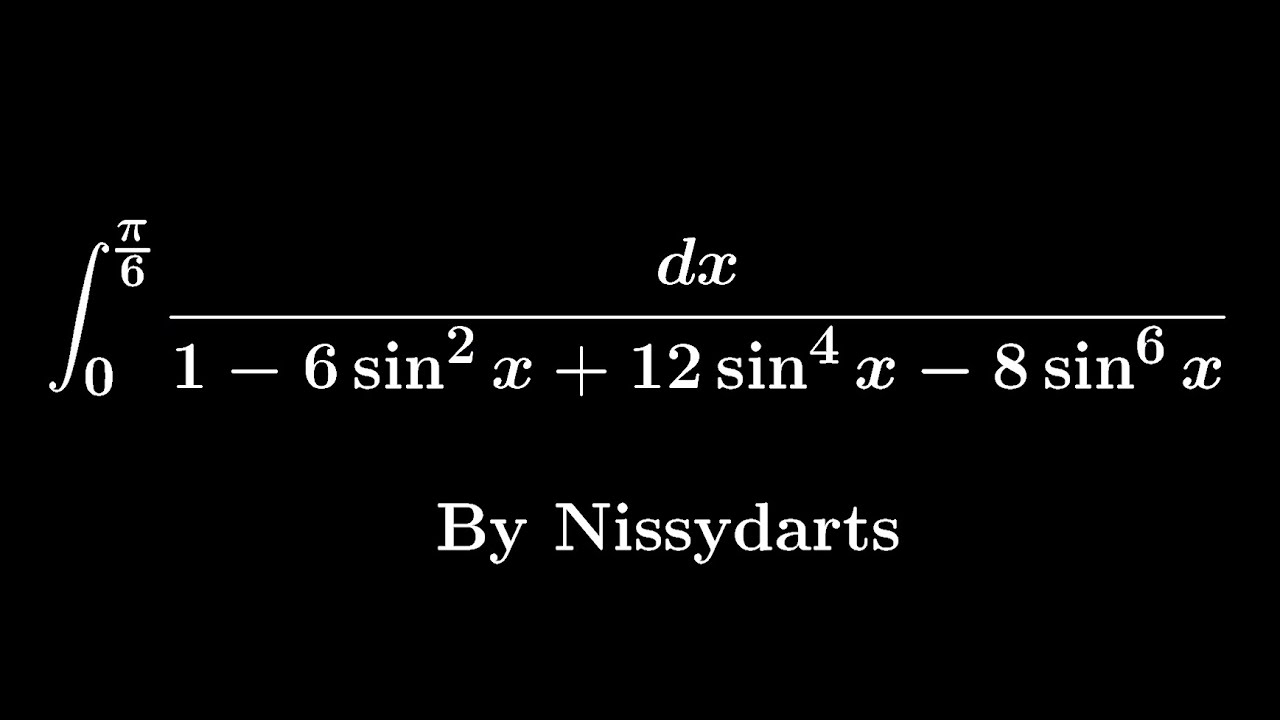
大学入試問題#512「受験生の心は折れる」 浜松医科大学(2015) #区分求積法
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#浜松医科大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty } (\displaystyle \frac{(3n^2+1^2)(3n^2+2^2)・・・(3n^2+n^2)}{(n^2+1^2)(n^2+2^2)・・・(n^2+n^2)})^{\frac{1}{n}}$
出典：2015年浜松医科大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty } (\displaystyle \frac{(3n^2+1^2)(3n^2+2^2)・・・(3n^2+n^2)}{(n^2+1^2)(n^2+2^2)・・・(n^2+n^2)})^{\frac{1}{n}}$
出典：2015年浜松医科大学 入試問題
大学入試問題#511「数検1級の1次に類題が出てるはず」 浜松医科大学(2015) #区分求積法
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#浜松医科大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty } (\displaystyle \frac{(3n+1)(3n+2)・・・(3n+n)}{(n+1)(n+2)・・・(n+n)})^{\frac{1}{n}}$
出典：2015年浜松医科大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty } (\displaystyle \frac{(3n+1)(3n+2)・・・(3n+n)}{(n+1)(n+2)・・・(n+n)})^{\frac{1}{n}}$
出典：2015年浜松医科大学 入試問題
大学入試問題#510「よくある形」 #防衛医科大学(2015) #数列
単元：
#大学入試過去問(数学)#数列#学校別大学入試過去問解説(数学)#数学(高校生)#数B#防衛医科大学
指導講師：
ますただ
問題文全文(内容文)：
$a_1=1,\ a_2=e$
$a_{n+2}=a_n^{-2}・a_{n+1}^3$
一般項$a_n$を求めよ
出典：2015年防衛医科大学 入試問題
この動画を見る
$a_1=1,\ a_2=e$
$a_{n+2}=a_n^{-2}・a_{n+1}^3$
一般項$a_n$を求めよ
出典：2015年防衛医科大学 入試問題
大学入試問題#509「あえて三角関数」 自治医科大学(2023) #曲線
単元：
#数Ⅱ#大学入試過去問(数学)#接線と増減表・最大値・最小値#学校別大学入試過去問解説(数学)#数学(高校生)#自治医科大学
指導講師：
ますただ
問題文全文(内容文)：
$x,\ 0 \leqq y$：実数
$\displaystyle \frac{x^2}{4}+\displaystyle \frac{y^2}{9}=1$を満たすとき
$5x+2y$の最大値を$M$、最小値を$m$とするとき$\sqrt{ M^2-m^2 }$を求めよ
出典：2023年自治医科大学 入試問題
この動画を見る
$x,\ 0 \leqq y$：実数
$\displaystyle \frac{x^2}{4}+\displaystyle \frac{y^2}{9}=1$を満たすとき
$5x+2y$の最大値を$M$、最小値を$m$とするとき$\sqrt{ M^2-m^2 }$を求めよ
出典：2023年自治医科大学 入試問題
大学入試問題#508「入試の1問目がこれは萎える」 防衛医科大学(2015) #整数問題
単元：
#数A#大学入試過去問(数学)#整数の性質#学校別大学入試過去問解説(数学)#数学(高校生)#防衛医科大学
指導講師：
ますただ
問題文全文(内容文)：
$a^5-12a^4+36a^3-81a+1,\ a^2-6a$が共に有理数となる無理数$a$を求めよ
出典：2015年防衛医科大学 入試問題
この動画を見る
$a^5-12a^4+36a^3-81a+1,\ a^2-6a$が共に有理数となる無理数$a$を求めよ
出典：2015年防衛医科大学 入試問題
大学入試問題#507「油断してると沼にはまりがち：良問」 長崎大学(2015) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#長崎大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{4}} \displaystyle \frac{\tan\ x}{2-\cos\ 2x} dx$
出典：2015年長崎大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}} \displaystyle \frac{\tan\ x}{2-\cos\ 2x} dx$
出典：2015年長崎大学 入試問題
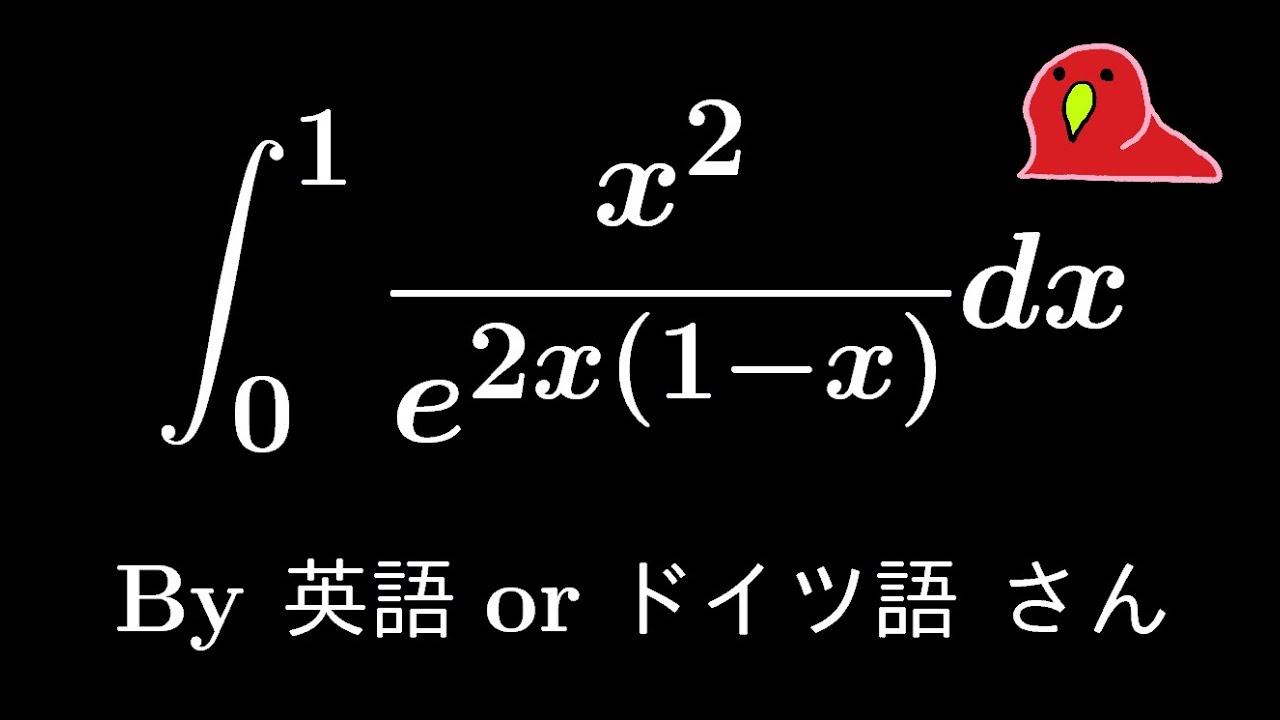
大学入試問題#506「どこから引っかけるか」By英語orドイツ語さん #定積分
単元：
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1} \displaystyle \frac{x^2}{e^{2x(1-x)}}dx$
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{x^2}{e^{2x(1-x)}}dx$
大学入試問題#505「綺麗な数列の問題」 #神戸大学 (2022) #数列 #極限
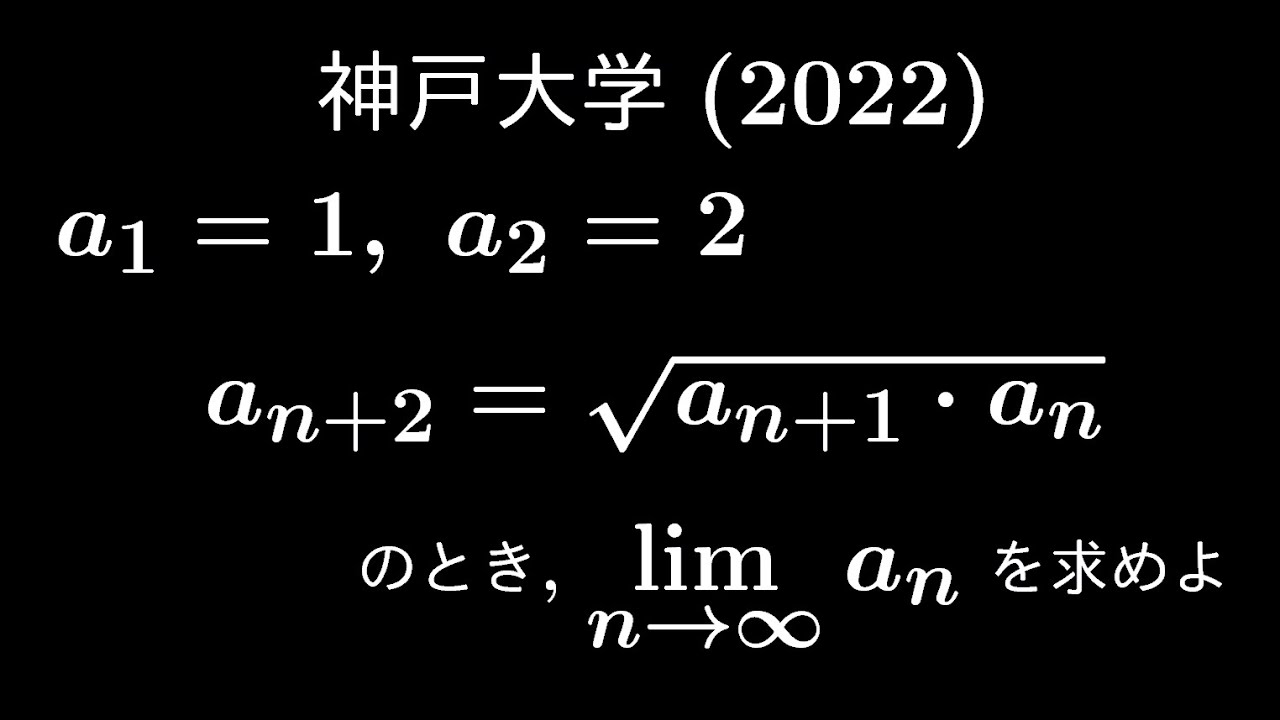
単元：
#大学入試過去問(数学)#関数と極限#数列の極限#学校別大学入試過去問解説(数学)#神戸大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$a_1=1,\ a_2=2$
$a_{n+2}=\sqrt{ a_{n+1}・a_n }$のとき
$\displaystyle \lim_{ n \to \infty } a_n$を求めよ
出典：2022年神戸大学 入試問題
この動画を見る
$a_1=1,\ a_2=2$
$a_{n+2}=\sqrt{ a_{n+1}・a_n }$のとき
$\displaystyle \lim_{ n \to \infty } a_n$を求めよ
出典：2022年神戸大学 入試問題
大学入試問題#504「ひたすら積分」 #京都工芸繊維大学 (2012) #定積分
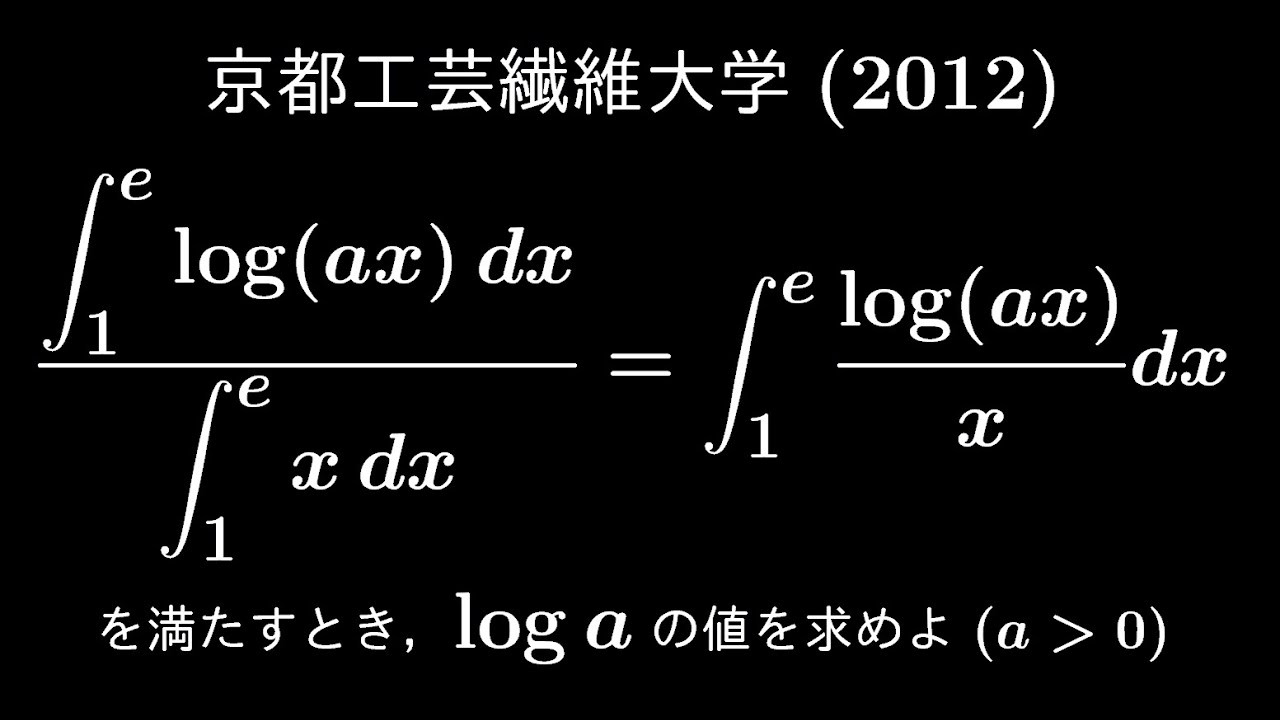
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$a \gt 0$
$\displaystyle \frac{\displaystyle \int_{1}^{e} log(ax) dx}{\displaystyle \int_{1}^{e} x\ dx}=\displaystyle \int_{1}^{e}\displaystyle \frac{ log(ax)}{x} dx$を満たすとき
$log\ a$の値を求めよ。
出典：2012年京都工芸繊維大学 入試問題
この動画を見る
$a \gt 0$
$\displaystyle \frac{\displaystyle \int_{1}^{e} log(ax) dx}{\displaystyle \int_{1}^{e} x\ dx}=\displaystyle \int_{1}^{e}\displaystyle \frac{ log(ax)}{x} dx$を満たすとき
$log\ a$の値を求めよ。
出典：2012年京都工芸繊維大学 入試問題
大学入試問題#503「微分してもよさげだけど・・・」 #藤田医科大学 (2023) #判別式
単元：
#大学入試過去問(数学)#微分とその応用#微分法#学校別大学入試過去問解説(数学)#数学(高校生)#藤田医科大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$f(x)=\displaystyle \frac{8x^2+5}{x^2-3x+6}$
の最大値を$M$、最小値を$m$とするとき$\displaystyle \frac{M}{m}$を求めよ
出典：2023年藤田医科大学 入試問題
この動画を見る
$f(x)=\displaystyle \frac{8x^2+5}{x^2-3x+6}$
の最大値を$M$、最小値を$m$とするとき$\displaystyle \frac{M}{m}$を求めよ
出典：2023年藤田医科大学 入試問題
大学入試問題#502「誘導ありで私立の医学部」 私立医学部医学科系 #定積分 by 英語orドイツ語さん
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-\pi-2}^{\pi(\pi+1)} \displaystyle \frac{dx}{x^2+\pi^2}$
この動画を見る
$\displaystyle \int_{-\pi-2}^{\pi(\pi+1)} \displaystyle \frac{dx}{x^2+\pi^2}$
大学入試問題#501「積分区間を2π→0にせんでも・・・・」 産業医科大学(2016) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{2\pi}^{0} |3\cos\ x-\sqrt{ 3 }\ \sin\ x|\ dx$
出典：2016年産業医科大学 入試問題
この動画を見る
$\displaystyle \int_{2\pi}^{0} |3\cos\ x-\sqrt{ 3 }\ \sin\ x|\ dx$
出典：2016年産業医科大学 入試問題
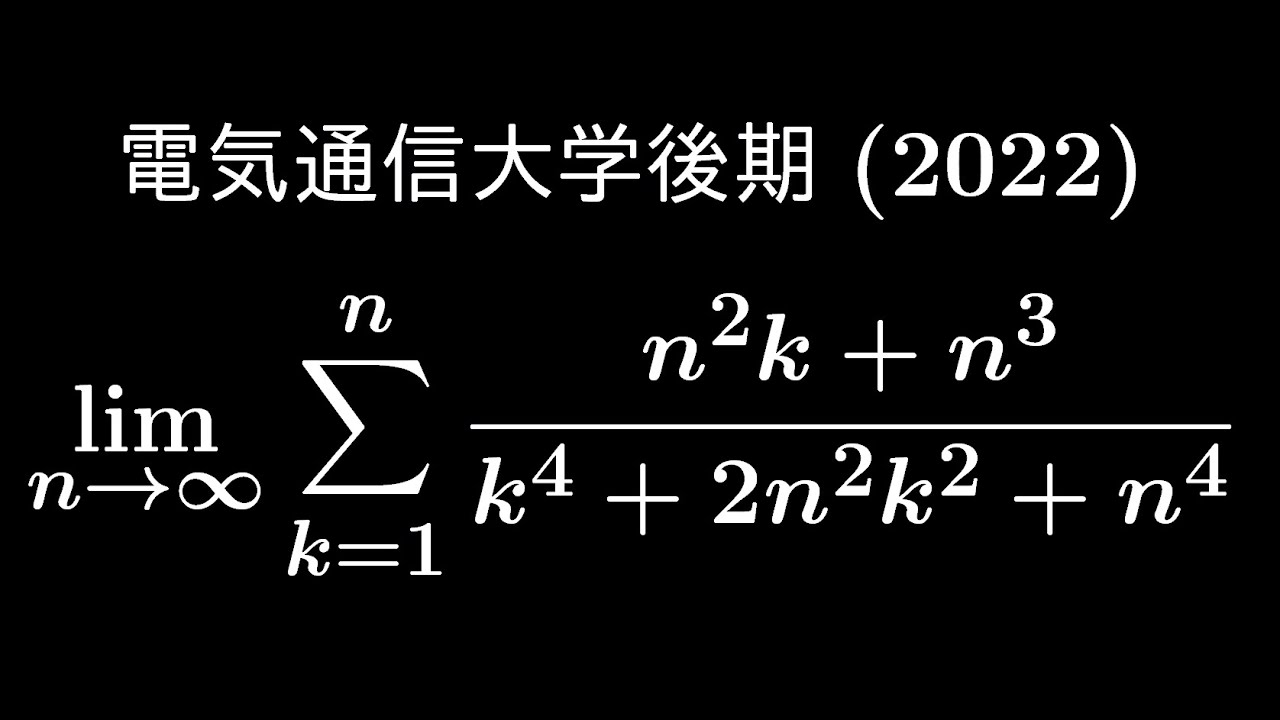
大学入試問題#500「基本に沿って」 電気通信大学後期(2022) #区分求積法
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=1}^n \displaystyle \frac{n^2k+n^3}{k^4+2n^2k^2+n^4}$
出典：2022年電気通信大学後期 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=1}^n \displaystyle \frac{n^2k+n^3}{k^4+2n^2k^2+n^4}$
出典：2022年電気通信大学後期 入試問題
大学入試問題#499「見た目以上に計算量が多い」 信州大学後期(2015) #広義積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ s \to +0 } \displaystyle \int_{s}^{\frac{\pi}{2}} (\displaystyle \frac{1}{\sin\ x}-\displaystyle \frac{1}{x}) dx$
出典：2015年信州大学後期 入試問題
この動画を見る
$\displaystyle \lim_{ s \to +0 } \displaystyle \int_{s}^{\frac{\pi}{2}} (\displaystyle \frac{1}{\sin\ x}-\displaystyle \frac{1}{x}) dx$
出典：2015年信州大学後期 入試問題
大学入試問題#498「類題はたくさん」 信州大学後期2011 #不定積分3
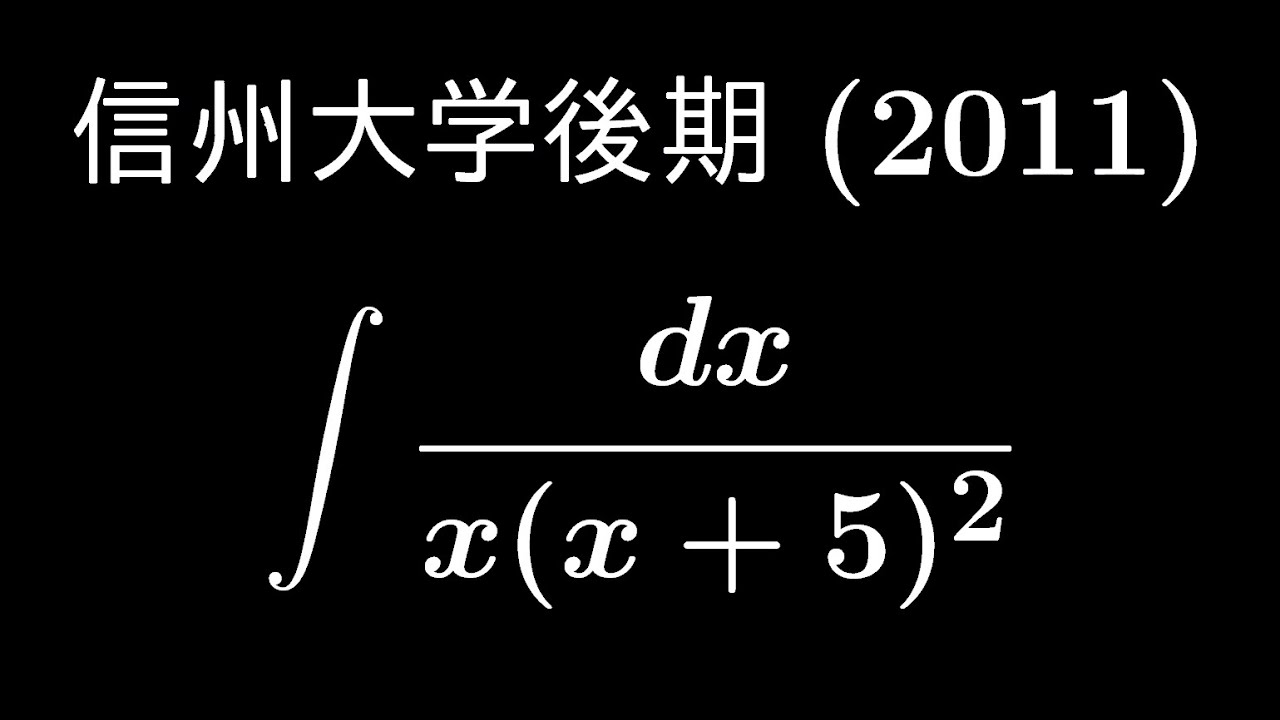
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{dx}{x(x+5)^2}$
出典：2011年信州大学後期 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{dx}{x(x+5)^2}$
出典：2011年信州大学後期 入試問題
大学入試問題#497「まあ、これがベターなのかな」 産業医科大学 改 (2016) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{\sqrt{ 2 }}^{\sqrt{ 3 }} x\ log(x^2-1)\ dx$
出典：2016年産業医科大学 入試問題
この動画を見る
$\displaystyle \int_{\sqrt{ 2 }}^{\sqrt{ 3 }} x\ log(x^2-1)\ dx$
出典：2016年産業医科大学 入試問題
大学入試問題#496「よくある問題」 産業医科大学 改 (2016) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{2}^{3} (x-1)(x-2)^{\frac{1}{3}} dx$
出典：2016年産業医科大学 入試問題
この動画を見る
$\displaystyle \int_{2}^{3} (x-1)(x-2)^{\frac{1}{3}} dx$
出典：2016年産業医科大学 入試問題
大学入試問題#495「知ってる形に」 産業医科大学(2016) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{0} \displaystyle \frac{x^2+x-1}{x^2+x+1} dx$
出典：2016年産業医科大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{0} \displaystyle \frac{x^2+x-1}{x^2+x+1} dx$
出典：2016年産業医科大学 入試問題
大学入試問題#494「基本問題」 信州大学後期(2011) #不定積分
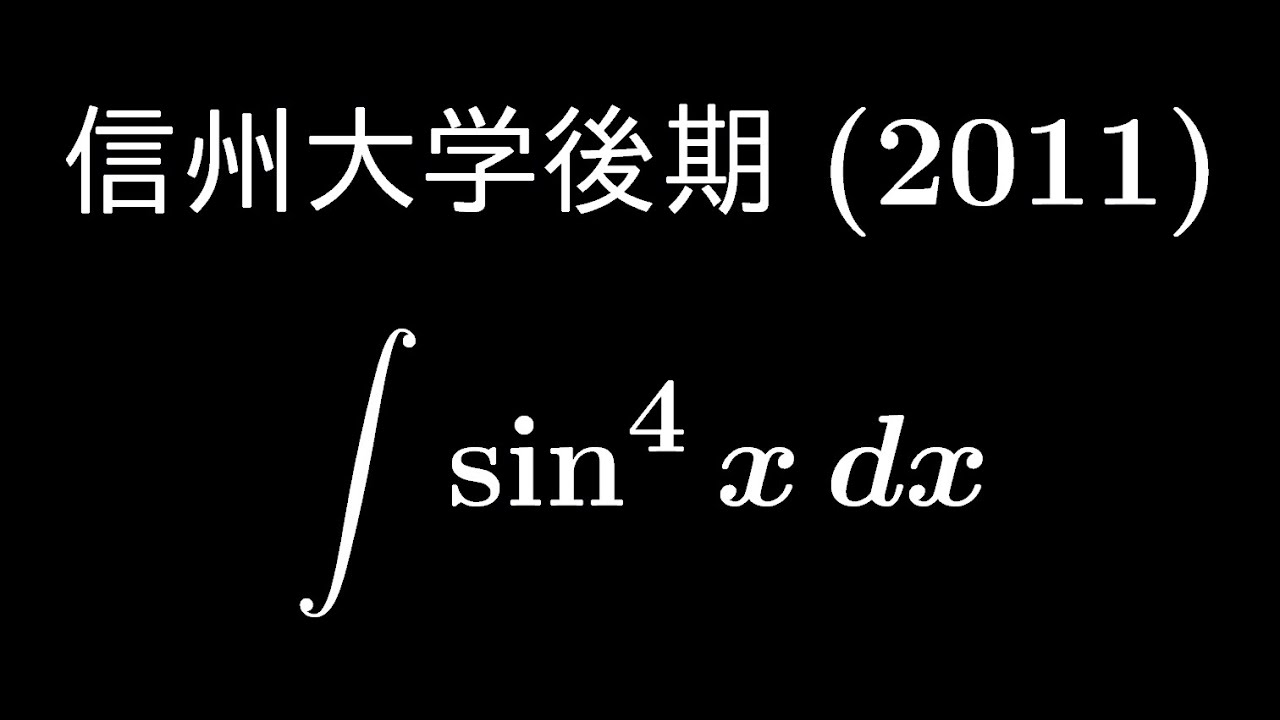
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \sin^4x\ dx$
出典：2011年信州大学後期 入試問題
この動画を見る
$\displaystyle \int \sin^4x\ dx$
出典：2011年信州大学後期 入試問題
大学入試問題#493「詰みまでの構想力が必要」 東京理科大学(2001) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int (t\sqrt{ 1+t^2 }+\displaystyle \frac{t^3}{\sqrt{ 1+t^2 }})dt$
出典：2001年東京理科大学 入試問題
この動画を見る
$\displaystyle \int (t\sqrt{ 1+t^2 }+\displaystyle \frac{t^3}{\sqrt{ 1+t^2 }})dt$
出典：2001年東京理科大学 入試問題
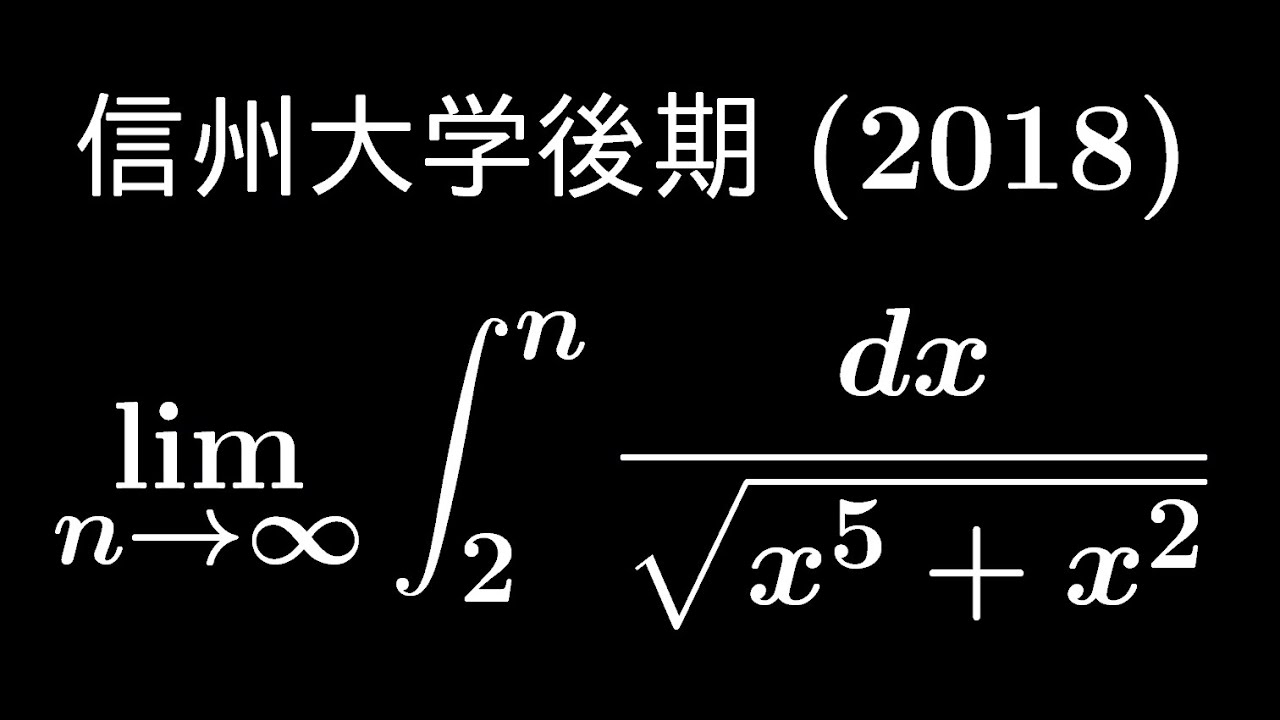
大学入試問題#492「置換方法はいろいろ」 信州大学後期(2018) #広義積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty } \displaystyle \int_{2}^{n} \displaystyle \frac{dx}{\sqrt{ x^5+x^2 }}$
出典：2018年信州大学後期 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \int_{2}^{n} \displaystyle \frac{dx}{\sqrt{ x^5+x^2 }}$
出典：2018年信州大学後期 入試問題
大学入試問題#491「綺麗な問題」 立教大学 類題 By 英語orドイツ語シはBかHか さん #不定積分
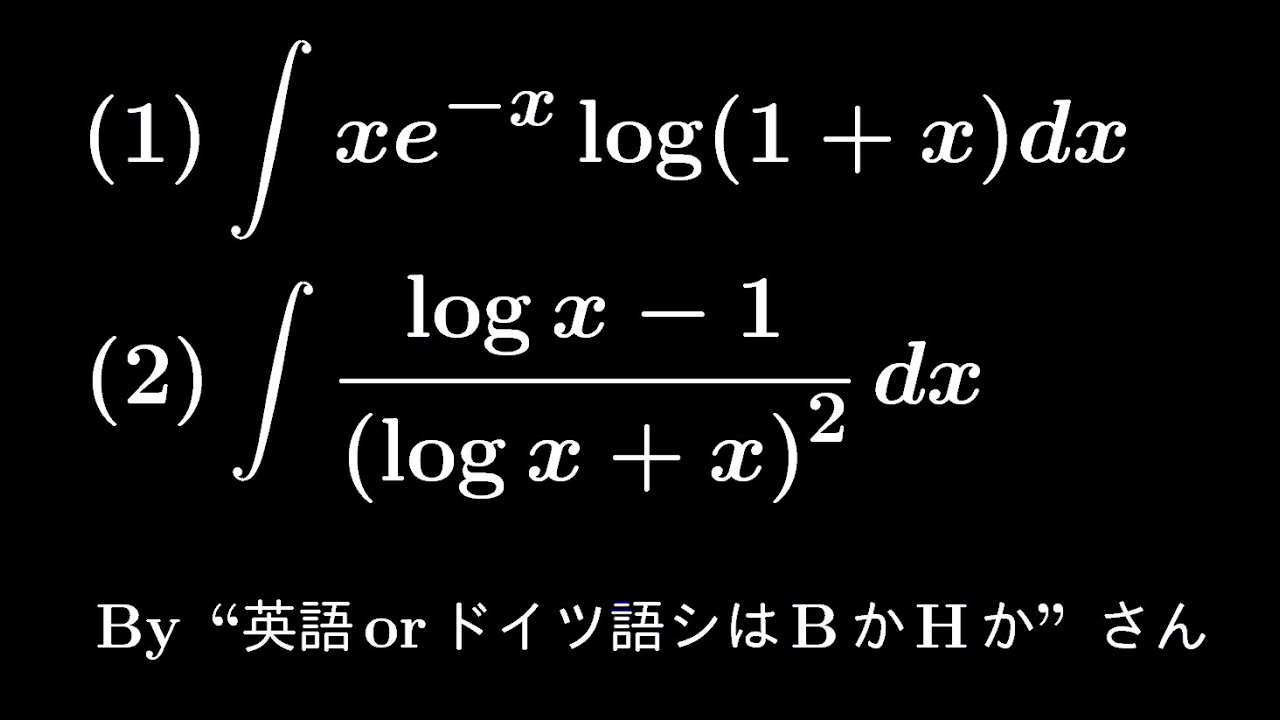
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#立教大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
（1）
$\displaystyle \int xe^{-x}log(x+1)dx$
（2）
$\displaystyle \int \displaystyle \frac{log\ x-1}{(log\ x+x)^2}dx$
出典：立教大学 入試問題
この動画を見る
（1）
$\displaystyle \int xe^{-x}log(x+1)dx$
（2）
$\displaystyle \int \displaystyle \frac{log\ x-1}{(log\ x+x)^2}dx$
出典：立教大学 入試問題
大学入試問題#490「よくみる形」 信州大学後期(2015) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ#大阪市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int x(log\ x)^2 dx$
出典：2015年信州大学後期 入試問題
この動画を見る
$\displaystyle \int x(log\ x)^2 dx$
出典：2015年信州大学後期 入試問題
【0≦θ≦πを問題文に追加】微分すると大変かも・・・ By ～らん～
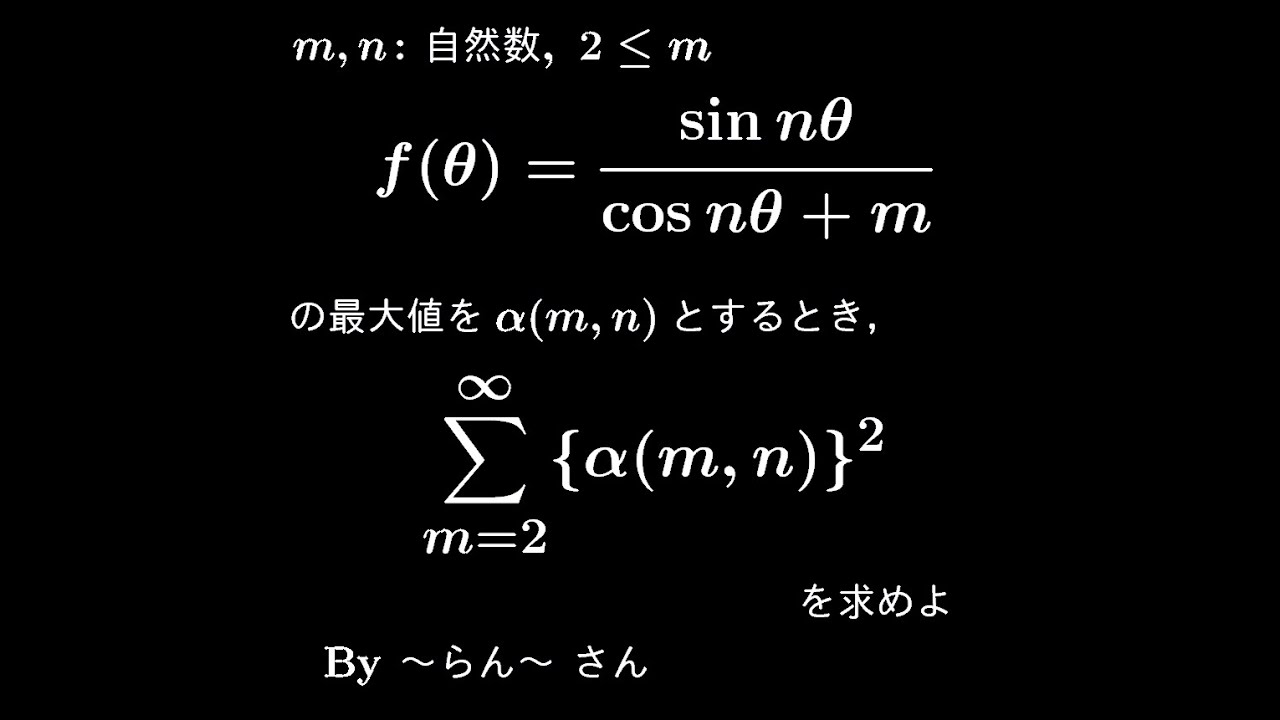
単元：
#数Ⅱ#三角関数#三角関数とグラフ#数列#数列とその和（等差・等比・階差・Σ）#関数と極限#関数（分数関数・無理関数・逆関数と合成関数）#数列の極限#関数の極限#数学(高校生)#数B#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$m,n$：自然数
$m \geqq 2$
$f(\theta)=\displaystyle \frac{\sin\ n\theta}{\cos\ n\theta+m}$の最大値を$\alpha(m,n)$とする
$\displaystyle \sum_{m=2}^\infty \{\alpha(m,n)\}^2$を求めよ
この動画を見る
$m,n$：自然数
$m \geqq 2$
$f(\theta)=\displaystyle \frac{\sin\ n\theta}{\cos\ n\theta+m}$の最大値を$\alpha(m,n)$とする
$\displaystyle \sum_{m=2}^\infty \{\alpha(m,n)\}^2$を求めよ
大学入試問題#489「これは教科書の例題」 兵庫医科大学(2023) #定積分
単元：
#数Ⅱ#微分法と積分法#不定積分・定積分#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1} (x+2)(x-1)^9 dx$
出典：2023年兵庫医科大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1} (x+2)(x-1)^9 dx$
出典：2023年兵庫医科大学 入試問題
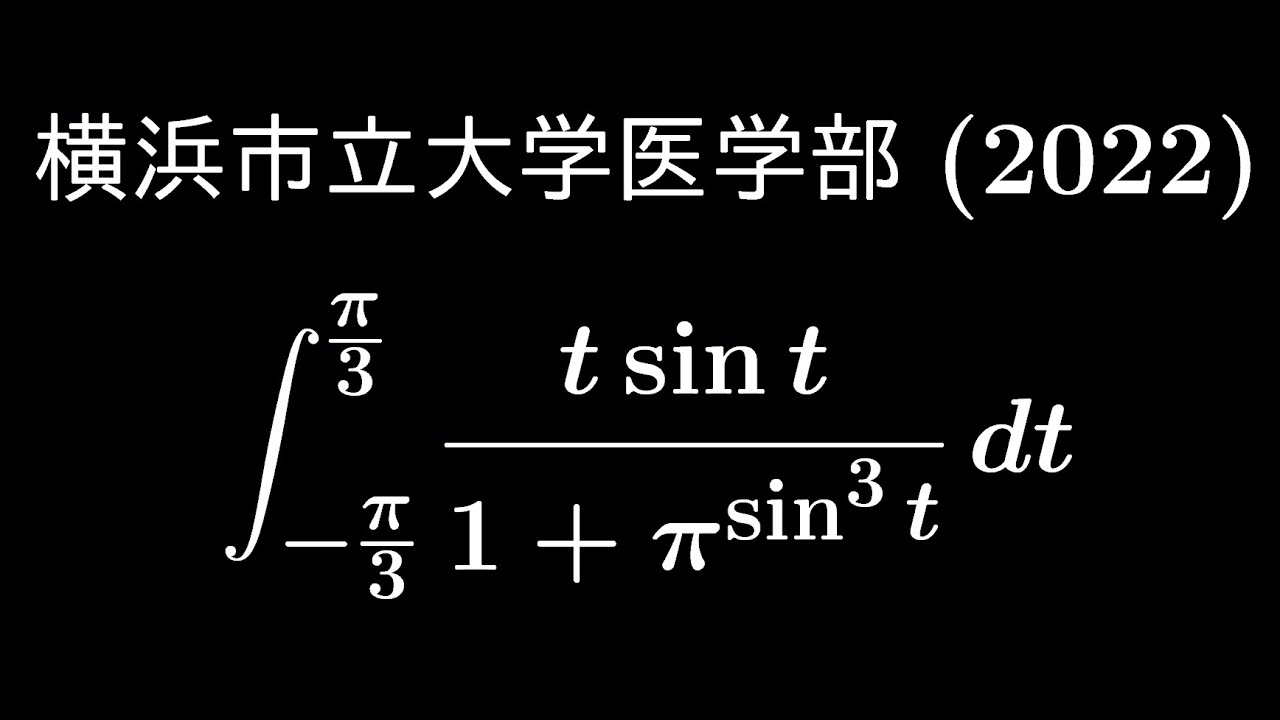
大学入試問題#488「もはや盤上この一手」 横浜市立大学医学部(2022) #定積分
単元：
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-\frac{\pi}{3}}^{\frac{\pi}{3}} \displaystyle \frac{t\ \sin\ t}{1+\pi^{\sin^3t}}dt$
出典：2022年横浜市立大学 入試問題
この動画を見る
$\displaystyle \int_{-\frac{\pi}{3}}^{\frac{\pi}{3}} \displaystyle \frac{t\ \sin\ t}{1+\pi^{\sin^3t}}dt$
出典：2022年横浜市立大学 入試問題