ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！
職業：数学者
職歴：高校非常勤（院生時代）、高専、大学 准教授
趣味：
①将棋 棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀 アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス 中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。
大学入試問題#863「ごちゃごちゃしとる」 #産業医科大学(2012) #極限
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty } \{\sqrt[ 3 ]{ (n^3-n^2)^2 }-2n\sqrt[ 3 ]{ n^3-n^2 }+n^2$
出典：2012年産業医科大学
この動画を見る
$\displaystyle \lim_{ n \to \infty } \{\sqrt[ 3 ]{ (n^3-n^2)^2 }-2n\sqrt[ 3 ]{ n^3-n^2 }+n^2$
出典：2012年産業医科大学
大学入試問題#862「一言、よくある良問」 #横浜国立大学 #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{2} x^3\sqrt{ 4-x^2 } dx$
出典：横浜国立大学
この動画を見る
$\displaystyle \int_{0}^{2} x^3\sqrt{ 4-x^2 } dx$
出典：横浜国立大学
大学入試問題#861「初見では苦しいか！？」 #学習院大学(2017) 視聴者の僚太さんの紹介
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#学習院大学
指導講師：
ますただ
問題文全文(内容文)：
$a \gt 0,b \gt 0$
$\displaystyle \lim_{ x \to \infty } x \sin(\sqrt{ a^2x^2+b }-ax)$
出典：2017年学習大学
この動画を見る
$a \gt 0,b \gt 0$
$\displaystyle \lim_{ x \to \infty } x \sin(\sqrt{ a^2x^2+b }-ax)$
出典：2017年学習大学
視聴者の僚太さんの難易度高めの積分です。大事な感覚が盛り込まれてます。
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#大学入試解答速報
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{x^2}{(1+x^2)\sqrt{ 1+x^2 }}$
この動画を見る
$\displaystyle \int \displaystyle \frac{x^2}{(1+x^2)\sqrt{ 1+x^2 }}$
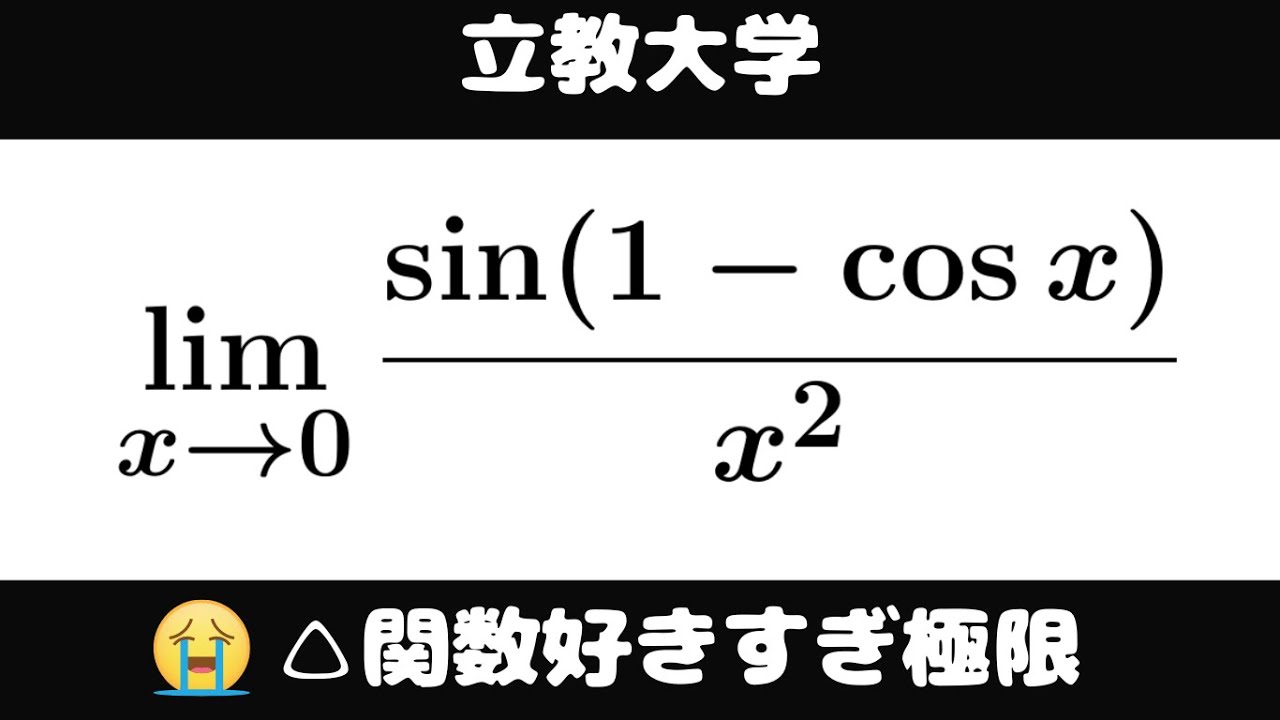
大学入試問題#860「これ、ええ問題」 #立教大学 #極限
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#立教大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{\sin(1-\cos x)}{x^2}$
出典：立教大学 入試問題
この動画を見る
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{\sin(1-\cos x)}{x^2}$
出典：立教大学 入試問題
大学入試問題#859「これは基本！」 #慶應義塾大学商学部(2012)
単元：
#英語(高校生)#大学入試過去問(英語)#学校別大学入試過去問解説(英語)#慶應義塾大学
指導講師：
ますただ
問題文全文(内容文)：
$0 \lt \theta \lt \displaystyle \frac{\pi}{2}$のとき
$log_4(\sin^5\theta)+log_4(\cos^5\theta)$の最大値を求めよ
出典：2012年慶應義塾大学商学部 入試問題
この動画を見る
$0 \lt \theta \lt \displaystyle \frac{\pi}{2}$のとき
$log_4(\sin^5\theta)+log_4(\cos^5\theta)$の最大値を求めよ
出典：2012年慶應義塾大学商学部 入試問題
大学入試問題#862「計算力と根性！」 #京都大学(2023) #数列
単元：
#大学入試過去問(数学)#数列#数列とその和（等差・等比・階差・Σ）#漸化式#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数B
指導講師：
ますただ
問題文全文(内容文)：
$\begin{eqnarray}
\left\{
\begin{array}{l}
a_1=3 \\
a_n=\displaystyle \frac{S_n}{n}+(n-1)・2^n
\end{array}
\right.
\end{eqnarray}$
を満たすような数列$\{a_n\}$の一般項を求めよ
出典：2023年京都大学 入試問題
この動画を見る
$\begin{eqnarray}
\left\{
\begin{array}{l}
a_1=3 \\
a_n=\displaystyle \frac{S_n}{n}+(n-1)・2^n
\end{array}
\right.
\end{eqnarray}$
を満たすような数列$\{a_n\}$の一般項を求めよ
出典：2023年京都大学 入試問題
大学入試問題#857「スッキリとした解答になるはず」 #大阪市立大学(1998) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#大阪市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{2} \displaystyle \frac{1}{\mathit{u}^{(\frac{3}{2})}}\{\sin(log\ \mathit{u})+\displaystyle \frac{1}{2}\cos(log\ \mathit{u})\}du$
出典：1998年大阪市立大学
この動画を見る
$\displaystyle \int_{1}^{2} \displaystyle \frac{1}{\mathit{u}^{(\frac{3}{2})}}\{\sin(log\ \mathit{u})+\displaystyle \frac{1}{2}\cos(log\ \mathit{u})\}du$
出典：1998年大阪市立大学
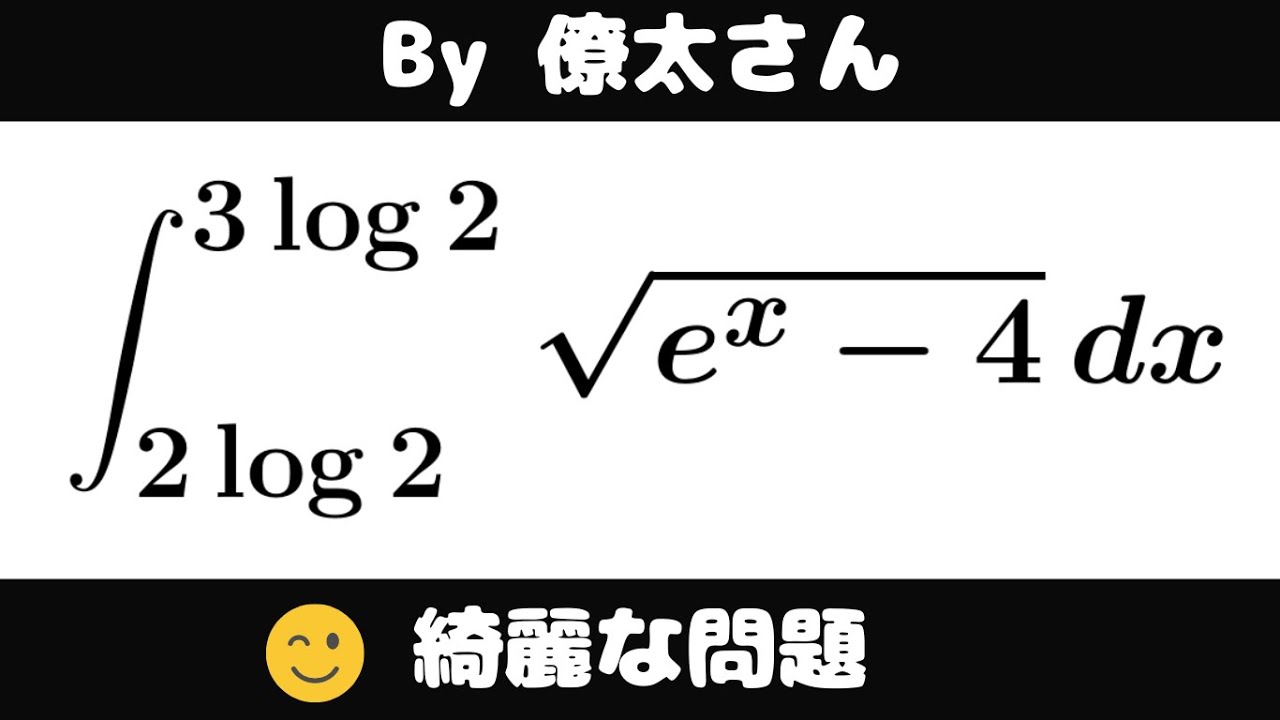
視聴者の僚太さんの積分「編集に5時間・・・・」
単元：
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{2\ log\ 2}^{3\ log\ 2} \sqrt{ e^x-4 } \ dx$
この動画を見る
$\displaystyle \int_{2\ log\ 2}^{3\ log\ 2} \sqrt{ e^x-4 } \ dx$
大学入試問題#856「対称性なら余裕！」 #産業医科大学(2012) #高次方程式
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$2x^4+7x^3+4x^2+7x+2=0$の実数解を求めよ
出典：2012年産業医科大学 入試問題
この動画を見る
$2x^4+7x^3+4x^2+7x+2=0$の実数解を求めよ
出典：2012年産業医科大学 入試問題
大学入試問題#855「47の主張が強すぎる」 #自治医科大学(2012) #式変形
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#自治医科大学
指導講師：
ますただ
問題文全文(内容文)：
$x^{\frac{1}{4}}+x^{-\frac{1}{4}}=3$のとき
$\displaystyle \frac{47}{2}(\displaystyle \frac{x^{\frac{3}{4}}+x^{-\frac{3}{4}}}{x+x^{-1}})$の値を求めよ。
$(0 \lt x:$実数$)$
出典：2012年自治医科大学
この動画を見る
$x^{\frac{1}{4}}+x^{-\frac{1}{4}}=3$のとき
$\displaystyle \frac{47}{2}(\displaystyle \frac{x^{\frac{3}{4}}+x^{-\frac{3}{4}}}{x+x^{-1}})$の値を求めよ。
$(0 \lt x:$実数$)$
出典：2012年自治医科大学
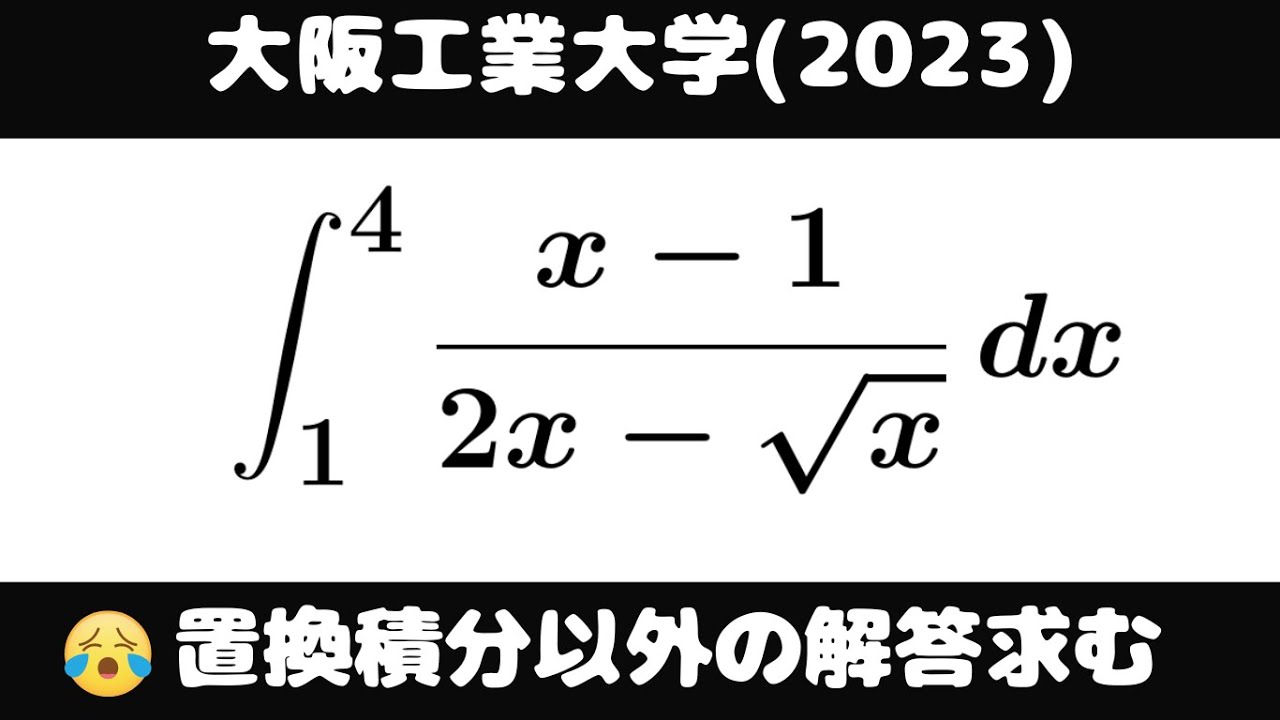
#大学入試問題#853「ファンタスティックな解答求む」 #大阪工業大学(2023) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#大阪工業大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{4} \displaystyle \frac{x-1}{2x-\sqrt{ x }} dx$
出典：2023年大阪工業大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{4} \displaystyle \frac{x-1}{2x-\sqrt{ x }} dx$
出典：2023年大阪工業大学 入試問題
大学入試問題#852「これは、大変・・・グラフでもいけるんかなー」 #小樽商科大学(2018) #整数問題
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#小樽商科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \frac{2n-2}{n^2+2n+2}$が整数となるような整数$n$をすべて求めよ
出典：2018年小樽商科大学
この動画を見る
$\displaystyle \frac{2n-2}{n^2+2n+2}$が整数となるような整数$n$をすべて求めよ
出典：2018年小樽商科大学
大学入試問題#851「いやー見た目がきつい」 #自治医科大(2018)
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#自治医科大学
指導講師：
ますただ
問題文全文(内容文)：
$x$が$0$以上の実数であるとき、関数$f(x)=\displaystyle \frac{x^4-2x^3-x^2+2x+34}{x^2-x+3}$の最小値を求めよ
出典：2018年自治医科大学 入試問題
この動画を見る
$x$が$0$以上の実数であるとき、関数$f(x)=\displaystyle \frac{x^4-2x^3-x^2+2x+34}{x^2-x+3}$の最小値を求めよ
出典：2018年自治医科大学 入試問題
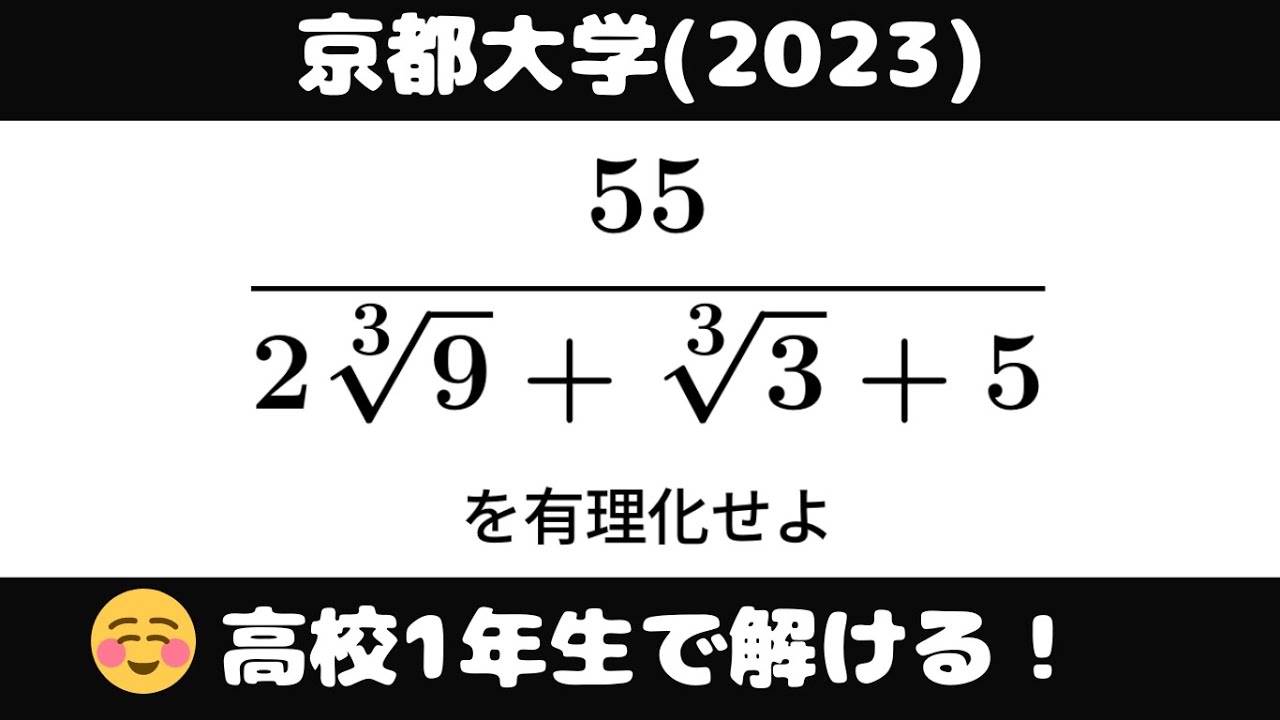
大学入試問題#850「おもろいパズル」 #京都大学(2023) #有理化 #式変形
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \frac{55}{2\sqrt[ 3 ]{ 9 }+\sqrt[ 3 ]{ 3 }+5}$を有利化せよ
出典：2023年京都大学
この動画を見る
$\displaystyle \frac{55}{2\sqrt[ 3 ]{ 9 }+\sqrt[ 3 ]{ 3 }+5}$を有利化せよ
出典：2023年京都大学
大学入試問題#849「これ得意かも」 #和歌山大学(2017) #式変形
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#和歌山大学
指導講師：
ますただ
問題文全文(内容文)：
$\sqrt[ 3 ]{ \sqrt{ 5 }+2 }-\sqrt[ 3 ]{ \sqrt{ 5 }-2 }$が整数であることを示せ
出典：2017年和歌山大学 入試問題
この動画を見る
$\sqrt[ 3 ]{ \sqrt{ 5 }+2 }-\sqrt[ 3 ]{ \sqrt{ 5 }-2 }$が整数であることを示せ
出典：2017年和歌山大学 入試問題
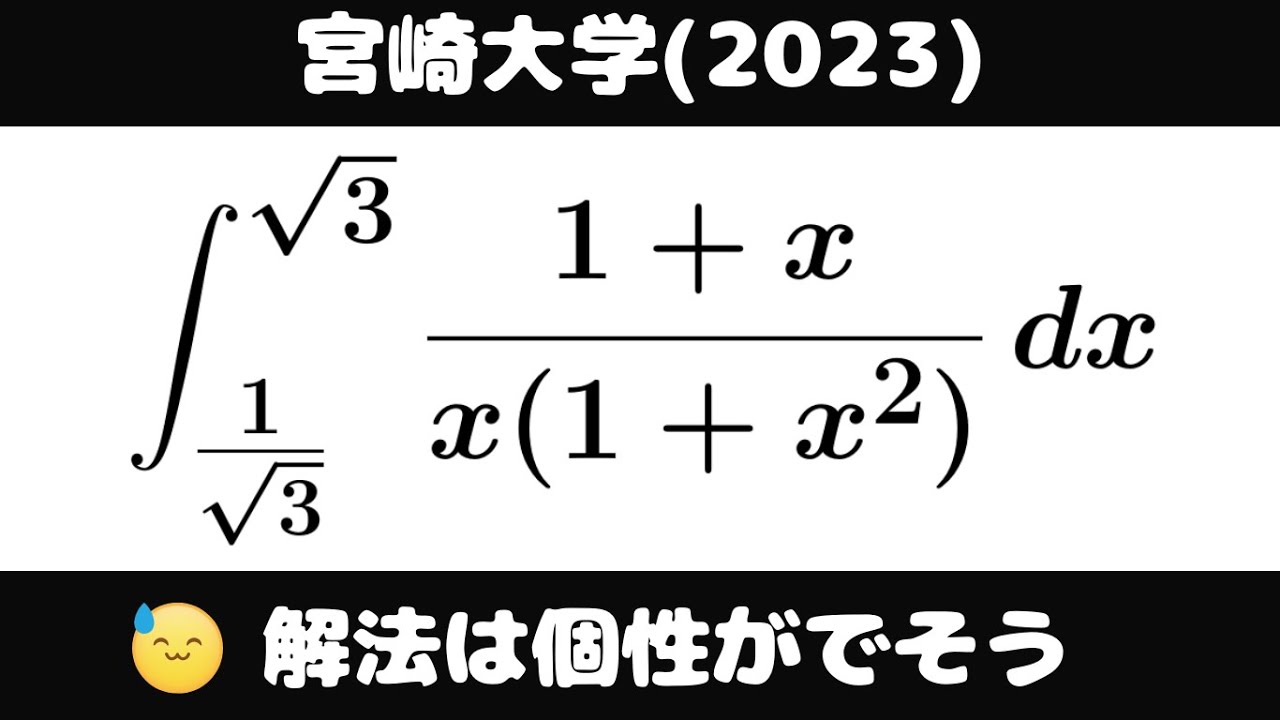
大学入試問題#848「何種類か解法がありそう」 #宮崎大学(2023) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#宮崎大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{\frac{1}{\sqrt{ 3 }}}^{\sqrt{ 3 }} \displaystyle \frac{1+x}{x(1+x^2)} dx$
出典：2023年宮崎大学
この動画を見る
$\displaystyle \int_{\frac{1}{\sqrt{ 3 }}}^{\sqrt{ 3 }} \displaystyle \frac{1+x}{x(1+x^2)} dx$
出典：2023年宮崎大学
大学入試問題#847「もうネタ切れ寸前」 #青山学院大学(2006) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数Ⅲ#青山学院大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1} \{log(4-x^2)+2\} dx$
出典：2006年青山学院大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1} \{log(4-x^2)+2\} dx$
出典：2006年青山学院大学 入試問題
#自治医科大(2015)
単元：
#数Ⅰ#大学入試過去問(数学)#数と式#実数と平方根（循環小数・有理数・無理数・絶対値・平方根計算・2重根号）#学校別大学入試過去問解説(数学)#自治医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \frac{1}{1+\sqrt{ 3 }}+\displaystyle \frac{1}{\sqrt{ 3 }+\sqrt{ 5 }}+\displaystyle \frac{1}{\sqrt{ 5 }+\sqrt{ 7 }}+\displaystyle \frac{1}{\sqrt{ 7 }+\sqrt{ 9 }}$
出典：2015年自治医科大学
この動画を見る
$\displaystyle \frac{1}{1+\sqrt{ 3 }}+\displaystyle \frac{1}{\sqrt{ 3 }+\sqrt{ 5 }}+\displaystyle \frac{1}{\sqrt{ 5 }+\sqrt{ 7 }}+\displaystyle \frac{1}{\sqrt{ 7 }+\sqrt{ 9 }}$
出典：2015年自治医科大学
大学入試問題#846「基本問題」 #岩手大学(2017) #極限
単元：
#大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ x \to 0 } (1+x)^{\frac{1}{x}}=e$を利用して
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{\tan x-\sin x}{x^4}\{log(x^2+x^3)-log\ x^2\}$を求めよ
出典：2017年岩手大学 入試問題
この動画を見る
$\displaystyle \lim_{ x \to 0 } (1+x)^{\frac{1}{x}}=e$を利用して
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{\tan x-\sin x}{x^4}\{log(x^2+x^3)-log\ x^2\}$を求めよ
出典：2017年岩手大学 入試問題
#広島市立大学(2013)
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \sin^9x \cos^3x\ dx$
出典：2013年広島市立大学
この動画を見る
$\displaystyle \int \sin^9x \cos^3x\ dx$
出典：2013年広島市立大学
大学入試問題#845「気持ち応用か！？」 #電気通信大学(2020) #区分求積法
単元：
#大学入試過去問(数学)#積分とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=n+1}^{2n} \displaystyle \frac{n}{k^2+3kn+2n^2}$
出典：2020年電気通信大学
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \sum_{k=n+1}^{2n} \displaystyle \frac{n}{k^2+3kn+2n^2}$
出典：2020年電気通信大学
#高知工科大学(2021)
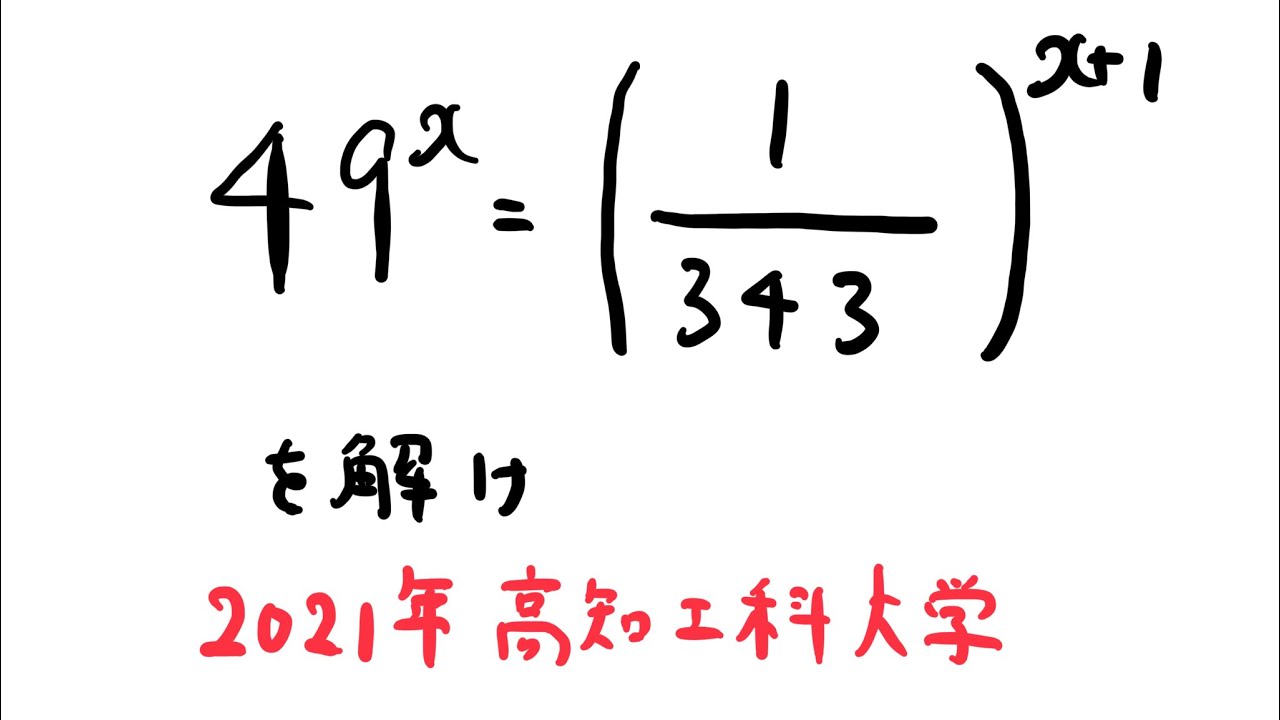
単元：
#数Ⅱ#大学入試過去問(数学)#指数関数と対数関数#指数関数#学校別大学入試過去問解説(数学)#数学(高校生)#高知工科大学
指導講師：
ますただ
問題文全文(内容文)：
$49^=(\displaystyle \frac{1}{343})^{x+1}$を解け
出典：2021年高知工科大学
この動画を見る
$49^=(\displaystyle \frac{1}{343})^{x+1}$を解け
出典：2021年高知工科大学
大学入試問題#844「まあ基本・・・」 #電気通信大学(2015) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{2}} (\sin x)(\sin 2x)(\sin 3x) dx$
出典：2015年電気通信大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} (\sin x)(\sin 2x)(\sin 3x) dx$
出典：2015年電気通信大学 入試問題
#大阪医科大学(2014) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#大阪医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-1}^{1} x \sin n \pi \ x\ dx$
$n$：自然数
出典：2014年大阪医科大学
この動画を見る
$\displaystyle \int_{-1}^{1} x \sin n \pi \ x\ dx$
$n$：自然数
出典：2014年大阪医科大学
大学入試問題#843「解き方色々ありそう」 ＃筑波大学(2013) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{1}{\sin x \cos x} dx$
出典：2013年筑波大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{1}{\sin x \cos x} dx$
出典：2013年筑波大学 入試問題
#大阪医科大学2014
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#大阪医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-1}^{1} \sin^2 n\pi \ x \ dx$
$n:$自然数
出典：2014年大阪医科大学
この動画を見る
$\displaystyle \int_{-1}^{1} \sin^2 n\pi \ x \ dx$
$n:$自然数
出典：2014年大阪医科大学
大学入試問題#842「公式は使っていません」 #電気通信大学(2018) ＃定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#電気通信大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-1}^{1} (1+x)^4(1-x)^2 dx$
出典：2018年電気通信大学 入試問題
この動画を見る
$\displaystyle \int_{-1}^{1} (1+x)^4(1-x)^2 dx$
出典：2018年電気通信大学 入試問題
#上智大学(2023) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#上智大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{2}{3}\pi} x\sin2x\ dx$
出典：2023年上智大学
この動画を見る
$\displaystyle \int_{0}^{\frac{2}{3}\pi} x\sin2x\ dx$
出典：2023年上智大学
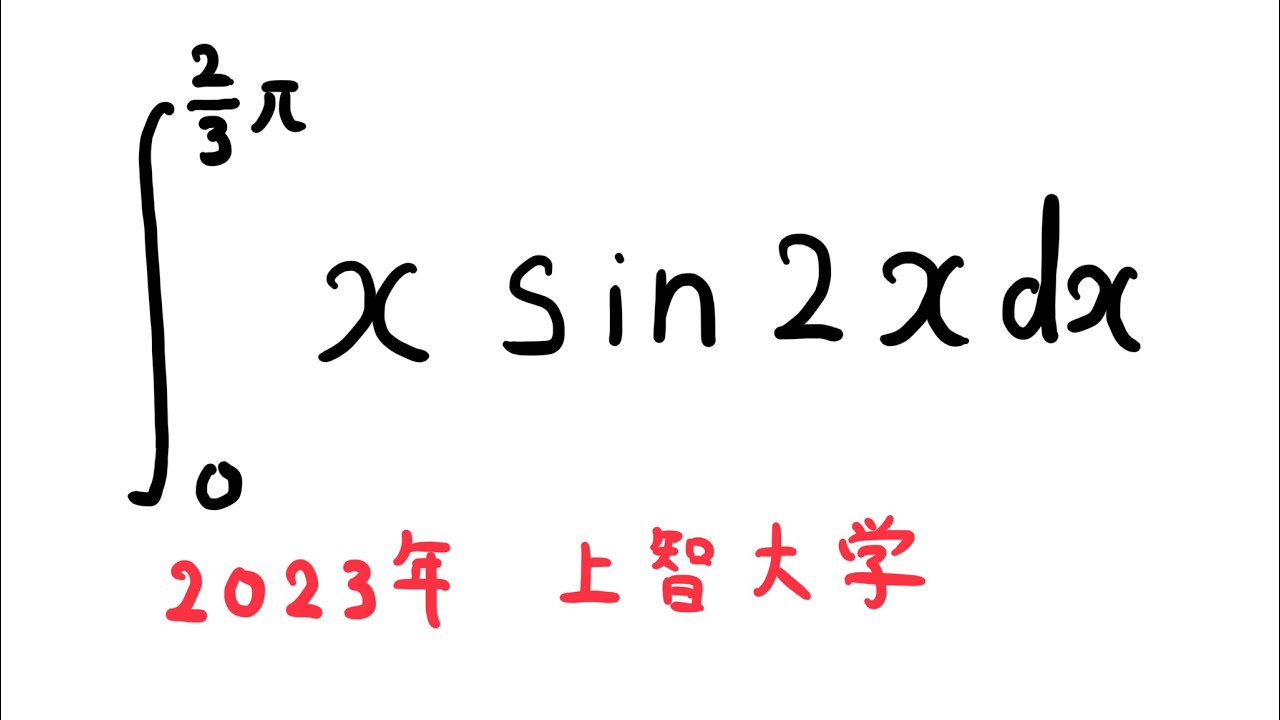
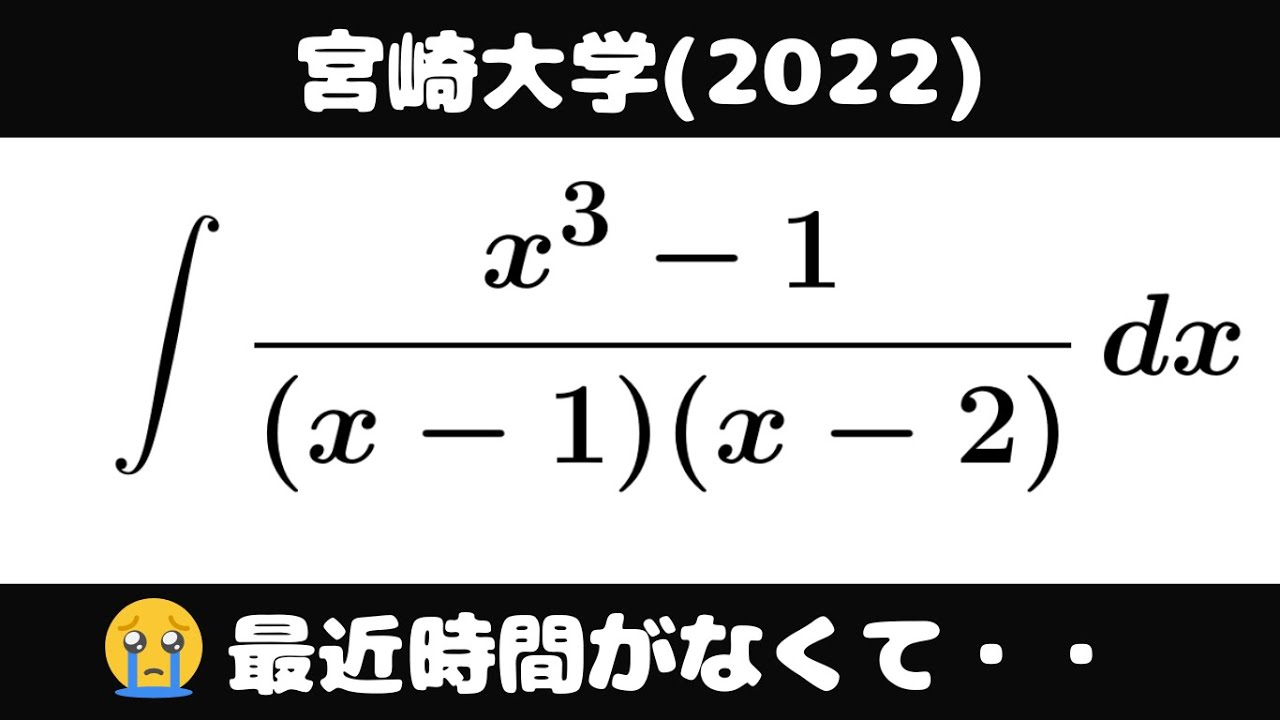
大学入試問題#841「因数分解が丸出し・・・・」 #宮崎大学(2022) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#宮崎大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{x^3-1}{(x-1)(x-2)} dx$
出典：2022年宮崎大学
この動画を見る
$\displaystyle \int \displaystyle \frac{x^3-1}{(x-1)(x-2)} dx$
出典：2022年宮崎大学