 学校別大学入試過去問解説(数学)
学校別大学入試過去問解説(数学)
 学校別大学入試過去問解説(数学)
学校別大学入試過去問解説(数学)
大学入試問題#831「教科書の章末問題」 #山形大学(2010) #三角関数
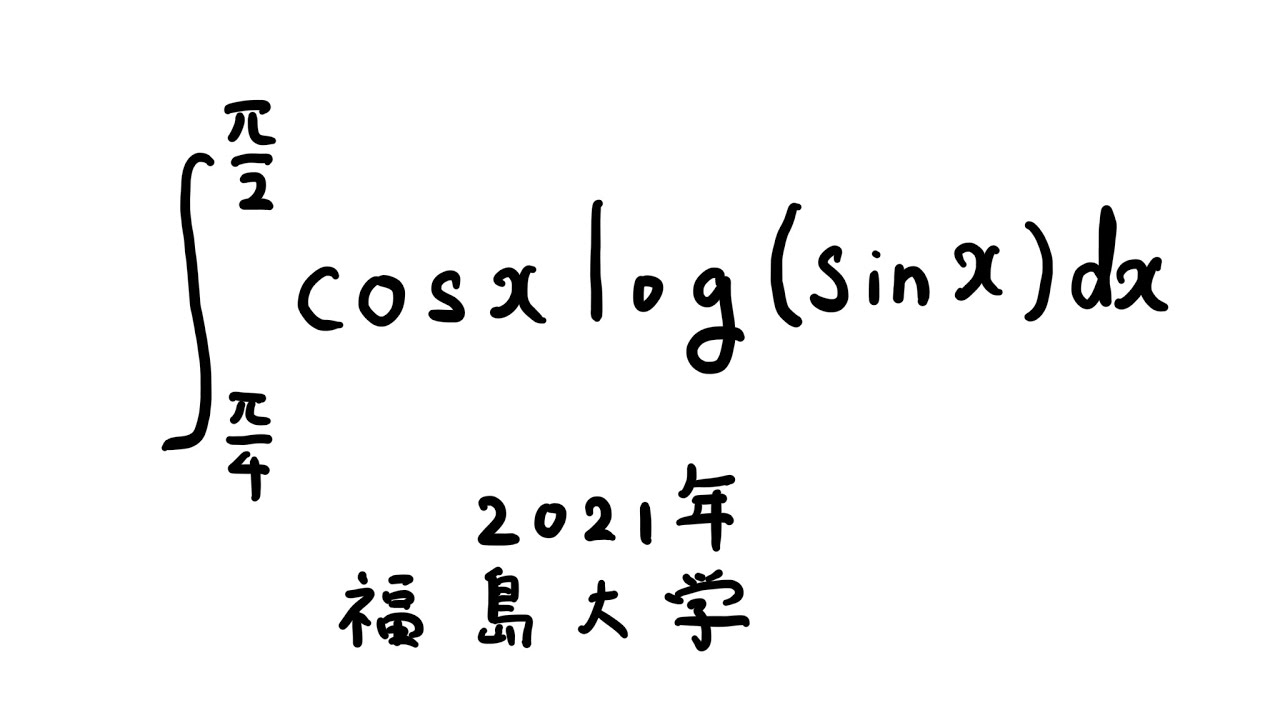
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#山形大学
指導講師:
ますただ
問題文全文(内容文):
$\sin\displaystyle \frac{19}{12}\pi$の値を求めよ
出典:2010年山形大学
この動画を見る
$\sin\displaystyle \frac{19}{12}\pi$の値を求めよ
出典:2010年山形大学
#福島大学(2021) #定積分 #Shorts
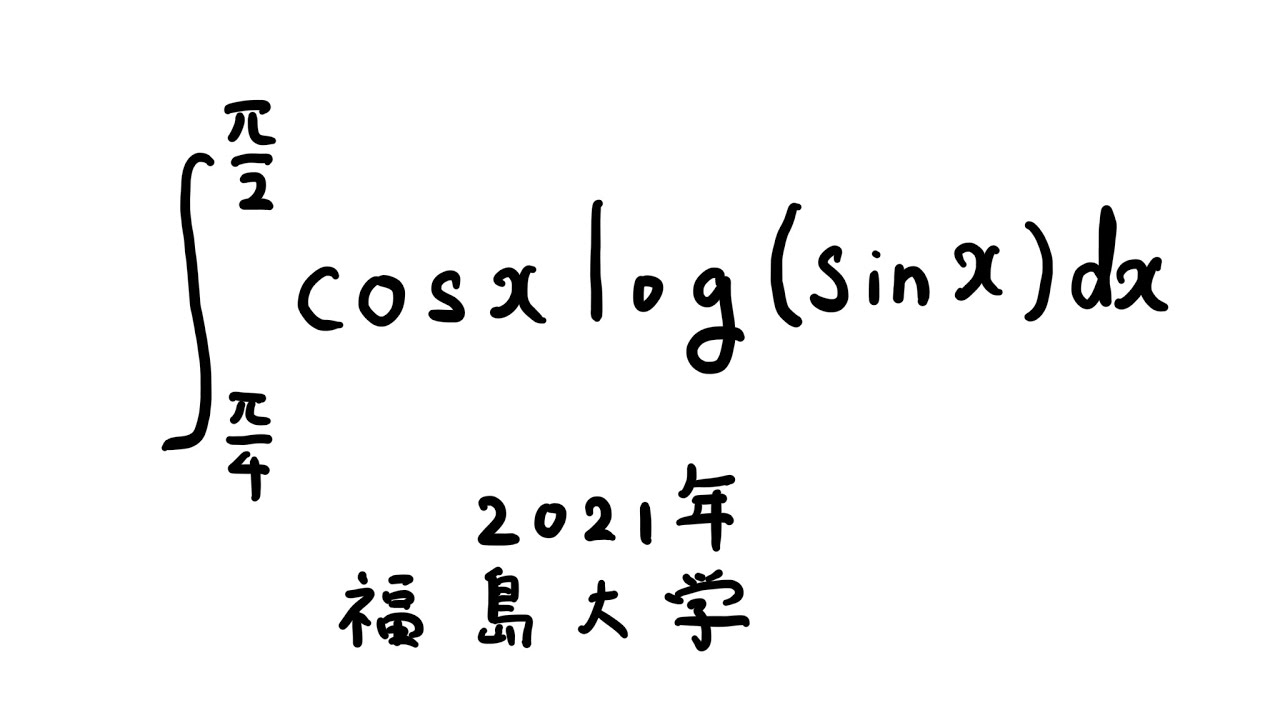
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#福島大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{4}}^{\frac{\pi}{2}} \cos\ x\ log(\sin\ x) dx$
出典:2021年福島大学
この動画を見る
$\displaystyle \int_{\frac{\pi}{4}}^{\frac{\pi}{2}} \cos\ x\ log(\sin\ x) dx$
出典:2021年福島大学
#岩手大学(2015) #定積分 #Shorts
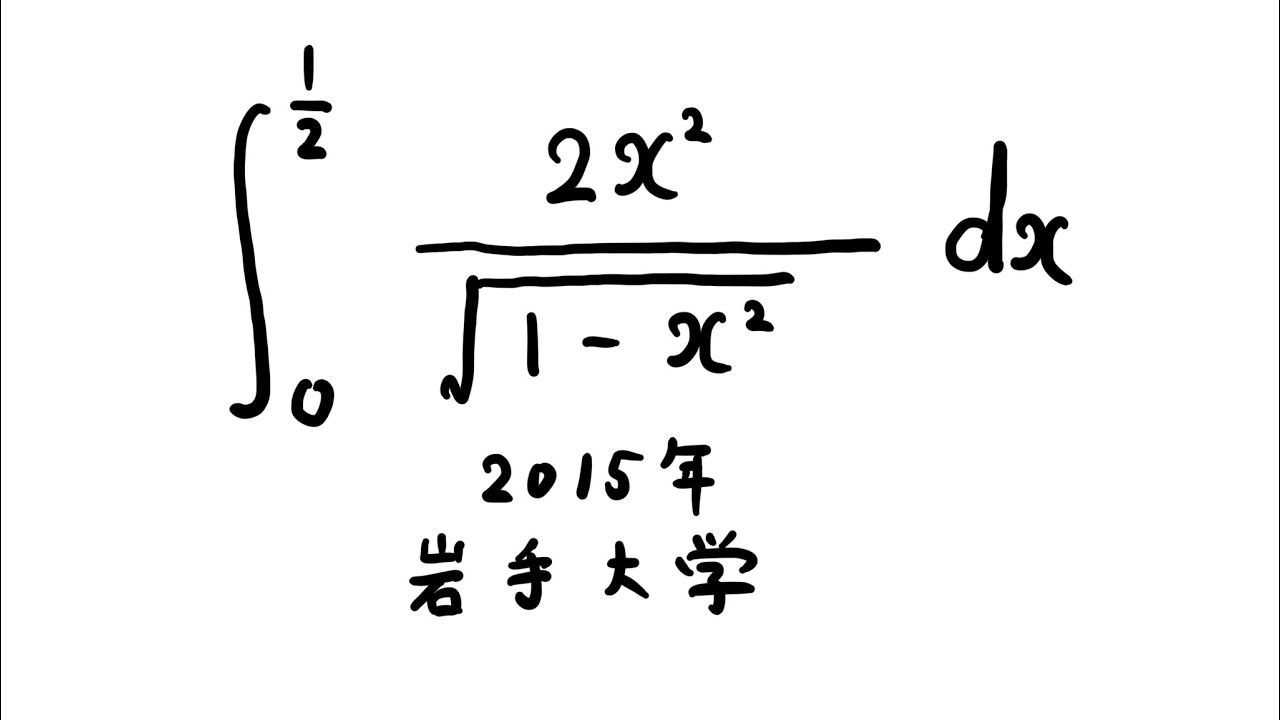
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{1}{2}} \displaystyle \frac{2x^2}{\sqrt{ 1-x^2 }} dx$
出典:2015年岩手大学
この動画を見る
$\displaystyle \int_{0}^{\frac{1}{2}} \displaystyle \frac{2x^2}{\sqrt{ 1-x^2 }} dx$
出典:2015年岩手大学
大学入試問題#830「たまには、こんな問題でも」 #筑波大学(2016) #極限

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{\cos4x-2\cos\ 2x+1}{x^2}$
出典:2016年筑波大学
この動画を見る
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{\cos4x-2\cos\ 2x+1}{x^2}$
出典:2016年筑波大学
#上智大学(2005) #Shorts

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#上智大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$f(x)=x^2+x+2\displaystyle \int_{0}^{1} f(t) dt$を満たす関数$f(x)$を求めよ
出典:2005年上智大学
この動画を見る
$f(x)=x^2+x+2\displaystyle \int_{0}^{1} f(t) dt$を満たす関数$f(x)$を求めよ
出典:2005年上智大学
#福島大学(2020) #不定積分 #Shorts
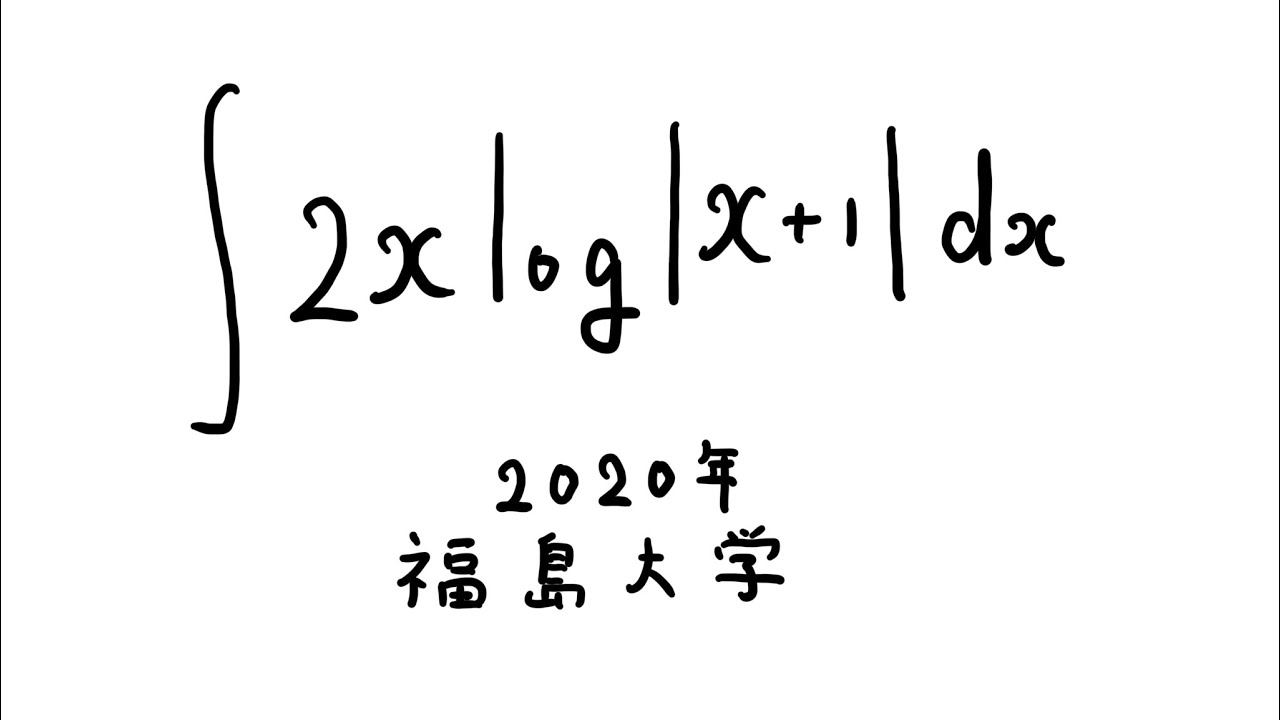
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#福島大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int 2\ x\ log|x+1|dx$
出典:2020年福島大学
この動画を見る
$\displaystyle \int 2\ x\ log|x+1|dx$
出典:2020年福島大学
大学入試問題#829「綺麗な詰将棋!」 #筑波大学(2016) #定積分

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} \displaystyle \frac{x-1}{x^3+1} dx$
出典:2016年筑波大学
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{x-1}{x^3+1} dx$
出典:2016年筑波大学
#立教大学(2010) #定積分 #Shorts
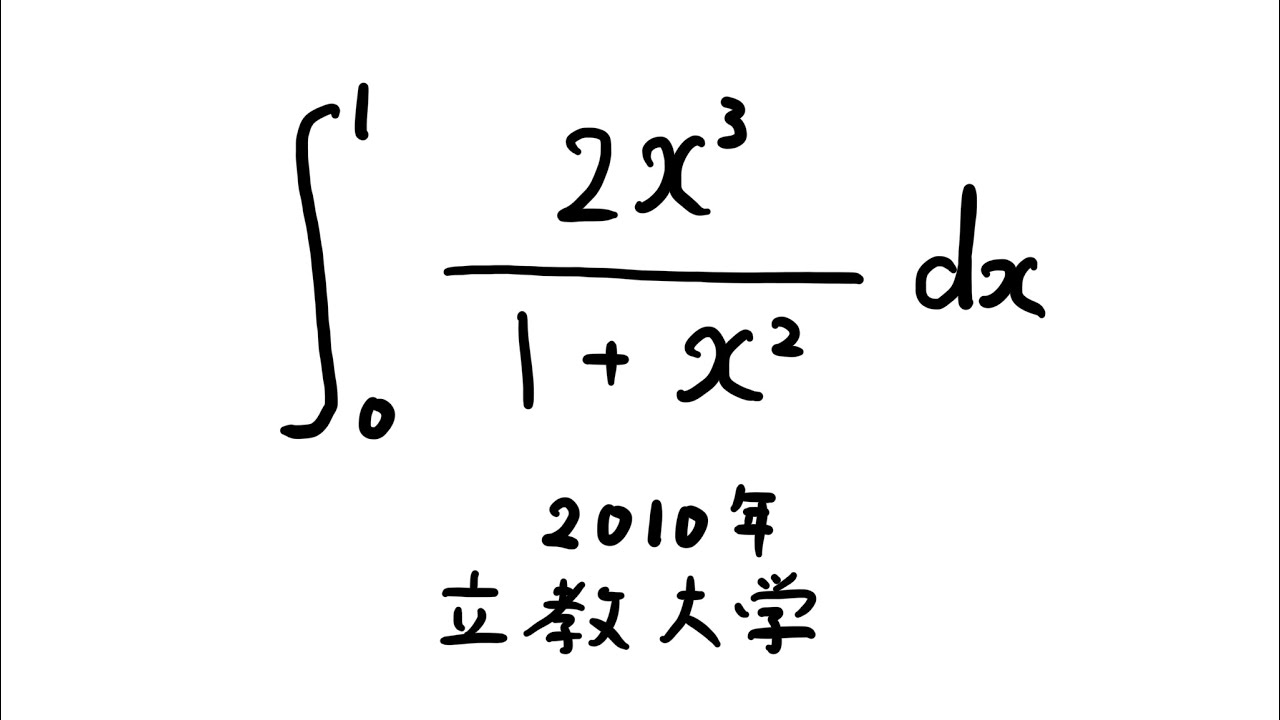
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#立教大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} \displaystyle \frac{2x^3}{1+x^2} dx$
出典:2010年立教大学
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{2x^3}{1+x^2} dx$
出典:2010年立教大学
#岩手大学(2019) #定積分 #Shorts
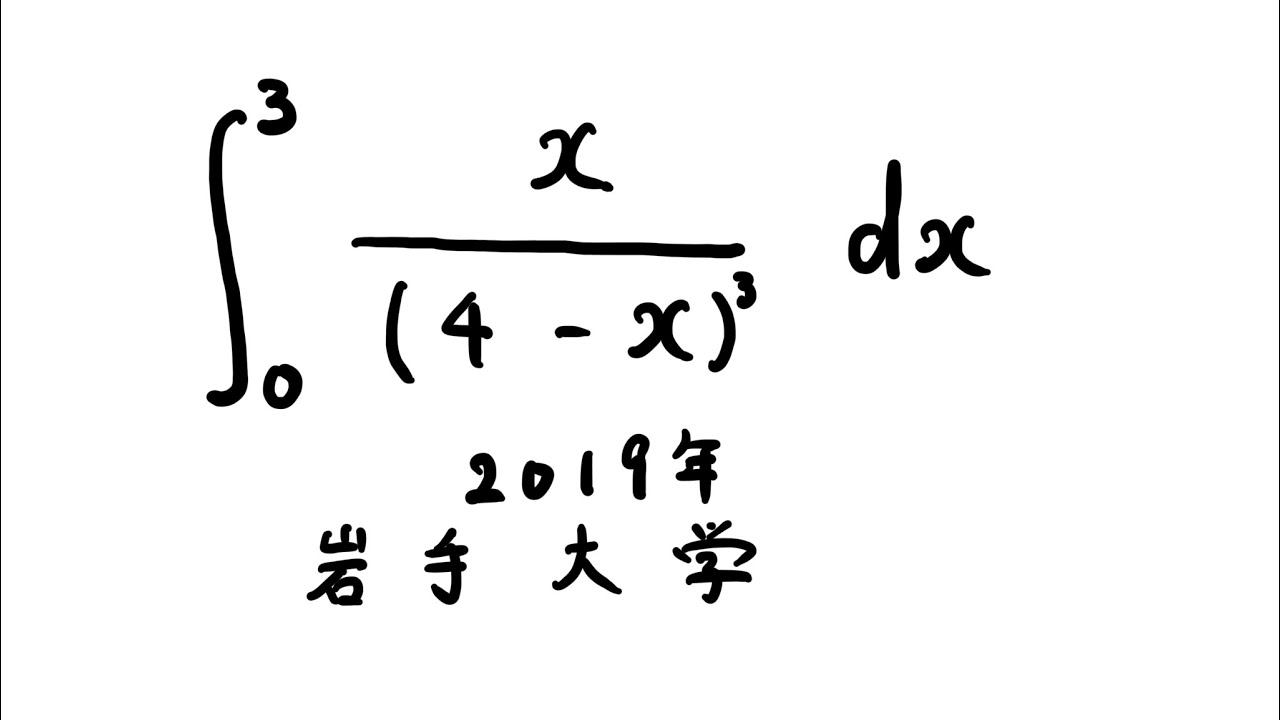
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{3} \displaystyle \frac{x}{(4-x)^3} dx$
出典:2019年岩手大学
この動画を見る
$\displaystyle \int_{0}^{3} \displaystyle \frac{x}{(4-x)^3} dx$
出典:2019年岩手大学
大学入試問題#828「式変形難しめの良問!」 #久留米大学医学部(2024) #数列

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#久留米大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \sum_{k=1}^n \displaystyle \frac{3k+5}{(3k-1)(3k+2)2^{k+1}}$
出典:2024年久留米大学医学部
この動画を見る
$\displaystyle \sum_{k=1}^n \displaystyle \frac{3k+5}{(3k-1)(3k+2)2^{k+1}}$
出典:2024年久留米大学医学部
#東京都市大学(2010) #不定積分 #Shorts
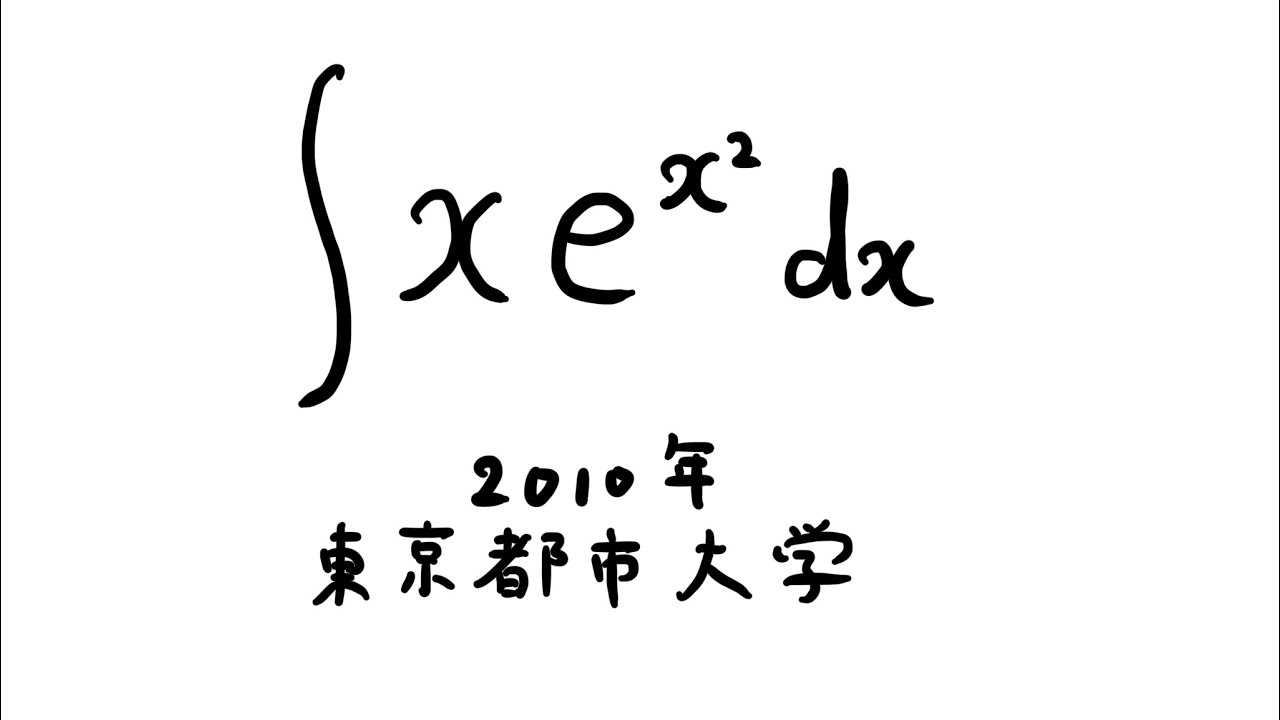
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int xe^{x^2} dx$
出典:2010年東京都市大学
この動画を見る
$\displaystyle \int xe^{x^2} dx$
出典:2010年東京都市大学
#岩手大学(2019) #極限 #Shorts
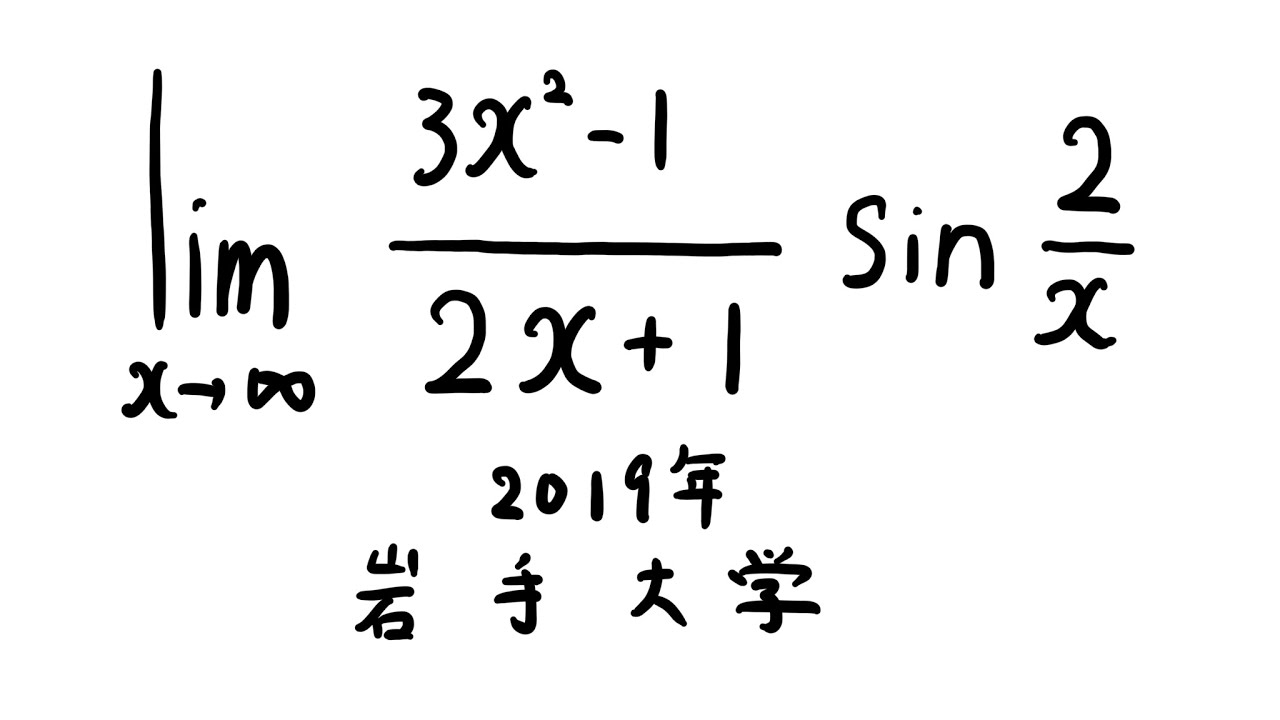
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ x \to \infty } \displaystyle \frac{3x^2-1}{2x+1}\sin\displaystyle \frac{2}{x}$
出典:2019年岩手大学
この動画を見る
$\displaystyle \lim_{ x \to \infty } \displaystyle \frac{3x^2-1}{2x+1}\sin\displaystyle \frac{2}{x}$
出典:2019年岩手大学
大学入試問題#827「とりま絶対値はずそ:0≦t≦π/2」 #筑波大学(2020) #定積分

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}} |\sin\ x-\sin\ t\ |\ dx$
出典:2020年筑波大学
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} |\sin\ x-\sin\ t\ |\ dx$
出典:2020年筑波大学
#岩手大学(2018) #定積分 #Shorts
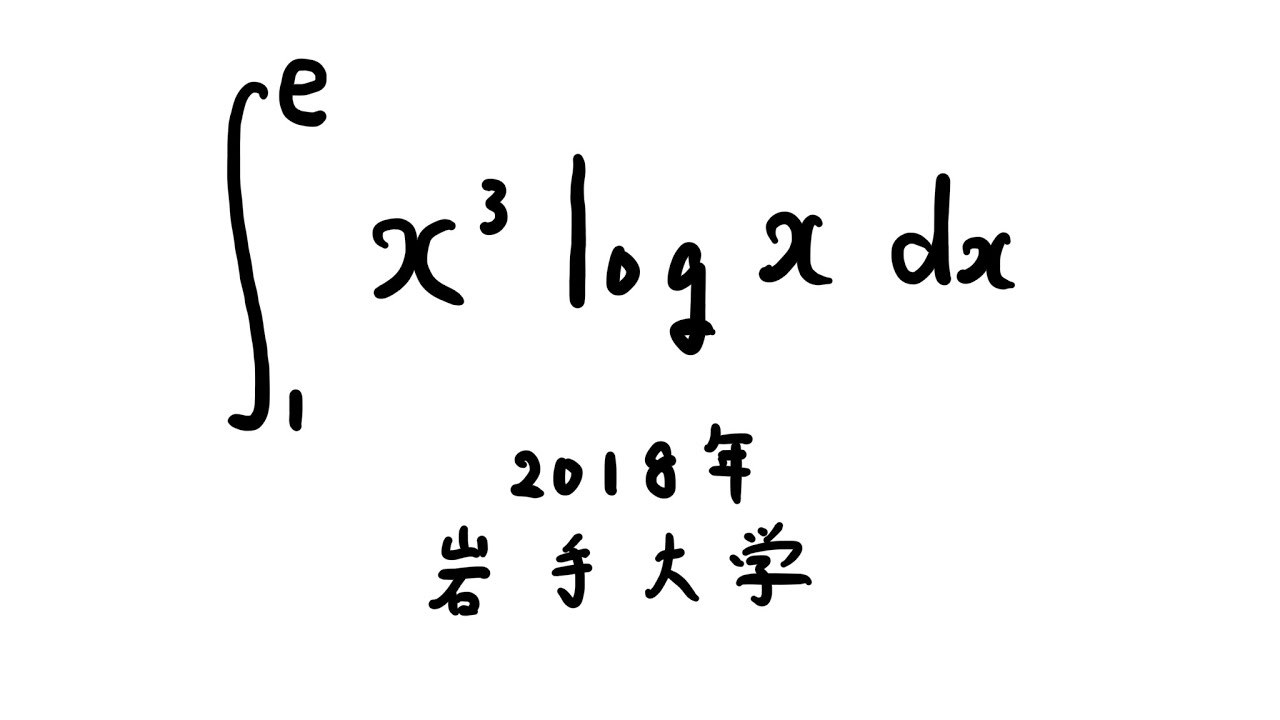
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{e} x^3log\ x\ dx$
出典:2018年岩手大学
この動画を見る
$\displaystyle \int_{1}^{e} x^3log\ x\ dx$
出典:2018年岩手大学
#筑波大学(2016) #定積分 #Shorts
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学
指導講師:
ますただ
問題文全文(内容文):
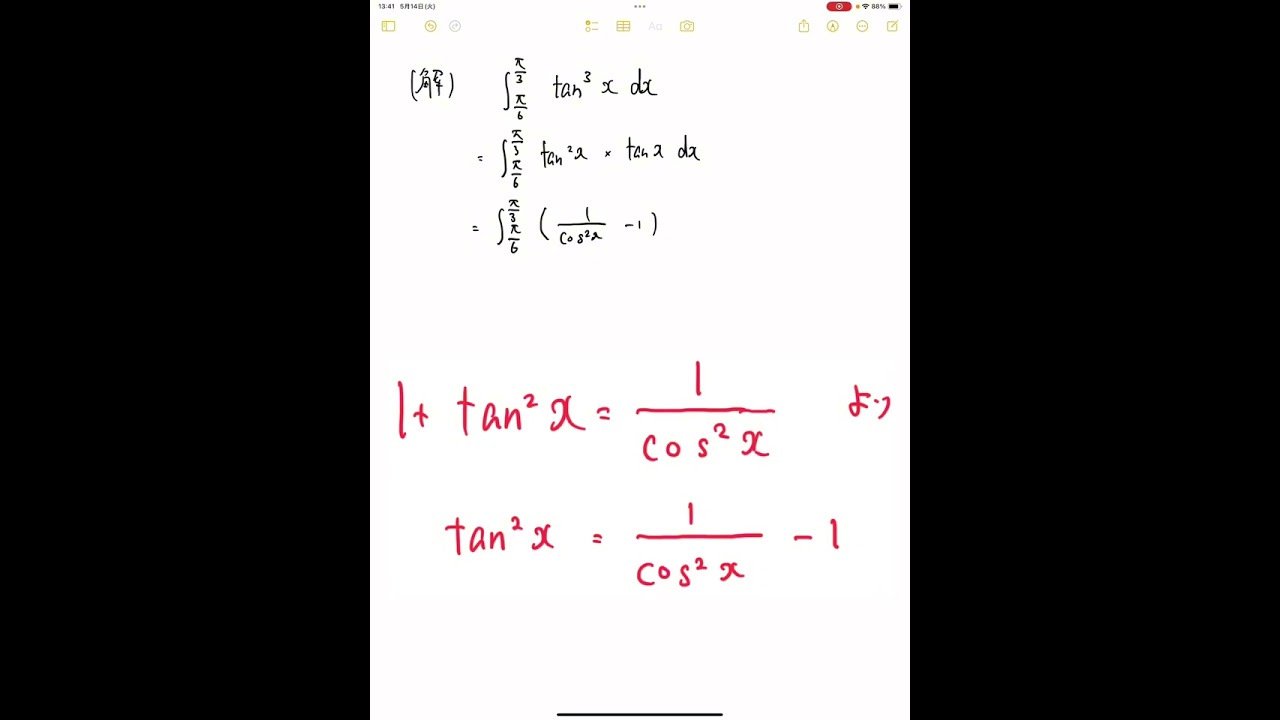
$\displaystyle \int_{\frac{\pi}{6}}^{\frac{\pi}{3}} \tan^3x\ dx$
出典:2016年筑波大学
この動画を見る
$\displaystyle \int_{\frac{\pi}{6}}^{\frac{\pi}{3}} \tan^3x\ dx$
出典:2016年筑波大学
大学入試問題#826「尺の関係で、解法2つ紹介!」 #筑波大学(2019) #定積分
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#筑波大学
指導講師:
ますただ
問題文全文(内容文):
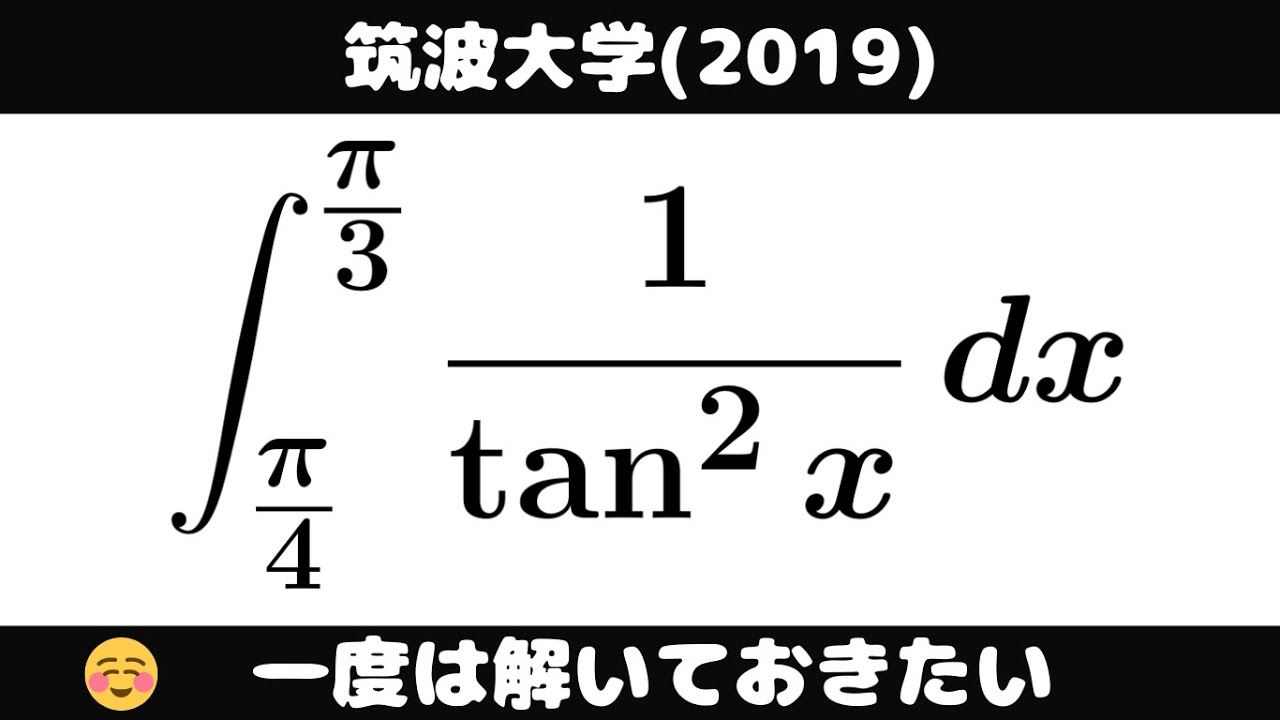
$\displaystyle \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \displaystyle \frac{1}{\tan^2x} dx$
出典:2019年筑波大学
この動画を見る
$\displaystyle \int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \displaystyle \frac{1}{\tan^2x} dx$
出典:2019年筑波大学
#上智大学(2014) #極限 #Shorts
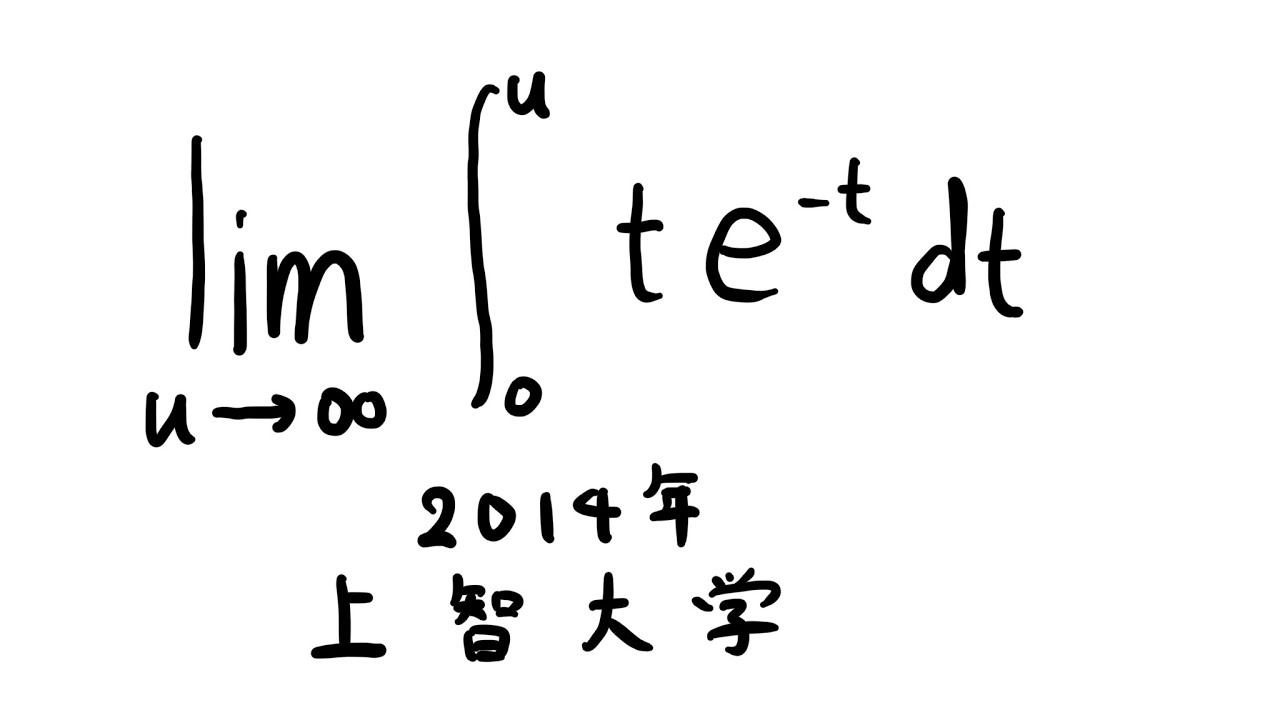
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#上智大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ u \to \infty } \displaystyle \int_{o}^{u} te^{-t} \ dt$
出典:2014年上智大学
この動画を見る
$\displaystyle \lim_{ u \to \infty } \displaystyle \int_{o}^{u} te^{-t} \ dt$
出典:2014年上智大学
#筑波大学(2016) #定積分 #Shorts

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#筑波大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{1}{2}}^{2} |log\ x| dx$
出典:2016年筑波大学
この動画を見る
$\displaystyle \int_{\frac{1}{2}}^{2} |log\ x| dx$
出典:2016年筑波大学
大学入試問題#825「まあまあ良問」 #茨城大学(2022) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-7}^{1}(2-x) \sqrt[ 3 ]{ 1-x }\ dx$
出典:2022年茨城大学
この動画を見る
$\displaystyle \int_{-7}^{1}(2-x) \sqrt[ 3 ]{ 1-x }\ dx$
出典:2022年茨城大学
福田の数学〜慶應義塾大学2024年商学部第2問(4)〜領域と集合の要素の個数

単元:
#数Ⅰ#数Ⅱ#大学入試過去問(数学)#数と式#集合と命題(集合・命題と条件・背理法)#図形と方程式#軌跡と領域#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)
指導講師:
福田次郎
問題文全文(内容文):
$\Large\boxed{2}$ (4)$xy$平面上で、不等式$x$≦5 の表す領域を$A$, 不等式$x$+$y$≧10 の表す領域を$B$とする。また、$xy$平面上の点の集合$S$は以下の3つの条件をすべて満たす。
(条件1)$S$に含まれるどの点も、その$x$座標と$y$座標はともに1以上10以下の自然数である。
(条件2)$S$の要素で領域$A$に含まれるものは、領域$B$に含まれる。
(条件3)$S$の要素で領域$B$に含まれるものは、領域$A$に含まれる。
$S$を、条件1~3を満たす中で要素の個数が最大のものとするとき、その要素の個数は$\boxed{シス}$である。
この動画を見る
$\Large\boxed{2}$ (4)$xy$平面上で、不等式$x$≦5 の表す領域を$A$, 不等式$x$+$y$≧10 の表す領域を$B$とする。また、$xy$平面上の点の集合$S$は以下の3つの条件をすべて満たす。
(条件1)$S$に含まれるどの点も、その$x$座標と$y$座標はともに1以上10以下の自然数である。
(条件2)$S$の要素で領域$A$に含まれるものは、領域$B$に含まれる。
(条件3)$S$の要素で領域$B$に含まれるものは、領域$A$に含まれる。
$S$を、条件1~3を満たす中で要素の個数が最大のものとするとき、その要素の個数は$\boxed{シス}$である。
#秋田大学(2019) #定積分 #Shorts
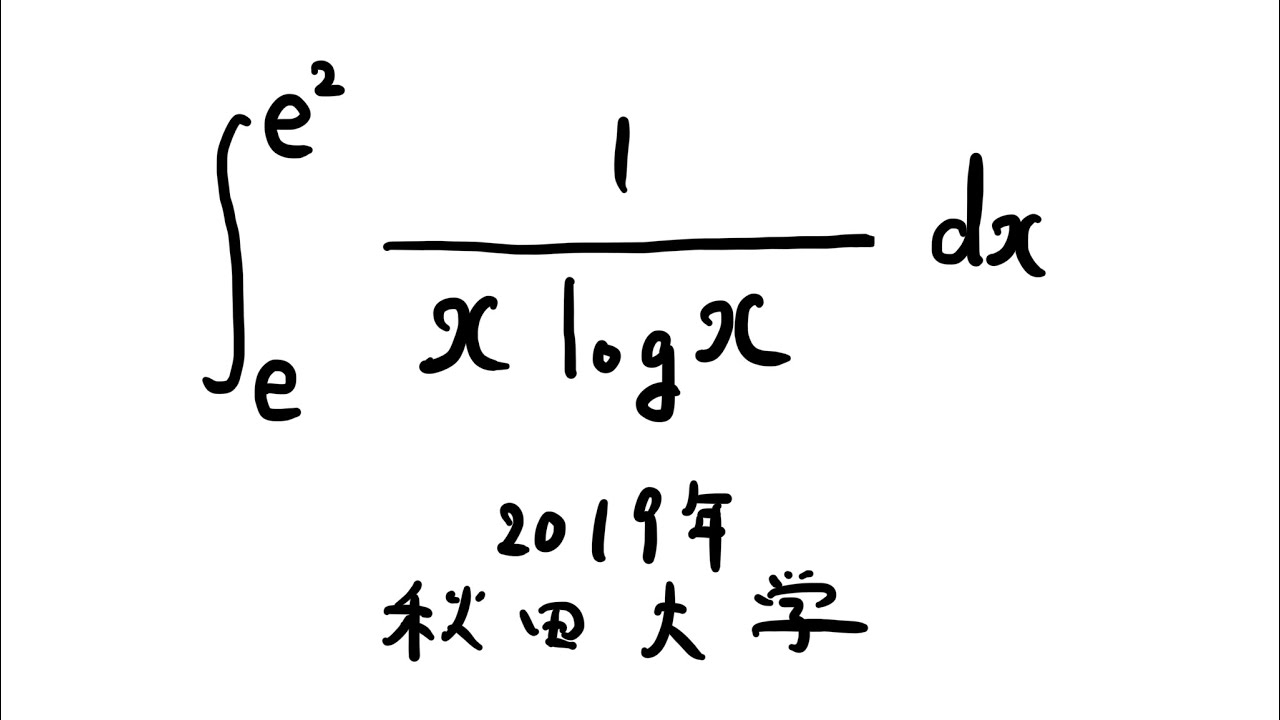
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#秋田大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{e}^{e^2} \displaystyle \frac{1}{x\ log\ x} dx$
出典:2019年秋田大学
この動画を見る
$\displaystyle \int_{e}^{e^2} \displaystyle \frac{1}{x\ log\ x} dx$
出典:2019年秋田大学
#岩手大学(2013) #極限 #Shorts
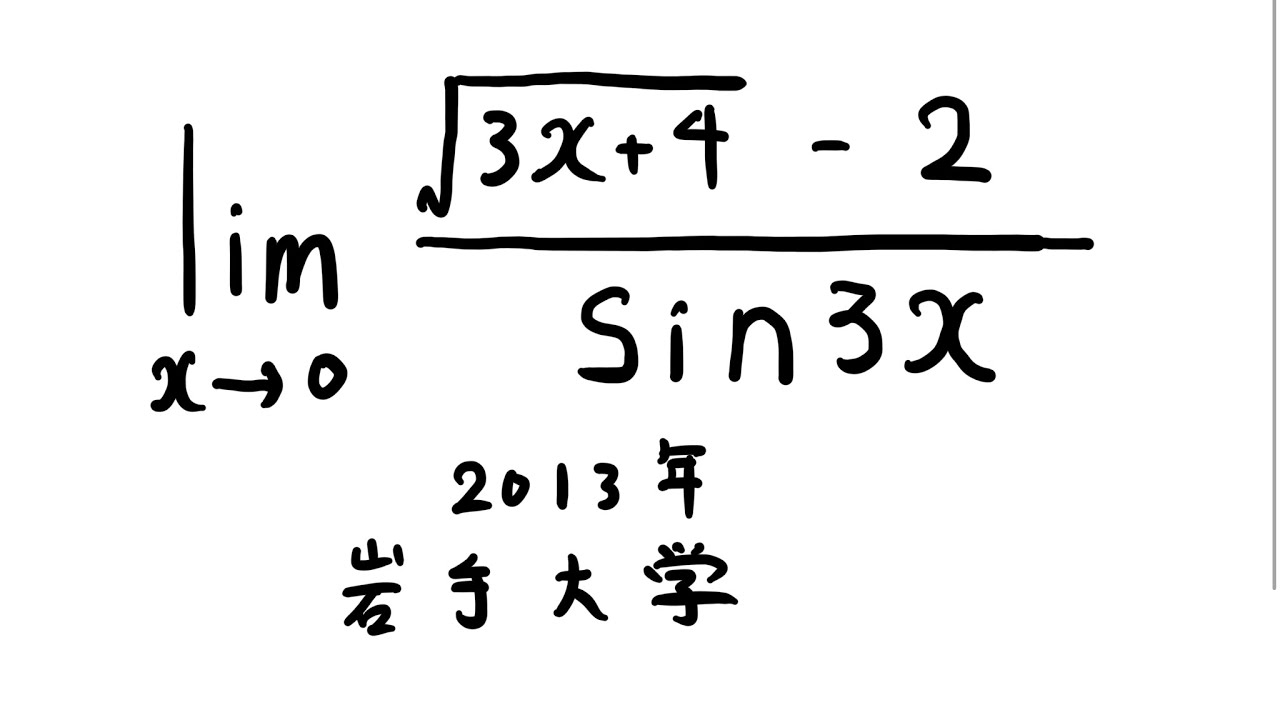
単元:
#大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{\sqrt{ 3x+4 }-2}{\sin3x}$
出典:2013年岩手大学
この動画を見る
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{\sqrt{ 3x+4 }-2}{\sin3x}$
出典:2013年岩手大学
福田のおもしろ数学143〜斜面の勾配

単元:
#数学(中学生)#中3数学#大学入試過去問(数学)#三平方の定理#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)
指導講師:
福田次郎
問題文全文(内容文):
傾いた平面上で、もっとも急な方向の勾配(傾き)が$\frac{1}{3}$であるという。いま南北方向の勾配を測ったところ$\frac{1}{5}$であった。
東西方向の勾配はどれだけか。
この動画を見る
傾いた平面上で、もっとも急な方向の勾配(傾き)が$\frac{1}{3}$であるという。いま南北方向の勾配を測ったところ$\frac{1}{5}$であった。
東西方向の勾配はどれだけか。
大学入試問題#824「たぶん良問」 #筑波大学(2022) #定積分
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#筑波大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
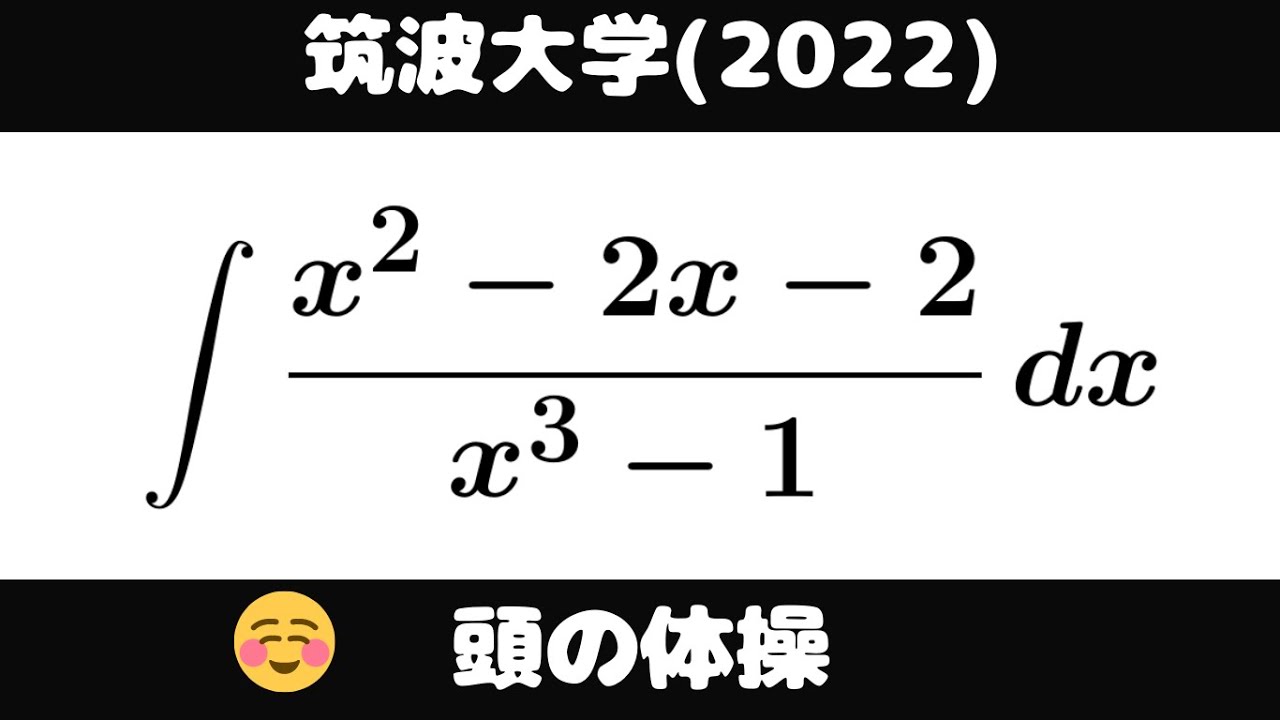
$\displaystyle \int \displaystyle \frac{x^2-2x-2}{x^3-1} dx$
出典:2022年筑波大学
この動画を見る
$\displaystyle \int \displaystyle \frac{x^2-2x-2}{x^3-1} dx$
出典:2022年筑波大学
福田の数学〜慶應義塾大学2024年商学部第2問(3)〜最小公倍数の変化と個数

単元:
#数A#大学入試過去問(数学)#整数の性質#約数・倍数・整数の割り算と余り・合同式#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)
指導講師:
福田次郎
問題文全文(内容文):
$\Large\boxed{2}$ (3)1から$n$までの$n$個の自然数の最小公倍数を$a_n$とする。
・$a_n$=$a_{n+1}$を満たす最小の自然数$n$は$\boxed{ケ}$である。
・$a_{n+1}$=$2a_n$を満たす10000以下の自然数$n$は$\boxed{コサ}$個ある。
この動画を見る
$\Large\boxed{2}$ (3)1から$n$までの$n$個の自然数の最小公倍数を$a_n$とする。
・$a_n$=$a_{n+1}$を満たす最小の自然数$n$は$\boxed{ケ}$である。
・$a_{n+1}$=$2a_n$を満たす10000以下の自然数$n$は$\boxed{コサ}$個ある。
#茨城大学(2023) #定積分 #Shorts
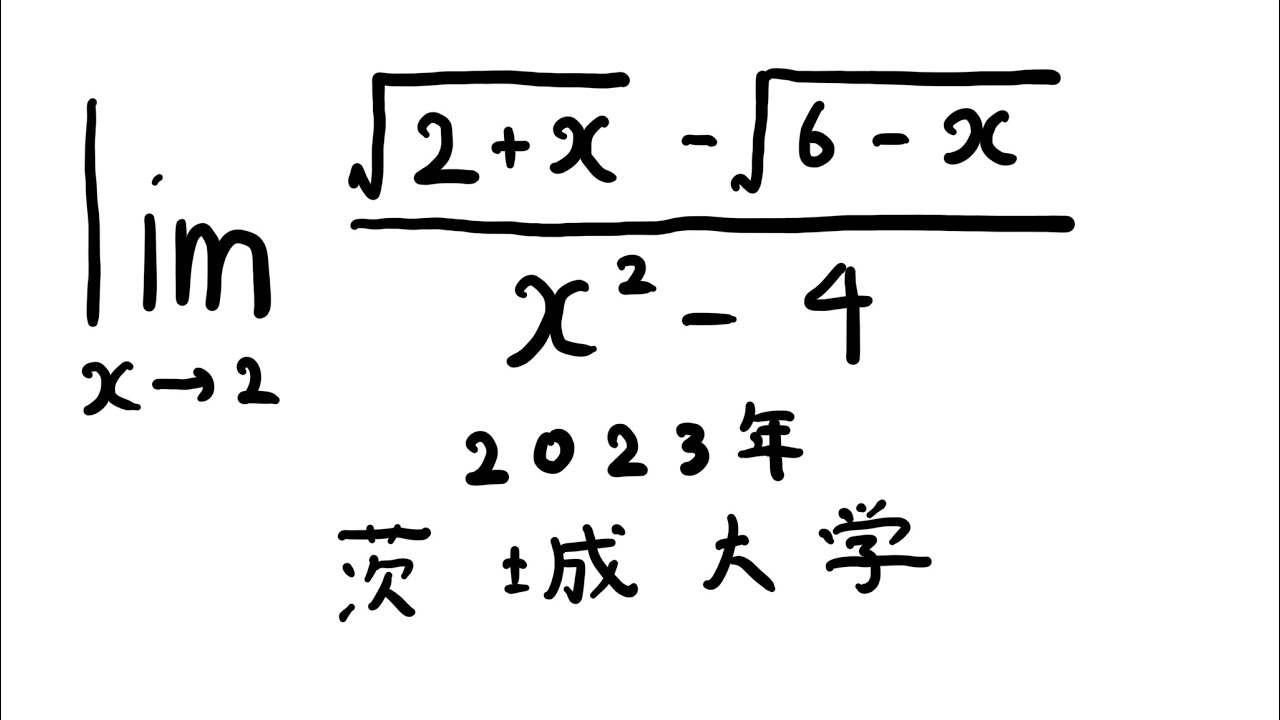
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ x \to 2 } \displaystyle \frac{\sqrt{ 2+x }-\sqrt{ 6-x }}{x^2-4}$
出典:2023年茨城大学
この動画を見る
$\displaystyle \lim_{ x \to 2 } \displaystyle \frac{\sqrt{ 2+x }-\sqrt{ 6-x }}{x^2-4}$
出典:2023年茨城大学
#茨城大学(2022) #定積分 #Shorts
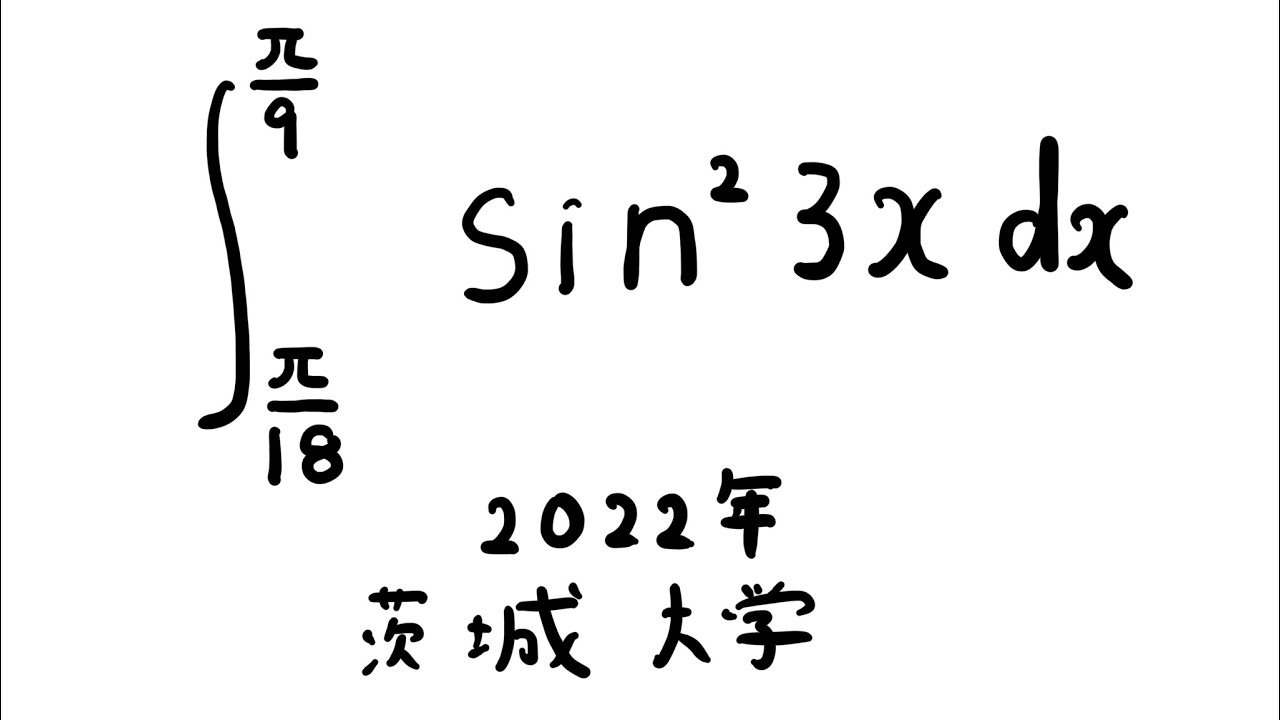
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{18}}^{\frac{\pi}{9}} \sin^23x\ dx$
出典:2022年茨城大学
この動画を見る
$\displaystyle \int_{\frac{\pi}{18}}^{\frac{\pi}{9}} \sin^23x\ dx$
出典:2022年茨城大学
大学入試問題#823「置換するかどうか」 #筑波大学(2019) #定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#筑波大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} (x+1)^2e-(x+1) dx$
出典:2019年筑波大学
この動画を見る
$\displaystyle \int_{0}^{1} (x+1)^2e-(x+1) dx$
出典:2019年筑波大学
福田の数学〜慶應義塾大学2024年商学部第2問(2)〜ベクトルの列とその絶対値の評価

単元:
#数Ⅱ#大学入試過去問(数学)#平面上のベクトル#指数関数と対数関数#対数関数#数列#平面上のベクトルと内積#漸化式#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)#数B#数C
指導講師:
福田次郎
問題文全文(内容文):
$\Large\boxed{2}$ (2)ベクトルの列 $\overrightarrow{a_1}$, $\overrightarrow{a_2}$, ..., $\overrightarrow{a_n}$, ...を条件
$\overrightarrow{a_1}$=(1,0), $\overrightarrow{a_2}$=$\left(\frac{1}{2}, \frac{\sqrt 3}{2}\right)$, $\overrightarrow{a_{n+2}}$=$\displaystyle\frac{\overrightarrow{a_{n+1}}・\overrightarrow{a_n}}{|\overrightarrow{a_n}|^2}\overrightarrow{a_n}$
で定める。このとき$\overrightarrow{a_9}$=$\left(\frac{\boxed{イ}}{\boxed{ウエオ}}, \boxed{カ}\right)$である。また、$|\overrightarrow{a_n}|$<$10^{-25}$を満たす最小の自然数$n$は$\boxed{キク}$である。ただし、必要であれば、$\log_{10}2$=0.301を近似として用いてよい。
この動画を見る
$\Large\boxed{2}$ (2)ベクトルの列 $\overrightarrow{a_1}$, $\overrightarrow{a_2}$, ..., $\overrightarrow{a_n}$, ...を条件
$\overrightarrow{a_1}$=(1,0), $\overrightarrow{a_2}$=$\left(\frac{1}{2}, \frac{\sqrt 3}{2}\right)$, $\overrightarrow{a_{n+2}}$=$\displaystyle\frac{\overrightarrow{a_{n+1}}・\overrightarrow{a_n}}{|\overrightarrow{a_n}|^2}\overrightarrow{a_n}$
で定める。このとき$\overrightarrow{a_9}$=$\left(\frac{\boxed{イ}}{\boxed{ウエオ}}, \boxed{カ}\right)$である。また、$|\overrightarrow{a_n}|$<$10^{-25}$を満たす最小の自然数$n$は$\boxed{キク}$である。ただし、必要であれば、$\log_{10}2$=0.301を近似として用いてよい。
#茨城大学(2022) #定積分 #Shorts
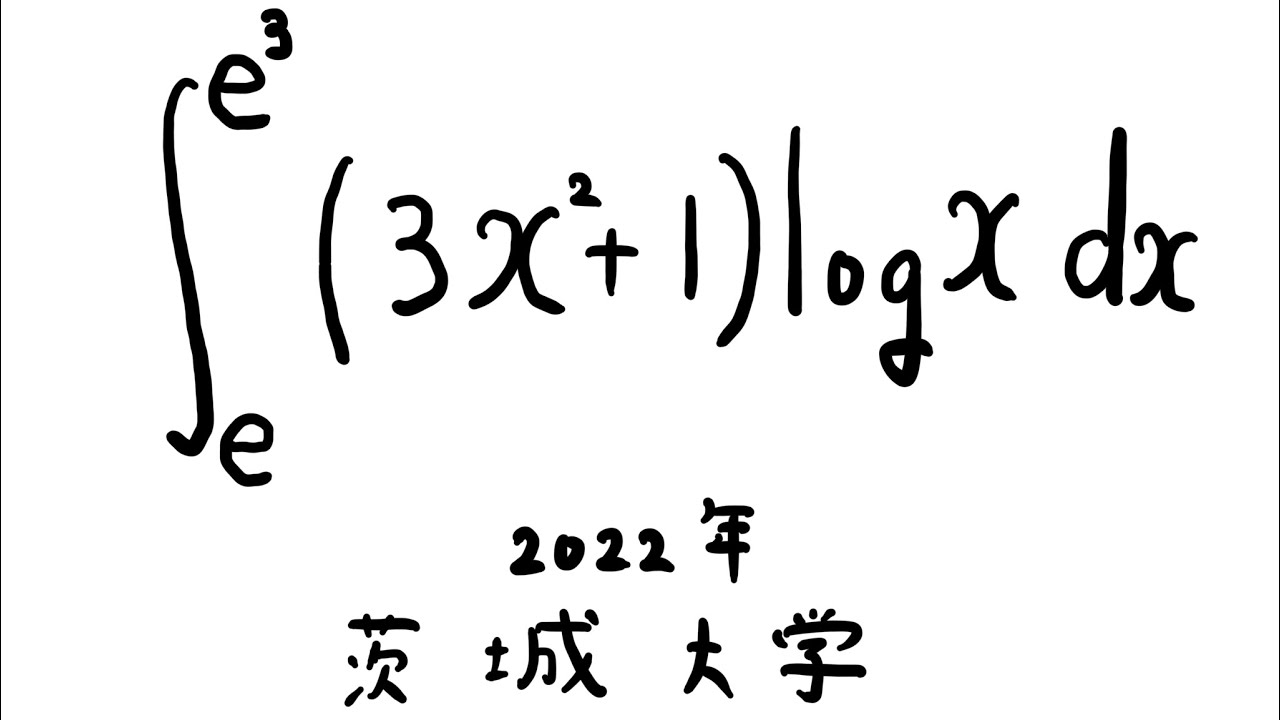
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{e}^{e^3} (3x^2+1)log\ x\ dx$
出典:2022年茨城大学
この動画を見る
$\displaystyle \int_{e}^{e^3} (3x^2+1)log\ x\ dx$
出典:2022年茨城大学
