 図形と計量
図形と計量
 図形と計量
図形と計量
COS36°を3通りで求めてね

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$\cos36^{ \circ }$を3通りで求めよ
この動画を見る
$\cos36^{ \circ }$を3通りで求めよ
成城大 ド・モアブル証明 6倍角の公式?

単元:
#数Ⅰ#数Ⅱ#大学入試過去問(数学)#式と証明#図形と計量#三角比(三角比・拡張・相互関係・単位円)#三角比への応用(正弦・余弦・面積)#恒等式・等式・不等式の証明#学校別大学入試過去問解説(数学)#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$z=\cos\theta+i\sin\theta$
(1)
$n$整数
$z^n=\cos n \theta + i \sin n \theta$を示せ
(2)
$z+\displaystyle \frac{1}{z}$を$\cos \theta$を用いて表せ
(3)
$\cos^6\theta$を$\cos2\theta,\cos4\theta,\cos6\theta$を用いて表せ
出典:2005年成城大学 過去問
この動画を見る
$z=\cos\theta+i\sin\theta$
(1)
$n$整数
$z^n=\cos n \theta + i \sin n \theta$を示せ
(2)
$z+\displaystyle \frac{1}{z}$を$\cos \theta$を用いて表せ
(3)
$\cos^6\theta$を$\cos2\theta,\cos4\theta,\cos6\theta$を用いて表せ
出典:2005年成城大学 過去問
Mr 東北大 1浪1留院試落ち 人生各駅停車 さがらごうち

単元:
#数Ⅰ#大学入試過去問(数学)#図形と計量#三角比への応用(正弦・余弦・面積)#センター試験・共通テスト関連#センター試験#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
動画内の図を参照して求めよ
(1)
$AP$
(2)
$OD$
(3)
$\cos \angle OAD$
(4)
$AC$
(5)
$\triangle ABC$
この動画を見る
動画内の図を参照して求めよ
(1)
$AP$
(2)
$OD$
(3)
$\cos \angle OAD$
(4)
$AC$
(5)
$\triangle ABC$
Prove π is larger than 3.05 ~Tokyo University Entrance Examination~

単元:
#数Ⅰ#大学入試過去問(数学)#数と式#図形と計量#三角比への応用(正弦・余弦・面積)#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$\pi$が3.05より大きいことを証明せよ
出典:東京大学 入試問題
この動画を見る
$\pi$が3.05より大きいことを証明せよ
出典:東京大学 入試問題
東大卒のもっちゃんと数学Vol.7 加法定理を証明しよう(東大過去問)

単元:
#数Ⅱ#大学入試過去問(数学)#図形と計量#三角比(三角比・拡張・相互関係・単位円)#三角比への応用(正弦・余弦・面積)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
加法定理を証明 解説動画です
$\cos (\alpha+\beta)=\cos \alpha \cos\beta -\sin \alpha \sin\beta$
この動画を見る
加法定理を証明 解説動画です
$\cos (\alpha+\beta)=\cos \alpha \cos\beta -\sin \alpha \sin\beta$
数弱私文の早大生バンカラジオにヨビノリたくみが「優しく」三角関数の基本を教えるよ。余弦定理

【数Ⅰ】図形と計量:単位円と三角比の関係

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
cos90°はなぜ0?鈍角でなぜマイナスに?単位円を使って分かりやすく教えます!
この動画を見る
cos90°はなぜ0?鈍角でなぜマイナスに?単位円を使って分かりやすく教えます!
東北大 三角方程式 Mathematics Japanese university entrance exam

単元:
#数Ⅰ#数Ⅱ#大学入試過去問(数学)#図形と計量#三角比(三角比・拡張・相互関係・単位円)#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$0 \leqq x \lt 2\pi$方程式を解け
(1)
$\sin^3x+\cos^3x=1$
(2)
$\sin^3x+\cos^3x+\sin x=2$
出典:2007年東北大学 過去問
この動画を見る
$0 \leqq x \lt 2\pi$方程式を解け
(1)
$\sin^3x+\cos^3x=1$
(2)
$\sin^3x+\cos^3x+\sin x=2$
出典:2007年東北大学 過去問
東大卒もっちゃんと数学 余弦定理 Mathematics Japanese university entrance exam

【高校数学】三角比4.5~例題・三角比といえばこれ・基礎~ 3-4.5【数学Ⅰ】

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
【楽しい授業動画】あきとんとん
問題文全文(内容文):
(1) 0°≦$\theta$≦180°のとき、sin$\theta$=$\frac{ \sqrt{3} }{ 2 }$を満たす$\theta$を求めよ。
(2) 0°≦$\theta$≦180°のとき、cos$\theta$=-$\frac{ 1 }{ \sqrt{2} }$を満たす$\theta$を求めよ。
(3) 0°≦$\theta$≦180°のとき、tan$\theta$=-$\sqrt{3}$を満たす$\theta$を求めよ。
(4) 0°≦$\theta$≦180°のとする。sin$\theta$=$\displaystyle \frac{3}{5}$のとき、cos$\theta$とtan$\theta$の値を求めよ。
(5) 直線y=$\sqrt{3}$xとx軸の正の向きとのなす角$\theta$を求めよ。
この動画を見る
(1) 0°≦$\theta$≦180°のとき、sin$\theta$=$\frac{ \sqrt{3} }{ 2 }$を満たす$\theta$を求めよ。
(2) 0°≦$\theta$≦180°のとき、cos$\theta$=-$\frac{ 1 }{ \sqrt{2} }$を満たす$\theta$を求めよ。
(3) 0°≦$\theta$≦180°のとき、tan$\theta$=-$\sqrt{3}$を満たす$\theta$を求めよ。
(4) 0°≦$\theta$≦180°のとする。sin$\theta$=$\displaystyle \frac{3}{5}$のとき、cos$\theta$とtan$\theta$の値を求めよ。
(5) 直線y=$\sqrt{3}$xとx軸の正の向きとのなす角$\theta$を求めよ。
東大 三角比 放物線 Mathematics Japanese university entrance exam Tokyo University

単元:
#数Ⅰ#数Ⅱ#大学入試過去問(数学)#2次関数#図形と計量#2次関数とグラフ#三角比(三角比・拡張・相互関係・単位円)#三角比への応用(正弦・余弦・面積)#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$y=2 \sqrt{ 3 }(x- \cos \theta)^2+ \sin \theta$
$y=-2 \sqrt{ 3 }(x+ \cos \theta)^2- \sin \theta$
この2つの放物線が相違となる2点で交わるような$\theta$の範囲
出典:2002年東京大学 過去問
この動画を見る
$y=2 \sqrt{ 3 }(x- \cos \theta)^2+ \sin \theta$
$y=-2 \sqrt{ 3 }(x+ \cos \theta)^2- \sin \theta$
この2つの放物線が相違となる2点で交わるような$\theta$の範囲
出典:2002年東京大学 過去問
【高校数学】三角比④~90°- θ,180° - θ考え方,イメージ~ 3-4【数学Ⅰ】

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
【楽しい授業動画】あきとんとん
問題文全文(内容文):
△ABCの3つの内角$\angle A$、$\angle B$、$\angle C$の大きさをそれぞれA、B、Cとするとき、
次の等式が成り立つことを証明せよ。
sin$\displaystyle \frac{A}{2}$=cos$\displaystyle \frac{B+C}{2}$
この動画を見る
△ABCの3つの内角$\angle A$、$\angle B$、$\angle C$の大きさをそれぞれA、B、Cとするとき、
次の等式が成り立つことを証明せよ。
sin$\displaystyle \frac{A}{2}$=cos$\displaystyle \frac{B+C}{2}$
【高校数学】三角比③~三角比の大切なイメージ~ 3-3【数学Ⅰ】

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
【楽しい授業動画】あきとんとん
問題文全文(内容文):
三角比 三角比の大切なイメージについての説明動画です
この動画を見る
三角比 三角比の大切なイメージについての説明動画です
【高校数学】三角比②~三角比の重要な公式~ 3-2【数学Ⅰ】

【高校数学】三角比①~三角比とは横顔??~ 3-1【数学Ⅰ】

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
【楽しい授業動画】あきとんとん
問題文全文(内容文):
木の根もとから水平に10m離れた地点で木の先端の仰角を測ったところ、
28°であった。
目の高さを1.6mとして、木の高さを求めよ。
この動画を見る
木の根もとから水平に10m離れた地点で木の先端の仰角を測ったところ、
28°であった。
目の高さを1.6mとして、木の高さを求めよ。
名古屋大 円の方程式 2円と直線に接する円 高校数学 Japanese university entrance exam questions

単元:
#数Ⅰ#数A#大学入試過去問(数学)#図形の性質#図形と計量#三角比(三角比・拡張・相互関係・単位円)#周角と円に内接する四角形・円と接線・接弦定理#数学(高校生)#名古屋大学
指導講師:
鈴木貫太郎
問題文全文(内容文):
'08名古屋大学過去問題
2つの円、$x^2+(y-2)^2=9$と$(x-4)^2+(y+4)^2=1$に外接し、x=6と接する円を求めよ。
この動画を見る
'08名古屋大学過去問題
2つの円、$x^2+(y-2)^2=9$と$(x-4)^2+(y+4)^2=1$に外接し、x=6と接する円を求めよ。
和歌山大 三項間漸化式 半角の公式 高校数学 Japanese university entrance exam questions
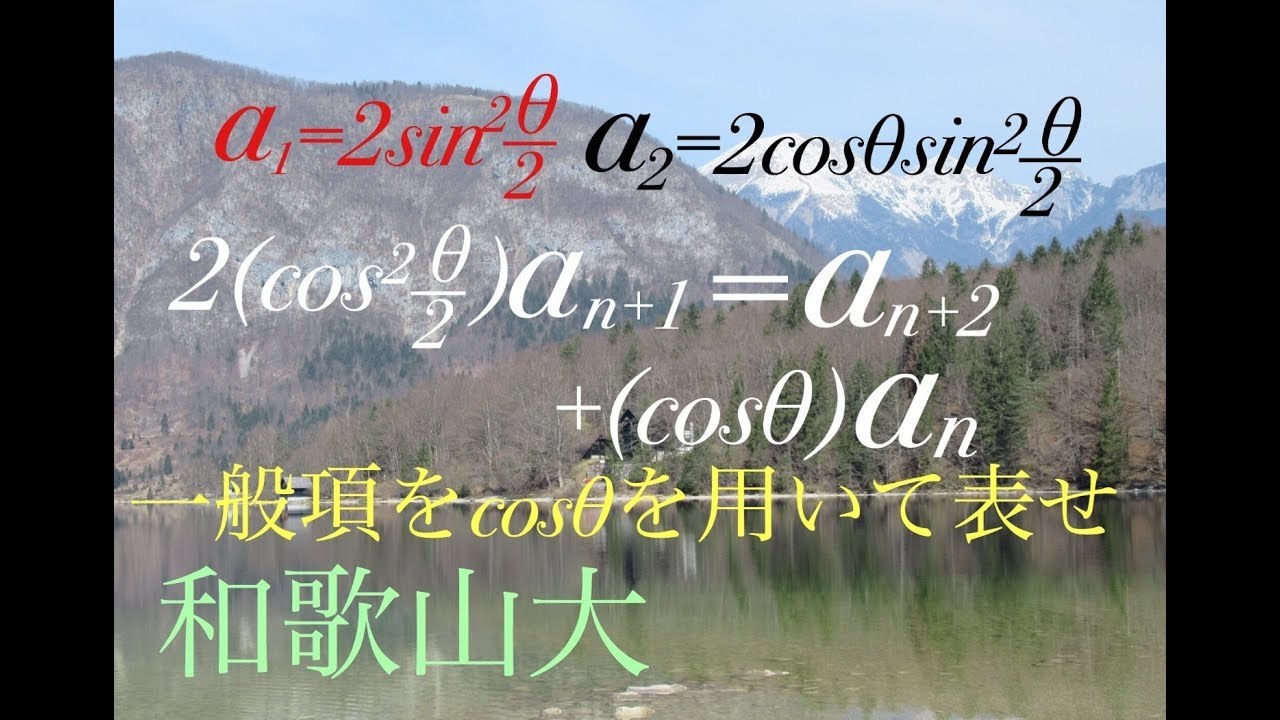
単元:
#数Ⅰ#数Ⅱ#大学入試過去問(数学)#図形と計量#三角比(三角比・拡張・相互関係・単位円)#三角比への応用(正弦・余弦・面積)#三角関数#三角関数とグラフ#数列#数列とその和(等差・等比・階差・Σ)#数学(高校生)#和歌山大学#数B
指導講師:
鈴木貫太郎
問題文全文(内容文):
和歌山大学過去問題
$a_1=2\sin^2\frac{θ}{2}$,$a_2=2\cosθ\sin^2\frac{θ}{2}$
$2(cos^2\frac{θ}{2})a_{n+1}=a_{n+2}+(\cosθ)a_n$
$a_n$を$\cosθ$を用いて表せ。
この動画を見る
和歌山大学過去問題
$a_1=2\sin^2\frac{θ}{2}$,$a_2=2\cosθ\sin^2\frac{θ}{2}$
$2(cos^2\frac{θ}{2})a_{n+1}=a_{n+2}+(\cosθ)a_n$
$a_n$を$\cosθ$を用いて表せ。
【高校数学】 数Ⅰ-95 多角形の面積
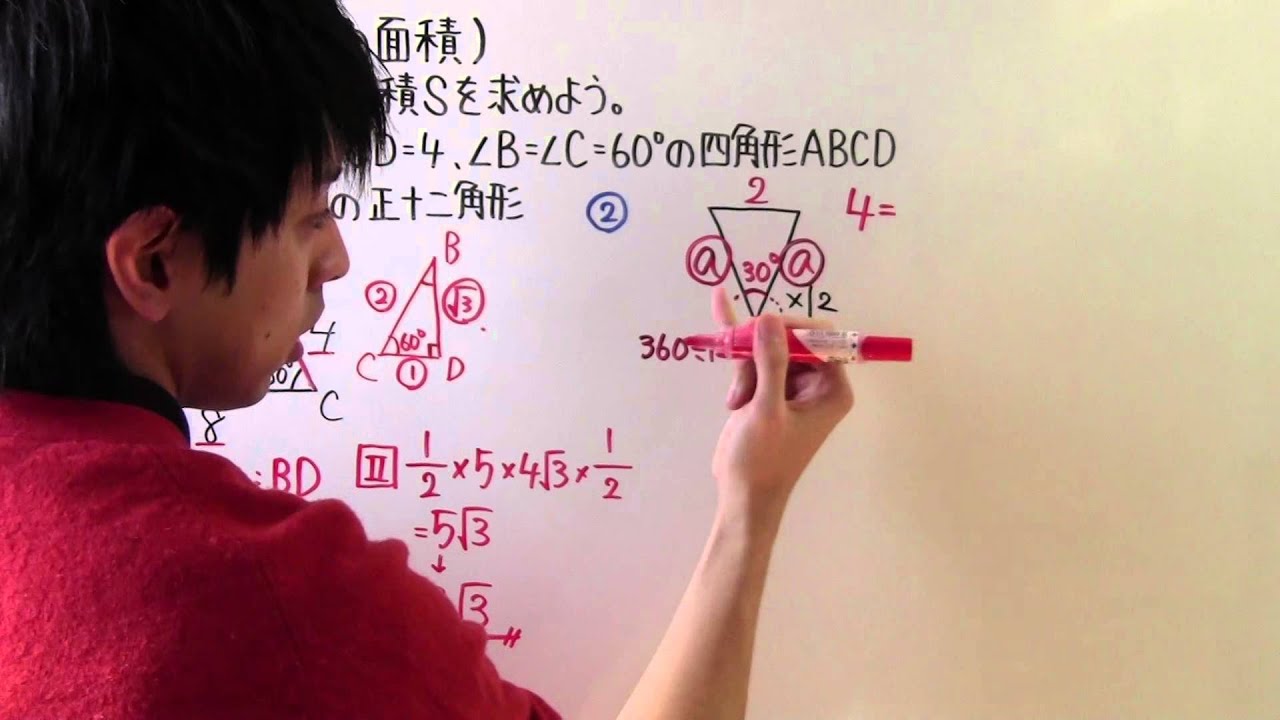
単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎次のような図形の面積Sを求めよう。
①$AB=5,BC=8,CD=4,\angle B=\angle C=60°$の四角形ABCD
②1辺の長さが2の正十二角形
この動画を見る
◎次のような図形の面積Sを求めよう。
①$AB=5,BC=8,CD=4,\angle B=\angle C=60°$の四角形ABCD
②1辺の長さが2の正十二角形
【高校数学】 数Ⅰ-94 三角形の面積② ・ ヘロンの公式編

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
3辺の長さがa,b,cである△ABCの面積Sは、
S=①____________(t=②____________)
◎次のような△ABCの面積を求めよう。
③a=8,b=6,C=4
④a=7,b=5,C=9
この動画を見る
3辺の長さがa,b,cである△ABCの面積Sは、
S=①____________(t=②____________)
◎次のような△ABCの面積を求めよう。
③a=8,b=6,C=4
④a=7,b=5,C=9
【高校数学】 数Ⅰ-93 三角形の面積① ・ 基本編

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
三角形の面積S=①__________________
△ABCの内接円の半径rとするとS=②____________
※図は動画内参照
◎次の△ABCの面積Sを求めよう。
③$b=3,C=2,A=120°$
④$a=2\sqrt{ 2 },b=3,A110°,B=25°$
⑤$a=6,b=3,c=7$
この動画を見る
三角形の面積S=①__________________
△ABCの内接円の半径rとするとS=②____________
※図は動画内参照
◎次の△ABCの面積Sを求めよう。
③$b=3,C=2,A=120°$
④$a=2\sqrt{ 2 },b=3,A110°,B=25°$
⑤$a=6,b=3,c=7$
【高校数学】 数Ⅰ-92 三角形となる条件

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎3辺の長さが、5,3,xである三角形が鈍角三角形となるように、xの範囲を定めよう。
この動画を見る
◎3辺の長さが、5,3,xである三角形が鈍角三角形となるように、xの範囲を定めよう。
【高校数学】 数Ⅰ-91 正弦定理と余弦定理④

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎△ABCの辺BCの中点をM、線分BMの中点をDとする。
a=8,b=4,C=6のとき、次のものを求めよう。
①$\cos B$の値
②$AM$の長さ
③$AD$の長さ
※図は動画内参照
この動画を見る
◎△ABCの辺BCの中点をM、線分BMの中点をDとする。
a=8,b=4,C=6のとき、次のものを求めよう。
①$\cos B$の値
②$AM$の長さ
③$AD$の長さ
※図は動画内参照
【高校数学】 数Ⅰ-90 正弦定理と余弦定理③

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎△ABCにおいて、次が成り立つとき、この三角形の最も大きい角の余弦の値を求めよう。
①$\displaystyle \frac{a}{13}=\displaystyle \frac{b}{8}=\displaystyle \frac{c}{7}$
②$\sin A:\sin B:\sin C=5:4:6$
この動画を見る
◎△ABCにおいて、次が成り立つとき、この三角形の最も大きい角の余弦の値を求めよう。
①$\displaystyle \frac{a}{13}=\displaystyle \frac{b}{8}=\displaystyle \frac{c}{7}$
②$\sin A:\sin B:\sin C=5:4:6$
【高校数学】 数Ⅰ-89 正弦定理と余弦定理②

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎△ABCにおいて、$a=2,b=\sqrt{ 6 },A=45°$のとき、
残りの底辺の長さと角の大きさを求めよう。
この動画を見る
◎△ABCにおいて、$a=2,b=\sqrt{ 6 },A=45°$のとき、
残りの底辺の長さと角の大きさを求めよう。
【高校数学】 数Ⅰ-88 正弦定理と余弦定理①

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎△ABCにおいて、次のものを求めよ。
①$B=60°,C=75°,b=2\sqrt{ 6 }$のとき$a$
②$a=4,b=\sqrt{ 21 },C=5$のとき$B$
③$b=60°,a:b=1:3$のとき$\sin A$
この動画を見る
◎△ABCにおいて、次のものを求めよ。
①$B=60°,C=75°,b=2\sqrt{ 6 }$のとき$a$
②$a=4,b=\sqrt{ 21 },C=5$のとき$B$
③$b=60°,a:b=1:3$のとき$\sin A$
【高校数学】 数Ⅰ-87 余弦定理

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
△ABCについて
①$a^2=$____
②$b^2=$____
③$c^2=$____
④$\cos A=$____
⑤$\cos B=$____
⑥$\cos C=$____
※図は動画内参照
◎△ABCにおいて、次のものを求めよう。
⑦$a=3,b=\sqrt{ 2 },C=45°$のとき $c$
⑧$b=7,c=5,B=60°$のとき$a$
この動画を見る
△ABCについて
①$a^2=$____
②$b^2=$____
③$c^2=$____
④$\cos A=$____
⑤$\cos B=$____
⑥$\cos C=$____
※図は動画内参照
◎△ABCにおいて、次のものを求めよう。
⑦$a=3,b=\sqrt{ 2 },C=45°$のとき $c$
⑧$b=7,c=5,B=60°$のとき$a$
【高校数学】 数Ⅰ-86 正弦定理

単元:
#数Ⅰ#図形と計量#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
△ABCの外接円の半径をRとすると
①____=②____=③____=2R
◎△ABCにおいて、外接円の半径をRとするとき、次のものを求めよう。
④B=120°,R=4のとき b
⑤a=5$\sqrt{ 3 }$,R=5のとき A
⑥A=60°,C=75°,a=$2\sqrt{ 6 }$のとき Rとb
※図は動画内参照
この動画を見る
△ABCの外接円の半径をRとすると
①____=②____=③____=2R
◎△ABCにおいて、外接円の半径をRとするとき、次のものを求めよう。
④B=120°,R=4のとき b
⑤a=5$\sqrt{ 3 }$,R=5のとき A
⑥A=60°,C=75°,a=$2\sqrt{ 6 }$のとき Rとb
※図は動画内参照
【高校数学】 数Ⅰ-85 三角比⑩
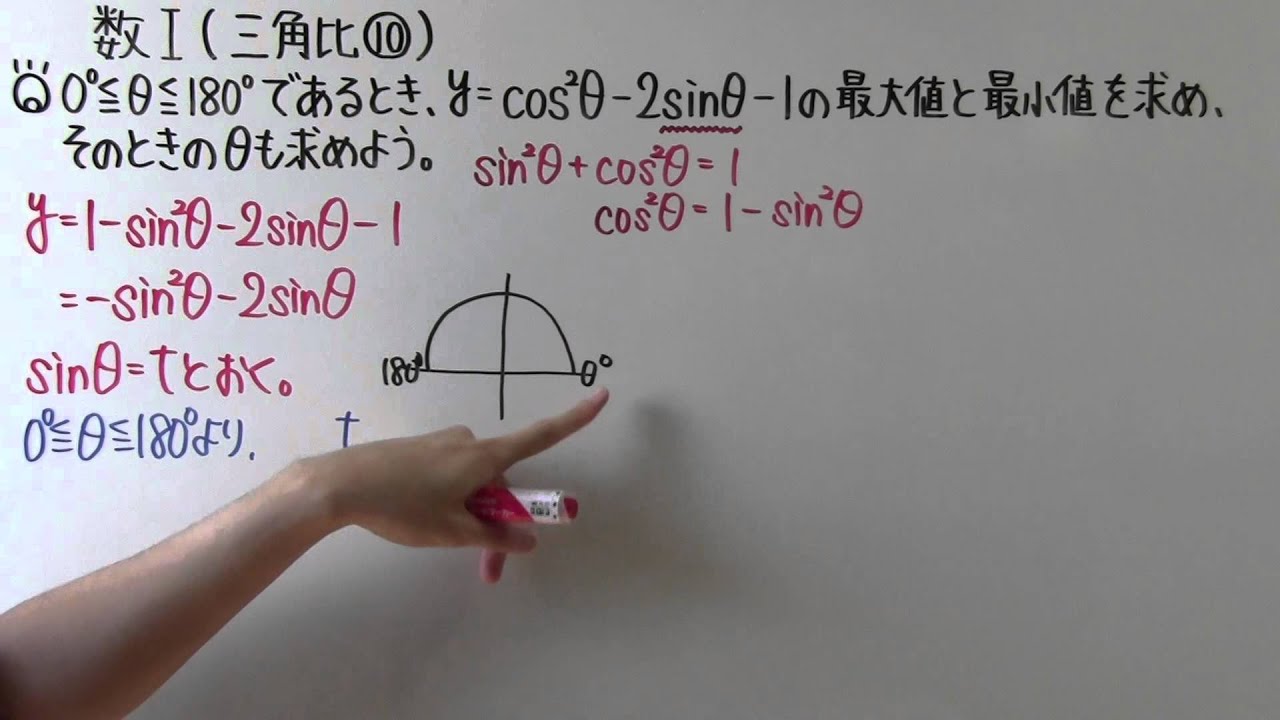
単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
$0° \leqq \theta \leqq 180°$であるとき、$y=\cos^2\theta-2\sin\theta-1$の最大値と最小値を求め、そのときの$\theta$も求めよう。
この動画を見る
$0° \leqq \theta \leqq 180°$であるとき、$y=\cos^2\theta-2\sin\theta-1$の最大値と最小値を求め、そのときの$\theta$も求めよう。
【高校数学】 数Ⅰ-84 三角比⑨

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#三角比への応用(正弦・余弦・面積)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
$0° \leqq \theta \leqq 180°$とする。次の不等式を満たす
$\theta $の範囲を求めよう。
①$\sin \theta \gt \displaystyle \frac{\sqrt{ 3 }}{2}$
②$\cos \theta \lt \displaystyle \frac{1}{2}$
③$\tan \theta \geqq \sqrt{ 3 }$
④$2\sin \theta-1\leqq0$
⑤$2\cos \theta+ \sqrt{ 3 } \gt 0$
⑥$\tan \theta +1 \geqq 0$
この動画を見る
$0° \leqq \theta \leqq 180°$とする。次の不等式を満たす
$\theta $の範囲を求めよう。
①$\sin \theta \gt \displaystyle \frac{\sqrt{ 3 }}{2}$
②$\cos \theta \lt \displaystyle \frac{1}{2}$
③$\tan \theta \geqq \sqrt{ 3 }$
④$2\sin \theta-1\leqq0$
⑤$2\cos \theta+ \sqrt{ 3 } \gt 0$
⑥$\tan \theta +1 \geqq 0$
【高校数学】 数Ⅰ-83 三角比⑧

単元:
#数Ⅰ#図形と計量#三角比(三角比・拡張・相互関係・単位円)#数学(高校生)
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
◎$0° \leqq \theta \leqq 180°,\sin \theta+\cos \theta=\displaystyle \frac{1}{2}$のとき、次の式の値を求めよう。
①$\sin \theta\cos \theta$
②$\sin^3 \theta+\cos^3 \theta$
③$\sin \theta-\cos \theta$
この動画を見る
◎$0° \leqq \theta \leqq 180°,\sin \theta+\cos \theta=\displaystyle \frac{1}{2}$のとき、次の式の値を求めよう。
①$\sin \theta\cos \theta$
②$\sin^3 \theta+\cos^3 \theta$
③$\sin \theta-\cos \theta$
