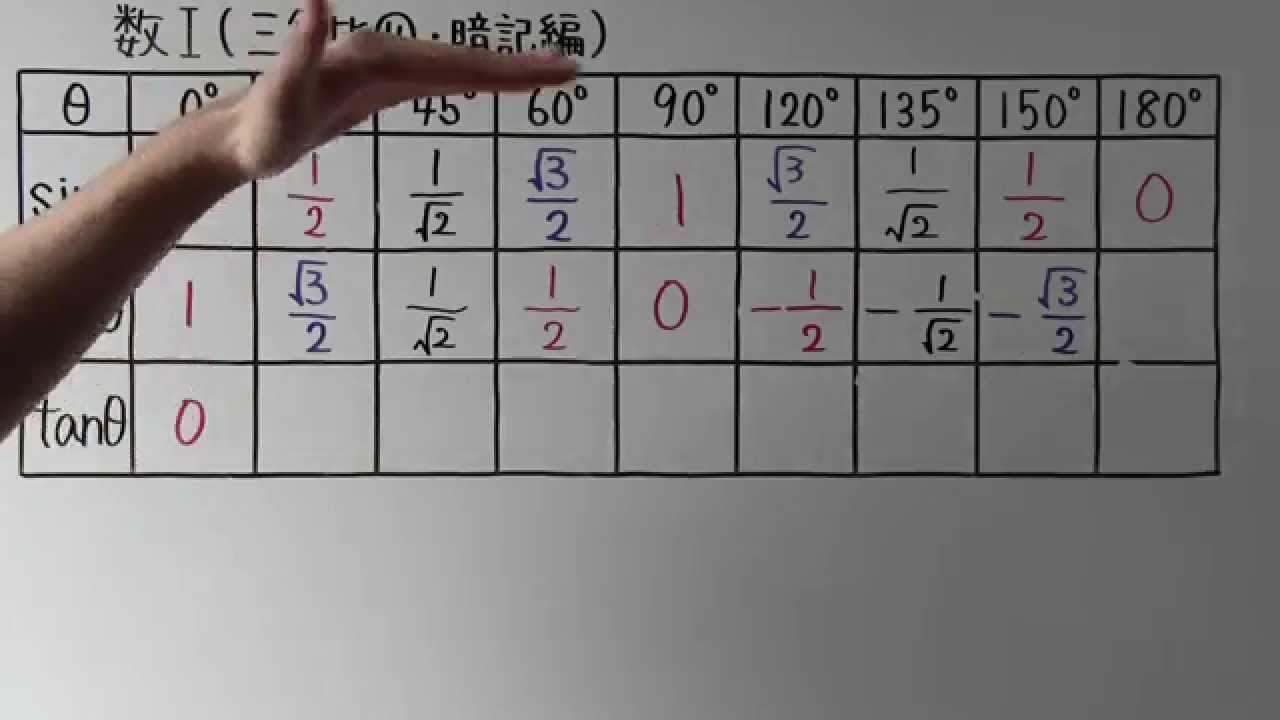
図形と計量

東大卒のもっちゃんと数学Vol.7 加法定理を証明しよう（東大過去問）

単元： #数Ⅱ#大学入試過去問(数学)#図形と計量#三角比（三角比・拡張・相互関係・単位円）#三角比への応用（正弦・余弦・面積）#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：
加法定理を証明　解説動画です

\cos (α + β) = \cos α \cos β - \sin α \sin β

この動画を見る　

数弱私文の早大生バンカラジオにヨビノリたくみが「優しく」三角関数の基本を教えるよ。余弦定理

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）

指導講師：鈴木貫太郎

問題文全文(内容文)：
三角関数の基本解説動画です

この動画を見る　

【数Ⅰ】図形と計量：単位円と三角比の関係

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：理数個別チャンネル

問題文全文(内容文)：
cos90°はなぜ0？鈍角でなぜマイナスに？単位円を使って分かりやすく教えます！

この動画を見る　

東北大　三角方程式 Mathematics Japanese university entrance exam

単元： #数Ⅰ#数Ⅱ#大学入試過去問(数学)#図形と計量#三角比（三角比・拡張・相互関係・単位円）#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：

0 ≦ x < 2 π

方程式を解け

（1）

\sin^{3} x + \cos^{3} x = 1

（2）

\sin^{3} x + \cos^{3} x + \sin x = 2

出典：2007年東北大学　過去問

この動画を見る　

東大卒もっちゃんと数学　余弦定理 Mathematics Japanese university entrance exam

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：
余弦定理の解説動画です

この動画を見る　

【高校数学】三角比4.5～例題・三角比といえばこれ・基礎～ 3-4.5【数学Ⅰ】

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
(1) 0°≦

θ

≦180°のとき、sin

θ

=

\frac{\sqrt{3}}{2}

を満たす

θ

を求めよ。

(2) 0°≦

θ

≦180°のとき、cos

θ

=-

\frac{1}{\sqrt{2}}

を満たす

θ

を求めよ。

(3) 0°≦

θ

≦180°のとき、tan

θ

=-

\sqrt{3}

を満たす

θ

を求めよ。

(4) 0°≦

θ

≦180°のとする。sin

θ

=

\frac{3}{5}

のとき、cos

θ

とtan

θ

の値を求めよ。

(5) 直線y=

\sqrt{3}

xとx軸の正の向きとのなす角

θ

を求めよ。

この動画を見る　

東大　三角比　放物線 Mathematics Japanese university entrance exam Tokyo University

単元： #数Ⅰ#数Ⅱ#大学入試過去問(数学)#２次関数#図形と計量#2次関数とグラフ#三角比（三角比・拡張・相互関係・単位円）#三角比への応用（正弦・余弦・面積）#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：

y = 2 \sqrt{3} (x - \cos θ)^{2} + \sin θ

y = - 2 \sqrt{3} (x + \cos θ)^{2} - \sin θ

この2つの放物線が相違となる2点で交わるような

θ

の範囲

出典：2002年東京大学　過去問

この動画を見る　

【高校数学】三角比④～90°- θ，180° - θ考え方，イメージ～ 3-4【数学Ⅰ】

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
△ABCの3つの内角

∠ A

、

∠ B

、

∠ C

の大きさをそれぞれA、B、Cとするとき、
次の等式が成り立つことを証明せよ。

sin

\frac{A}{2}

=cos

\frac{B + C}{2}

この動画を見る　

【高校数学】三角比③～三角比の大切なイメージ～ 3-3【数学Ⅰ】

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
三角比　三角比の大切なイメージについての説明動画です

この動画を見る　

【高校数学】三角比②～三角比の重要な公式～ 3-2【数学Ⅰ】

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
三角比の重要な公式説明動画です

この動画を見る　

【高校数学】三角比①～三角比とは横顔？？～ 3-1【数学Ⅰ】

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
木の根もとから水平に10m離れた地点で木の先端の仰角を測ったところ、
28°であった。
目の高さを1.6mとして、木の高さを求めよ。

この動画を見る　

名古屋大　円の方程式　２円と直線に接する円　高校数学 Japanese university entrance exam questions

単元： #数Ⅰ#数A#大学入試過去問(数学)#図形の性質#図形と計量#三角比（三角比・拡張・相互関係・単位円）#周角と円に内接する四角形・円と接線・接弦定理#数学(高校生)#名古屋大学

指導講師：鈴木貫太郎

問題文全文(内容文)：
'08名古屋大学過去問題
2つの円、

x^{2} + (y - 2)^{2} = 9

と

(x - 4)^{2} + (y + 4)^{2} = 1

に外接し、x=6と接する円を求めよ。

この動画を見る　

和歌山大　三項間漸化式　半角の公式　高校数学 Japanese university entrance exam questions

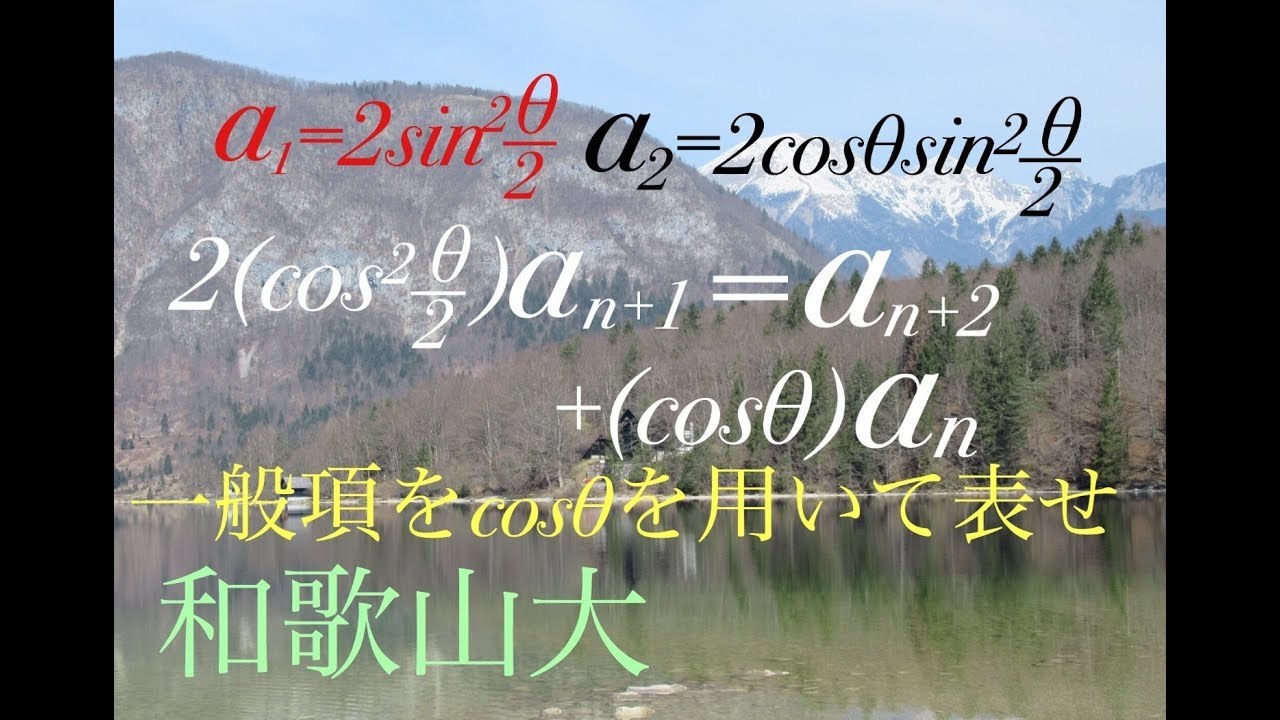
単元： #数Ⅰ#数Ⅱ#大学入試過去問(数学)#図形と計量#三角比（三角比・拡張・相互関係・単位円）#三角比への応用（正弦・余弦・面積）#三角関数#三角関数とグラフ#数列#数列とその和（等差・等比・階差・Σ）#数学(高校生)#和歌山大学#数B

指導講師：鈴木貫太郎

問題文全文(内容文)：
和歌山大学過去問題

a_{1} = 2 \sin^{2} \frac{θ}{2}

,

a_{2} = 2 \cos θ \sin^{2} \frac{θ}{2}

2 (c o s^{2} \frac{θ}{2}) a_{n + 1} = a_{n + 2} + (\cos θ) a_{n}

a_{n}

を

\cos θ

を用いて表せ。

この動画を見る　

【高校数学】　　数Ⅰ－９５　　多角形の面積

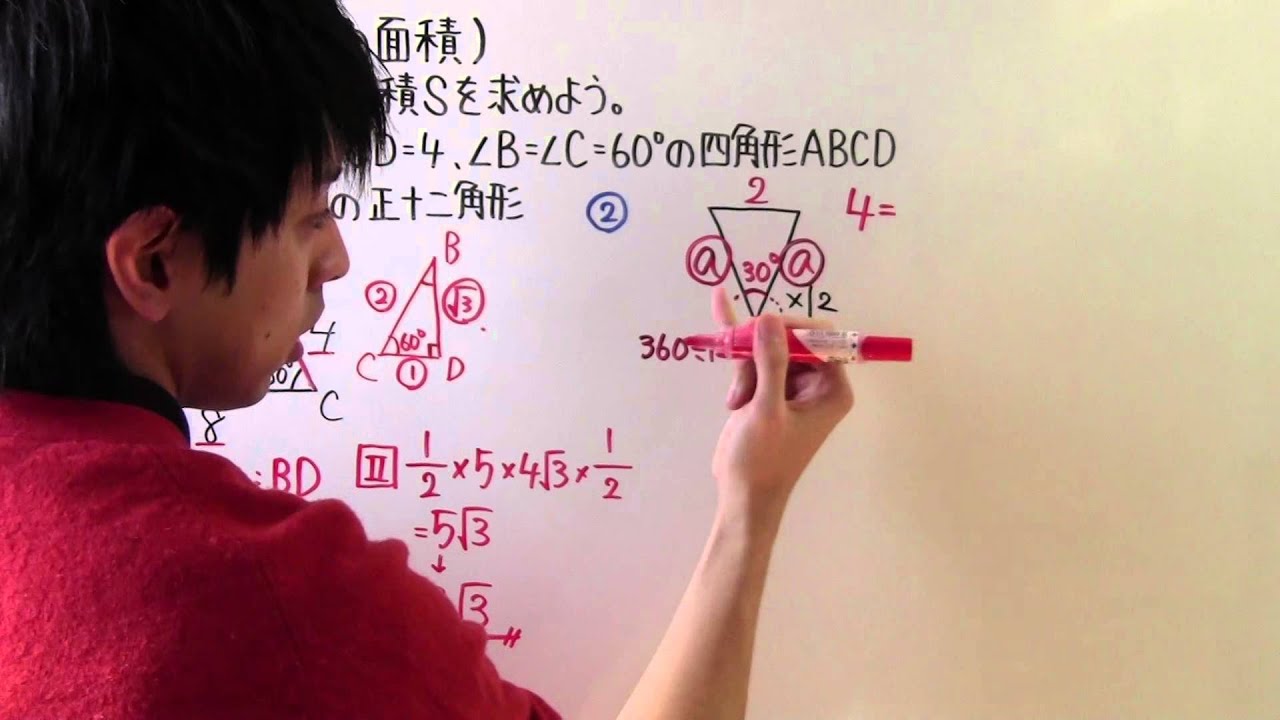
単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎次のような図形の面積Sを求めよう。
①

A B = 5, B C = 8, C D = 4, ∠ B = ∠ C = 60 °

の四角形ABCD
②1辺の長さが2の正十二角形

この動画を見る　

【高校数学】　　数Ⅰ－９４　　三角形の面積②　・　ヘロンの公式編

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
3辺の長さがa,b,cである△ABCの面積Sは、
S=①＿＿＿＿＿＿＿＿＿＿＿＿(t=②＿＿＿＿＿＿＿＿＿＿＿＿)

◎次のような△ABCの面積を求めよう。

③a=8,b=6,C=4

④a=7,b=5,C=9

この動画を見る　

【高校数学】　　数Ⅰ－９３　　三角形の面積①　・　基本編

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
三角形の面積S=①＿＿＿＿＿＿＿＿＿＿＿＿＿＿＿＿＿＿
△ABCの内接円の半径rとするとS＝②＿＿＿＿＿＿＿＿＿＿＿＿
※図は動画内参照

◎次の△ABCの面積Sを求めよう。

③

b = 3, C = 2, A = 120 °

④

a = 2 \sqrt{2}, b = 3, A 110 °, B = 25 °

⑤

a = 6, b = 3, c = 7

この動画を見る　

【高校数学】　　数Ⅰ－９２　　三角形となる条件

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎3辺の長さが、5,3,xである三角形が鈍角三角形となるように、xの範囲を定めよう。

この動画を見る　

【高校数学】　　数Ⅰ－９１　　正弦定理と余弦定理④

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎△ABCの辺BCの中点をM、線分BMの中点をDとする。
a=8,b=4,C=6のとき、次のものを求めよう。

①

\cos B

の値
②

A M

の長さ
③

A D

の長さ
※図は動画内参照

この動画を見る　

【高校数学】　　数Ⅰ－９０　　正弦定理と余弦定理③

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎△ABCにおいて、次が成り立つとき、この三角形の最も大きい角の余弦の値を求めよう。

①

\frac{a}{13} = \frac{b}{8} = \frac{c}{7}

②

\sin A : \sin B : \sin C = 5 : 4 : 6

この動画を見る　

【高校数学】　　数Ⅰ－８９　　正弦定理と余弦定理②

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎△ABCにおいて、

a = 2, b = \sqrt{6}, A = 45 °

のとき、
残りの底辺の長さと角の大きさを求めよう。

この動画を見る　

【高校数学】　　数Ⅰ－８８　　正弦定理と余弦定理①

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎△ABCにおいて、次のものを求めよ。
①

B = 60 °, C = 75 °, b = 2 \sqrt{6}

のとき

a

②

a = 4, b = \sqrt{21}, C = 5

のとき

B

③

b = 60 °, a : b = 1 : 3

のとき

\sin A

この動画を見る　

【高校数学】　　数Ⅰ－８７　　余弦定理

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
△ABCについて
①

a^{2} =

＿＿＿＿
②

b^{2} =

＿＿＿＿
③

c^{2} =

＿＿＿＿
④

\cos A =

＿＿＿＿
⑤

\cos B =

＿＿＿＿
⑥

\cos C =

＿＿＿＿
※図は動画内参照

◎△ABCにおいて、次のものを求めよう。
⑦

a = 3, b = \sqrt{2}, C = 45 °

のとき

c

⑧

b = 7, c = 5, B = 60 °

のとき

a

この動画を見る　

【高校数学】　　数Ⅰ－８６　　正弦定理

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
△ABCの外接円の半径をRとすると

①＿＿＿＿＝②＿＿＿＿＝③＿＿＿＿＝2R

◎△ABCにおいて、外接円の半径をRとするとき、次のものを求めよう。

④B=120°,R=4のとき b

⑤a=5

\sqrt{3}

,R=5のとき A

⑥A=60°,C=75°,a=

2 \sqrt{6}

のとき Rとb

※図は動画内参照

この動画を見る　

【高校数学】　　数Ⅰ－８５　　三角比⑩

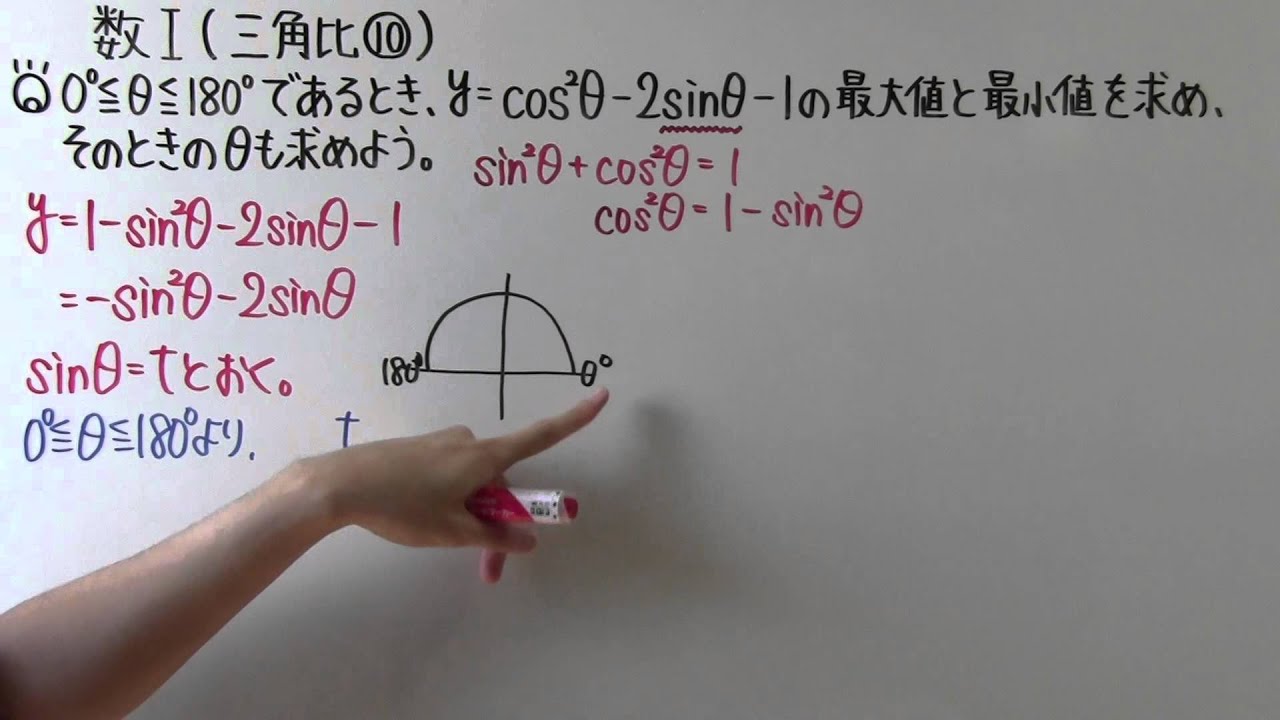
単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：

0 ° ≦ θ ≦ 180 °

であるとき、

y = \cos^{2} θ - 2 \sin θ - 1

の最大値と最小値を求め、そのときの

θ

も求めよう。

この動画を見る　

【高校数学】　　数Ⅰ－８４　　三角比⑨

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：

0 ° ≦ θ ≦ 180 °

とする。次の不等式を満たす

θ

の範囲を求めよう。

①

\sin θ > \frac{\sqrt{3}}{2}

②

\cos θ < \frac{1}{2}

③

\tan θ ≧ \sqrt{3}

④

2 \sin θ - 1 ≦ 0

⑤

2 \cos θ + \sqrt{3} > 0

⑥

\tan θ + 1 ≧ 0

この動画を見る　

【高校数学】　　数Ⅰ－８３　　三角比⑧

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎

0 ° ≦ θ ≦ 180 °, \sin θ + \cos θ = \frac{1}{2}

のとき、次の式の値を求めよう。

①

\sin θ \cos θ

②

\sin^{3} θ + \cos^{3} θ

③

\sin θ - \cos θ

この動画を見る　

【高校数学】　　数Ⅰ－８２　　三角比⑦

単元： #数Ⅰ#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎次の式のとりうる値の範囲を求めよう。

①

\cos θ + 2 (0 ° ≦ θ ≦ 180 °)

②

3 \sin θ - 1 (0 ° ≦ θ ≦ 180 °)

③

\sqrt{2} \sin θ + 3 (45 ° ≦ θ ≦ 120 °)

④

\sqrt{3} \tan θ - 3 (30 ° ≦ θ < 60 °)

この動画を見る　

【高校数学】　　数Ⅰ－８１　　三角比⑥

単元： #数Ⅰ#図形と計量#三角比（三角比・拡張・相互関係・単位円）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎

0 ° ≦ θ ≦ 180 °

のとき、次の等式を満たす

θ

を求めよう。

①

\cos θ = \frac{1}{\sqrt{2}}

②

\sin θ = \sqrt{3}

③

\sqrt{3} \tan θ + 1 = 0

④

0 ° ≦ θ ≦ 180 °

とする。

\sin θ = \frac{4}{5}

のとき、

\cos θ, \tan θ

の値を求めよう。

この動画を見る　

【高校数学】　　数Ⅰ－８０　　三角比⑤

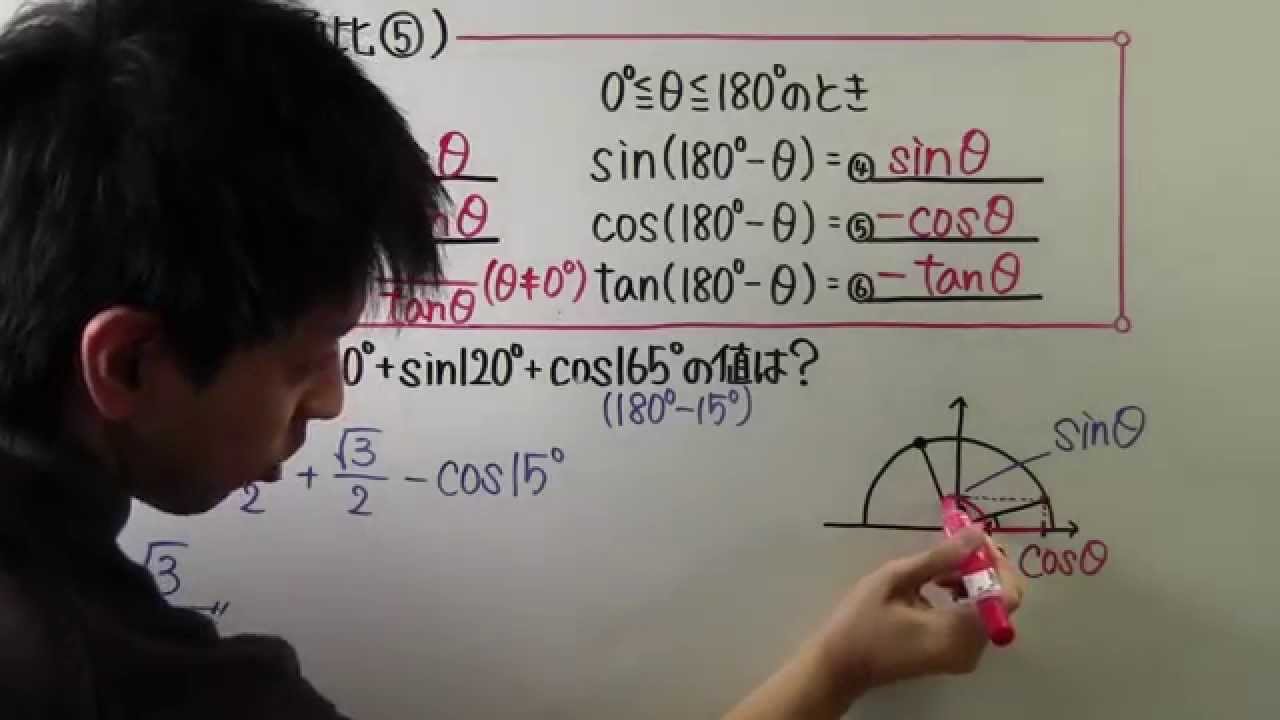
単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：

0 ° ≦ θ ≦ 90 °

のとき

\sin (90 ° + θ) =

①＿＿＿＿

\cos (90 ° + θ) =

②＿＿＿＿

\tan (90 ° + θ) =

③＿＿＿＿

0 ° ≦ θ ≦ 180 °

とき

\sin (180 ° - θ) =

④＿＿＿＿

\cos (180 ° - θ) =

⑤＿＿＿＿

\tan (180 ° - θ) =

⑥＿＿＿＿
⑦

\sin 105 ° - \cos 150 ° + \sin 120 ° + \cos 165 °

の値は？

この動画を見る　

【高校数学】　　数Ⅰ－７９　　三角比④　・　暗記編

単元： #数Ⅰ#図形と計量#三角比への応用（正弦・余弦・面積）#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
空欄を埋めよ。

\begin{array}{ccc} θ & 0 ° & 30 ° & 45 ° & 60 ° & 90 ° & 120 ° & 135 ° & 150 ° & 180 ° \\ \sin θ \\ \cos θ \\ \tan θ \end{array}

この動画を見る　

<img src="https://kaiketsu-db.net/wp-content/uploads/2021/11/112-book-morph-outline.gif" alt="" data-eio="l"> 図形と計量

東大卒のもっちゃんと数学Vol.7 加法定理を証明しよう（東大過去問）

数弱私文の早大生バンカラジオにヨビノリたくみが「優しく」三角関数の基本を教えるよ。余弦定理

【数Ⅰ】図形と計量：単位円と三角比の関係

東北大 三角方程式 Mathematics Japanese university entrance exam

東大卒もっちゃんと数学 余弦定理 Mathematics Japanese university entrance exam

【高校数学】三角比4.5～例題・三角比といえばこれ・基礎～ 3-4.5【数学Ⅰ】

東大 三角比 放物線 Mathematics Japanese university entrance exam Tokyo University

【高校数学】三角比④～90°- θ，180° - θ考え方，イメージ～ 3-4【数学Ⅰ】

【高校数学】三角比③～三角比の大切なイメージ～ 3-3【数学Ⅰ】

【高校数学】三角比②～三角比の重要な公式～ 3-2【数学Ⅰ】

【高校数学】三角比①～三角比とは横顔？？～ 3-1【数学Ⅰ】

名古屋大 円の方程式 ２円と直線に接する円 高校数学 Japanese university entrance exam questions

和歌山大 三項間漸化式 半角の公式 高校数学 Japanese university entrance exam questions

【高校数学】 数Ⅰ－９５ 多角形の面積

【高校数学】 数Ⅰ－９４ 三角形の面積② ・ ヘロンの公式編

【高校数学】 数Ⅰ－９３ 三角形の面積① ・ 基本編

【高校数学】 数Ⅰ－９２ 三角形となる条件

【高校数学】 数Ⅰ－９１ 正弦定理と余弦定理④

【高校数学】 数Ⅰ－９０ 正弦定理と余弦定理③

【高校数学】 数Ⅰ－８９ 正弦定理と余弦定理②

【高校数学】 数Ⅰ－８８ 正弦定理と余弦定理①

【高校数学】 数Ⅰ－８７ 余弦定理

【高校数学】 数Ⅰ－８６ 正弦定理

【高校数学】 数Ⅰ－８５ 三角比⑩

【高校数学】 数Ⅰ－８４ 三角比⑨

【高校数学】 数Ⅰ－８３ 三角比⑧

【高校数学】 数Ⅰ－８２ 三角比⑦

【高校数学】 数Ⅰ－８１ 三角比⑥

【高校数学】 数Ⅰ－８０ 三角比⑤

【高校数学】 数Ⅰ－７９ 三角比④ ・ 暗記編

東北大　三角方程式 Mathematics Japanese university entrance exam

東大卒もっちゃんと数学　余弦定理 Mathematics Japanese university entrance exam

東大　三角比　放物線 Mathematics Japanese university entrance exam Tokyo University

名古屋大　円の方程式　２円と直線に接する円　高校数学 Japanese university entrance exam questions

和歌山大　三項間漸化式　半角の公式　高校数学 Japanese university entrance exam questions

【高校数学】　　数Ⅰ－９５　　多角形の面積

【高校数学】　　数Ⅰ－９４　　三角形の面積②　・　ヘロンの公式編

【高校数学】　　数Ⅰ－９３　　三角形の面積①　・　基本編

【高校数学】　　数Ⅰ－９２　　三角形となる条件

【高校数学】　　数Ⅰ－９１　　正弦定理と余弦定理④

【高校数学】　　数Ⅰ－９０　　正弦定理と余弦定理③

【高校数学】　　数Ⅰ－８９　　正弦定理と余弦定理②

【高校数学】　　数Ⅰ－８８　　正弦定理と余弦定理①

【高校数学】　　数Ⅰ－８７　　余弦定理

【高校数学】　　数Ⅰ－８６　　正弦定理

【高校数学】　　数Ⅰ－８５　　三角比⑩

【高校数学】　　数Ⅰ－８４　　三角比⑨

【高校数学】　　数Ⅰ－８３　　三角比⑧

【高校数学】　　数Ⅰ－８２　　三角比⑦

【高校数学】　　数Ⅰ－８１　　三角比⑥

【高校数学】　　数Ⅰ－８０　　三角比⑤

【高校数学】　　数Ⅰ－７９　　三角比④　・　暗記編