 三角関数
三角関数
 三角関数
三角関数
福田のわかった数学〜高校3年生理系027〜極限(27)関数の極限、三角関数の極限(7)

単元:
#数Ⅱ#三角関数#関数と極限#関数の極限#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
\begin{eqnarray}
数学\textrm{III} 三角関数の極限(7)\\
\\
\lim_{x \to 0}\frac{\sin(2\sin x)}{3x(1+2x)} を求めよ。
\end{eqnarray}
この動画を見る
\begin{eqnarray}
数学\textrm{III} 三角関数の極限(7)\\
\\
\lim_{x \to 0}\frac{\sin(2\sin x)}{3x(1+2x)} を求めよ。
\end{eqnarray}
福田のわかった数学〜高校3年生理系024〜極限(24)関数の極限、三角関数の極限(4)

単元:
#数Ⅱ#三角関数#関数と極限#関数の極限#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
数学$\textrm{III}$ 三角関数の極限(4)
次の極限を求めよ。
(1)$\lim_{x \to 0}x\sin\displaystyle \frac{1}{x}$ (2)$\lim_{x \to -\infty}x\sin\displaystyle \frac{1}{x}$
この動画を見る
数学$\textrm{III}$ 三角関数の極限(4)
次の極限を求めよ。
(1)$\lim_{x \to 0}x\sin\displaystyle \frac{1}{x}$ (2)$\lim_{x \to -\infty}x\sin\displaystyle \frac{1}{x}$
【高校数学】象限と三角関数の符号の関係 4-2【数学Ⅱ】
福田の数学〜早稲田大学2021年理工学部第1問〜2直線のなす角の最小

単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#微分とその応用#微分法#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
${\Large\boxed{1}}$ $xy$平面上の曲線$y=x^3$を$C$とする。$C$上の2点$A(-1,-1), B(1,1)$をとる。
さらに、$C$上で原点$O$と$B$の間に動点$P(t,t^3)(0 \lt t \lt 1)$をとる。このとき、
以下の問いに答えよ。
(1)直線$AP$と$x$軸のなす角を$\alpha$とし、直線$PB$と$x$軸のなす角を$\beta$とするとき、
$\tan\alpha,\tan\beta$を$t$を用いて表せ。ただし、$0 \lt \alpha \lt \displaystyle \frac{\pi}{2},\ 0 \lt \beta \lt \displaystyle \frac{\pi}{2}$とする。
(2)$\tan\angle APB$を$t$を用いて表せ。
(3)$\angle APB$を最小にする$t$の値を求めよ。
2021早稲田大学理工学部過去問
この動画を見る
${\Large\boxed{1}}$ $xy$平面上の曲線$y=x^3$を$C$とする。$C$上の2点$A(-1,-1), B(1,1)$をとる。
さらに、$C$上で原点$O$と$B$の間に動点$P(t,t^3)(0 \lt t \lt 1)$をとる。このとき、
以下の問いに答えよ。
(1)直線$AP$と$x$軸のなす角を$\alpha$とし、直線$PB$と$x$軸のなす角を$\beta$とするとき、
$\tan\alpha,\tan\beta$を$t$を用いて表せ。ただし、$0 \lt \alpha \lt \displaystyle \frac{\pi}{2},\ 0 \lt \beta \lt \displaystyle \frac{\pi}{2}$とする。
(2)$\tan\angle APB$を$t$を用いて表せ。
(3)$\angle APB$を最小にする$t$の値を求めよ。
2021早稲田大学理工学部過去問
加法定理の証明をベクトルで

単元:
#数Ⅱ#三角関数#加法定理とその応用#数学(高校生)
指導講師:
数学を数楽に
問題文全文(内容文):
cos(α+β)=cosαcosβ-sinαsinβ
cos(α-β)=cosαcosβ+sinαsinβ
cosα・cosβ+sinα・sinβ =
この動画を見る
cos(α+β)=cosαcosβ-sinαsinβ
cos(α-β)=cosαcosβ+sinαsinβ
cosα・cosβ+sinα・sinβ =
【行列ができる!】証明:三角関数の加法定理~全国入試問題解法

単元:
#数Ⅱ#三角関数#加法定理とその応用#数学(高校生)
指導講師:
高校入試から見た数学の世界「全部入試問題」by しろたん
問題文全文(内容文):
三角関数における加法定理の証明
【回転変換の解説付き!】
$\sin (a \pmβ)=\sin a \cos β \pm \cos a \sin β$
$\cos (a \pmβ)= \cos a \cos β \mp \sin a \sinβ$
この動画を見る
三角関数における加法定理の証明
【回転変換の解説付き!】
$\sin (a \pmβ)=\sin a \cos β \pm \cos a \sin β$
$\cos (a \pmβ)= \cos a \cos β \mp \sin a \sinβ$
【高校数学】三角関数の最初~一般角と弧度法について~ 4-1【数学Ⅱ】

数学「大学入試良問集」【8−2 三角関数の解の個数】を宇宙一わかりやすく

単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#島根大学
指導講師:
ハクシ高校【数学科】良問演習チャンネル
問題文全文(内容文):
関数$f(\theta)=a(\sqrt{ 3 }\ \sin\theta+\cos\theta)+\sin\theta(\sin\theta+\sqrt{ 3 }\ \cos\theta)$について、次の各問いに答えよ。
ただし、$0 \leqq x \leqq \pi$とする。
(1)$t=\sqrt{ 3 }\ \sin\theta+\cos\theta$のグラフをかけ。
(2)$\sin\theta(\sin\theta+\sqrt{ 3 }\ \cos\theta)$を$t$を用いて表せ。
(3)方程式$f(\theta)=0$が相異なる3つの解をもつときの$a$の値の範囲を求めよ。
この動画を見る
関数$f(\theta)=a(\sqrt{ 3 }\ \sin\theta+\cos\theta)+\sin\theta(\sin\theta+\sqrt{ 3 }\ \cos\theta)$について、次の各問いに答えよ。
ただし、$0 \leqq x \leqq \pi$とする。
(1)$t=\sqrt{ 3 }\ \sin\theta+\cos\theta$のグラフをかけ。
(2)$\sin\theta(\sin\theta+\sqrt{ 3 }\ \cos\theta)$を$t$を用いて表せ。
(3)方程式$f(\theta)=0$が相異なる3つの解をもつときの$a$の値の範囲を求めよ。
数学「大学入試良問集」【8−3 2直線のなす角】を宇宙一わかりやすく

単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)
指導講師:
ハクシ高校【数学科】良問演習チャンネル
問題文全文(内容文):
$x$を正の実数とする。
座標平面上の3点$A(0,1),B(0,2),P(x,x)$をとり、$\triangle ABC$を考える。
$x$の値が変化するとき、$\angle APB$の最大値を求めよ。
この動画を見る
$x$を正の実数とする。
座標平面上の3点$A(0,1),B(0,2),P(x,x)$をとり、$\triangle ABC$を考える。
$x$の値が変化するとき、$\angle APB$の最大値を求めよ。
数学「大学入試良問集」【8−1 三角関数の最大・最小】を宇宙一わかりやすく

単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#富山大学#関西大学
指導講師:
ハクシ高校【数学科】良問演習チャンネル
問題文全文(内容文):
次の問いに答えよ。
(1)
$0 \leqq x \leqq 2\pi$のとき、関数
$y=\sin^2x+\sqrt{ 3 }\ \sin\ x\ \cos\ x-2\cos^2x$の最大値と最小値、および、そのときの$x$の値を求めよ。
(2)
点$(x,y)$が原点を中心とする半径1の円周上を動くとき、$xy(x+y-1)$の最大値と最小値を求めよ。
この動画を見る
次の問いに答えよ。
(1)
$0 \leqq x \leqq 2\pi$のとき、関数
$y=\sin^2x+\sqrt{ 3 }\ \sin\ x\ \cos\ x-2\cos^2x$の最大値と最小値、および、そのときの$x$の値を求めよ。
(2)
点$(x,y)$が原点を中心とする半径1の円周上を動くとき、$xy(x+y-1)$の最大値と最小値を求めよ。
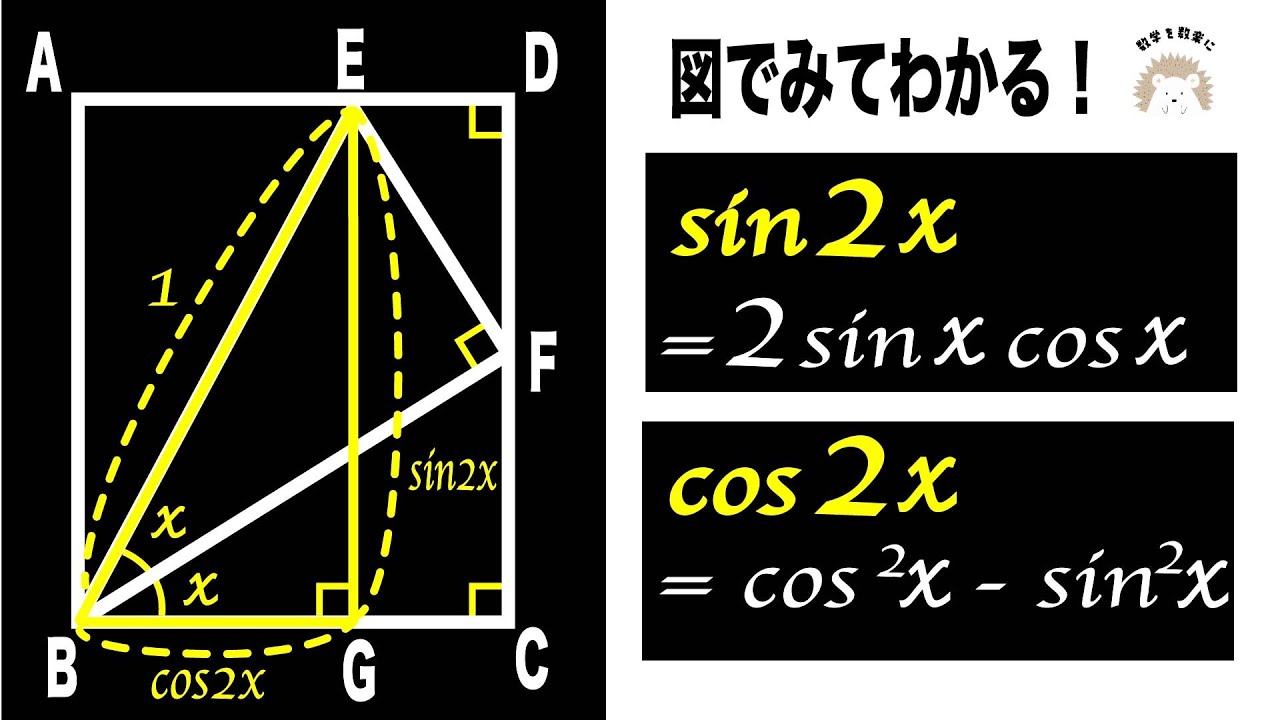
2倍角の公式
単元:
#数Ⅱ#三角関数#加法定理とその応用#数学(高校生)
指導講師:
数学を数楽に
問題文全文(内容文):
$sin2x=2sinxcosx$
$cos2x=cos^2x-sin^2x$
*図は動画内参照
この動画を見る
$sin2x=2sinxcosx$
$cos2x=cos^2x-sin^2x$
*図は動画内参照
ドモアブルの定理の証明と応用

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$n$は整数である.
$Z=\sin\theta+i\cos\theta$
$Z^n$を$\cos n\theta$と$\sin n\theta$を用いて表せ.
2021京都工芸大過去問
この動画を見る
$n$は整数である.
$Z=\sin\theta+i\cos\theta$
$Z^n$を$\cos n\theta$と$\sin n\theta$を用いて表せ.
2021京都工芸大過去問
綺麗な問題

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
次の値を求めよ.
$\cos\dfrac{\pi}{33}・\cos\dfrac{2\pi}{33}・\cos\dfrac{4\pi}{33}・\cos\dfrac{8\pi}{33}・\cos\dfrac{16\pi}{33}$
この動画を見る
次の値を求めよ.
$\cos\dfrac{\pi}{33}・\cos\dfrac{2\pi}{33}・\cos\dfrac{4\pi}{33}・\cos\dfrac{8\pi}{33}・\cos\dfrac{16\pi}{33}$
コメント欄の別解は本当にありがたいです

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$16^{\cos^2 x}+16^{\sin^2 x}=10$の別解に関して解説していきます.
この動画を見る
$16^{\cos^2 x}+16^{\sin^2 x}=10$の別解に関して解説していきます.
【数Ⅱ】三角関数:解が三角関数で表される2次方程式:p>0とする。xの方程式4x²+2(1-p)x-p=0の解が、sinθとcosθ(0≦θ<2π)であるとき、pとθの値を求めよう。

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
解が三角関数で表される2次方程式:p>0とする。xの方程式$4x^2+2(1-p)x-p=0$の解が、$sinθ$と$cosθ(0≦θ<2\pi)$であるとき、$p$と$\theta$の値を求めよう。
この動画を見る
解が三角関数で表される2次方程式:p>0とする。xの方程式$4x^2+2(1-p)x-p=0$の解が、$sinθ$と$cosθ(0≦θ<2\pi)$であるとき、$p$と$\theta$の値を求めよう。
2021東大 円と3次関数の共有点

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$y=ax^3-2x$と原点が中心で半径$1$の円と$6$つの共有点をもつ$a$の範囲を求めよ.$(a\gt 0)$
2021東大過去問
この動画を見る
$y=ax^3-2x$と原点が中心で半径$1$の円と$6$つの共有点をもつ$a$の範囲を求めよ.$(a\gt 0)$
2021東大過去問
【数Ⅱ】三角関数:関数y=-sin²θ+cosθ(0≦θ<2π)の最大値と最小値を求めよう。その時のθも求めよう。

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
関数$y=-\sin^2\theta+\cos\theta(0≦\theta<2\pi)$の最大値と最小値を求めよう。その時の$\theta$も求めよう。
この動画を見る
関数$y=-\sin^2\theta+\cos\theta(0≦\theta<2\pi)$の最大値と最小値を求めよう。その時の$\theta$も求めよう。
産業医科大 cos sin 和の値

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
これを解け.
$\cos\dfrac{2}{7}\pi+\cos\dfrac{4}{7}\pi+\cos\dfrac{8}{7}\pi=\Box$
$\sin\dfrac{2}{7}\pi+\sin\dfrac{4}{7}\pi+\sin\dfrac{8}{7}\pi=\Box$
2019産業医大過去問
この動画を見る
これを解け.
$\cos\dfrac{2}{7}\pi+\cos\dfrac{4}{7}\pi+\cos\dfrac{8}{7}\pi=\Box$
$\sin\dfrac{2}{7}\pi+\sin\dfrac{4}{7}\pi+\sin\dfrac{8}{7}\pi=\Box$
2019産業医大過去問
コメント欄はありがたい。本当に2秒で答えが出た

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$\dfrac{1}{\tan\dfrac{\pi}{24}}$の値を求めよ.
2019横浜市立(医)過去問
この動画を見る
$\dfrac{1}{\tan\dfrac{\pi}{24}}$の値を求めよ.
2019横浜市立(医)過去問
横浜市立(医)tanの半角

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$\dfrac{1}{\tan\dfrac{\pi}{24}}$の値を求めよ.
2019横浜市立(医)過去問
この動画を見る
$\dfrac{1}{\tan\dfrac{\pi}{24}}$の値を求めよ.
2019横浜市立(医)過去問
札幌医科大2021 三角関数 複数解法

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$\triangle ABC$で$\sin C=2\cos A\sin B$である.
$\triangle ABC$の形を求めよ.
2021札幌医大過去問
この動画を見る
$\triangle ABC$で$\sin C=2\cos A\sin B$である.
$\triangle ABC$の形を求めよ.
2021札幌医大過去問
cosの積 華麗な解法で綺麗な答え

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
これを解け.
$\displaystyle \prod_{k=1}^7 \cos\dfrac{\pi}{15}\pi=$
$\cos\dfrac{\pi}{15}\cos\dfrac{2\pi}{15}\cos\dfrac{3\pi}{15}\cos\dfrac{4\pi}{15}\cos\dfrac{5\pi}{15}\cos\dfrac{6\pi}{15}\cos\dfrac{7\pi}{15}$
この動画を見る
これを解け.
$\displaystyle \prod_{k=1}^7 \cos\dfrac{\pi}{15}\pi=$
$\cos\dfrac{\pi}{15}\cos\dfrac{2\pi}{15}\cos\dfrac{3\pi}{15}\cos\dfrac{4\pi}{15}\cos\dfrac{5\pi}{15}\cos\dfrac{6\pi}{15}\cos\dfrac{7\pi}{15}$
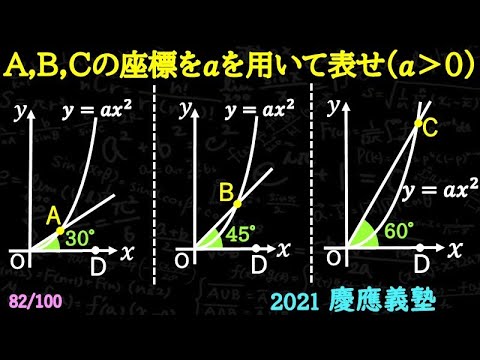
30度 45度 60度の直線の式 A 慶應義塾 2021
単元:
#数学(中学生)#数Ⅱ#三角関数#三角関数とグラフ#高校入試過去問(数学)#数学(高校生)
指導講師:
数学を数楽に
問題文全文(内容文):
A,B,Cの座標をaを用いて表せ
*図は動画内参照
2021慶應義塾高等学校
この動画を見る
A,B,Cの座標をaを用いて表せ
*図は動画内参照
2021慶應義塾高等学校
約束記号 C 慶應義塾 2021
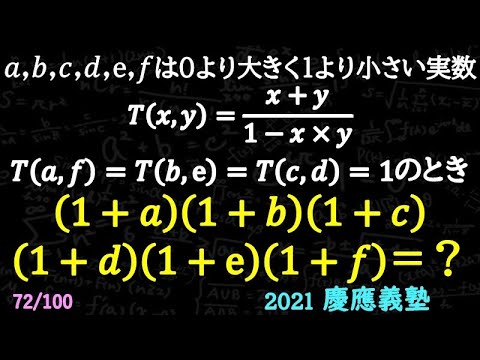
単元:
#数学(中学生)#数Ⅱ#三角関数#加法定理とその応用#高校入試過去問(数学)#数学(高校生)
指導講師:
数学を数楽に
問題文全文(内容文):
a,b,c,d,e,fは0より大きく1より小さい実数
$T(x,y)=\frac{x+y}{1-x \times y}$
$T(a,f) = T(b,e) = T(c,d) = 1$のとき
$(1+a)(1+b)(1+c)(1+d)(1+e)(1+f) =$
2021慶應義塾高等学校
この動画を見る
a,b,c,d,e,fは0より大きく1より小さい実数
$T(x,y)=\frac{x+y}{1-x \times y}$
$T(a,f) = T(b,e) = T(c,d) = 1$のとき
$(1+a)(1+b)(1+c)(1+d)(1+e)(1+f) =$
2021慶應義塾高等学校
瞬殺!!関数の典型問題 A 2021 秋田県

共通テスト第2日程2021年数学詳しい解説〜共通テスト第2日程2021年2B第1問〜対数関数と三角関数

単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#指数関数と対数関数#三角関数とグラフ#加法定理とその応用#指数関数#対数関数#センター試験・共通テスト関連#共通テスト#数学(高校生)
指導講師:
福田次郎
問題文全文(内容文):
${\large第1問}$
[1] (1)$\log_{10}10=\boxed{\ \ ア\ \ }$である。また、$\log_{10}5,\log_{10}15$をそれぞれ
$\log_{10}2と\log_{10}3$を用いて表すと
$\log_{10}5=\boxed{\ \ イ\ \ }\log_{10}2+\boxed{\ \ ウ\ \ }$
$\log_{10}15=\boxed{\ \ エ\ \ }\log_{10}2+\log_{10}3+\boxed{\ \ オ\ \ }$
(2)太郎さんと花子さんは、$15^{20}$について話している。
以下では、$\log_{10}2=0.3010、\log_{10}3=0.4771$とする。
太郎:$15^{20}$は何桁の数だろう。
花子:$15$の20乗を求めるのは大変だね。$\log_{10}15^{20}$の整数部分に
着目してみようよ。
$\log_{10}15^{20}$は
$\boxed{\ \ カキ\ \ } \lt \log_{10}15^{20} \lt \boxed{\ \ カキ\ \ }+1$
を満たす。よって、$15^{20}は\boxed{\ \ クケ\ \ }$桁の数である。
太郎:$15^{20}$の最高位の数字も知りたいね。だけど、$\log_{10}15^{20}$の
整数部分にだけ着目してもわからないな。
花子:$N・10^{\boxed{カキ}} \lt 15^{20} \lt (N+1)・10^{\boxed{カキ}}$を満たすような
正の整数Nに着目してみたらどうかな。
$\log_{10}15^{20}$の小数部分は$\log_{10}15^{20}-\boxed{\ \ カキ\ \ }$であり
$\log_{10}\boxed{\ \ コ\ \ } \lt \log_{10}15^{20}-\boxed{\ \ カキ\ \ } \lt \log_{10}(\boxed{\ \ コ\ \ }+1)$
が成り立つので、$15^{20}$の最高位の数字は$\boxed{\ \ サ\ \ }$である。
[2]座標平面上の原点を中心とする半径1の円周上に3点$P(\cos\theta,\sin\theta),$
$Q(\cos\alpha,\sin\alpha),R(\cos\beta,\sin\beta)$がある。ただし、$0 \leqq \theta \lt \alpha \lt \beta \lt 2\pi$
とする。このとき、$s$と$t$を次のように定める。
$s=\cos\theta+\cos\alpha+\cos\beta, t=\sin\theta+\sin\alpha+\sin\beta$
(1)$\triangle PQR$が正三角形や二等辺三角形のときの$s$と$t$の値について考察しよう。
考察$1:\triangle PQR$が正三角形である場合を考える。
この場合、$\alpha,\beta$を$\theta$で表すと
$\alpha=\theta+\displaystyle \frac{\boxed{\ \ シ\ \ }}{3}\pi, \beta=\theta+\displaystyle \frac{\boxed{\ \ ス\ \ }}{3}\pi$
であり、加法定理により
$\cos\alpha=\boxed{\boxed{\ \ セ\ \ }}, \sin\alpha=\boxed{\boxed{\ \ ソ\ \ }}$
である。同様に、$\cos\beta$および$\sin\beta$を、$\sin\theta$と$\cos\theta$を用いて表すことができる。
これらのことから、$s=t=\boxed{\ \ タ\ \ }$である。
$\boxed{\boxed{\ \ セ\ \ }},\boxed{\boxed{\ \ ソ\ \ }}$の解答群(同じものを繰り返し選んでもよい。)
⓪$\displaystyle \frac{1}{2}\sin\theta+\displaystyle \frac{\sqrt3}{2}\cos\theta$
①$\displaystyle \frac{\sqrt3}{2}\sin\theta+\displaystyle \frac{1}{2}\cos\theta$
②$\displaystyle \frac{1}{2}\sin\theta-\displaystyle \frac{\sqrt3}{2}\cos\theta$
③$\displaystyle \frac{\sqrt3}{2}\sin\theta-\displaystyle \frac{1}{2}\cos\theta$
④$-\displaystyle \frac{1}{2}\sin\theta+\displaystyle \frac{\sqrt3}{2}\cos\theta$
⑤$-\displaystyle \frac{\sqrt3}{2}\sin\theta+\displaystyle \frac{1}{2}\cos\theta$
②$-\displaystyle \frac{1}{2}\sin\theta-\displaystyle \frac{\sqrt3}{2}\cos\theta$
③$-\displaystyle \frac{\sqrt3}{2}\sin\theta-\displaystyle \frac{1}{2}\cos\theta$
考察2:$\triangle PQR$が$PQ=PR$となる二等辺三角形である場合を考える。
例えば、点$P$が直線$y=x$上にあり、点$Q,R$が直線$y=x$に関して対称
であるときを考える。このとき、$\theta=\displaystyle \frac{\pi}{4}$である。また、$\alpha$は
$\alpha \lt \displaystyle \frac{5}{4}\pi, \beta$は$\displaystyle \frac{5}{4}\pi \lt \beta$を満たし、点$Q,R$の座標について、
$\sin\beta=\cos\alpha, \cos\beta=\sin\alpha$が成り立つ。よって
$s=t=\displaystyle \frac{\sqrt{\boxed{\ \ チ\ \ }}}{\boxed{\ \ ツ\ \ }}+\sin\alpha+\cos\alpha$
である。
ここで、三角関数の合成により
$\sin\alpha+\cos\alpha=\sqrt{\boxed{\ \ テ\ \ }}\sin\left(\alpha+\displaystyle \frac{\pi}{\boxed{\ \ ト\ \ }}\right)$
である。したがって
$\alpha=\displaystyle \frac{\boxed{\ \ ナニ\ \ }}{12}\pi, \beta=\displaystyle \frac{\boxed{\ \ ヌネ\ \ }}{12}\pi$
のとき、$s=t=0$である。
(2)次に、$s$と$t$の値を定めるときの$\theta,\alpha,\beta$の関係について考察しよう。
考察$3:s=t=0$の場合を考える。
この場合、$\sin^2\theta+\cos^2\theta=1$により、$\alpha$と$\beta$について考えると
$\cos\alpha\cos\beta+\sin\alpha\sin\beta=\displaystyle \frac{\boxed{\ \ ノハ\ \ }}{\boxed{\ \ ヒ\ \ }}$
である。
同様に、$\theta$と$\alpha$について考えると
$\cos\theta\cos\alpha+\sin\theta\sin\alpha=\displaystyle \frac{\boxed{\ \ ノハ\ \ }}{\boxed{\ \ ヒ\ \ }}$
であるから、$\theta,\alpha,\beta$の範囲に注意すると
$\beta-\alpha=\alpha-\theta=\displaystyle \frac{\boxed{\ \ フ\ \ }}{\boxed{\ \ ヘ\ \ }}\pi$
という関係が得られる。
(3)これまでの考察を振り返ると、次の⓪~③のうち、
正しいものは$\boxed{\boxed{\ \ ホ\ \ }}$であることが分かる。
$\boxed{\boxed{\ \ ホ\ \ }}$の解答群
⓪$\triangle PQR$が正三角形ならば$s=t=0$であり、$s=t=0$ならば
$\triangle PQR$は正三角形である。
①$\triangle PQR$が正三角形ならば$s=t=0$であり、$s=t=0$で
あっても$\triangle PQR$は正三角形でない場合がある。
②$\triangle PQR$が正三角形であっても$s=t=0$でない場合があるが
$s=t=0$ならば$\triangle PQR$は正三角形である。
③$\triangle PQR$が正三角形であっても$s=t=0$でない場合があり、
$s=t=0$であっても$\triangle PQR$が正三角形でない場合がある。
この動画を見る
${\large第1問}$
[1] (1)$\log_{10}10=\boxed{\ \ ア\ \ }$である。また、$\log_{10}5,\log_{10}15$をそれぞれ
$\log_{10}2と\log_{10}3$を用いて表すと
$\log_{10}5=\boxed{\ \ イ\ \ }\log_{10}2+\boxed{\ \ ウ\ \ }$
$\log_{10}15=\boxed{\ \ エ\ \ }\log_{10}2+\log_{10}3+\boxed{\ \ オ\ \ }$
(2)太郎さんと花子さんは、$15^{20}$について話している。
以下では、$\log_{10}2=0.3010、\log_{10}3=0.4771$とする。
太郎:$15^{20}$は何桁の数だろう。
花子:$15$の20乗を求めるのは大変だね。$\log_{10}15^{20}$の整数部分に
着目してみようよ。
$\log_{10}15^{20}$は
$\boxed{\ \ カキ\ \ } \lt \log_{10}15^{20} \lt \boxed{\ \ カキ\ \ }+1$
を満たす。よって、$15^{20}は\boxed{\ \ クケ\ \ }$桁の数である。
太郎:$15^{20}$の最高位の数字も知りたいね。だけど、$\log_{10}15^{20}$の
整数部分にだけ着目してもわからないな。
花子:$N・10^{\boxed{カキ}} \lt 15^{20} \lt (N+1)・10^{\boxed{カキ}}$を満たすような
正の整数Nに着目してみたらどうかな。
$\log_{10}15^{20}$の小数部分は$\log_{10}15^{20}-\boxed{\ \ カキ\ \ }$であり
$\log_{10}\boxed{\ \ コ\ \ } \lt \log_{10}15^{20}-\boxed{\ \ カキ\ \ } \lt \log_{10}(\boxed{\ \ コ\ \ }+1)$
が成り立つので、$15^{20}$の最高位の数字は$\boxed{\ \ サ\ \ }$である。
[2]座標平面上の原点を中心とする半径1の円周上に3点$P(\cos\theta,\sin\theta),$
$Q(\cos\alpha,\sin\alpha),R(\cos\beta,\sin\beta)$がある。ただし、$0 \leqq \theta \lt \alpha \lt \beta \lt 2\pi$
とする。このとき、$s$と$t$を次のように定める。
$s=\cos\theta+\cos\alpha+\cos\beta, t=\sin\theta+\sin\alpha+\sin\beta$
(1)$\triangle PQR$が正三角形や二等辺三角形のときの$s$と$t$の値について考察しよう。
考察$1:\triangle PQR$が正三角形である場合を考える。
この場合、$\alpha,\beta$を$\theta$で表すと
$\alpha=\theta+\displaystyle \frac{\boxed{\ \ シ\ \ }}{3}\pi, \beta=\theta+\displaystyle \frac{\boxed{\ \ ス\ \ }}{3}\pi$
であり、加法定理により
$\cos\alpha=\boxed{\boxed{\ \ セ\ \ }}, \sin\alpha=\boxed{\boxed{\ \ ソ\ \ }}$
である。同様に、$\cos\beta$および$\sin\beta$を、$\sin\theta$と$\cos\theta$を用いて表すことができる。
これらのことから、$s=t=\boxed{\ \ タ\ \ }$である。
$\boxed{\boxed{\ \ セ\ \ }},\boxed{\boxed{\ \ ソ\ \ }}$の解答群(同じものを繰り返し選んでもよい。)
⓪$\displaystyle \frac{1}{2}\sin\theta+\displaystyle \frac{\sqrt3}{2}\cos\theta$
①$\displaystyle \frac{\sqrt3}{2}\sin\theta+\displaystyle \frac{1}{2}\cos\theta$
②$\displaystyle \frac{1}{2}\sin\theta-\displaystyle \frac{\sqrt3}{2}\cos\theta$
③$\displaystyle \frac{\sqrt3}{2}\sin\theta-\displaystyle \frac{1}{2}\cos\theta$
④$-\displaystyle \frac{1}{2}\sin\theta+\displaystyle \frac{\sqrt3}{2}\cos\theta$
⑤$-\displaystyle \frac{\sqrt3}{2}\sin\theta+\displaystyle \frac{1}{2}\cos\theta$
②$-\displaystyle \frac{1}{2}\sin\theta-\displaystyle \frac{\sqrt3}{2}\cos\theta$
③$-\displaystyle \frac{\sqrt3}{2}\sin\theta-\displaystyle \frac{1}{2}\cos\theta$
考察2:$\triangle PQR$が$PQ=PR$となる二等辺三角形である場合を考える。
例えば、点$P$が直線$y=x$上にあり、点$Q,R$が直線$y=x$に関して対称
であるときを考える。このとき、$\theta=\displaystyle \frac{\pi}{4}$である。また、$\alpha$は
$\alpha \lt \displaystyle \frac{5}{4}\pi, \beta$は$\displaystyle \frac{5}{4}\pi \lt \beta$を満たし、点$Q,R$の座標について、
$\sin\beta=\cos\alpha, \cos\beta=\sin\alpha$が成り立つ。よって
$s=t=\displaystyle \frac{\sqrt{\boxed{\ \ チ\ \ }}}{\boxed{\ \ ツ\ \ }}+\sin\alpha+\cos\alpha$
である。
ここで、三角関数の合成により
$\sin\alpha+\cos\alpha=\sqrt{\boxed{\ \ テ\ \ }}\sin\left(\alpha+\displaystyle \frac{\pi}{\boxed{\ \ ト\ \ }}\right)$
である。したがって
$\alpha=\displaystyle \frac{\boxed{\ \ ナニ\ \ }}{12}\pi, \beta=\displaystyle \frac{\boxed{\ \ ヌネ\ \ }}{12}\pi$
のとき、$s=t=0$である。
(2)次に、$s$と$t$の値を定めるときの$\theta,\alpha,\beta$の関係について考察しよう。
考察$3:s=t=0$の場合を考える。
この場合、$\sin^2\theta+\cos^2\theta=1$により、$\alpha$と$\beta$について考えると
$\cos\alpha\cos\beta+\sin\alpha\sin\beta=\displaystyle \frac{\boxed{\ \ ノハ\ \ }}{\boxed{\ \ ヒ\ \ }}$
である。
同様に、$\theta$と$\alpha$について考えると
$\cos\theta\cos\alpha+\sin\theta\sin\alpha=\displaystyle \frac{\boxed{\ \ ノハ\ \ }}{\boxed{\ \ ヒ\ \ }}$
であるから、$\theta,\alpha,\beta$の範囲に注意すると
$\beta-\alpha=\alpha-\theta=\displaystyle \frac{\boxed{\ \ フ\ \ }}{\boxed{\ \ ヘ\ \ }}\pi$
という関係が得られる。
(3)これまでの考察を振り返ると、次の⓪~③のうち、
正しいものは$\boxed{\boxed{\ \ ホ\ \ }}$であることが分かる。
$\boxed{\boxed{\ \ ホ\ \ }}$の解答群
⓪$\triangle PQR$が正三角形ならば$s=t=0$であり、$s=t=0$ならば
$\triangle PQR$は正三角形である。
①$\triangle PQR$が正三角形ならば$s=t=0$であり、$s=t=0$で
あっても$\triangle PQR$は正三角形でない場合がある。
②$\triangle PQR$が正三角形であっても$s=t=0$でない場合があるが
$s=t=0$ならば$\triangle PQR$は正三角形である。
③$\triangle PQR$が正三角形であっても$s=t=0$でない場合があり、
$s=t=0$であっても$\triangle PQR$が正三角形でない場合がある。
【意外とミスる】三角関数・基本問題

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
カサニマロ【べんとう・ふきのとうの授業動画】
問題文全文(内容文):
三角関数・基本問題解説動画です
-----------------
$0 \leqq \theta \lt 2\pi$のとき、$\sin(2 \theta + \displaystyle \frac{\pi}{4})=\displaystyle \frac{\sqrt{ 3 }}{2}$を満たす$\theta$の値を求めよ。
この動画を見る
三角関数・基本問題解説動画です
-----------------
$0 \leqq \theta \lt 2\pi$のとき、$\sin(2 \theta + \displaystyle \frac{\pi}{4})=\displaystyle \frac{\sqrt{ 3 }}{2}$を満たす$\theta$の値を求めよ。
【数Ⅱ】三角関数のグラフ③ 横の変化(y=sin(θ-π/2)、y=sin2θのグラフ)

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
$y=sin(θ-π/2)、y=sin2θ$のグラフを解説していきます.
この動画を見る
$y=sin(θ-π/2)、y=sin2θ$のグラフを解説していきます.
【数Ⅱ】三角関数のグラフ② 縦の変化(y=2sinθ、y=sinθ+1のグラフ)

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
三角関数のグラフ①の続きです。この動画では縦の変化($y=2sinθ、y=sinθ+1$のグラフ)を扱います。
この動画を見る
三角関数のグラフ①の続きです。この動画では縦の変化($y=2sinθ、y=sinθ+1$のグラフ)を扱います。
【数Ⅱ】三角関数のグラフ①(y=sinθ、y=cosθ、y=tanθのグラフ)

