 三角関数
三角関数
 三角関数
三角関数
共通テスト2021年数学詳しい解説〜共通テスト2021年2B第1問〜三角関数、指数関数

単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#指数関数と対数関数#三角関数とグラフ#加法定理とその応用#指数関数#対数関数#センター試験・共通テスト関連#共通テスト#センター試験#数学(高校生)
指導講師:
福田次郎
問題文全文(内容文):
${\large第1問}$
[1](1)次の問題$A$について考えよう。
$\boxed{\boxed{問題A} 関数y=\sin\theta+\sqrt3\cos\theta\left(0 \leqq \theta \leqq \displaystyle \frac{\pi}{2}\right)$の最大値を求めよ。}$
$\sin\displaystyle \frac{\pi}{\boxed{\ \ ア\ \ }}=\displaystyle \frac{\sqrt3}{2},$ $\cos\displaystyle \frac{\pi}{\boxed{\ \ ア\ \ }}=\displaystyle \frac{1}{2}$
であるから、三角関数の合成により
$y=\boxed{\ \ イ\ \ }\sin\left(\theta+\displaystyle \frac{\pi}{\boxed{\ \ ア\ \ }}\right)$
と変形できる。よって、$y$は$\theta=\displaystyle \frac{\pi}{\boxed{\ \ ウ\ \ }}$で最大値$\ \boxed{\ \ エ\ \ }\ $をとる。
(2)$p$を定数とし、次の問題$B$について考えよう。
$\boxed{\boxed{問題B} 関数y=\sin\theta+p\cos\theta\left(0 \leqq \theta \leqq \frac{\pi}{2}\right)の最大値を求めよ。}$
$(\textrm{i})$ $p=0$のとき、$y$は$\theta=\displaystyle \frac{\pi}{\boxed{\ \ オ\ \ }}$で最大値$\ \boxed{\ \ カ\ \ }\ $をとる。
$(\textrm{ii})$ $p \gt 0$のときは、加法定理
$\cos(\theta-\alpha)=\cos\theta\cos\alpha+\sin\theta\sin\alpha$
を用いると
$y=\sin\theta+p\cos\theta=\sqrt{\boxed{\boxed{\ \ キ\ \ }}}\cos(\theta-\alpha)$
と表すことができる。ただし、$\alpha$は
$\sin\alpha=\displaystyle \frac{\boxed{\boxed{\ \ ク\ \ }}}{\sqrt{\boxed{\boxed{\ \ キ\ \ }}}}$、$\cos\alpha=\frac{\boxed{\boxed{\ \ ケ\ \ }}}{\sqrt{\boxed{\boxed{\ \ キ\ \ }}}}$、$0 \lt \alpha \lt \displaystyle \frac{\pi}{2}$
を満たすものとする。このとき、$y$は$\theta=\boxed{\boxed{\ \ コ\ \ }}$で最大値
$\sqrt{\boxed{\boxed{\ \ サ\ \ }}}$をとる。
$(\textrm{iii})$ $p \lt 0$のとき、$y$は$\theta=\boxed{\boxed{\ \ シ\ \ }}$で最大値$\boxed{\boxed{\ \ ス\ \ }}$をとる。
$\boxed{\boxed{\ \ キ\ \ }}~\boxed{\boxed{\ \ ケ\ \ }}、\boxed{\boxed{\ \ サ\ \ }}、\boxed{\boxed{\ \ ス\ \ }}$の解答群(同じものを繰り返
し選んでもよい。)
⓪$-1$
①$1$
②$-p$
③$p$
④$1-p$
⑤$1+p$
⑥$-p^2$
⑦$p^2$
⑧$1-p^2$
⑨$1+p^2$
ⓐ$(1-p)^2$
ⓑ$(1+p)^2$
$\boxed{\boxed{\ \ コ\ \ }}、\boxed{\boxed{\ \ シ\ \ }}$の解答群(同じものを繰り返し選んでもよい。)
⓪$0$
①$\alpha$
②$\displaystyle \frac{\pi}{2}$
[2]二つの関数$f(x)=\displaystyle \frac{2^x+2^{-x}}{2}$、$g(x)=\displaystyle \frac{2^x-2^{-x}}{2}$ について考える。
(1)$f(0)=\boxed{\ \ セ\ \ }、g(0)=\boxed{\ \ ソ\ \ }$である。また、$f(x)$は相加平均
と相乗平均の関係から、$x=\boxed{\ \ タ\ \ }$で最小値$\ \boxed{\ \ チ\ \ }\$ をとる。
$g(x)=-2\$ となる$x$の値は$\log_2\left(\sqrt{\boxed{\ \ ツ\ \ }}-\boxed{\ \ テ\ \ }\right)$である。
(3)次の①~④は、$x$にどのような値を代入しても常に成り立つ。
$f(-x)=\boxed{\boxed{\ \ ト\ \ }}$ $\cdots$①
$g(-x)=\boxed{\boxed{\ \ ナ\ \ }}$ $\cdots$②
$\left\{f(x)\right\}^2-\left\{g(x)\right\}^2=\boxed{\ \ ニ\ \ }$ $\cdots$③
$g(2x)=\boxed{\ \ ヌ\ \ }\ f(x)g(x)$ $\cdots$④
$\boxed{\boxed{\ \ ト\ \ }}、\boxed{\boxed{\ \ ナ\ \ }}$の解答群(同じものを繰り返し選んでもよい。)
⓪$f(x)$
①$-f(x)$
②$g(x)$
③$-g(x)$
(3)花子さんと太郎さんは、$f(x)$と$g(x)$の性質について話している。
花子:①~④は三角関数の性質に似ているね。
太郎:三角関数の加法定理に類似した式($\textrm{A}$)~($\textrm{D}$)を考えてみたけど、
常に成り立つ式はあるだろうか。
花子:成り立たない式を見つけるために、式($\textrm{A}$)~($\textrm{D}$)の$\beta$に何か具体
的な値を代入して調べてみたらどうかな。
太郎さんが考えた式
$f(\alpha-\beta)=f(\alpha)g(\beta)+g(\alpha)f(\beta)$ $\cdots(\textrm{A})$
$f(\alpha+\beta)=f(\alpha)f(\beta)+g(\alpha)g(\beta)$ $\cdots(\textrm{B})$
$g(\alpha-\beta)=f(\alpha)f(\beta)+g(\alpha)g(\beta)$ $\cdots(\textrm{C})$
$g(\alpha+\beta)=f(\alpha)g(\beta)-g(\alpha)f(\beta)$ $\cdots(\textrm{D})$
(1),(2)で示されたことのいくつかを利用すると、式($\textrm{A}$)~($\textrm{D}$)のうち、
$\boxed{\boxed{\ \ ネ\ \ }}$以外の三つは成り立たないことが分かる。$\boxed{\boxed{\ \ ネ\ \ }}$は左辺と右辺
をそれぞれ計算することによって成り立つことが確かめられる。
$\boxed{\boxed{\ \ ネ\ \ }}$の解答群
⓪$(\textrm{A})$
①$(\textrm{B})$
②$(\textrm{C})$
③$(\textrm{D})$
2021共通テスト過去問
この動画を見る
${\large第1問}$
[1](1)次の問題$A$について考えよう。
$\boxed{\boxed{問題A} 関数y=\sin\theta+\sqrt3\cos\theta\left(0 \leqq \theta \leqq \displaystyle \frac{\pi}{2}\right)$の最大値を求めよ。}$
$\sin\displaystyle \frac{\pi}{\boxed{\ \ ア\ \ }}=\displaystyle \frac{\sqrt3}{2},$ $\cos\displaystyle \frac{\pi}{\boxed{\ \ ア\ \ }}=\displaystyle \frac{1}{2}$
であるから、三角関数の合成により
$y=\boxed{\ \ イ\ \ }\sin\left(\theta+\displaystyle \frac{\pi}{\boxed{\ \ ア\ \ }}\right)$
と変形できる。よって、$y$は$\theta=\displaystyle \frac{\pi}{\boxed{\ \ ウ\ \ }}$で最大値$\ \boxed{\ \ エ\ \ }\ $をとる。
(2)$p$を定数とし、次の問題$B$について考えよう。
$\boxed{\boxed{問題B} 関数y=\sin\theta+p\cos\theta\left(0 \leqq \theta \leqq \frac{\pi}{2}\right)の最大値を求めよ。}$
$(\textrm{i})$ $p=0$のとき、$y$は$\theta=\displaystyle \frac{\pi}{\boxed{\ \ オ\ \ }}$で最大値$\ \boxed{\ \ カ\ \ }\ $をとる。
$(\textrm{ii})$ $p \gt 0$のときは、加法定理
$\cos(\theta-\alpha)=\cos\theta\cos\alpha+\sin\theta\sin\alpha$
を用いると
$y=\sin\theta+p\cos\theta=\sqrt{\boxed{\boxed{\ \ キ\ \ }}}\cos(\theta-\alpha)$
と表すことができる。ただし、$\alpha$は
$\sin\alpha=\displaystyle \frac{\boxed{\boxed{\ \ ク\ \ }}}{\sqrt{\boxed{\boxed{\ \ キ\ \ }}}}$、$\cos\alpha=\frac{\boxed{\boxed{\ \ ケ\ \ }}}{\sqrt{\boxed{\boxed{\ \ キ\ \ }}}}$、$0 \lt \alpha \lt \displaystyle \frac{\pi}{2}$
を満たすものとする。このとき、$y$は$\theta=\boxed{\boxed{\ \ コ\ \ }}$で最大値
$\sqrt{\boxed{\boxed{\ \ サ\ \ }}}$をとる。
$(\textrm{iii})$ $p \lt 0$のとき、$y$は$\theta=\boxed{\boxed{\ \ シ\ \ }}$で最大値$\boxed{\boxed{\ \ ス\ \ }}$をとる。
$\boxed{\boxed{\ \ キ\ \ }}~\boxed{\boxed{\ \ ケ\ \ }}、\boxed{\boxed{\ \ サ\ \ }}、\boxed{\boxed{\ \ ス\ \ }}$の解答群(同じものを繰り返
し選んでもよい。)
⓪$-1$
①$1$
②$-p$
③$p$
④$1-p$
⑤$1+p$
⑥$-p^2$
⑦$p^2$
⑧$1-p^2$
⑨$1+p^2$
ⓐ$(1-p)^2$
ⓑ$(1+p)^2$
$\boxed{\boxed{\ \ コ\ \ }}、\boxed{\boxed{\ \ シ\ \ }}$の解答群(同じものを繰り返し選んでもよい。)
⓪$0$
①$\alpha$
②$\displaystyle \frac{\pi}{2}$
[2]二つの関数$f(x)=\displaystyle \frac{2^x+2^{-x}}{2}$、$g(x)=\displaystyle \frac{2^x-2^{-x}}{2}$ について考える。
(1)$f(0)=\boxed{\ \ セ\ \ }、g(0)=\boxed{\ \ ソ\ \ }$である。また、$f(x)$は相加平均
と相乗平均の関係から、$x=\boxed{\ \ タ\ \ }$で最小値$\ \boxed{\ \ チ\ \ }\$ をとる。
$g(x)=-2\$ となる$x$の値は$\log_2\left(\sqrt{\boxed{\ \ ツ\ \ }}-\boxed{\ \ テ\ \ }\right)$である。
(3)次の①~④は、$x$にどのような値を代入しても常に成り立つ。
$f(-x)=\boxed{\boxed{\ \ ト\ \ }}$ $\cdots$①
$g(-x)=\boxed{\boxed{\ \ ナ\ \ }}$ $\cdots$②
$\left\{f(x)\right\}^2-\left\{g(x)\right\}^2=\boxed{\ \ ニ\ \ }$ $\cdots$③
$g(2x)=\boxed{\ \ ヌ\ \ }\ f(x)g(x)$ $\cdots$④
$\boxed{\boxed{\ \ ト\ \ }}、\boxed{\boxed{\ \ ナ\ \ }}$の解答群(同じものを繰り返し選んでもよい。)
⓪$f(x)$
①$-f(x)$
②$g(x)$
③$-g(x)$
(3)花子さんと太郎さんは、$f(x)$と$g(x)$の性質について話している。
花子:①~④は三角関数の性質に似ているね。
太郎:三角関数の加法定理に類似した式($\textrm{A}$)~($\textrm{D}$)を考えてみたけど、
常に成り立つ式はあるだろうか。
花子:成り立たない式を見つけるために、式($\textrm{A}$)~($\textrm{D}$)の$\beta$に何か具体
的な値を代入して調べてみたらどうかな。
太郎さんが考えた式
$f(\alpha-\beta)=f(\alpha)g(\beta)+g(\alpha)f(\beta)$ $\cdots(\textrm{A})$
$f(\alpha+\beta)=f(\alpha)f(\beta)+g(\alpha)g(\beta)$ $\cdots(\textrm{B})$
$g(\alpha-\beta)=f(\alpha)f(\beta)+g(\alpha)g(\beta)$ $\cdots(\textrm{C})$
$g(\alpha+\beta)=f(\alpha)g(\beta)-g(\alpha)f(\beta)$ $\cdots(\textrm{D})$
(1),(2)で示されたことのいくつかを利用すると、式($\textrm{A}$)~($\textrm{D}$)のうち、
$\boxed{\boxed{\ \ ネ\ \ }}$以外の三つは成り立たないことが分かる。$\boxed{\boxed{\ \ ネ\ \ }}$は左辺と右辺
をそれぞれ計算することによって成り立つことが確かめられる。
$\boxed{\boxed{\ \ ネ\ \ }}$の解答群
⓪$(\textrm{A})$
①$(\textrm{B})$
②$(\textrm{C})$
③$(\textrm{D})$
2021共通テスト過去問
sin10 vs sin11(弧度法)どっちがでかい?

単元:
#数Ⅱ#三角関数#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
どちらが大きいか?$3\lt \pi \lt 4$である.
$\sin 10$ VS $\sin 11$
この動画を見る
どちらが大きいか?$3\lt \pi \lt 4$である.
$\sin 10$ VS $\sin 11$
【数Ⅱ】三角関数:弧度法の考え方② -19π/6って第何象限でどんな形?!

【数Ⅱ】三角関数:弧度法の考え方①

数検準1級2次過去問【2020年12月】1番:三角関数

単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#三角関数#加法定理とその応用#数学検定#数学検定準1級#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
1⃣
(1) $θ=\frac{\pi}{10}$のとき
$sin2θ=cos3θ$を示せ
(2)$sin \frac{\pi}{10}$を求めよ。
この動画を見る
1⃣
(1) $θ=\frac{\pi}{10}$のとき
$sin2θ=cos3θ$を示せ
(2)$sin \frac{\pi}{10}$を求めよ。
14京都府教員採用試験(数学:5番 三角関数)

単元:
#数Ⅱ#三角関数#三角関数とグラフ#その他#数学(高校生)#教員採用試験
指導講師:
ますただ
問題文全文(内容文):
5⃣$y=a(1+sinx)cosx(0 \leqq x \leqq 2\pi)$
の最大値が18のときaの値を求めよ。(a>0)
この動画を見る
5⃣$y=a(1+sinx)cosx(0 \leqq x \leqq 2\pi)$
の最大値が18のときaの値を求めよ。(a>0)
【数学Ⅱ】三角関数の式の証明

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
カサニマロ【べんとう・ふきのとうの授業動画】
問題文全文(内容文):
【数学Ⅱ】三角関数の式の証明解説動画です
-----------------
$\displaystyle \frac{cos^2 \theta - \sin^2 \theta}{1+2 \sin \theta \cos \theta}=\displaystyle \frac{1- \tan \theta}{1+ \tan \theta}$
この動画を見る
【数学Ⅱ】三角関数の式の証明解説動画です
-----------------
$\displaystyle \frac{cos^2 \theta - \sin^2 \theta}{1+2 \sin \theta \cos \theta}=\displaystyle \frac{1- \tan \theta}{1+ \tan \theta}$
【数Ⅱ】三角関数:2倍角の公式の利用! 直線y=1/3 xが直線y=axとx軸の正の向きとのなす角の二等分線となっているとき、aの値を求めよ。

単元:
#数Ⅱ#三角関数#加法定理とその応用#数学(高校生)
指導講師:
理数個別チャンネル
問題文全文(内容文):
直線$y=\dfrac{1}{3}$ xが直線$y=ax$とx軸の正の向きとのなす角の二等分線となっているとき、aの値を求めよ。
この動画を見る
直線$y=\dfrac{1}{3}$ xが直線$y=ax$とx軸の正の向きとのなす角の二等分線となっているとき、aの値を求めよ。
19奈良県教員採用試験(数学:2番 三角関数)

単元:
#数Ⅱ#三角関数#三角関数とグラフ#接線と増減表・最大値・最小値#その他#数学(高校生)#教員採用試験
指導講師:
ますただ
問題文全文(内容文):
2⃣$0 \leqq θ \leqq \pi$
$y= sin2θ + 2(sinθ+cosθ)-i$のMAX、minとそのときのθの値を求めよ。
この動画を見る
2⃣$0 \leqq θ \leqq \pi$
$y= sin2θ + 2(sinθ+cosθ)-i$のMAX、minとそのときのθの値を求めよ。
京都大学 5倍角の公式

単元:
#数学(中学生)#中2数学#式の計算(単項式・多項式・式の四則計算)#数Ⅱ#三角関数#三角関数とグラフ
指導講師:
鈴木貫太郎
問題文全文(内容文):
(1)$\cos5\theta=f(\cos\theta)$を満たす多項式$f(n)$を求めよ.
(2)$\cos\dfrac{\pi}{10}\cos\dfrac{3\pi}{10}\cos\dfrac{7\pi}{10}\cos\dfrac{9\pi}{10}=\dfrac{5}{16}$を示せ.
1996京都大過去問
この動画を見る
(1)$\cos5\theta=f(\cos\theta)$を満たす多項式$f(n)$を求めよ.
(2)$\cos\dfrac{\pi}{10}\cos\dfrac{3\pi}{10}\cos\dfrac{7\pi}{10}\cos\dfrac{9\pi}{10}=\dfrac{5}{16}$を示せ.
1996京都大過去問
【コツ】三角関数のグラフの書き方

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
カサニマロ【べんとう・ふきのとうの授業動画】
問題文全文(内容文):
三角関数のグラフの書き方紹介動画です
-----------------
(1)$y=\sin(\theta -\displaystyle \frac{\pi}{3})$
(2)$y=\cos(\theta+\displaystyle \frac{\pi}{6})$
(3)$y=\tan(\theta-\displaystyle \frac{\pi}{4})$
この動画を見る
三角関数のグラフの書き方紹介動画です
-----------------
(1)$y=\sin(\theta -\displaystyle \frac{\pi}{3})$
(2)$y=\cos(\theta+\displaystyle \frac{\pi}{6})$
(3)$y=\tan(\theta-\displaystyle \frac{\pi}{4})$
埼玉大 3次関数の極値の差 ヨビノリ技

単元:
#数Ⅱ#三角関数#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$f(x)=x^3+ax^2-x$の極大値と極小値は差が$4$で和が正である.
$a$の値を求めよ.
2018埼玉大過去問
この動画を見る
$f(x)=x^3+ax^2-x$の極大値と極小値は差が$4$で和が正である.
$a$の値を求めよ.
2018埼玉大過去問
15東京都教員採用試験(数学:3番 積分)

単元:
#数Ⅱ#三角関数#三角関数とグラフ#積分とその応用#面積・体積・長さ・速度#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
3⃣$C_1:y=sin2x,C_2:y=k sinx$
$0 \leqq x \leqq \frac{\pi}{2}$ , $0 < k <2$
(1)$C_1$とx軸で囲まれた図形の面積
(2)$C_1$と$C_2$の原点以外の支点のx座標をαとする。cosαを求めよ。
(3)$C_1$とx軸で囲まれた部分の面積を$C_2$が2等分するときkの値を求めよ。
この動画を見る
3⃣$C_1:y=sin2x,C_2:y=k sinx$
$0 \leqq x \leqq \frac{\pi}{2}$ , $0 < k <2$
(1)$C_1$とx軸で囲まれた図形の面積
(2)$C_1$と$C_2$の原点以外の支点のx座標をαとする。cosαを求めよ。
(3)$C_1$とx軸で囲まれた部分の面積を$C_2$が2等分するときkの値を求めよ。
中央大2020微分 3次関数と直線の交点

単元:
#数Ⅱ#三角関数#微分法と積分法#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$f(x)=x^3+3x^2-2$と$y=k(x-1)-2$が相異なる3点で交わる$k$の範囲を求めよ.
2020中央大(経)過去問
この動画を見る
$f(x)=x^3+3x^2-2$と$y=k(x-1)-2$が相異なる3点で交わる$k$の範囲を求めよ.
2020中央大(経)過去問
早稲田大2019微分・3次関数と直線の交点

単元:
#数Ⅱ#三角関数#微分法と積分法#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$y=x^2$上の$(a,a^2)$における接線が$y=x^3-ax$と3点で交わる$a$の範囲を求めよ.
2019早稲田大過去問
この動画を見る
$y=x^2$上の$(a,a^2)$における接線が$y=x^3-ax$と3点で交わる$a$の範囲を求めよ.
2019早稲田大過去問
19神奈川県教員採用試験(数学:5番 三角関数)
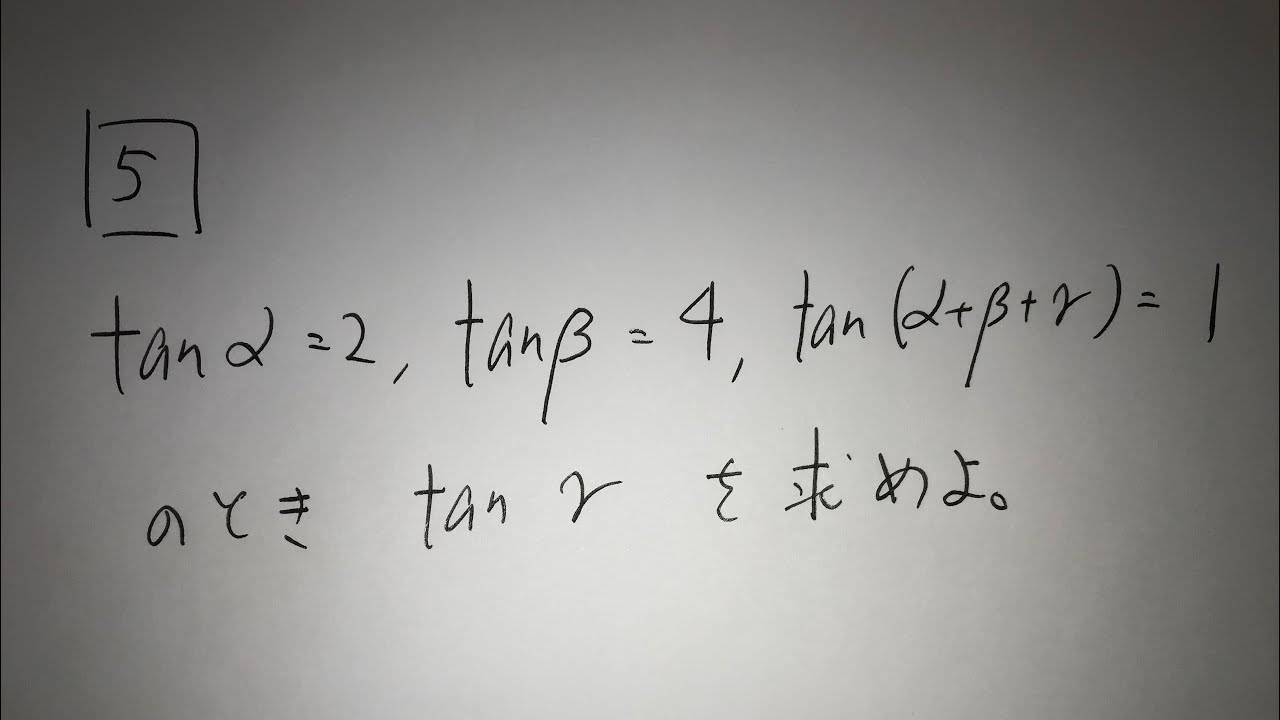
単元:
#数Ⅱ#三角関数#加法定理とその応用#その他#数学(高校生)#教員採用試験
指導講師:
ますただ
問題文全文(内容文):
5⃣
tanα=2,tanβ=4,tan(α+β+γ)=1のときtanγを求めよ。
この動画を見る
5⃣
tanα=2,tanβ=4,tan(α+β+γ)=1のときtanγを求めよ。
早稲田(商)三角関数・微分

単元:
#数Ⅱ#三角関数#微分法と積分法#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$(\sin\theta+\cos\theta)^6-6\sin\theta\cos\theta$の最大値・最小値を求めよ.
1996早稲田(商)過去問
この動画を見る
$(\sin\theta+\cos\theta)^6-6\sin\theta\cos\theta$の最大値・最小値を求めよ.
1996早稲田(商)過去問
20年5月数学検定1級1次試験(微分)
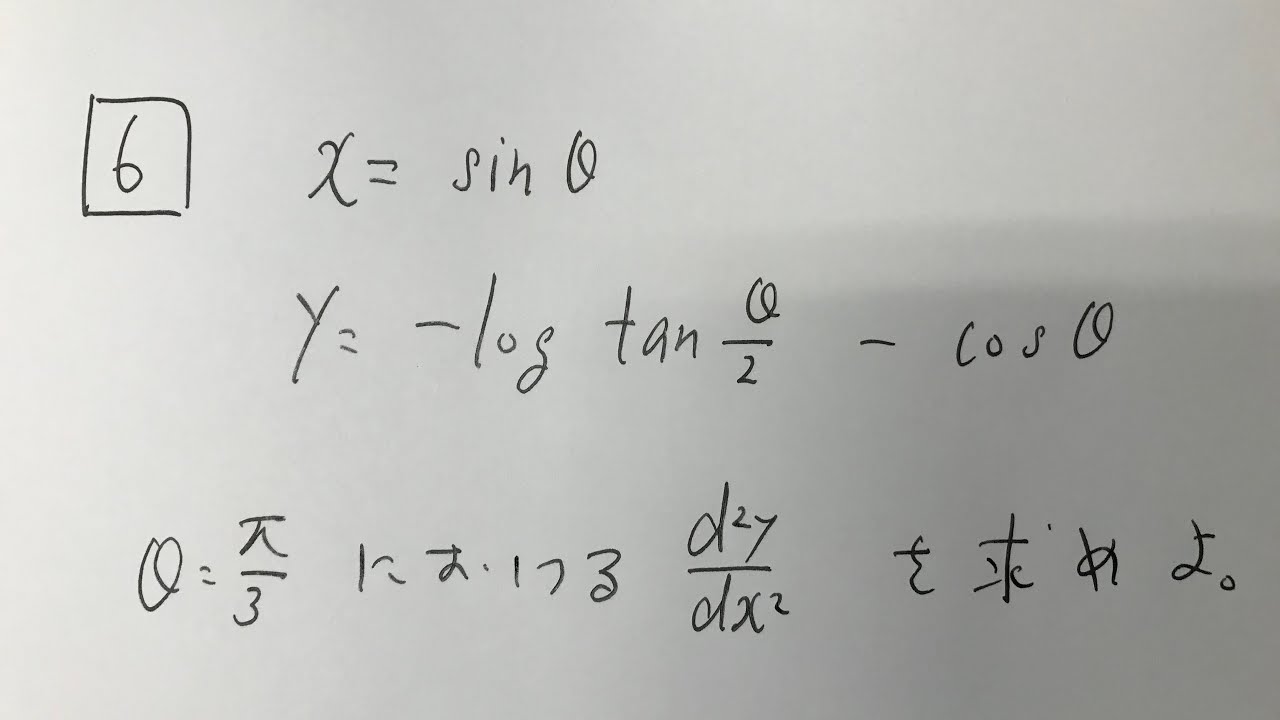
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#三角関数#三角関数とグラフ#関数と極限#関数(分数関数・無理関数・逆関数と合成関数)#数学検定#数学検定1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
6⃣
x=sinθ
$y=-log tan \frac{θ}{2}-cosθ$
$θ=\frac{\pi}{3}$における$\frac{dy}{dx^2}$を求めよ。
この動画を見る
6⃣
x=sinθ
$y=-log tan \frac{θ}{2}-cosθ$
$θ=\frac{\pi}{3}$における$\frac{dy}{dx^2}$を求めよ。
20年5月数学検定1級1次試験(三角関数)

単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#三角関数#数学検定#数学検定1級#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\boxed{2}$
$\tan(2Arc\tan\dfrac{1}{3}+Arc\tan\dfrac{1}{12})$
$Arc\tan a=\tan^{-1}a=t\Leftrightarrow t=\tan a$
$\tan(\tan^{-1}a)=a$
$\tan(\alpha+\beta)=\dfrac{\tan\alpha+\tan\beta}{1-\tan\alpha\tan\beta}$
20年5月数学検定1級1次試験(三角関数)過去問
この動画を見る
$\boxed{2}$
$\tan(2Arc\tan\dfrac{1}{3}+Arc\tan\dfrac{1}{12})$
$Arc\tan a=\tan^{-1}a=t\Leftrightarrow t=\tan a$
$\tan(\tan^{-1}a)=a$
$\tan(\alpha+\beta)=\dfrac{\tan\alpha+\tan\beta}{1-\tan\alpha\tan\beta}$
20年5月数学検定1級1次試験(三角関数)過去問
20年5月数学検定1級1次試験(三角関数)

単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#三角関数#三角関数とグラフ#数学検定#数学検定1級#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
2⃣
$tan(2Arctan \frac{1}{3} + Arctan \frac{1}{12} )$
この動画を見る
2⃣
$tan(2Arctan \frac{1}{3} + Arctan \frac{1}{12} )$
3次関数 三角形の面積最大 お茶の水女子大

単元:
#数A#数Ⅱ#大学入試過去問(数学)#図形の性質#三角形の辺の比(内分・外分・二等分線)#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#数学(高校生)#お茶の水女子大学
指導講師:
鈴木貫太郎
問題文全文(内容文):
$f(x)=x^3-6x^2+8x$,3点$O,A(3,f(3))$,$P(t,f(t)),0\lt t\leqq 4,t\neq 3$である.
$\triangle OAP$の面積が最大となる$t$の値を求めよ.
1987お茶の水女子大過去問
この動画を見る
$f(x)=x^3-6x^2+8x$,3点$O,A(3,f(3))$,$P(t,f(t)),0\lt t\leqq 4,t\neq 3$である.
$\triangle OAP$の面積が最大となる$t$の値を求めよ.
1987お茶の水女子大過去問
20年5月数学検定準1級1次試験(三角関数)
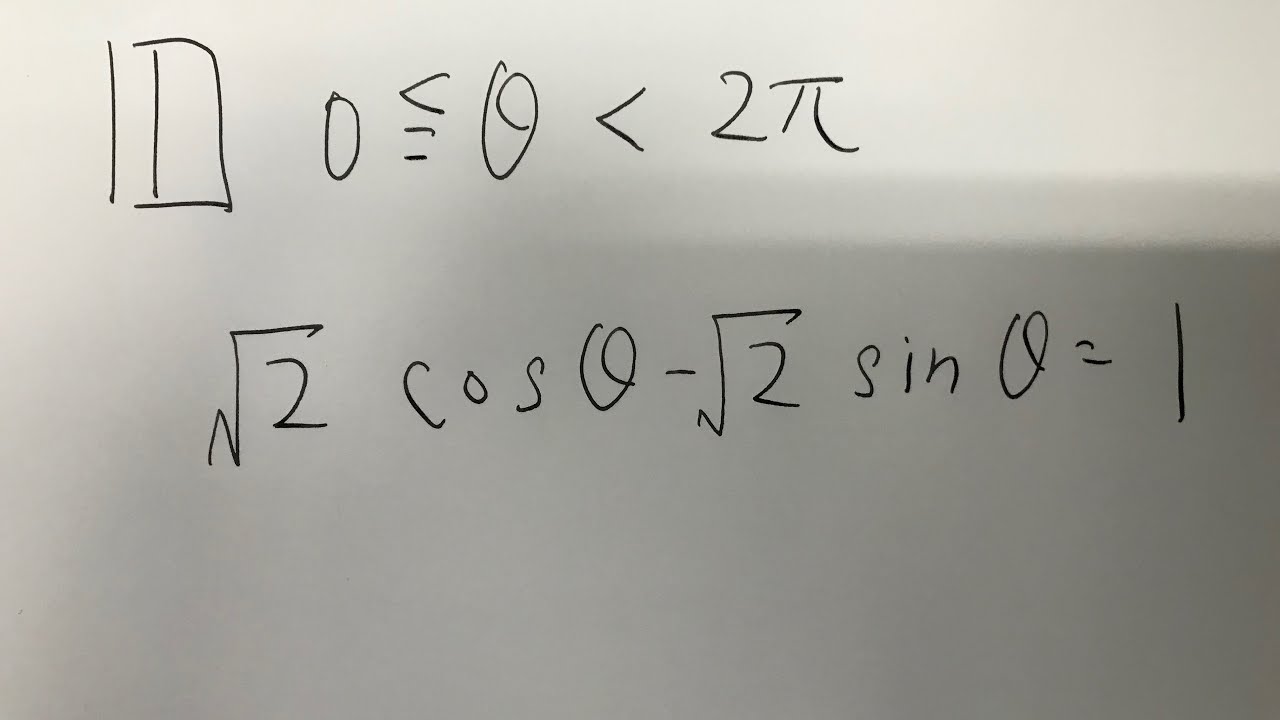
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#三角関数#数学検定#数学検定準1級#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\boxed{1}$
$0\leqq \theta \lt 2\pi$
$\sqrt2 \cos \theta -\sqrt2 \sin \theta=1$
20年5月数学検定準1級1次試験(三角関数)過去問
この動画を見る
$\boxed{1}$
$0\leqq \theta \lt 2\pi$
$\sqrt2 \cos \theta -\sqrt2 \sin \theta=1$
20年5月数学検定準1級1次試験(三角関数)過去問
20年5月数学検定準1級1次試験(三角関数)
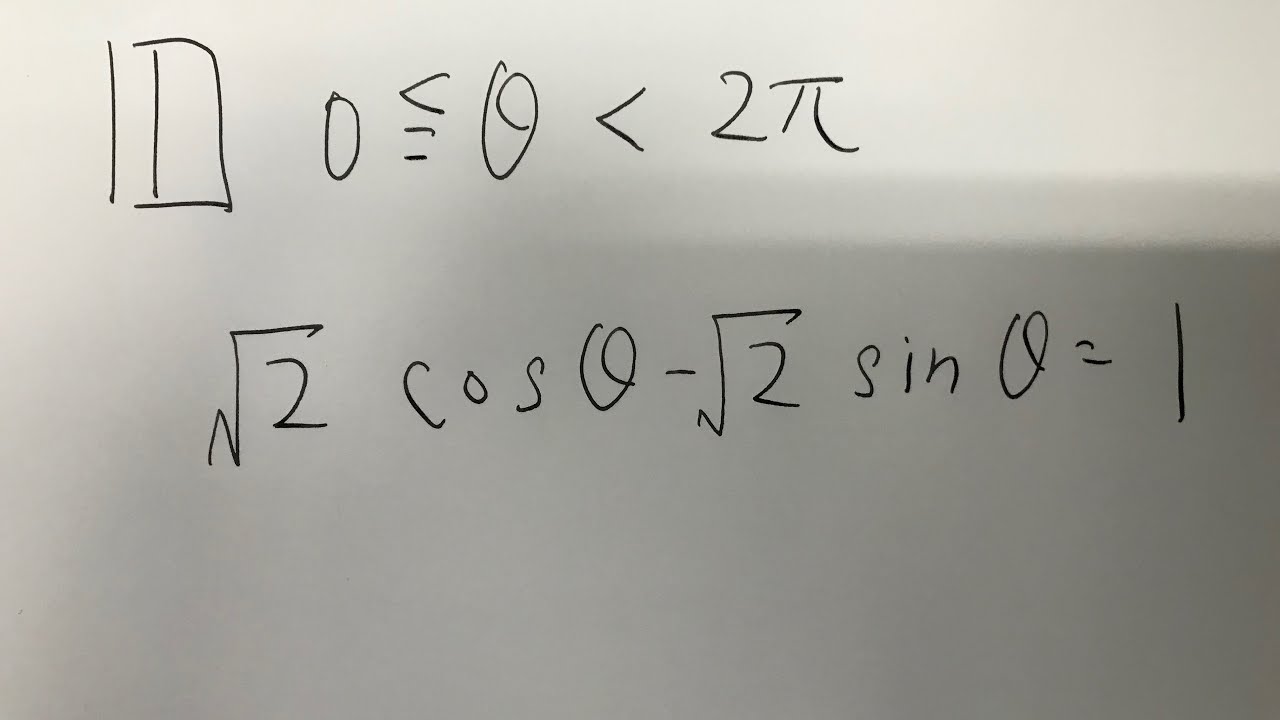
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#三角関数#三角関数とグラフ#数学検定#数学検定準1級#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
1⃣ $0 \leqq θ < 2\pi$
$\sqrt 2 cosθ - \sqrt 2 sinθ = 1$
この動画を見る
1⃣ $0 \leqq θ < 2\pi$
$\sqrt 2 cosθ - \sqrt 2 sinθ = 1$
三角関数の基本 合成公式 図書館情報大

単元:
#数Ⅱ#三角関数#関数と極限#関数(分数関数・無理関数・逆関数と合成関数)#数学(高校生)#数Ⅲ
指導講師:
鈴木貫太郎
問題文全文(内容文):
$\sqrt3\sin 2x+2\sin^2x-1$,$0\leqq x\lt \pi$における最大値,最小値を求めよ.
1985図書館情報大過去問
この動画を見る
$\sqrt3\sin 2x+2\sin^2x-1$,$0\leqq x\lt \pi$における最大値,最小値を求めよ.
1985図書館情報大過去問
三次関数の最大値 微分の基礎 大阪教育大

単元:
#数Ⅱ#三角関数#微分法と積分法#三角関数とグラフ#数学(高校生)
指導講師:
鈴木貫太郎
問題文全文(内容文):
$f(x)=-x^3-3x^2+3kx+3k+2$の$-1\leqq x\leqq 1$における最大値を求めよ.
2008大阪教育大過去問
この動画を見る
$f(x)=-x^3-3x^2+3kx+3k+2$の$-1\leqq x\leqq 1$における最大値を求めよ.
2008大阪教育大過去問
東邦(医)三角関数 最大値

単元:
#数Ⅱ#大学入試過去問(数学)#三角関数#学校別大学入試過去問解説(数学)#数学(高校生)#東邦大学
指導講師:
鈴木貫太郎
問題文全文(内容文):
$2\sin x\cos x+3\sqrt{ 2 }(\cos x+\sin x)$の最大値を求めよ
出典:東邦大学医学部 過去問
この動画を見る
$2\sin x\cos x+3\sqrt{ 2 }(\cos x+\sin x)$の最大値を求めよ
出典:東邦大学医学部 過去問
【高校数学】三角関数のグラフの裏技~これを覚えればグラフは余裕~【数学Ⅱ】

最速。2020年センター試験解説。福田の入試問題解説〜2020年センター試験IIB第1問〜三角関数、指数対数関数、図形と方程式
単元:
#数A#数Ⅱ#大学入試過去問(数学)#図形の性質#図形と方程式#三角関数#指数関数と対数関数#指数関数#センター試験・共通テスト関連#センター試験#数学(高校生)
指導講師:
福田次郎
問題文全文(内容文):
${\large第1問}$
[1](1)$0 \leqq \theta \lt 2\pi$のとき
$\sin\theta \gt \sqrt3\cos\left(\theta-\displaystyle \frac{\pi}{3}\right)$ $\cdots$①
となる$\theta$の値の範囲を求めよう。
加法定理を用いると
$\sqrt3\cos\left(\theta-\frac{\pi}{3}\right)=\displaystyle\frac{\sqrt{\boxed{\ \ ア\ \ }}}{\boxed{\ \ イ\ \ }}\cos\theta+\displaystyle\frac{\boxed{\ \ ウ\ \ }}{\boxed{\ \ イ\ \ }}\sin\theta$
である。よって、三角関数の合成を用いると、①は
$\sin\left(\theta+\displaystyle\frac{\pi}{\boxed{\ \ エ\ \ }}\right) \lt 0$
と変形できる。したがって、求める範囲は
$\displaystyle\frac{\boxed{\ \ オ\ \ }}{\boxed{\ \ カ\ \ }}\pi \lt \theta \lt \frac{\boxed{\ \ キ\ \ }}{\boxed{\ \ ク\ \ }}\pi$
である。
(2)$0 \leqq \theta \leqq \displaystyle\frac{\pi}{2}$とし、$k$を実数とする。$\sin\theta$と$\cos\theta$は$x$の2次方程式
$25x^2-35x+k=0$の解であるとする。このとき、解と係数の関係に
より$\sin\theta+\cos\theta$と$\sin\theta\cos\theta$の値を考えれば、$k=\boxed{\ \ ケコ\ \ }$で
あることがわかる。
さらに、$\theta$が$\sin\theta \geqq \cos\theta$を満たすとすると、$\sin\theta=\displaystyle\frac{\boxed{\ \ サ\ \ }}{\boxed{\ \ シ\ \ }},$
$\cos\theta=\displaystyle\frac{\boxed{\ \ ス\ \ }}{\boxed{\ \ セ\ \ }}$である。このとき、$\theta$は$\boxed{\ \ ソ\ \ }$を満たす。
$\boxed{\ \ ソ\ \ }$に当てはまるものを、次の⓪~⑤のうちから一つ選べ。
⓪$0 \leqq \theta \lt \displaystyle\frac{\pi}{12}$
①$\displaystyle\frac{\pi}{12} \leqq \theta \lt \displaystyle\frac{\pi}{6}$
②$\displaystyle\frac{\pi}{6} \leqq \theta \lt \displaystyle\frac{\pi}{4}$
③$\displaystyle\frac{\pi}{4} \leqq \theta \lt \displaystyle\frac{\pi}{3}$
④$\displaystyle\frac{\pi}{3} \leqq \theta \lt \displaystyle\frac{5}{12}\pi$
⑤$\displaystyle\frac{5}{12}\pi \leqq \theta \leqq \displaystyle\frac{\pi}{2}$
[2](1)$t$は正の実数であり、$t^{\displaystyle\frac{1}{3}}-t^{-\displaystyle\frac{1}{3}}=-3$を満たすとする。このとき
$t^{\displaystyle\frac{2}{3}}+t^{-\displaystyle\frac{2}{3}}=\boxed{\ \ タチ\ \ }$
である。さらに
$t^{\frac{1}{2}}+t^{-\frac{1}{2}}=\sqrt{\boxed{\ \ ツテ\ \ }}, t-t^{-1}=\boxed{\ \ トナニ\ \ }$
である。
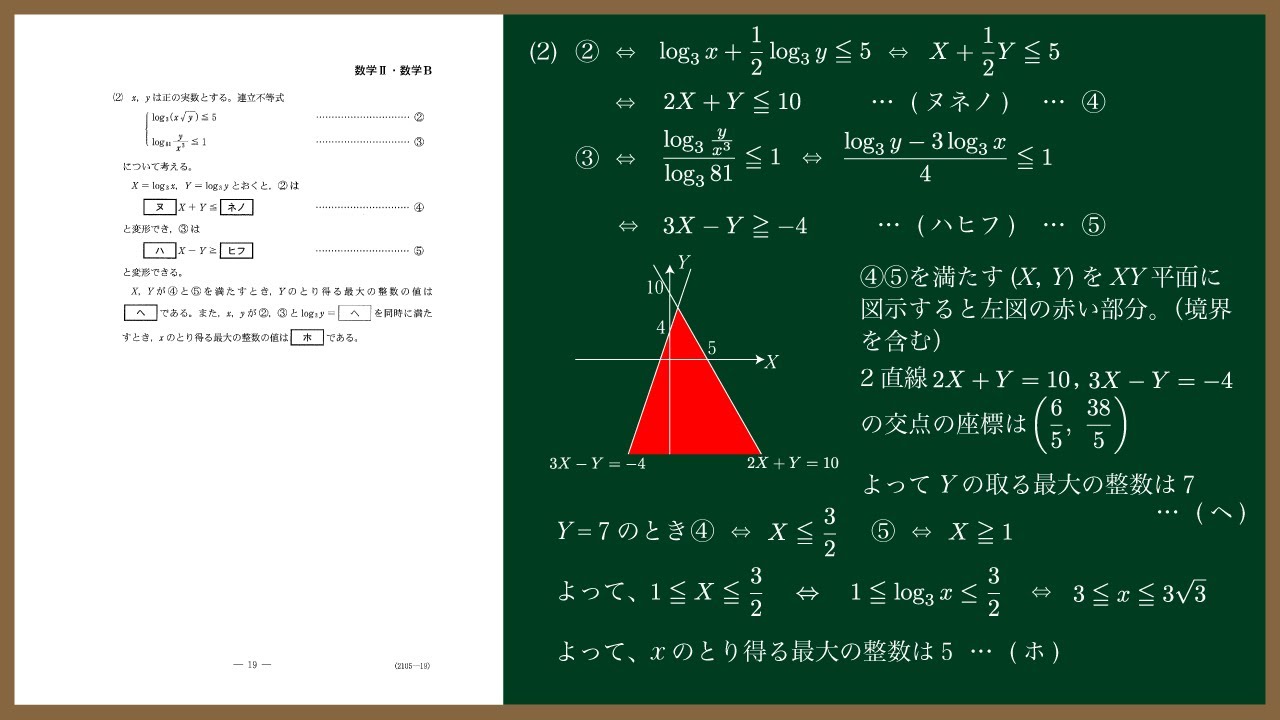
(2)$x,y$は正の実数とする。連立方程式
$\begin{eqnarray}
\left\{
\begin{array}{l}
\log_3(x\sqrt y) \leqq 5 \cdots②\\
\log_{81}\frac{y}{x^3} \leqq 1 \cdots③
\end{array}
\right.
\end{eqnarray}$
について考える。
$X=\log_3x,$ $Y=\log_3y$とおくと、②は
$\boxed{\ \ ヌ\ \ }\ X+Y \leqq \boxed{\ \ ネノ\ \ }$ $\cdots$④
と変形でき、③は
$\boxed{\ \ ハ\ \ }\ X-Y \geqq \boxed{\ \ ヒフ\ \ }$ $\cdots$⑤
と変形できる。
$X,Y$が④と⑤を満たすとき、$Y$の取り得る最大の整数の値は
$\boxed{\ \ ヘ\ \ }$である。また、$x,y$が②,③と$\log_3y=\boxed{\ \ ヘ\ \ }$を同時に
満たすとき、xの取り得る最大の整数の値は$\boxed{\ \ ホ\ \ }$である。
2020センター試験過去問
この動画を見る
${\large第1問}$
[1](1)$0 \leqq \theta \lt 2\pi$のとき
$\sin\theta \gt \sqrt3\cos\left(\theta-\displaystyle \frac{\pi}{3}\right)$ $\cdots$①
となる$\theta$の値の範囲を求めよう。
加法定理を用いると
$\sqrt3\cos\left(\theta-\frac{\pi}{3}\right)=\displaystyle\frac{\sqrt{\boxed{\ \ ア\ \ }}}{\boxed{\ \ イ\ \ }}\cos\theta+\displaystyle\frac{\boxed{\ \ ウ\ \ }}{\boxed{\ \ イ\ \ }}\sin\theta$
である。よって、三角関数の合成を用いると、①は
$\sin\left(\theta+\displaystyle\frac{\pi}{\boxed{\ \ エ\ \ }}\right) \lt 0$
と変形できる。したがって、求める範囲は
$\displaystyle\frac{\boxed{\ \ オ\ \ }}{\boxed{\ \ カ\ \ }}\pi \lt \theta \lt \frac{\boxed{\ \ キ\ \ }}{\boxed{\ \ ク\ \ }}\pi$
である。
(2)$0 \leqq \theta \leqq \displaystyle\frac{\pi}{2}$とし、$k$を実数とする。$\sin\theta$と$\cos\theta$は$x$の2次方程式
$25x^2-35x+k=0$の解であるとする。このとき、解と係数の関係に
より$\sin\theta+\cos\theta$と$\sin\theta\cos\theta$の値を考えれば、$k=\boxed{\ \ ケコ\ \ }$で
あることがわかる。
さらに、$\theta$が$\sin\theta \geqq \cos\theta$を満たすとすると、$\sin\theta=\displaystyle\frac{\boxed{\ \ サ\ \ }}{\boxed{\ \ シ\ \ }},$
$\cos\theta=\displaystyle\frac{\boxed{\ \ ス\ \ }}{\boxed{\ \ セ\ \ }}$である。このとき、$\theta$は$\boxed{\ \ ソ\ \ }$を満たす。
$\boxed{\ \ ソ\ \ }$に当てはまるものを、次の⓪~⑤のうちから一つ選べ。
⓪$0 \leqq \theta \lt \displaystyle\frac{\pi}{12}$
①$\displaystyle\frac{\pi}{12} \leqq \theta \lt \displaystyle\frac{\pi}{6}$
②$\displaystyle\frac{\pi}{6} \leqq \theta \lt \displaystyle\frac{\pi}{4}$
③$\displaystyle\frac{\pi}{4} \leqq \theta \lt \displaystyle\frac{\pi}{3}$
④$\displaystyle\frac{\pi}{3} \leqq \theta \lt \displaystyle\frac{5}{12}\pi$
⑤$\displaystyle\frac{5}{12}\pi \leqq \theta \leqq \displaystyle\frac{\pi}{2}$
[2](1)$t$は正の実数であり、$t^{\displaystyle\frac{1}{3}}-t^{-\displaystyle\frac{1}{3}}=-3$を満たすとする。このとき
$t^{\displaystyle\frac{2}{3}}+t^{-\displaystyle\frac{2}{3}}=\boxed{\ \ タチ\ \ }$
である。さらに
$t^{\frac{1}{2}}+t^{-\frac{1}{2}}=\sqrt{\boxed{\ \ ツテ\ \ }}, t-t^{-1}=\boxed{\ \ トナニ\ \ }$
である。
(2)$x,y$は正の実数とする。連立方程式
$\begin{eqnarray}
\left\{
\begin{array}{l}
\log_3(x\sqrt y) \leqq 5 \cdots②\\
\log_{81}\frac{y}{x^3} \leqq 1 \cdots③
\end{array}
\right.
\end{eqnarray}$
について考える。
$X=\log_3x,$ $Y=\log_3y$とおくと、②は
$\boxed{\ \ ヌ\ \ }\ X+Y \leqq \boxed{\ \ ネノ\ \ }$ $\cdots$④
と変形でき、③は
$\boxed{\ \ ハ\ \ }\ X-Y \geqq \boxed{\ \ ヒフ\ \ }$ $\cdots$⑤
と変形できる。
$X,Y$が④と⑤を満たすとき、$Y$の取り得る最大の整数の値は
$\boxed{\ \ ヘ\ \ }$である。また、$x,y$が②,③と$\log_3y=\boxed{\ \ ヘ\ \ }$を同時に
満たすとき、xの取り得る最大の整数の値は$\boxed{\ \ ホ\ \ }$である。
2020センター試験過去問
光文社新書「中学の知識でオイラー公式がわかる」Vol 4 加法定理

光文社新書「中学の知識でオイラーの公式がわかる」Vol.2三角関数

