 不定積分
不定積分
 不定積分
不定積分
【数Ⅲ】【積分とその応用】不定積分置換積分、部分積分3 ※問題文は概要欄
単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
教材:
#4S数学#4S数学ⅢのB問題解説#中高教材#積分法の応用
指導講師:
理数個別チャンネル
問題文全文(内容文):
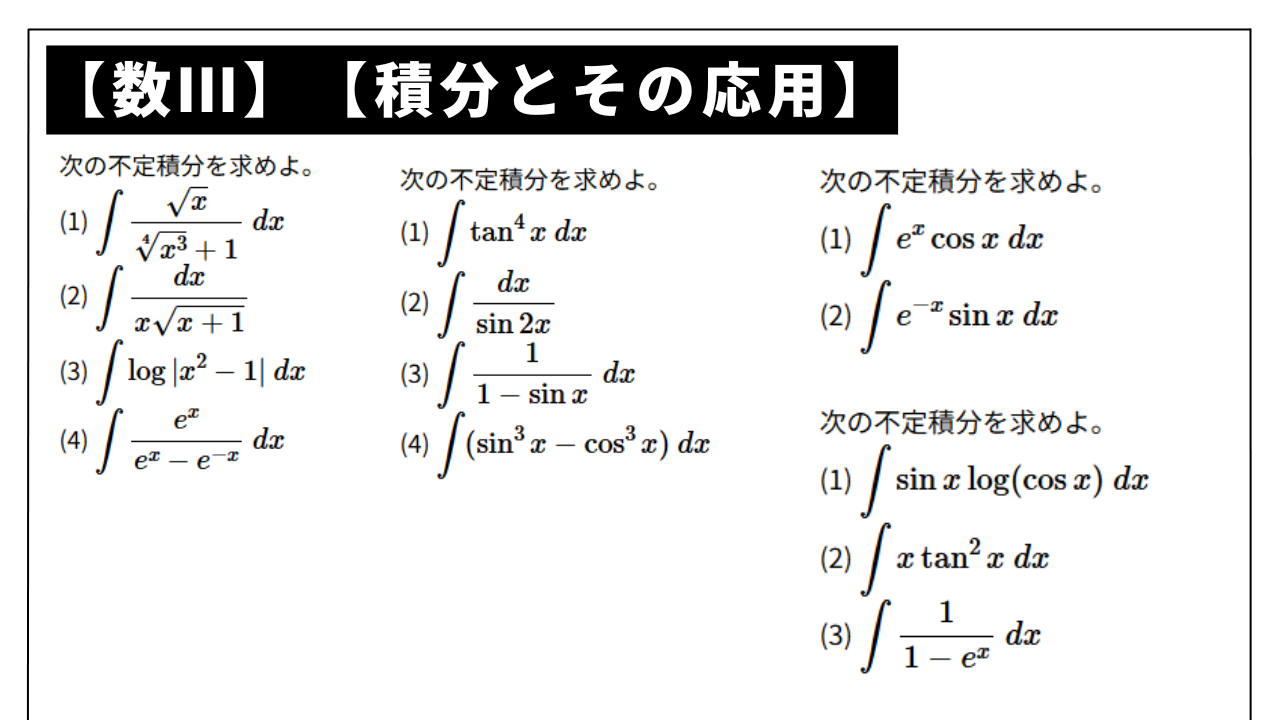
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
次の不定積分を求めよ。
(1)
(2)
次の不定積分を求めよ。
(1)
(2)
(3)
この動画を見る
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
次の不定積分を求めよ。
(1)
(2)
次の不定積分を求めよ。
(1)
(2)
(3)
【数Ⅲ】【積分とその応用】不定積分置換積分、部分積分2 ※問題文は概要欄

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
教材:
#4S数学#4S数学ⅢのB問題解説#中高教材#積分法の応用
指導講師:
理数個別チャンネル
問題文全文(内容文):
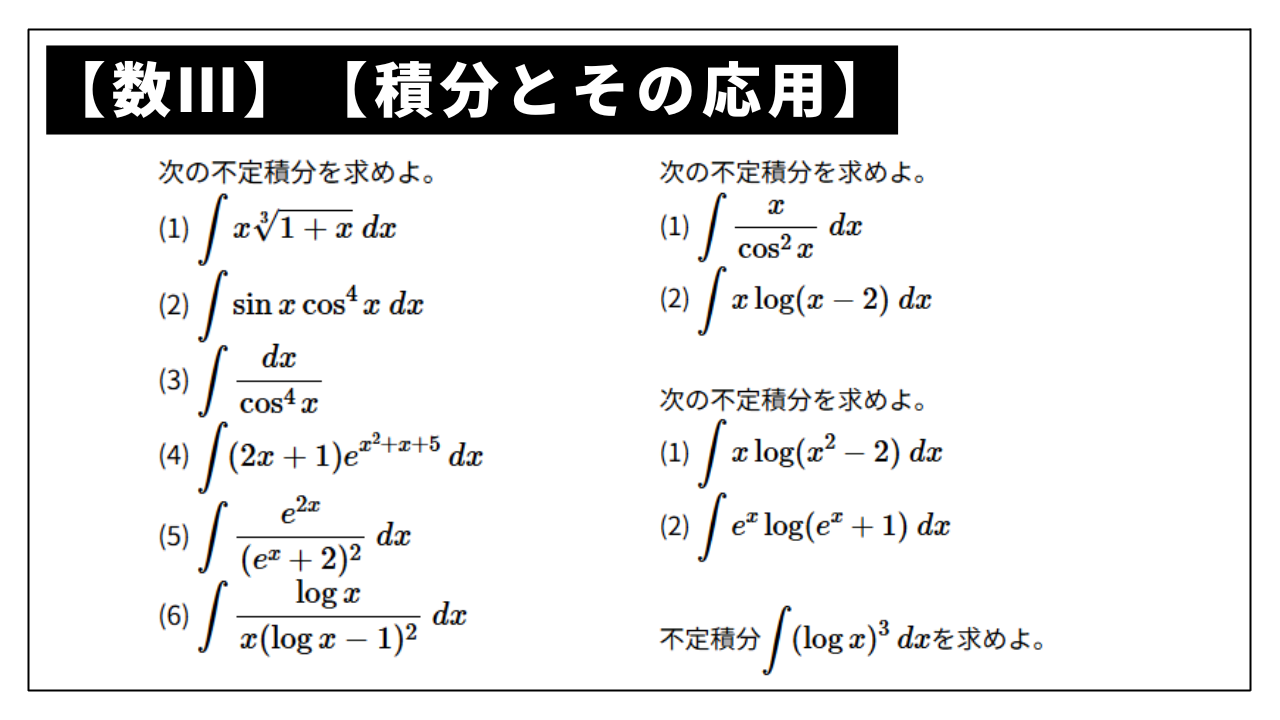
次の不定積分を求めよ。
(1)
(2)
(1)次の等式が成り立つように、定数
(2)不定積分
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
(5)
(6)
次の不定積分を求めよ。
(1)
(2)
この動画を見る
次の不定積分を求めよ。
(1)
(2)
(1)次の等式が成り立つように、定数
(2)不定積分
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
(5)
(6)
次の不定積分を求めよ。
(1)
(2)
【数Ⅲ】【積分とその応用】不定積分置換積分、部分積分1 ※問題文は概要欄
単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
教材:
#4S数学#4S数学ⅢのB問題解説#中高教材#積分法の応用
指導講師:
理数個別チャンネル
問題文全文(内容文):
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
(5)
(6)
次の不定積分を求めよ。
(1)
(2)
次の不定積分を求めよ。
(1)
(2)
不定積分
この動画を見る
次の不定積分を求めよ。
(1)
(2)
(3)
(4)
(5)
(6)
次の不定積分を求めよ。
(1)
(2)
次の不定積分を求めよ。
(1)
(2)
不定積分
#広島市立大学2024#不定積分_29#元高校教員
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
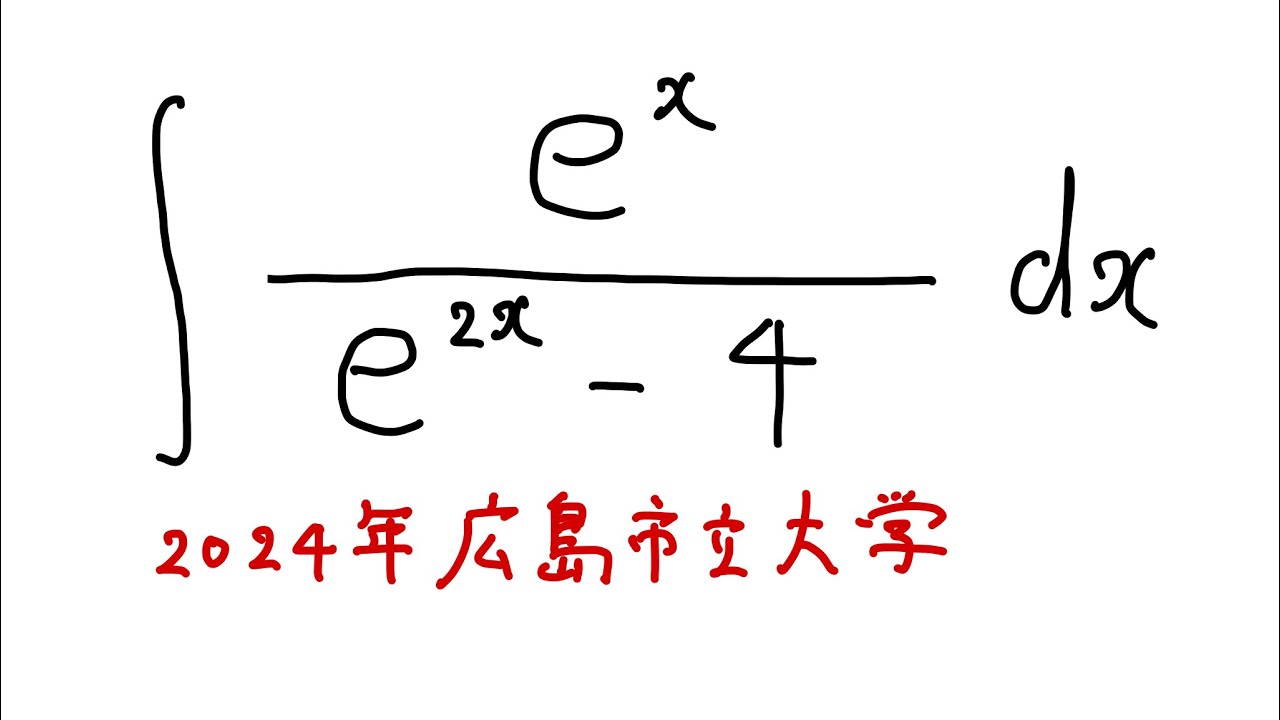
出典:2024年広島市立大学
この動画を見る
出典:2024年広島市立大学
#高専#不定積分_19#元高専教員
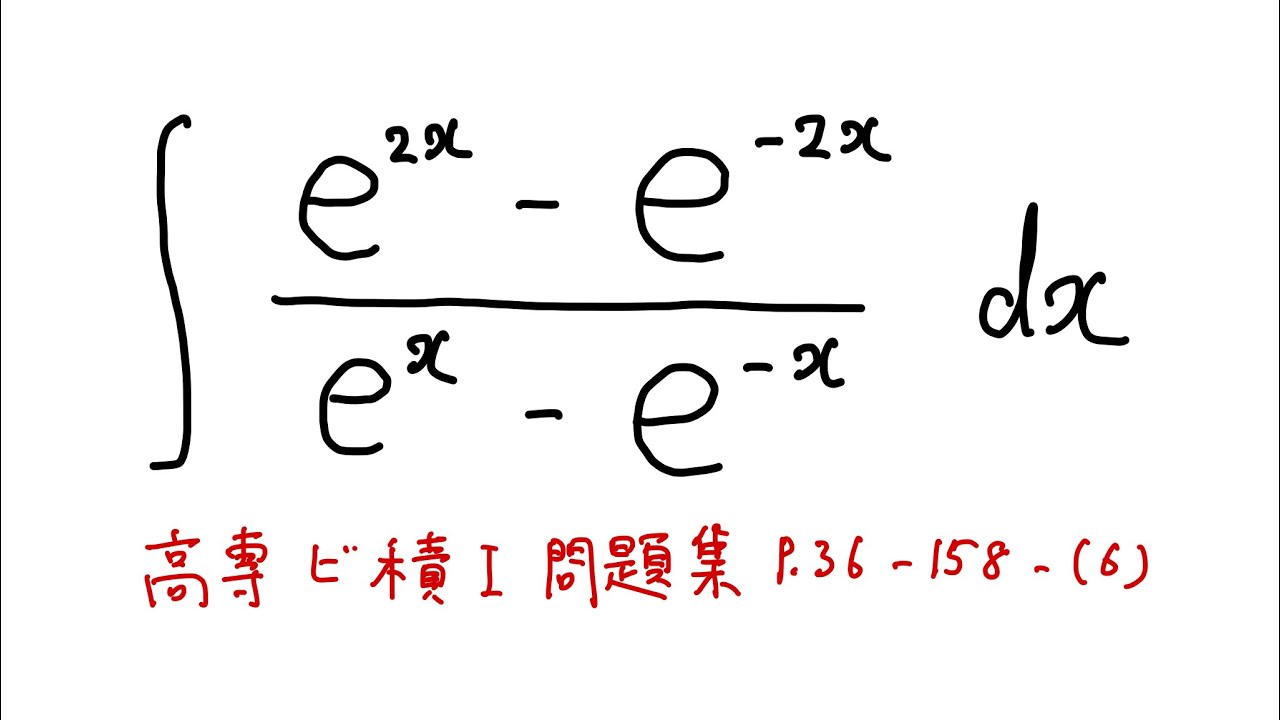
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#高専(高等専門学校)
指導講師:
ますただ
問題文全文(内容文):
出典:国立高等専門学校機構
この動画を見る
出典:国立高等専門学校機構
#高専#不定積分_18#元高専教員

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#高専(高等専門学校)
指導講師:
ますただ
問題文全文(内容文):
出典:国立高等専門学校機構
この動画を見る
出典:国立高等専門学校機構
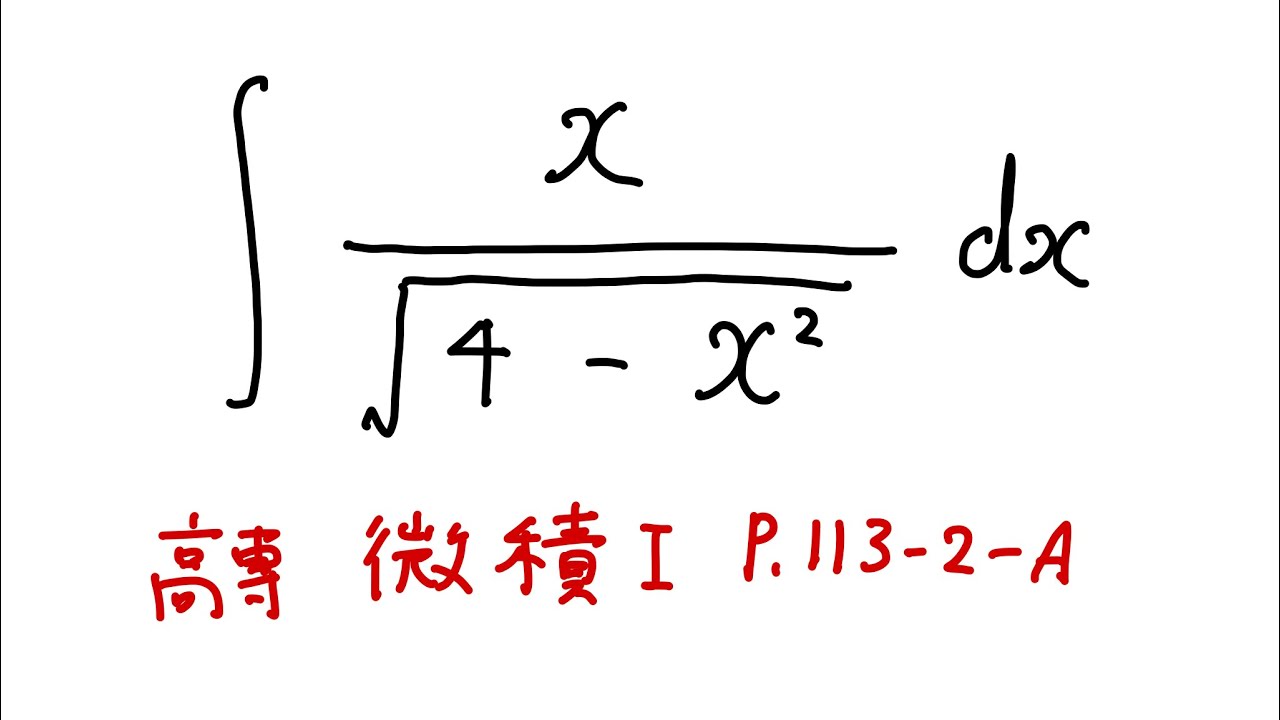
#高専#不定積分_17#元高専教員
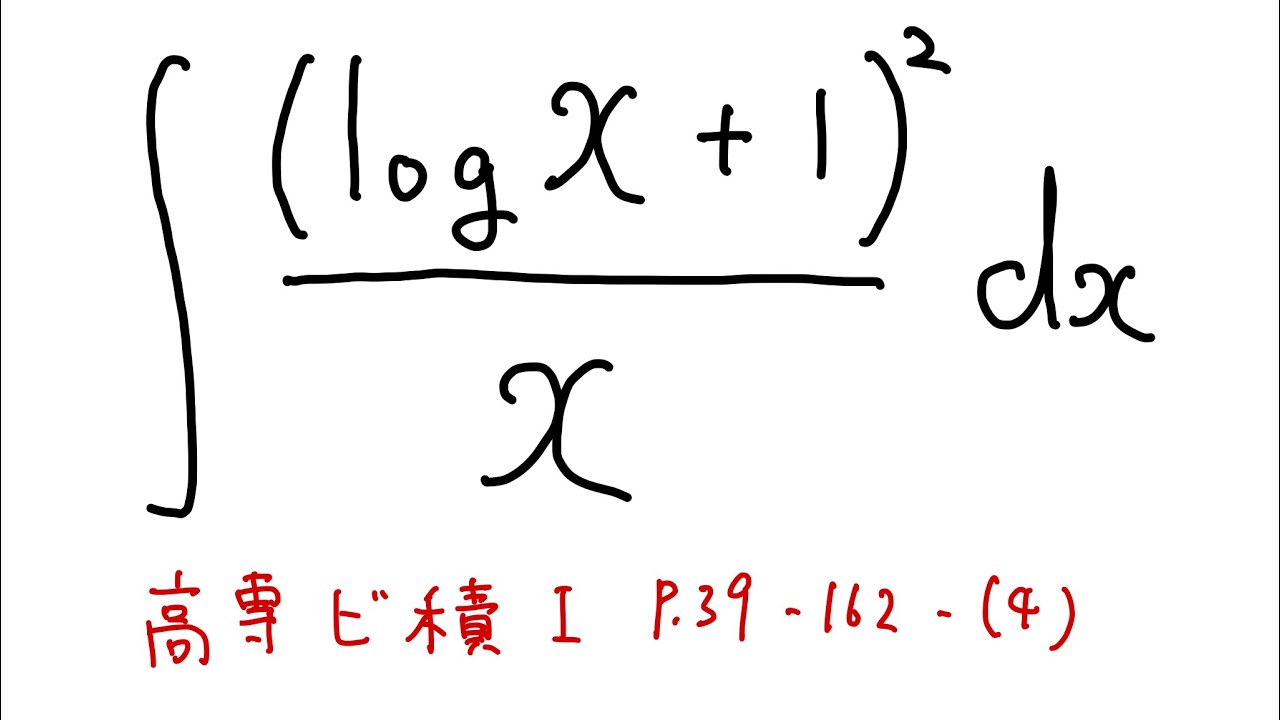
#高専#不定積分_16#元高専教員

#高知工科大学2024#不定積分_23#元高校教員
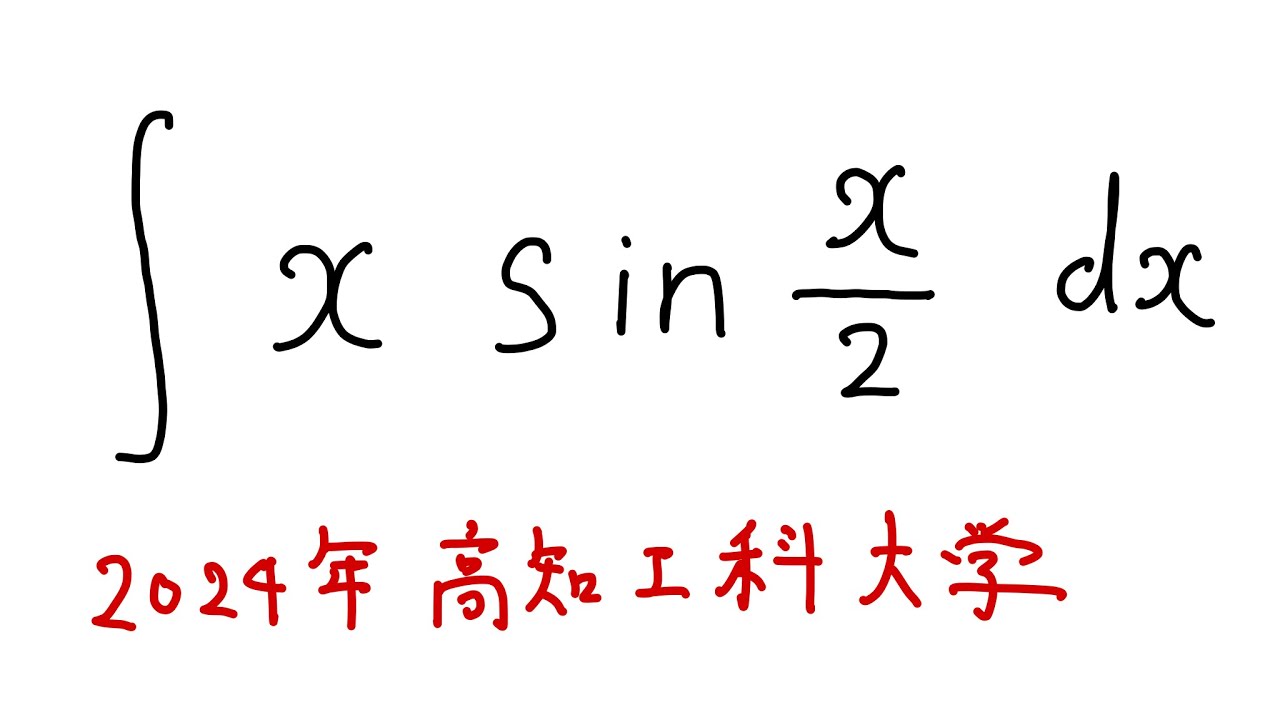
#高専#ウォリス積分_15#元高専教員
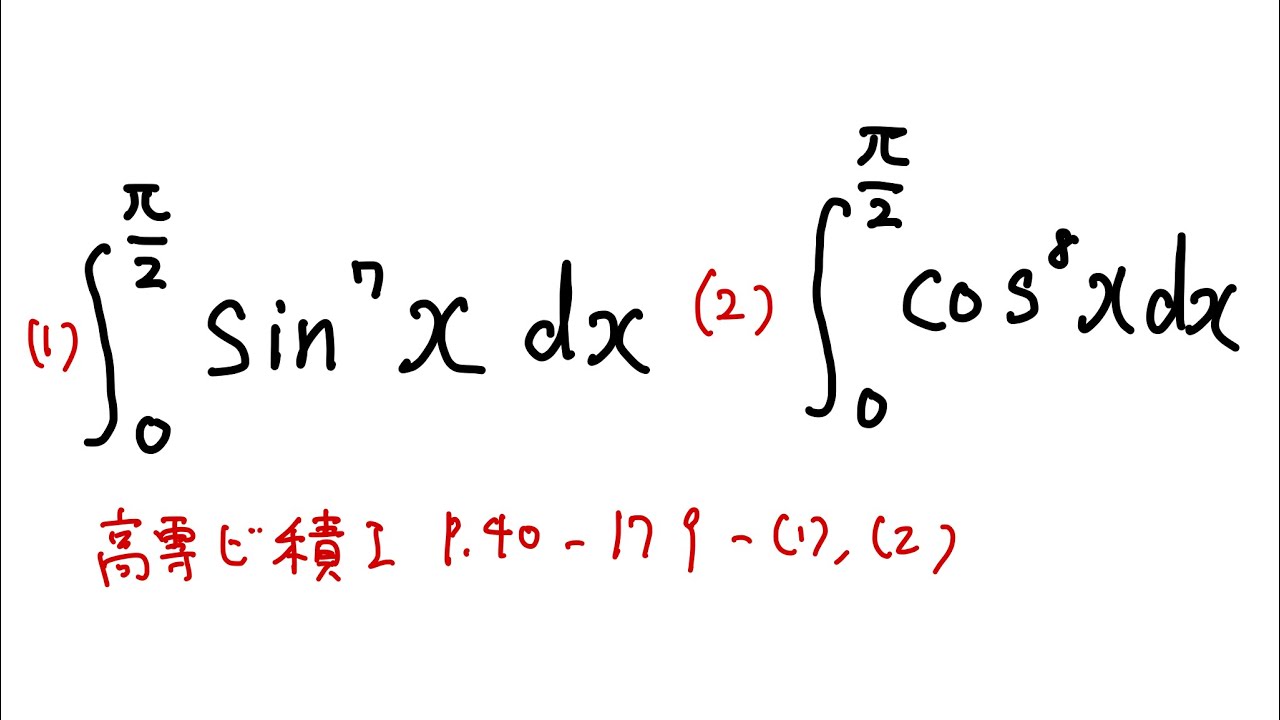
#広島市立大学2024#不定積分_22#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
出典:2024年広島市立大学後期 不定積分問題
この動画を見る
出典:2024年広島市立大学後期 不定積分問題
大学入試問題#915「減点祭りの問題」 #京都大学1965 #積分方程式

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:1965年京都大学
この動画を見る
出典:1965年京都大学
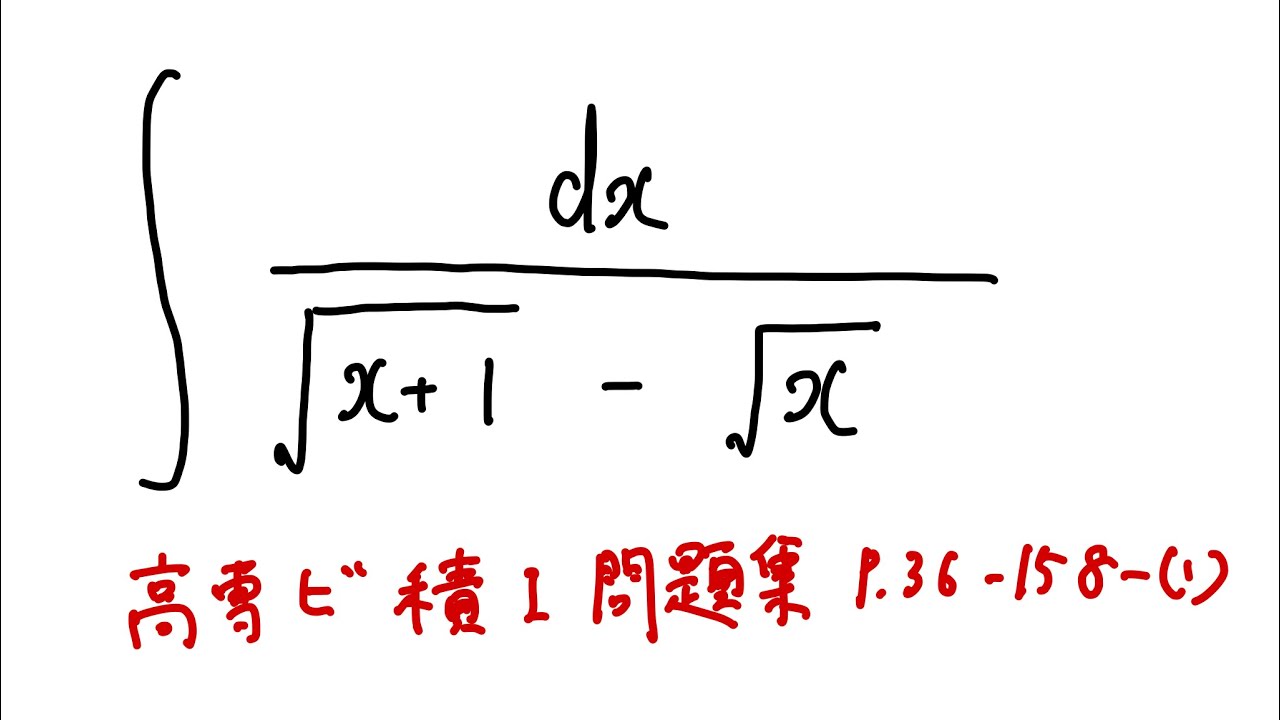
#高専数学#不定積分_13#元高専教員
#宮崎大学2024#不定積分_20#元高校教員
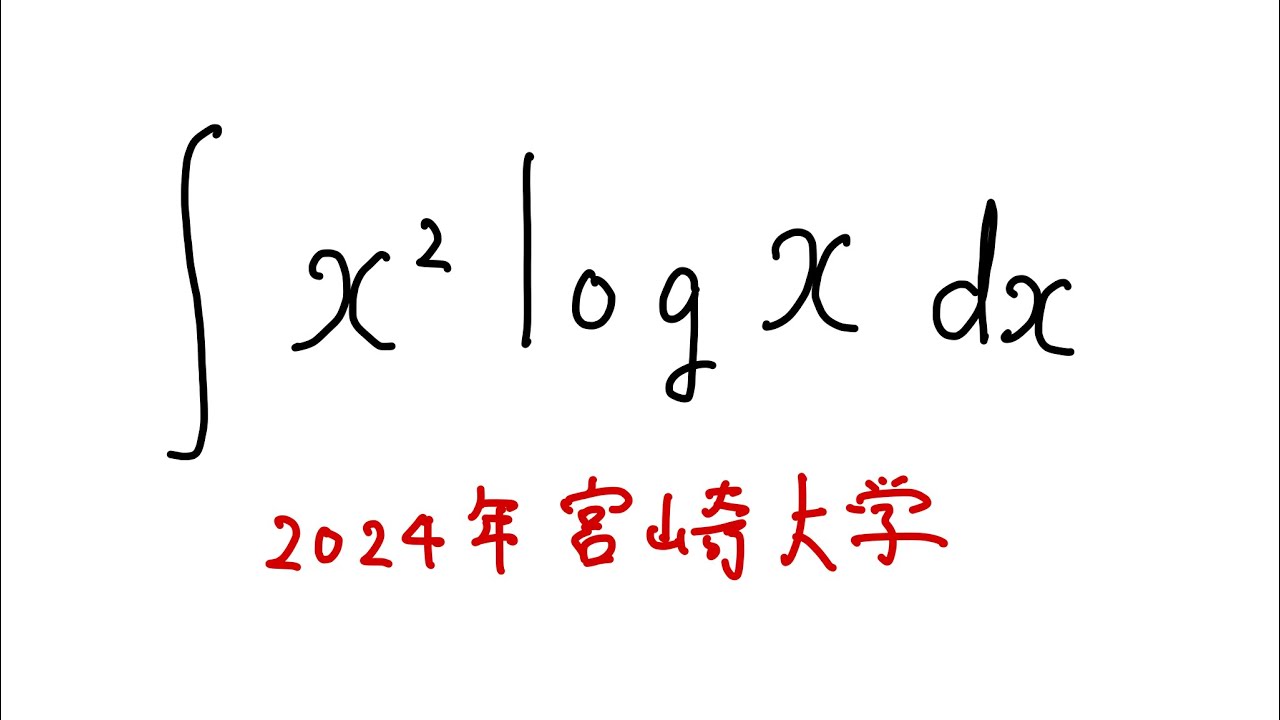
#宮崎大学2024#不定積分_19#元高校教員
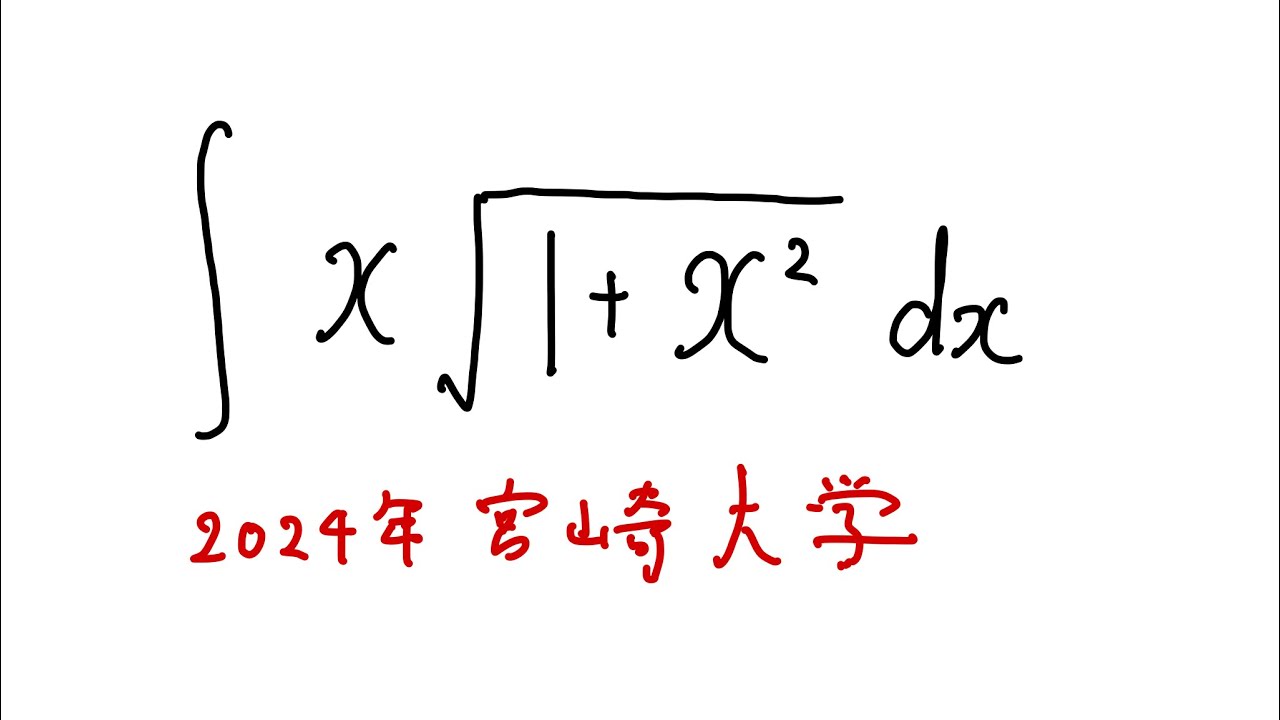
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#宮崎大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2024年宮崎大学
この動画を見る
出典:2024年宮崎大学
#名古屋工業大学2024#不定積分_18#元高校教員

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#名古屋大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2024年 名古屋工業大学
この動画を見る
出典:2024年 名古屋工業大学
どゆこと?
#高専数学#不定積分_12#元高専教員
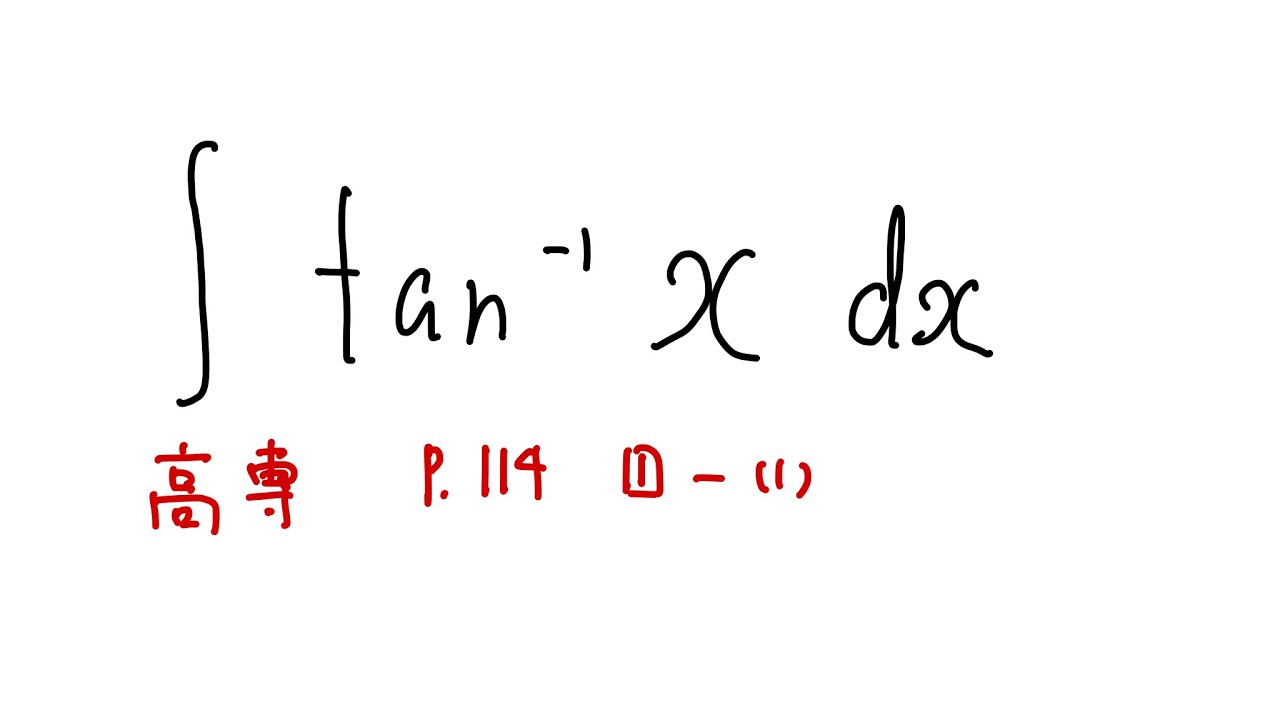
#高専数学_10#不定積分#元高専教員

#高専数学_9#不定積分#元高専教員

#高専_8#不定積分#元高専教員
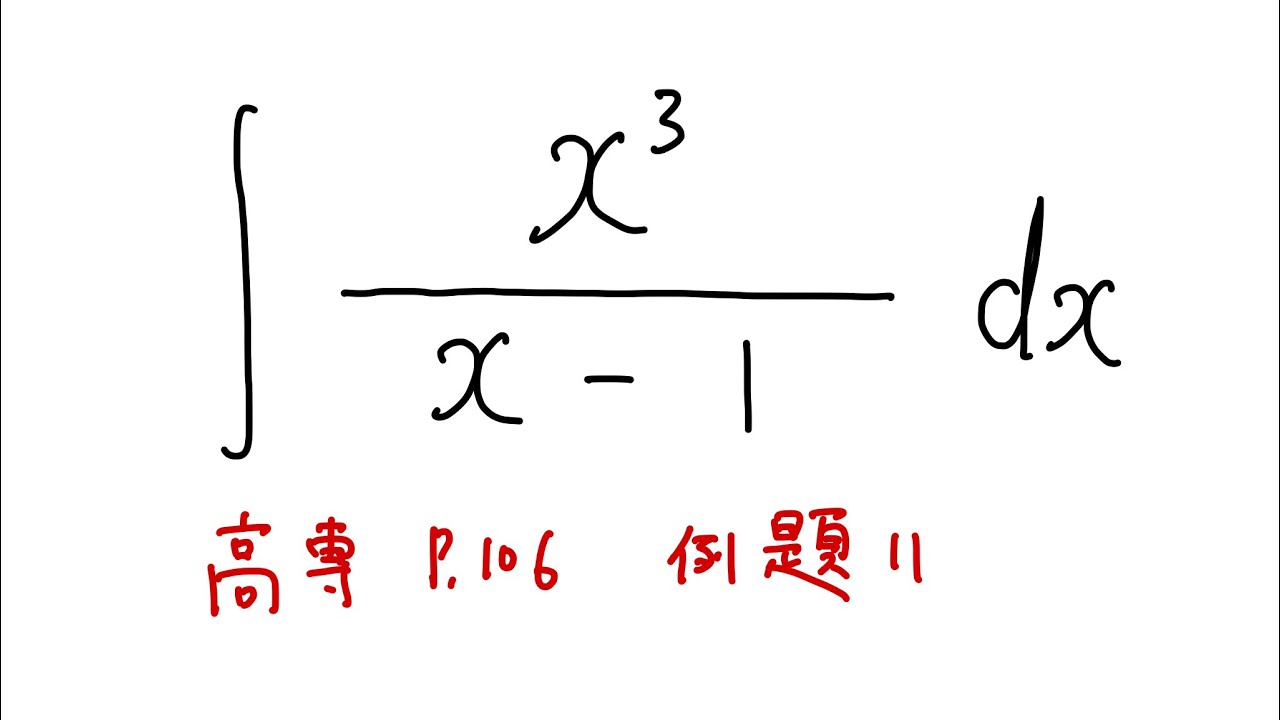
#千葉大学2021#不定積分#元高専教員
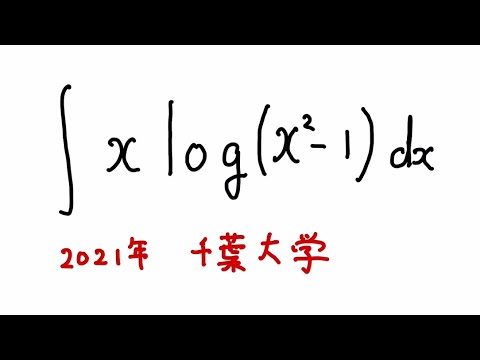
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
以下の不定積分を解け。
出典:2021年千葉大学
この動画を見る
以下の不定積分を解け。
出典:2021年千葉大学
#千葉大学2018#不定積分#数学者
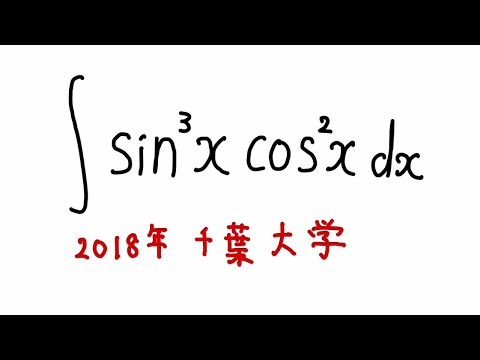
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
以下の不定積分を解け。
出典:2018年千葉大学
この動画を見る
以下の不定積分を解け。
出典:2018年千葉大学
#高専_6#不定積分#元高専教員

#高専数学_5#不定積分#元高専教員

#千葉大学2020#不定積分#ますただ
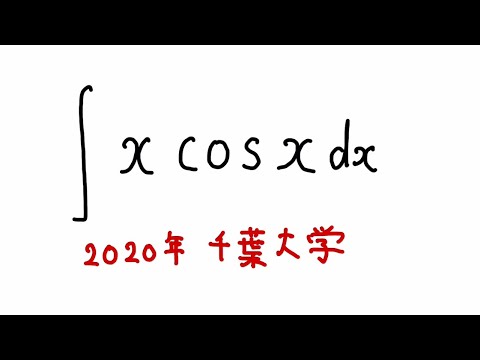
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2024年千葉大学
この動画を見る
出典:2024年千葉大学
#高専_4#不定積分#元高専教員

#高専#不定積分-1
#広島市立大学#不定積分#ますただ
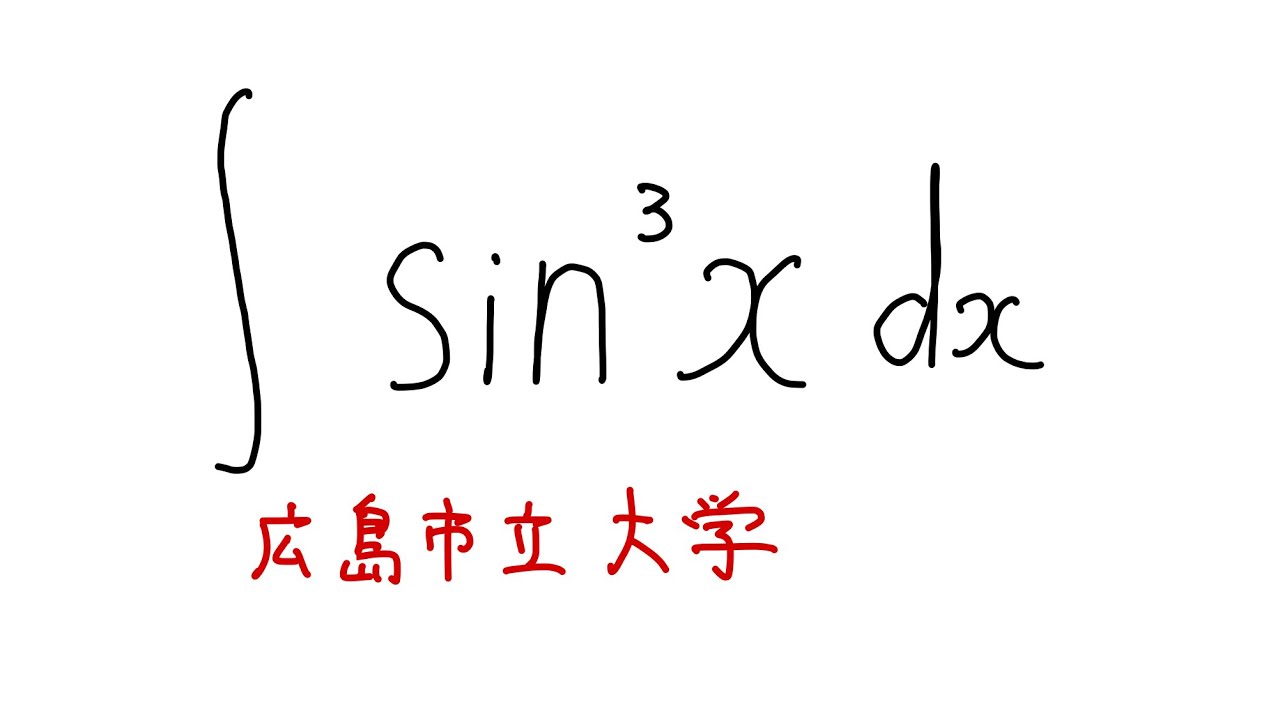
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
広島市立大過去問
この動画を見る
広島市立大過去問
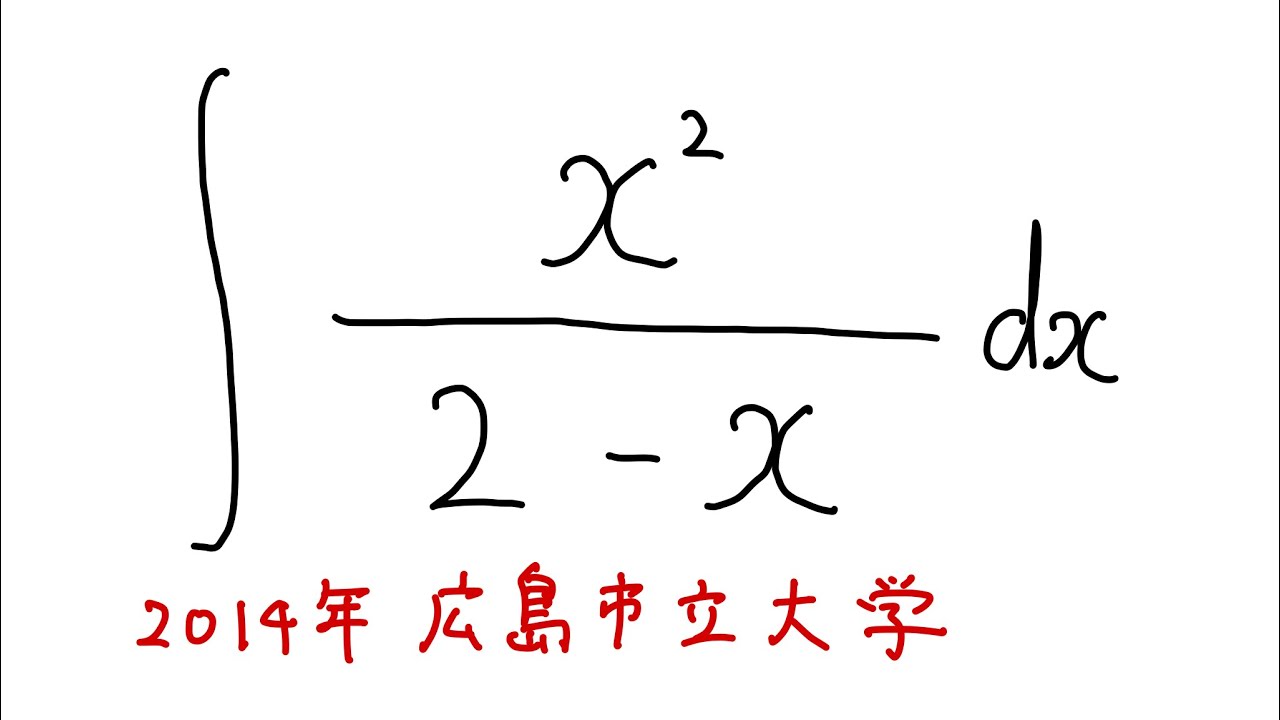
#広島市立大学2014#不定積分#ますただ
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
出典:2014年広島市立大学
この動画を見る
出典:2014年広島市立大学
