 定積分
定積分
 定積分
定積分
大学入試問題#402「答えが透けてみえそう」 山形大学(2015) #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{3} (x-1)(x-2)(x-3) dx$
出典:2015年山形大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{3} (x-1)(x-2)(x-3) dx$
出典:2015年山形大学 入試問題
大学入試問題#401「よくあるセットメニュー」 富山県立大学(2012) #定積分 #極限

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$p \lt 0$
$\displaystyle \lim_{ p \to -\infty } \displaystyle \int_{p}^{0} \displaystyle \frac{3}{1+2e^{-x}} dx$
出典:2012年富山県立大学 入試問題
この動画を見る
$p \lt 0$
$\displaystyle \lim_{ p \to -\infty } \displaystyle \int_{p}^{0} \displaystyle \frac{3}{1+2e^{-x}} dx$
出典:2012年富山県立大学 入試問題
大学入試問題#400「使いたくないけど・・・・」三重大学医学部2009 #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}} \displaystyle \frac{d\theta}{1+\sin\theta-\cos\theta}$
出典:2009年三重大学医学部 入試問題
この動画を見る
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}} \displaystyle \frac{d\theta}{1+\sin\theta-\cos\theta}$
出典:2009年三重大学医学部 入試問題
福田の1.5倍速演習〜合格する重要問題032〜千葉大学2016年度理系第8問〜不等式の証明

単元:
#数Ⅱ#大学入試過去問(数学)#式と証明#恒等式・等式・不等式の証明#微分とその応用#積分とその応用#微分法#定積分#学校別大学入試過去問解説(数学)#千葉大学#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
以下の問いに答えよ。
(1)$x \gt 0$において、不等式$\log x \lt x $を示せ。
(2)$1 \lt a \lt b$のとき、不等式
$\frac{1}{\log a}-\frac{1}{\log b} \lt \frac{b-a}{a(\log a)^2}$
を示せ。
(3)$x \geqq e$において、不等式
$\int_e^x\frac{dt}{t\log(t+1)} \geqq \log(\log x)+\frac{1}{2(\log x)^2}-\frac{1}{2}$
を示せ。ただし、eは自然対数の底である。
2016千葉大学理系過去問
この動画を見る
以下の問いに答えよ。
(1)$x \gt 0$において、不等式$\log x \lt x $を示せ。
(2)$1 \lt a \lt b$のとき、不等式
$\frac{1}{\log a}-\frac{1}{\log b} \lt \frac{b-a}{a(\log a)^2}$
を示せ。
(3)$x \geqq e$において、不等式
$\int_e^x\frac{dt}{t\log(t+1)} \geqq \log(\log x)+\frac{1}{2(\log x)^2}-\frac{1}{2}$
を示せ。ただし、eは自然対数の底である。
2016千葉大学理系過去問
大学入試問題#399「なんだこりゃ!」 東京商船大学 #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\pi} \sin(\pi\ \cos\ x) dx$
出典:東京商船大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\pi} \sin(\pi\ \cos\ x) dx$
出典:東京商船大学 入試問題
大学入試問題#398「あえての正面突破!!」 京都教育大学2009 #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{4}} log(1+\tan\ x) dx$
出典:2009年京都教育大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}} log(1+\tan\ x) dx$
出典:2009年京都教育大学 入試問題
大学入試問題#395「使う技は、関数から・・・」 大阪市立大学2009 #極限 誘導は概要欄

単元:
#関数と極限#積分とその応用#関数の極限#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
(1)
$0 \leqq x \leqq \displaystyle \frac{\pi}{2}$のとき
$\sin\ x \geqq \displaystyle \frac{2}{\pi}x$を示せ
(2)
$\displaystyle \lim_{ n \to \infty } \displaystyle \int_{0}^{\frac{\pi}{2}} e^{-n\ \sin\ x}dx=0$を示せ
出典:2009年大阪市立大学 入試問題
この動画を見る
(1)
$0 \leqq x \leqq \displaystyle \frac{\pi}{2}$のとき
$\sin\ x \geqq \displaystyle \frac{2}{\pi}x$を示せ
(2)
$\displaystyle \lim_{ n \to \infty } \displaystyle \int_{0}^{\frac{\pi}{2}} e^{-n\ \sin\ x}dx=0$を示せ
出典:2009年大阪市立大学 入試問題
福田の1.5倍速演習〜合格する重要問題027〜神戸大学2016年度理系数学第3問〜2曲線の相接条件と回転体の体積

単元:
#大学入試過去問(数学)#微分とその応用#積分とその応用#微分法#定積分#学校別大学入試過去問解説(数学)#神戸大学#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
aを正の定数とし、2曲線$C_1:y=\log x,C_2:y=ax^2$が点Pで接している。
以下の問いに答えよ。
(1)Pの座標とaの値を求めよ。
(2)2曲線$C_1,C_2$とx軸で囲まれた部分をx軸のまわりに1回転させてできる
立体の体積を求めよ。
2016神戸大学理系過去問
この動画を見る
aを正の定数とし、2曲線$C_1:y=\log x,C_2:y=ax^2$が点Pで接している。
以下の問いに答えよ。
(1)Pの座標とaの値を求めよ。
(2)2曲線$C_1,C_2$とx軸で囲まれた部分をx軸のまわりに1回転させてできる
立体の体積を求めよ。
2016神戸大学理系過去問
福田の1.5倍速演習〜合格する重要問題026〜神戸大学2016年度理系数学第5問〜極方程式と媒介変数表示

単元:
#数Ⅱ#大学入試過去問(数学)#複素数と方程式#平面上の曲線#剰余の定理・因数定理・組み立て除法と高次方程式#微分とその応用#積分とその応用#微分法#定積分#学校別大学入試過去問解説(数学)#媒介変数表示と極座標#神戸大学#数学(高校生)#数C#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
極方程式で表されたxy平面上の曲線$r=1+\cos\theta(0 \leqq \theta \leqq 2\pi)$をCとする。
(1)曲線C上の点を直交座標(x,y)で表したとき、$\frac{dx}{d\theta}=0$となる点、および
$\frac{dy}{d\theta}=0$となる点の直交座標を求めよ。
(2)$\lim_{\theta \to \pi}\frac{dy}{dx}$を求めよ。
(3)曲線Cの概形をxy平面上にかけ。
(4)曲線Cの長さを求めよ。
2016神戸大学理系過去問
この動画を見る
極方程式で表されたxy平面上の曲線$r=1+\cos\theta(0 \leqq \theta \leqq 2\pi)$をCとする。
(1)曲線C上の点を直交座標(x,y)で表したとき、$\frac{dx}{d\theta}=0$となる点、および
$\frac{dy}{d\theta}=0$となる点の直交座標を求めよ。
(2)$\lim_{\theta \to \pi}\frac{dy}{dx}$を求めよ。
(3)曲線Cの概形をxy平面上にかけ。
(4)曲線Cの長さを求めよ。
2016神戸大学理系過去問
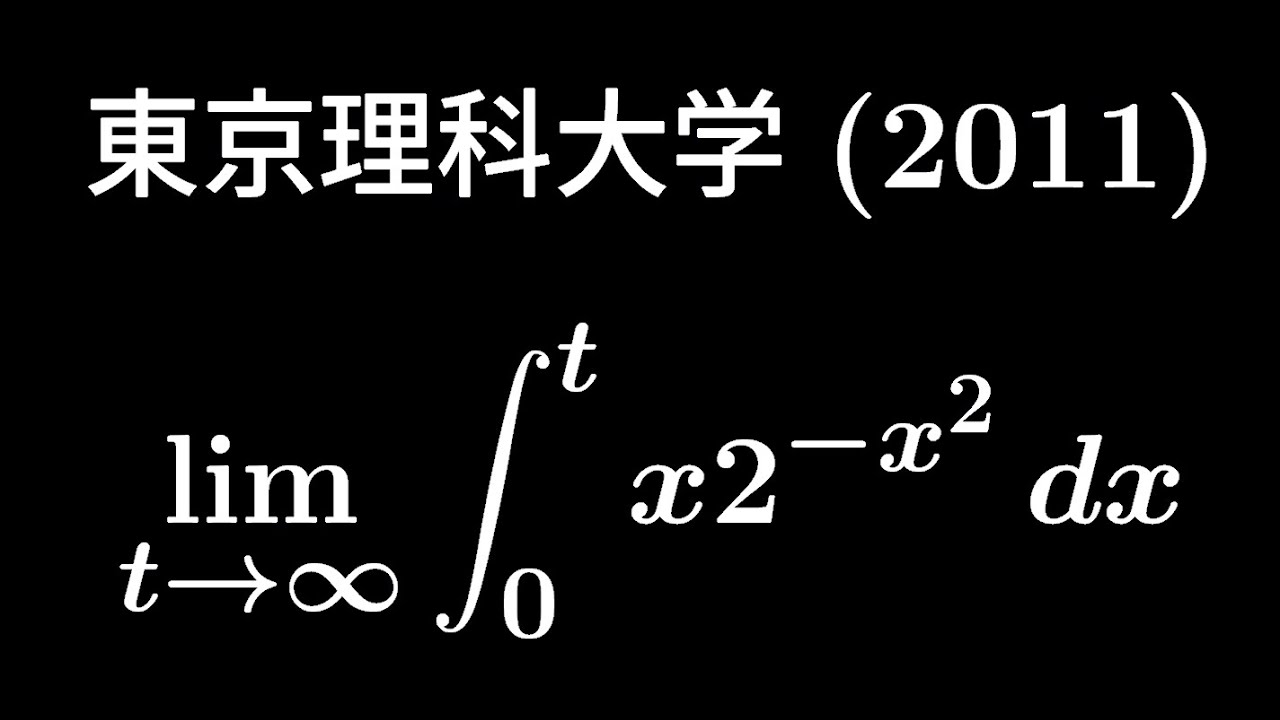
大学入試問題#392「よく見る積分!!!」 #東京理科大学2011 #定積分 #極限
単元:
#大学入試過去問(数学)#関数と極限#積分とその応用#関数の極限#定積分#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ t \to \infty } \displaystyle \int_{0}^{t} x\ 2^{-x^2} dx$
出典:2011年東京理科大学 入試問題
この動画を見る
$\displaystyle \lim_{ t \to \infty } \displaystyle \int_{0}^{t} x\ 2^{-x^2} dx$
出典:2011年東京理科大学 入試問題
福田の1.5倍速演習〜合格する重要問題025〜大阪大学2016年度理系数学第3問〜回転体の体積

単元:
#数Ⅱ#大学入試過去問(数学)#図形と方程式#積分とその応用#定積分#学校別大学入試過去問解説(数学)#大阪大学#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
座標平面において、原点Oを中心とする半径rの円と放物線$y=\sqrt2(x-1)^2$
は、ただ1つの共有点(a,b)をもつとする。
(1)a,b,rの値をそれぞれ求めよ。
(2)連立不等式
$a \leqq x \leqq 1, 0 \leqq y \leqq \sqrt2(x-1)^2, x^2+y^2 \geqq r^2$
の表す領域をx軸のまわりに1回転してできる回転体の体積を求めよ。
2016大阪大学理系過去問
この動画を見る
座標平面において、原点Oを中心とする半径rの円と放物線$y=\sqrt2(x-1)^2$
は、ただ1つの共有点(a,b)をもつとする。
(1)a,b,rの値をそれぞれ求めよ。
(2)連立不等式
$a \leqq x \leqq 1, 0 \leqq y \leqq \sqrt2(x-1)^2, x^2+y^2 \geqq r^2$
の表す領域をx軸のまわりに1回転してできる回転体の体積を求めよ。
2016大阪大学理系過去問
大学入試問題#391「正面突破が王道だと思いますが、あえて」 東北学院大学(2009) #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\pi} (\sin^3x-\cos^3x) dx$
出典:2009年東北学院大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\pi} (\sin^3x-\cos^3x) dx$
出典:2009年東北学院大学 入試問題
大学入試問題#390「一瞬aが実数でドキッとするが・・・」 慶應義塾大学2011 #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$a \gt 0$
$\displaystyle \int_{0}^{a} x^2(1-\displaystyle \frac{x}{a})^a dx$
出典:2011年慶應義塾大学 入試問題
この動画を見る
$a \gt 0$
$\displaystyle \int_{0}^{a} x^2(1-\displaystyle \frac{x}{a})^a dx$
出典:2011年慶應義塾大学 入試問題
大学入試問題#389「基本問題」 #茨城大学(2009) #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\pi} x\ \cos(x+\displaystyle \frac{\pi}{3})\ dx$
出典:2009年茨城大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\pi} x\ \cos(x+\displaystyle \frac{\pi}{3})\ dx$
出典:2009年茨城大学 入試問題
福田の1.5倍速演習〜合格する重要問題020〜東京工業大学2016年度理系数学第5問〜媒介変数で表された曲線の追跡と面積

単元:
#大学入試過去問(数学)#平面上の曲線#微分とその応用#積分とその応用#微分法#定積分#学校別大学入試過去問解説(数学)#媒介変数表示と極座標#東京工業大学#数学(高校生)#数C#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
次のように媒介変数表示されたxy平面上の曲線をCとする。
$\left\{\begin{array}{1}
x=3\cos t-\cos3t
y=3\sin t-\sin3t
\end{array}\right.$
ただし、$0 \leqq t \leqq \frac{\pi}{2}$である。
(1)$\frac{dx}{dt}$および$\frac{dy}{dt}$を計算し、Cの概形を図示せよ。
(2)Cとx軸とy軸で囲まれた部分の面積を求めよ。
2016東京工業大学理系過去問
この動画を見る
次のように媒介変数表示されたxy平面上の曲線をCとする。
$\left\{\begin{array}{1}
x=3\cos t-\cos3t
y=3\sin t-\sin3t
\end{array}\right.$
ただし、$0 \leqq t \leqq \frac{\pi}{2}$である。
(1)$\frac{dx}{dt}$および$\frac{dy}{dt}$を計算し、Cの概形を図示せよ。
(2)Cとx軸とy軸で囲まれた部分の面積を求めよ。
2016東京工業大学理系過去問
大学入試問題#386「よく見かける問題」 #弘前大学(2009) #定積分

単元:
#積分とその応用#定積分#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} \displaystyle \frac{dx}{(3+x^2)^2}$
出典:2009年弘前大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{dx}{(3+x^2)^2}$
出典:2009年弘前大学 入試問題
大学入試問題#385「もはや日曜日の朝食のメニュー」 信州大学(2009) #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$a \gt 0$
$\displaystyle \int_{-a}^{a} \displaystyle \frac{x^2\cos\ x+e^x}{e^x+1} dx$
出典:2009年信州大学 入試問題
この動画を見る
$a \gt 0$
$\displaystyle \int_{-a}^{a} \displaystyle \frac{x^2\cos\ x+e^x}{e^x+1} dx$
出典:2009年信州大学 入試問題
福田の1.5倍速演習〜合格する重要問題017〜東北大学2016年度理系数学第6問〜定積分で表された関数

単元:
#大学入試過去問(数学)#微分とその応用#積分とその応用#関数の変化(グラフ・最大最小・方程式・不等式)#定積分#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
関数
$f(x)=\int_0^{\pi}|\sin(t-x)-\sin2t|dt$
の区間$\ 0 \leqq x \leqq \pi\ $における最大値と最小値を求めよ。
2016東北大学理系過去問
この動画を見る
関数
$f(x)=\int_0^{\pi}|\sin(t-x)-\sin2t|dt$
の区間$\ 0 \leqq x \leqq \pi\ $における最大値と最小値を求めよ。
2016東北大学理系過去問
#大学への数学 学力コンテスト(3)「どこで技をかけにいくか・・・」 #定積分

単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$f(x)=\sqrt{ \displaystyle \frac{x}{1+x} }(0 \leqq x \leqq 1)$
(1)
$f'(x)$を求めよ。
(2)
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin\ x-\sin^2x }\ dx$
(3)
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin^3x-\sin^4x }\ dx$
この動画を見る
$f(x)=\sqrt{ \displaystyle \frac{x}{1+x} }(0 \leqq x \leqq 1)$
(1)
$f'(x)$を求めよ。
(2)
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin\ x-\sin^2x }\ dx$
(3)
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin^3x-\sin^4x }\ dx$
#大学への数学「大学受験で、たまに使う技」 学力コンテスト (1)(2) #定積分
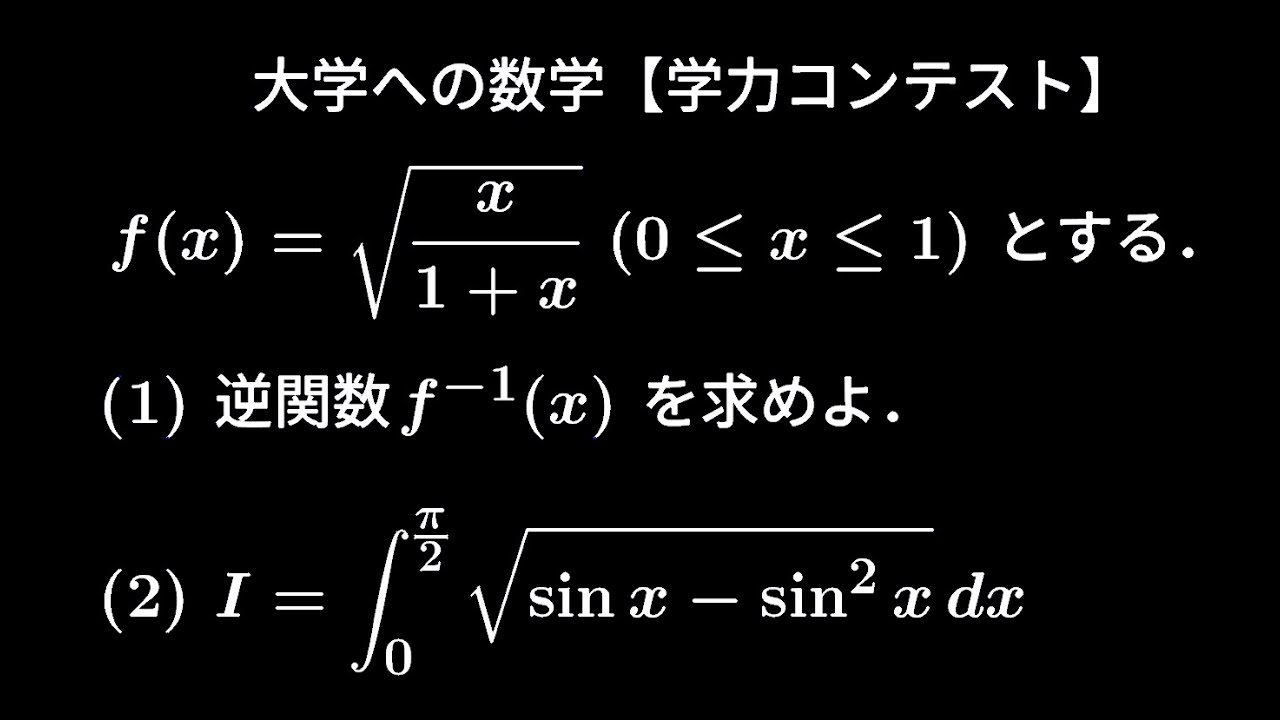
単元:
#微分とその応用#積分とその応用#微分法#色々な関数の導関数#定積分#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$f(x)=\sqrt{ \displaystyle \frac{x}{1+x} }(0 \leqq x \leqq 1)$
(1)
逆関数$f^{-1}(x)$を求めよ。
(2)
$I=\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin\ x-\sin^2x }\ dx$
(3)
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin^3x-\sin^4x }\ dx$
この動画を見る
$f(x)=\sqrt{ \displaystyle \frac{x}{1+x} }(0 \leqq x \leqq 1)$
(1)
逆関数$f^{-1}(x)$を求めよ。
(2)
$I=\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin\ x-\sin^2x }\ dx$
(3)
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ \sin^3x-\sin^4x }\ dx$
大学入試問題#377「基本的な手筋」 琉球大学(2015) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#琉球大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-1}^{1}|x|\sqrt{ 1-x^2 }\ dx$
出典:2015年琉球大学 入試問題
この動画を見る
$\displaystyle \int_{-1}^{1}|x|\sqrt{ 1-x^2 }\ dx$
出典:2015年琉球大学 入試問題
大学入試問題#375「定跡どおり」 広島市立大学2015 #定積分
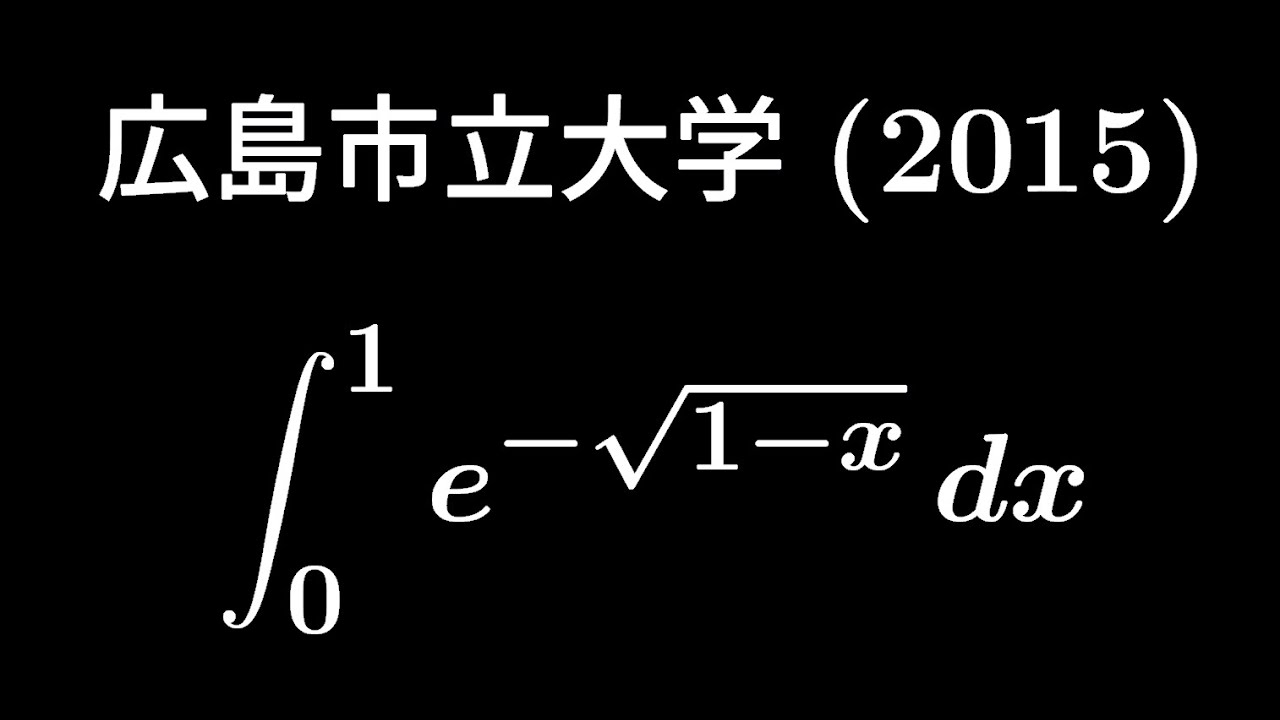
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}e^{-\sqrt{ 1-x }}dx$
出典:2015年広島市立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}e^{-\sqrt{ 1-x }}dx$
出典:2015年広島市立大学 入試問題
大学入試問題#374「技をかける前の味付け」 富山県立大学(2015) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#富山県立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x}{3+4\cos^2x}dx$
出典:2015年富山県立大学 入試問題
この動画を見る
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x}{3+4\cos^2x}dx$
出典:2015年富山県立大学 入試問題
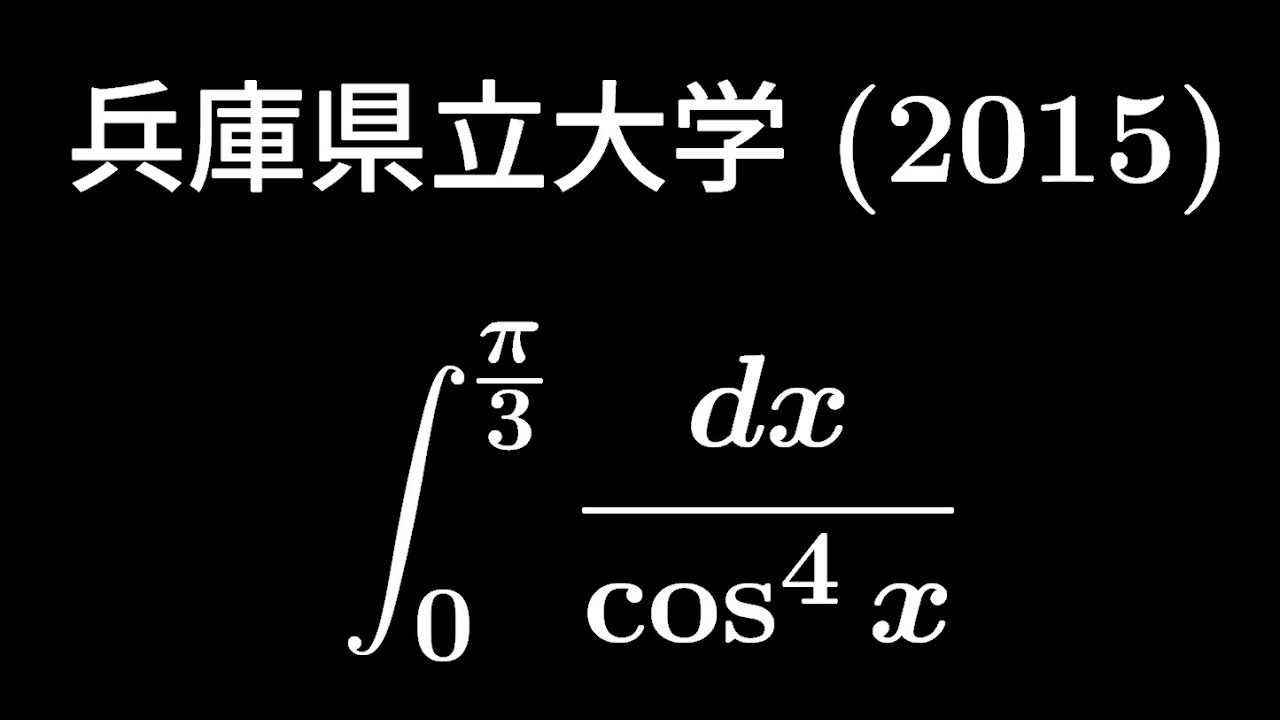
大学入試問題#372「初手が命」 兵庫県立大学2015 #定積分
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#兵庫県立大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{3}}\displaystyle \frac{dx}{\cos^4x}$
出典:2015年兵庫県立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{3}}\displaystyle \frac{dx}{\cos^4x}$
出典:2015年兵庫県立大学 入試問題
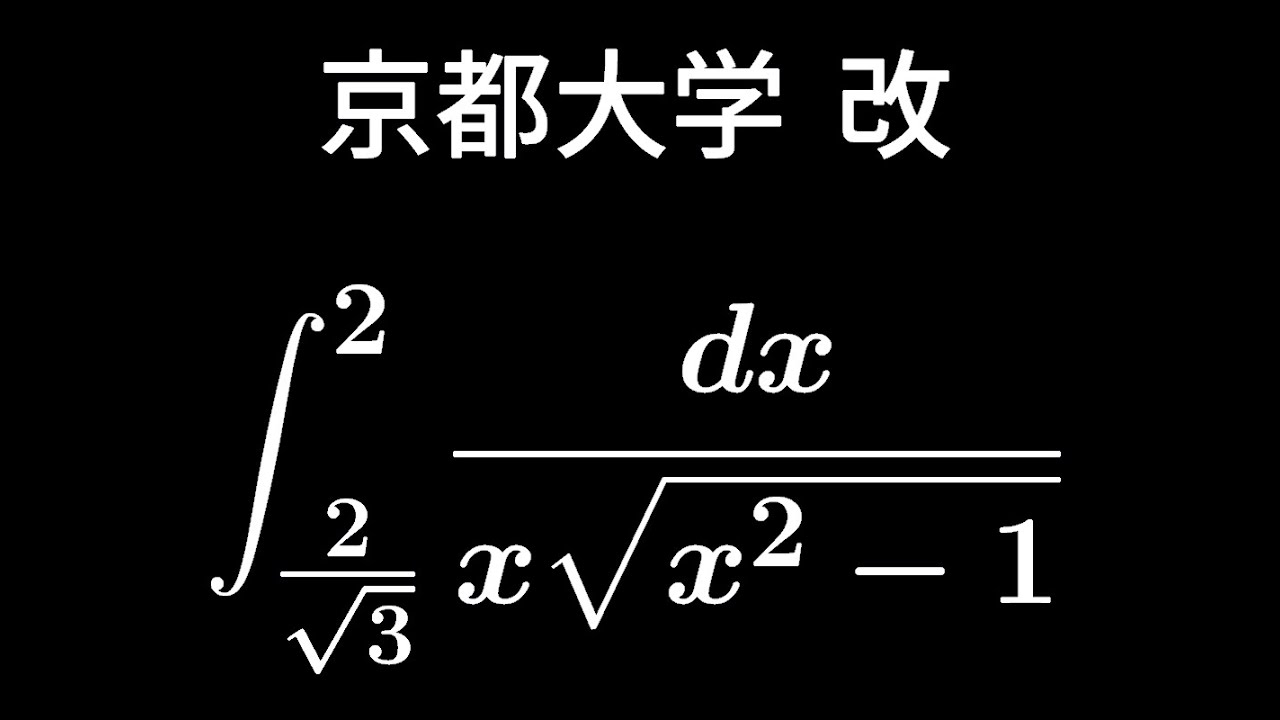
大学入試問題#371「少し変わった置換積分」 京都大学 改 #定積分
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{2}{\sqrt{ 3 }}}^{2}\displaystyle \frac{dx}{x\sqrt{ x^2-1 }}$
出典:京都大学 入試問題
この動画を見る
$\displaystyle \int_{\frac{2}{\sqrt{ 3 }}}^{2}\displaystyle \frac{dx}{x\sqrt{ x^2-1 }}$
出典:京都大学 入試問題
大学入試問題#370「初手は好み」 滋賀医科大学2012 #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#滋賀医科大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}\displaystyle \frac{1+x}{1+x^2}dx$
出典:2012年滋賀医科大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}\displaystyle \frac{1+x}{1+x^2}dx$
出典:2012年滋賀医科大学 入試問題
大学入試問題#369「2種類準備しました」 広島市立大学2014 #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{\cos\ x}{\cos^2x+2\sin\ x-2}dx$
出典:2014年広島市立大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{\cos\ x}{\cos^2x+2\sin\ x-2}dx$
出典:2014年広島市立大学 入試問題
大学入試問題#367「これは、たぶん一撃で倒せる」 横浜国立大学2012 #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}}\displaystyle \frac{2+\sin\ x}{1+\cos\ x}dx$
出典:2012年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}}\displaystyle \frac{2+\sin\ x}{1+\cos\ x}dx$
出典:2012年横浜国立大学 入試問題
大学入試問題#366「これは有名問題」 静岡大学2014 #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#静岡大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\cos^3x}{\cos\ x+\sin\ x}dx$
出典:2014年静岡大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\cos^3x}{\cos\ x+\sin\ x}dx$
出典:2014年静岡大学 入試問題
大学入試問題#365「さすがに小問」 旭川医科大学(2014) #定積分
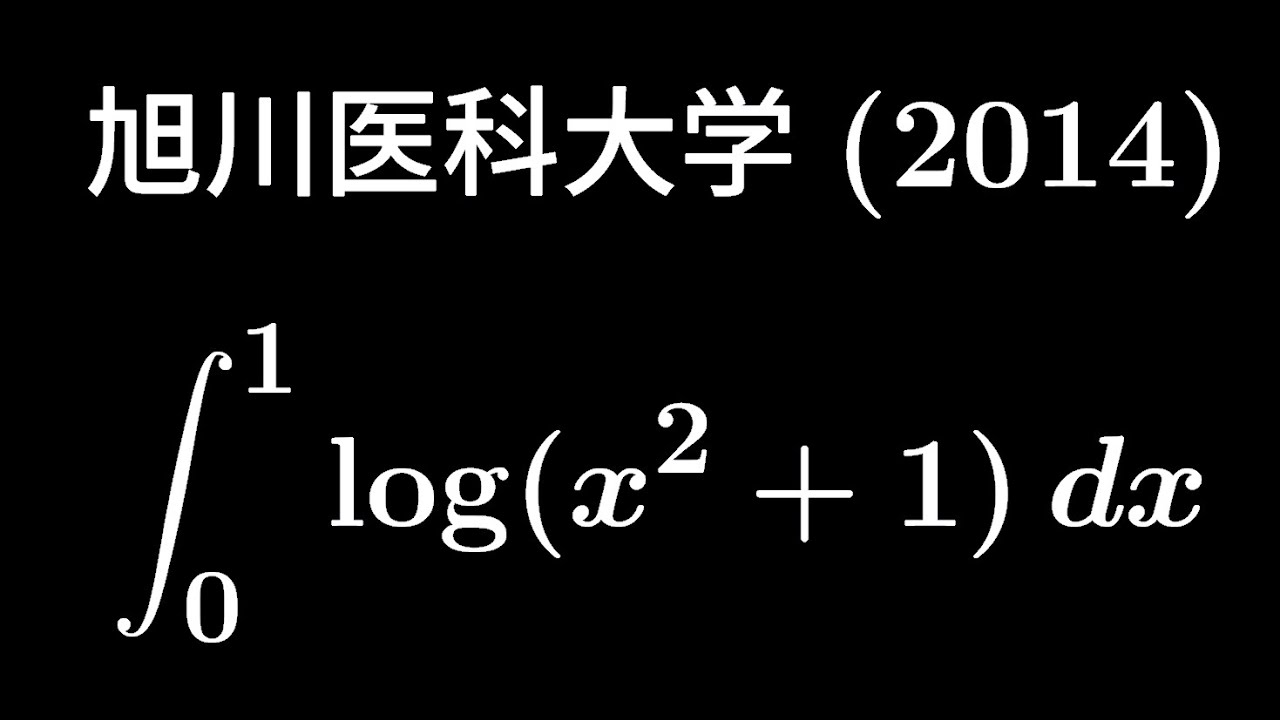
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#旭川医科大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}log(x^2+1)dx$
出典:2014年旭川医科大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}log(x^2+1)dx$
出典:2014年旭川医科大学 入試問題
