ますただ
ますただ
 ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
#宮崎大学 2020年 #定積分 #Shorts
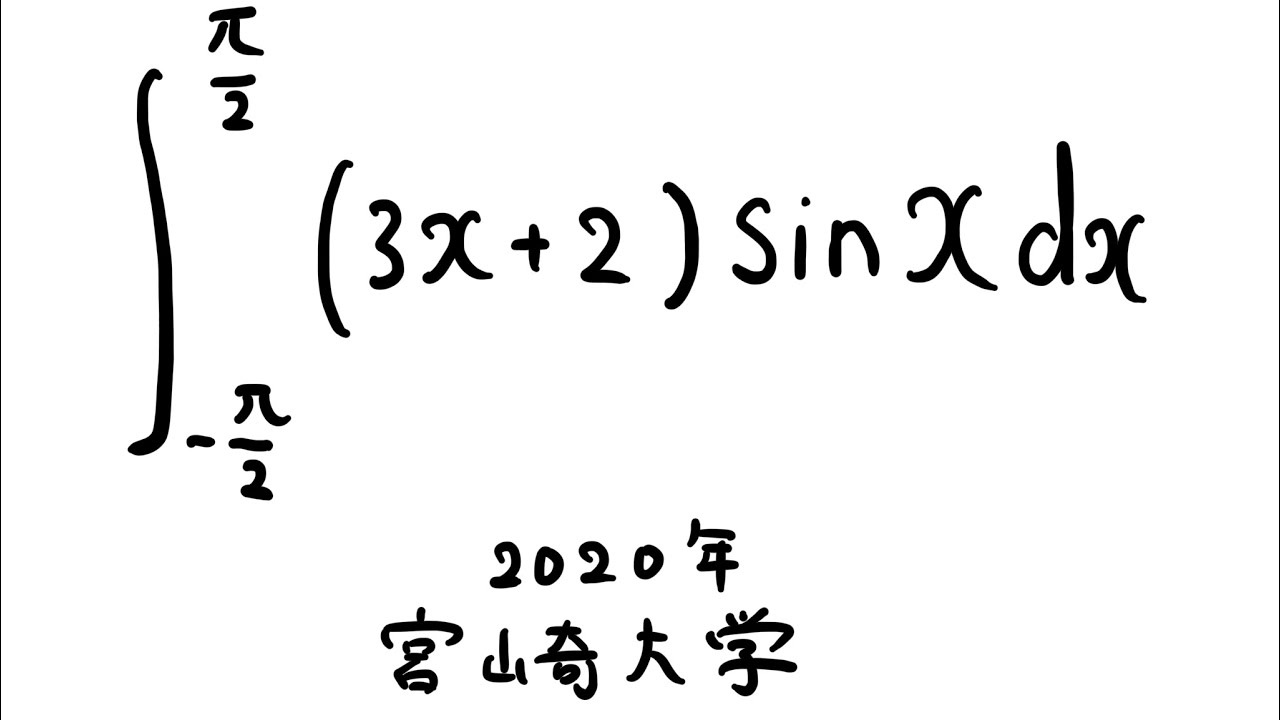
大学入試問題#762「再生回数は、期待できない」 東京理科大学工学部(2003) #曲線の長さ

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
曲線
出典:2003年東京理科大学工学部 入試問題
この動画を見る
曲線
出典:2003年東京理科大学工学部 入試問題
#宮崎大学 2022年 #定積分 #Shorts
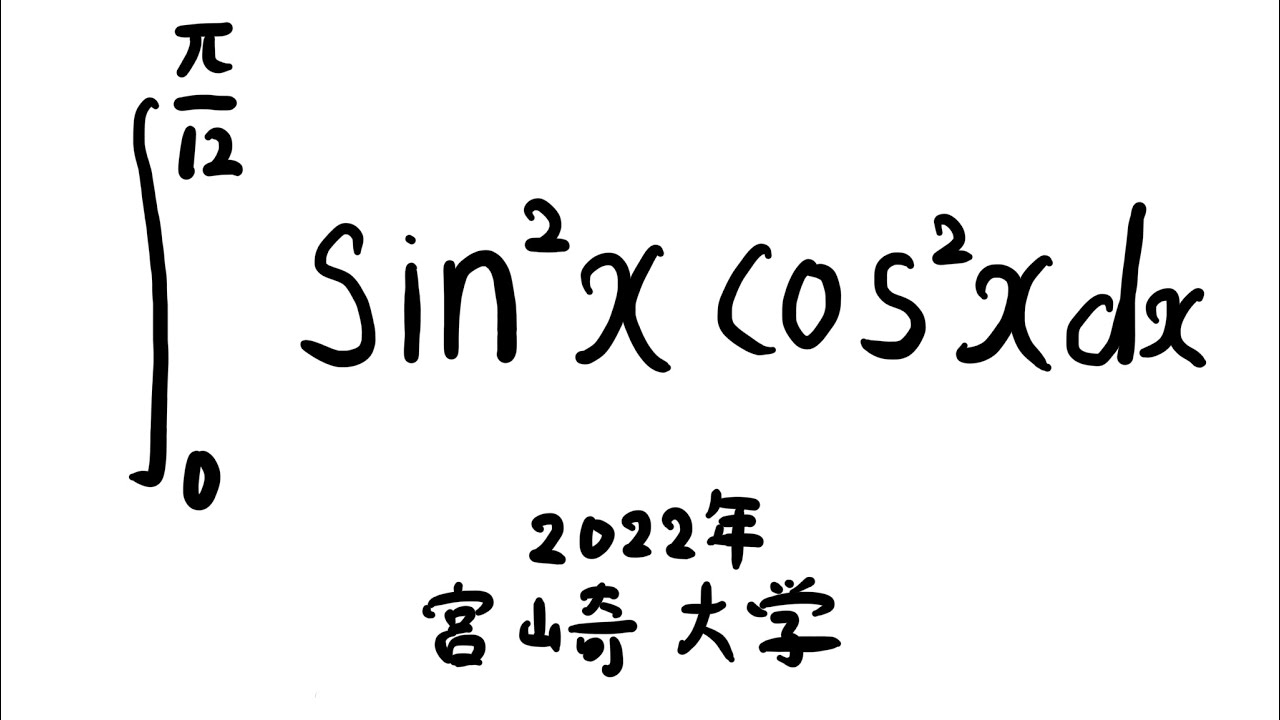
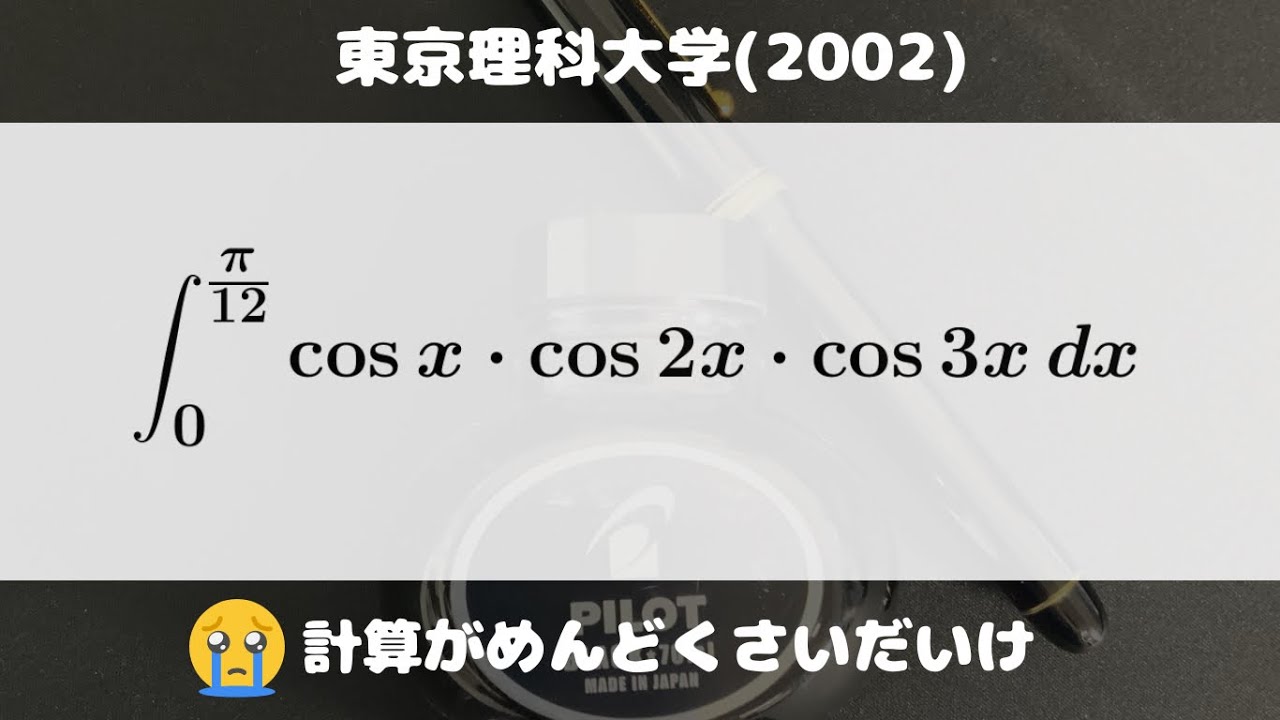
大学入試問題#761「微積の入試勉強は、まずこれから!」 東京理科大学理学部(2002) #微積

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
関数
出典:2002年東京理科大学理学部 入試問題
この動画を見る
関数
出典:2002年東京理科大学理学部 入試問題
大学入試問題#760「ほぼ一直線」 東京理科大学(2003) #定積分

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
定積分
出典:2003年東京理科大学 入試問題
この動画を見る
定積分
出典:2003年東京理科大学 入試問題
大学入試問題#759「サムネみすった」 東京理科大学(2002) #定積分
#広島市立大学 2010年 #不定積分 #Shorts
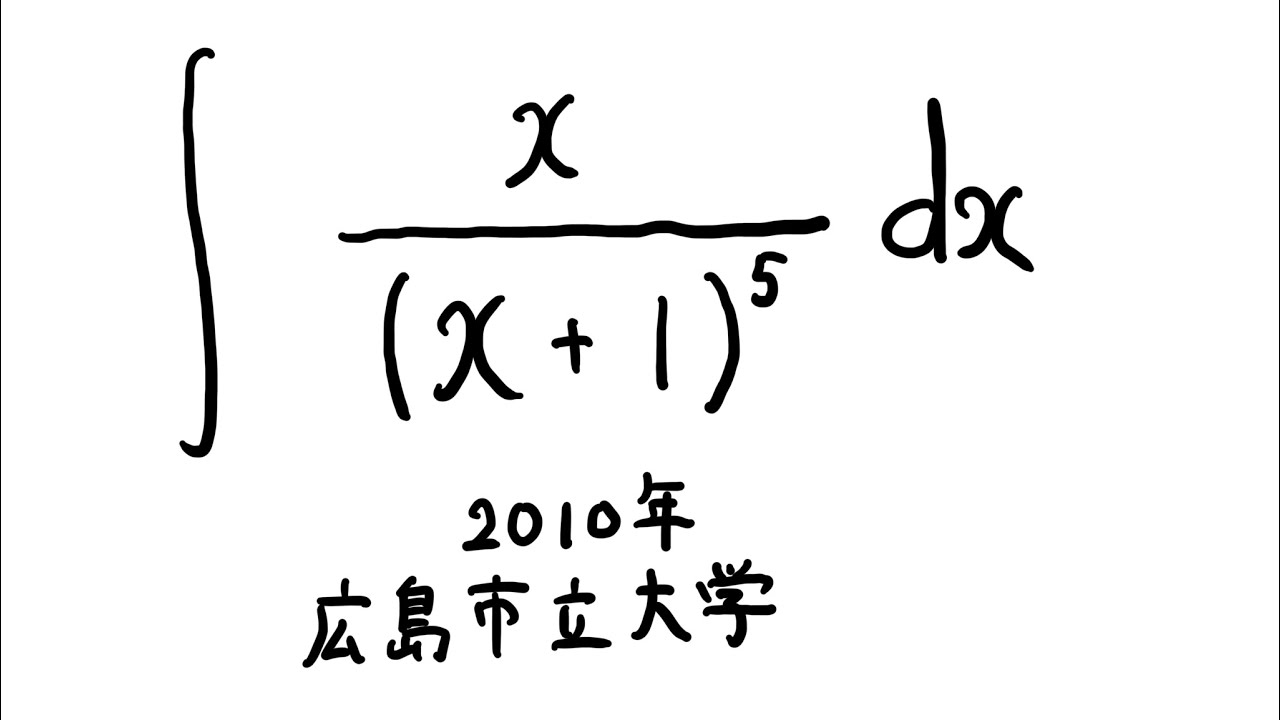
#広島市立大学 2010年 #不定積分 #Shorts
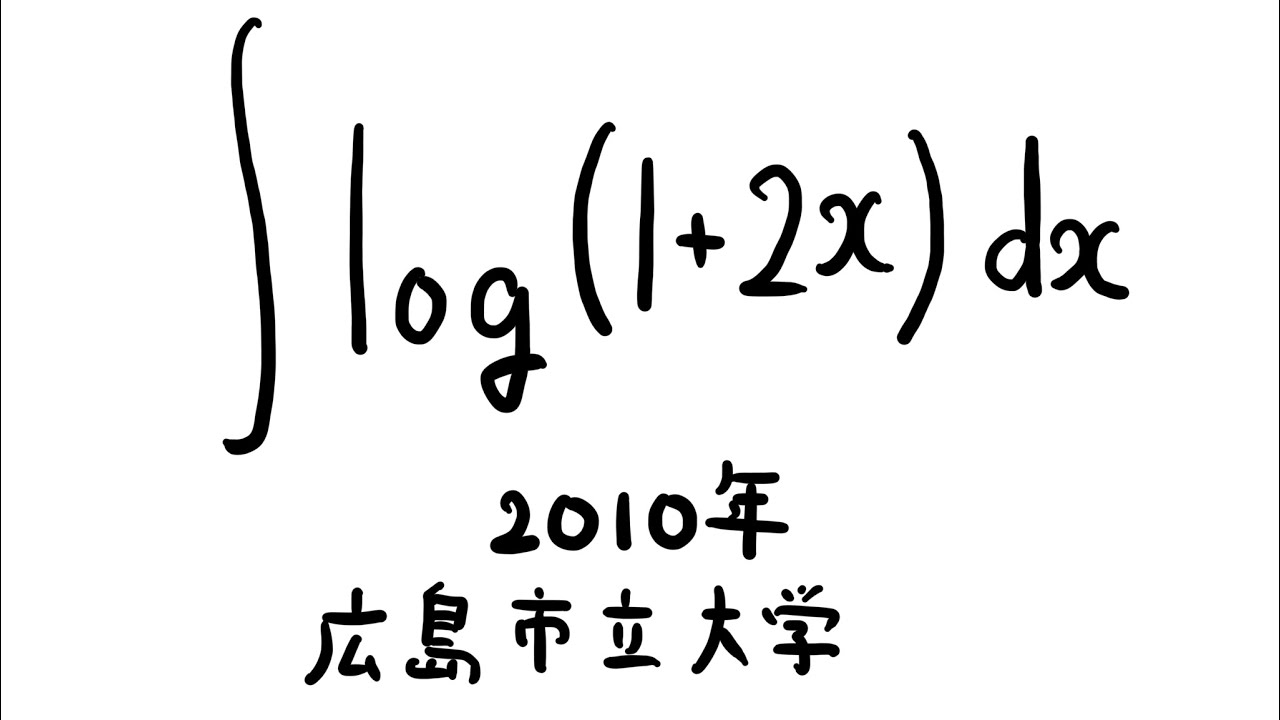
大学入試問題#758 「ミスりようがない。」 東京理科大学理学部(2002) #方程式

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
方程式
出典:2002年東京理科大学理学部 入試問題
この動画を見る
方程式
出典:2002年東京理科大学理学部 入試問題
#岡山県立大学 2023年 #Shorts
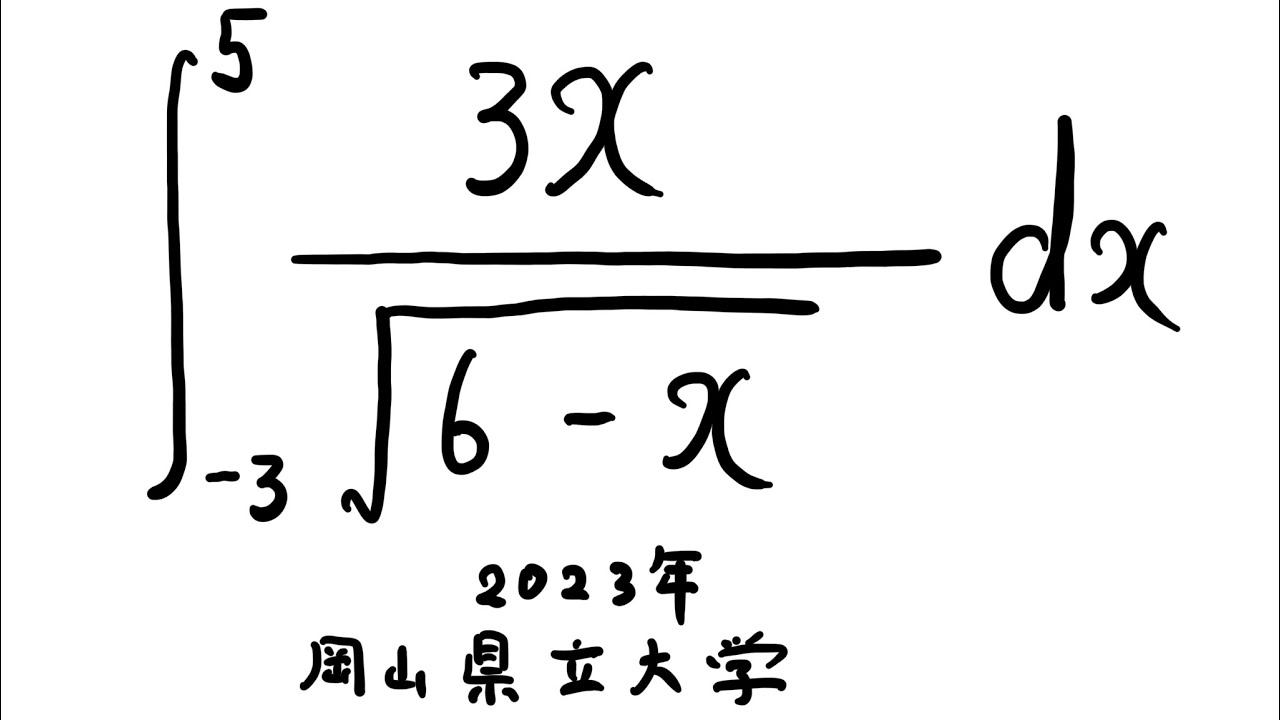
大学入試問題#757「綺麗な基本問題」 東京理科大学(2001) #積分方程式

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
関数
このとき、
出典:2001年東京理科大学工学部 入試問題
この動画を見る
関数
このとき、
出典:2001年東京理科大学工学部 入試問題
大学入試問題#756「ほぼ定石通り」 藤田医科大学(2024) #級数

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#藤田医科大学
指導講師:
ますただ
問題文全文(内容文):
1.数列
2.
出典:2024年藤田医科大学 入試問題
この動画を見る
1.数列
2.
出典:2024年藤田医科大学 入試問題
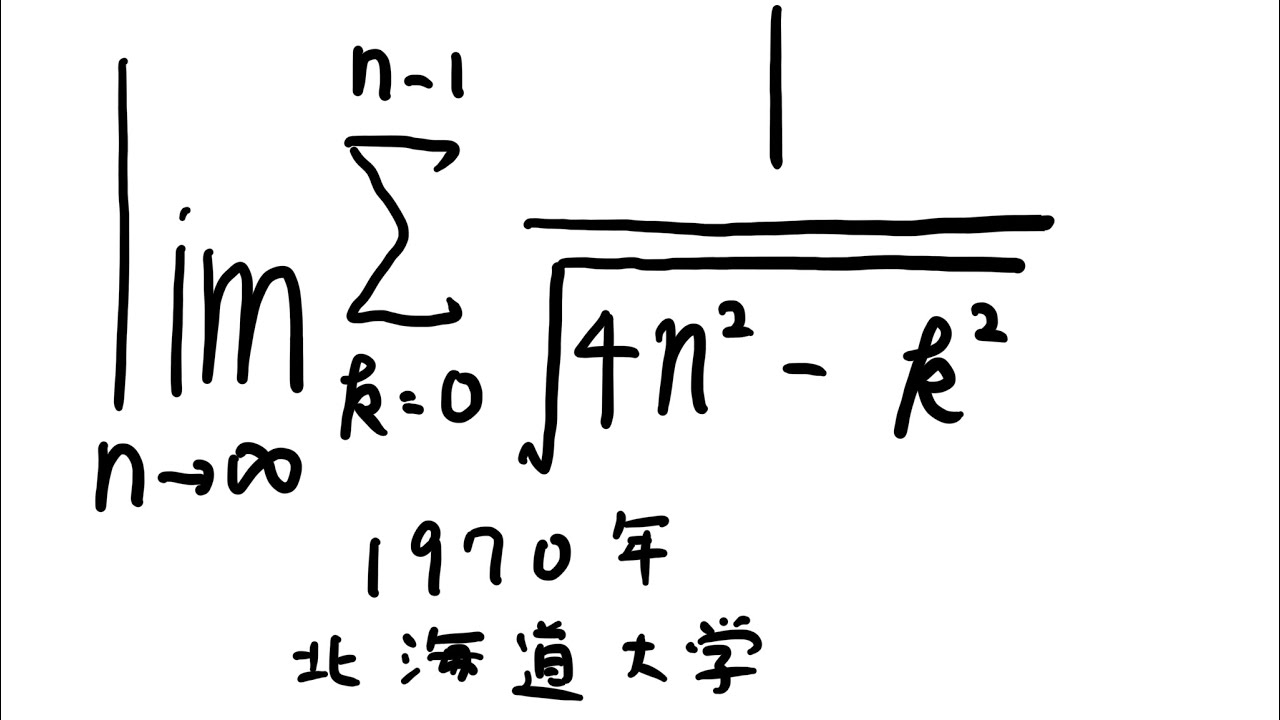
大学入試問題#755「基本問題」 北海道大学(1970) #微分方程式

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#北海道大学
指導講師:
ますただ
問題文全文(内容文):
(1)
(2)
出典:1970年北海道大学 入試問題
この動画を見る
(1)
(2)
出典:1970年北海道大学 入試問題
#岡山県立大学 2011 #定積分 #Shorts
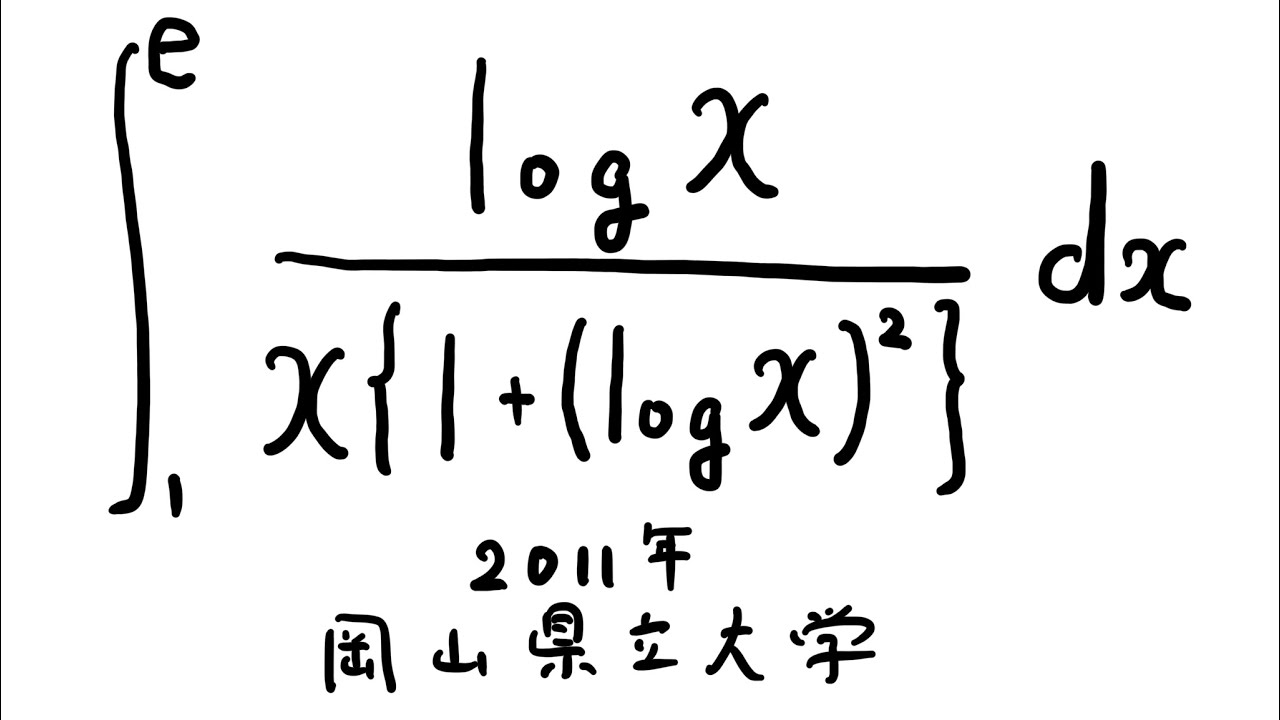
大学入試問題#754「スッキリと解きたい」 早稲田大学人間科学部(2022) #整数問題

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
等式
を満たす整数
出典:2022年早稲田大学人間科学部 入試問題
この動画を見る
等式
を満たす整数
出典:2022年早稲田大学人間科学部 入試問題
大学入試問題#753「普通に超良問」 東京理科大学理工学部(1999) #積分方程式

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
を満たすとき、定数
出典:1999年東京理科大学理工学部 入試問題
この動画を見る
を満たすとき、定数
出典:1999年東京理科大学理工学部 入試問題
#国士舘大学2022 #定積分 #Shorts
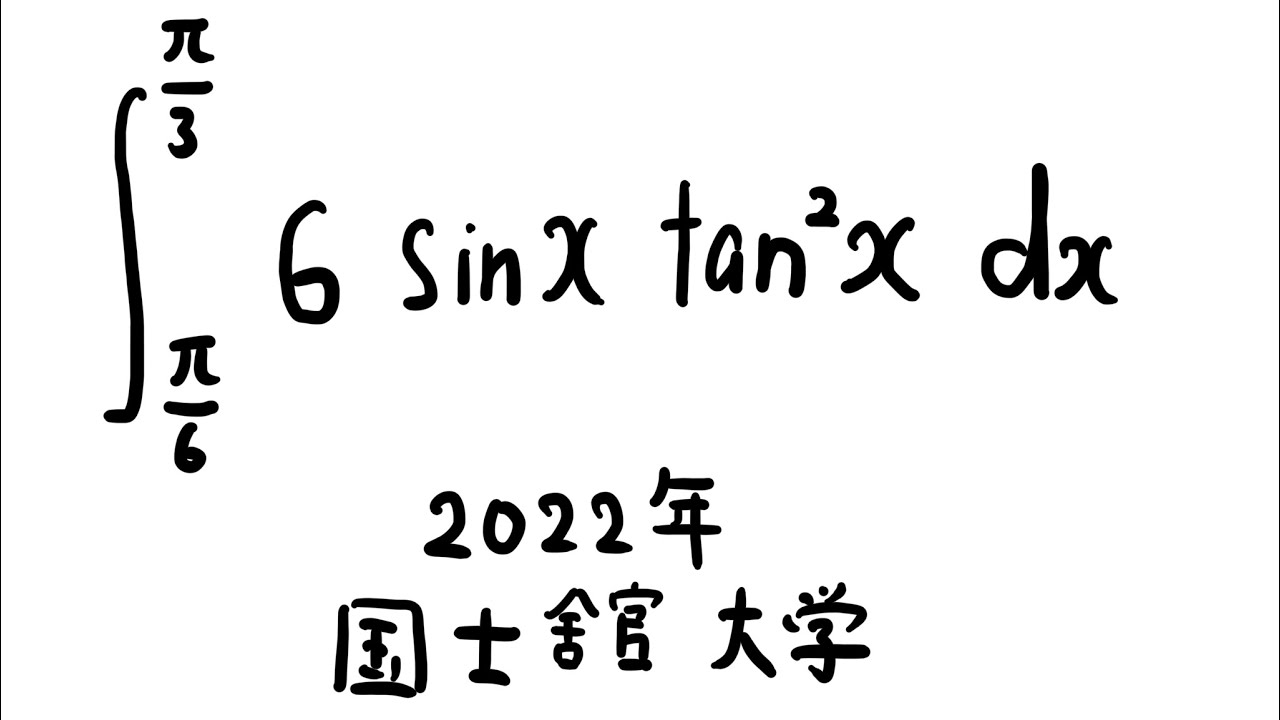
大学入試問題#752「初見だと少し焦る」 電気通信大学後期(2023) #区分求積法

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#電気通信大学
指導講師:
ますただ
問題文全文(内容文):
出典:2023年電子通信大学後期 入試問題
この動画を見る
出典:2023年電子通信大学後期 入試問題
#日本医科大学2020 #定積分 #Shorts
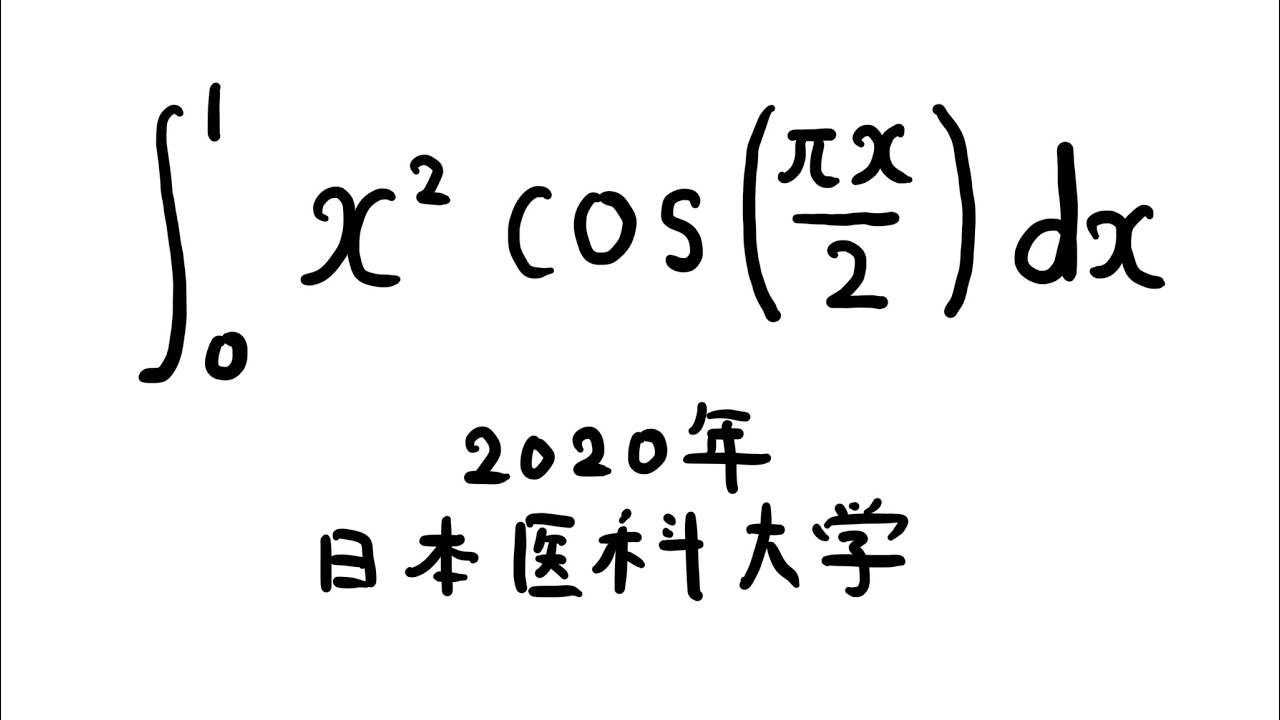
大学入試問題#751「定石通り」 東京女子医科大学(2002) #複素数

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#東京女子医科大学
指導講師:
ますただ
問題文全文(内容文):
出典:2002年東京女子医科大学 入試問題
この動画を見る
出典:2002年東京女子医科大学 入試問題
大学入試問題#750「超良問!」 東京理科大学工学部(2001) #定積分

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
出典:2001年東京理科大学工学部 入試問題
この動画を見る
出典:2001年東京理科大学工学部 入試問題
#整数問題 #早稲田大学2022 #Shorts

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
出典:2022年早稲田大学人間科学部
この動画を見る
出典:2022年早稲田大学人間科学部
大学入試問題#749「まあミスれん」 東京理科大学(2000) #不定積分

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
出典:2000年東京理科大学工学部 入試問題
この動画を見る
出典:2000年東京理科大学工学部 入試問題
大学入試問題#748「計算力が試される」 早稲田大学人間科学部(2006) 積分方程式

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
出典:2006年早稲田大学人間科学部 入試問題
この動画を見る
出典:2006年早稲田大学人間科学部 入試問題
#東海大学医学部(2019) #極限 #Shorts
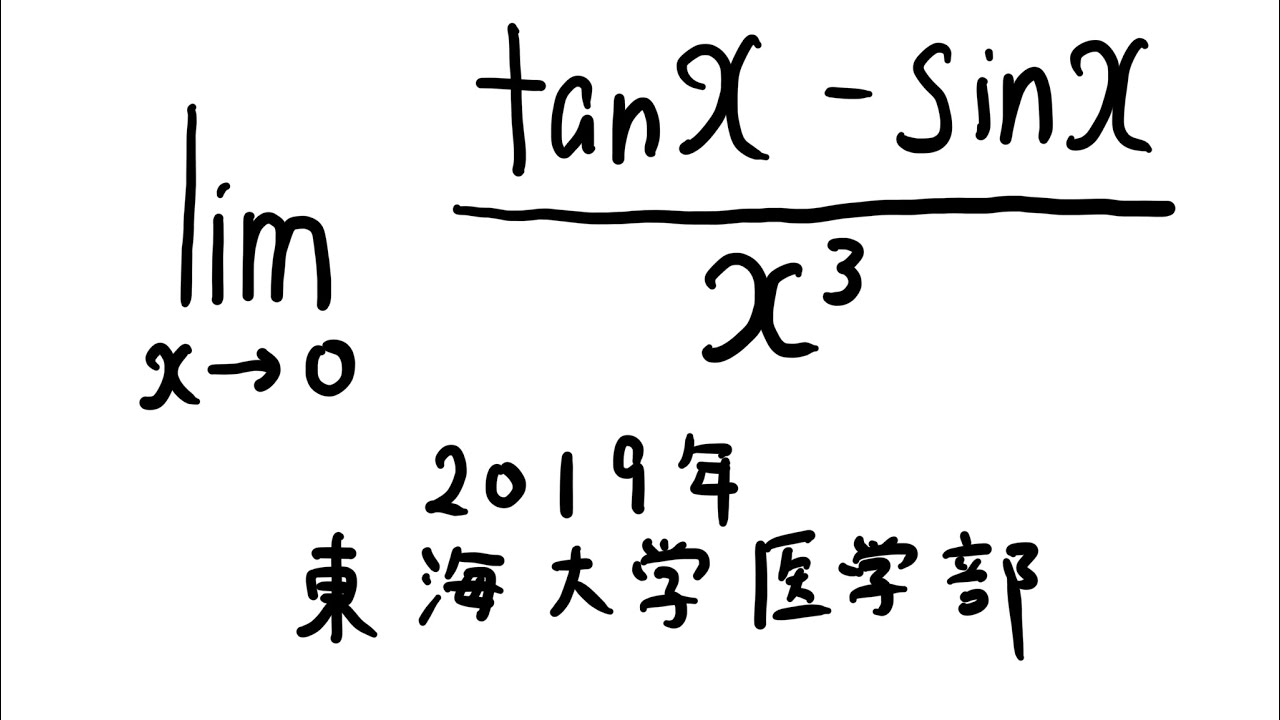
大学入試問題#747「解き方は好み」 早稲田大学国際教養学部(2006)

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
実数
出典:2006年早稲田大学国際教養学部 入試問題
この動画を見る
実数
出典:2006年早稲田大学国際教養学部 入試問題
大学入試問題#746「慣れれば瞬間部分積分!?」 東京理科大学(2024) #定積分

昭和大学医学部(2006) #極限 #Shorts
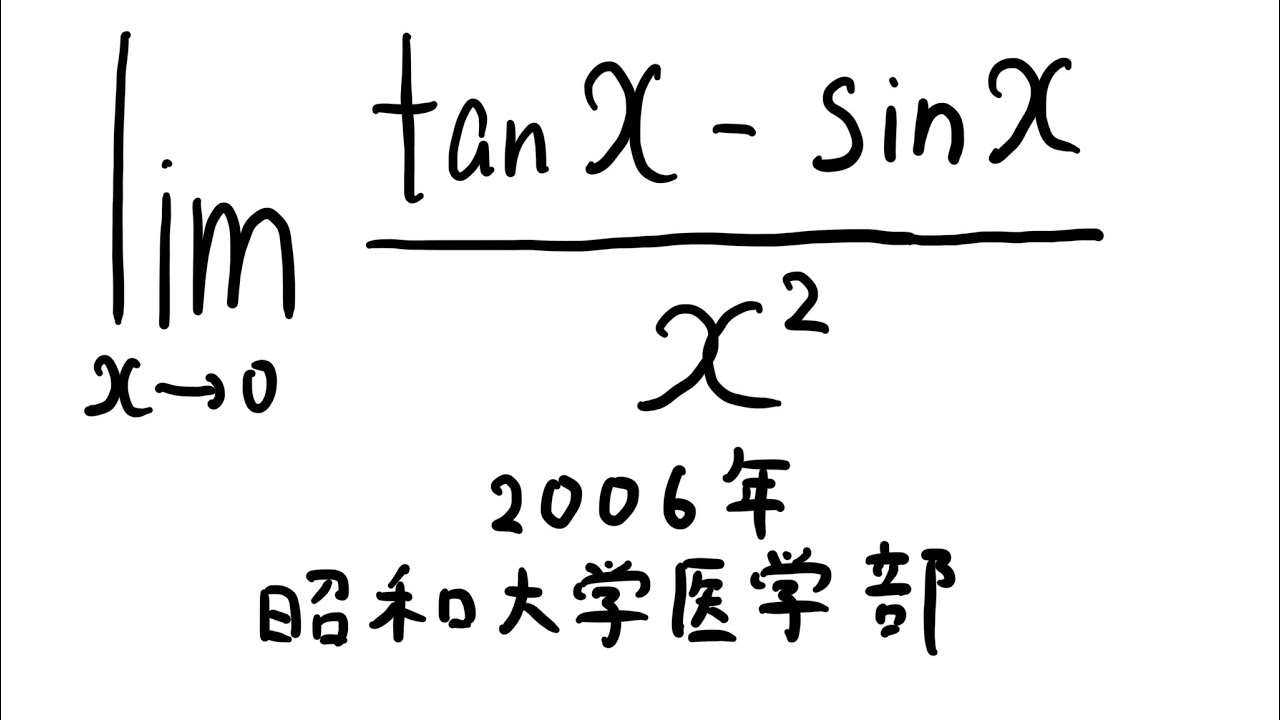
大学入試問題#745「落ち着けばどうにかなる」 早稲田大学理工学部(2002) 微積の応用

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
(1)
(2)
出典:2002年早稲田大学理工学部 入試問題
この動画を見る
(1)
(2)
出典:2002年早稲田大学理工学部 入試問題
北海道大学(1970) #定積分 #Shorts