ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！
職業：数学者
職歴：高校非常勤（院生時代）、高専、大学 准教授
趣味：
①将棋 棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀 アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス 中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。
#数検準1級1次過去問#定積分
単元：
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{e^2-1} log(x+1)$ $dx$
出典：数検準1級1次
この動画を見る
$\displaystyle \int_{0}^{e^2-1} log(x+1)$ $dx$
出典：数検準1級1次
大学入試問題#885「油断したら沼るかも」 #奈良県立医科大学(2014) 三角関数と整数問題
単元：
#数A#数Ⅱ#大学入試過去問(数学)#整数の性質#約数・倍数・整数の割り算と余り・合同式#三角関数#学校別大学入試過去問解説(数学)#数学(高校生)#奈良県立医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\sqrt{ \displaystyle \frac{a}{20} } \lt \cos\displaystyle \frac{\pi}{8} \lt \sqrt{ \displaystyle \frac{a+1}{20} }$を満たす整数$a$を求めよ。
出典：2014年奈良県立医科大学
この動画を見る
$\sqrt{ \displaystyle \frac{a}{20} } \lt \cos\displaystyle \frac{\pi}{8} \lt \sqrt{ \displaystyle \frac{a+1}{20} }$を満たす整数$a$を求めよ。
出典：2014年奈良県立医科大学
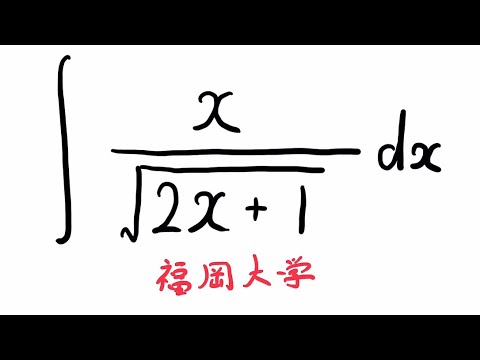
#福岡大学#不定積分#ますただ
単元：
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#福岡大学
指導講師：
ますただ
問題文全文(内容文)：
以下の不定積分を解け
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+1 }}$ $dx$
出典：福岡大学
この動画を見る
以下の不定積分を解け
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+1 }}$ $dx$
出典：福岡大学
#福島大学2013#定積分#ますただ
単元：
#数Ⅱ#微分法と積分法#不定積分・定積分#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{4}} x$ $\sin2$ $x$ $dx$
出典：2013年福島大学
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}} x$ $\sin2$ $x$ $dx$
出典：2013年福島大学
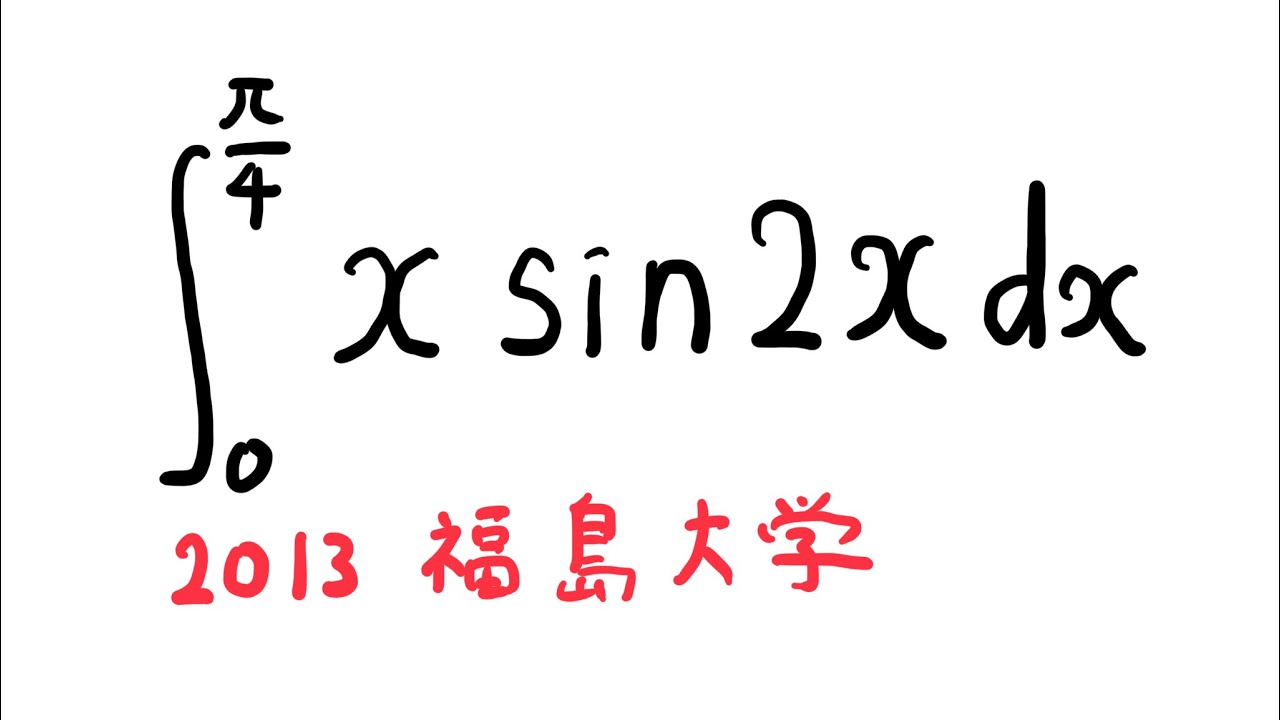
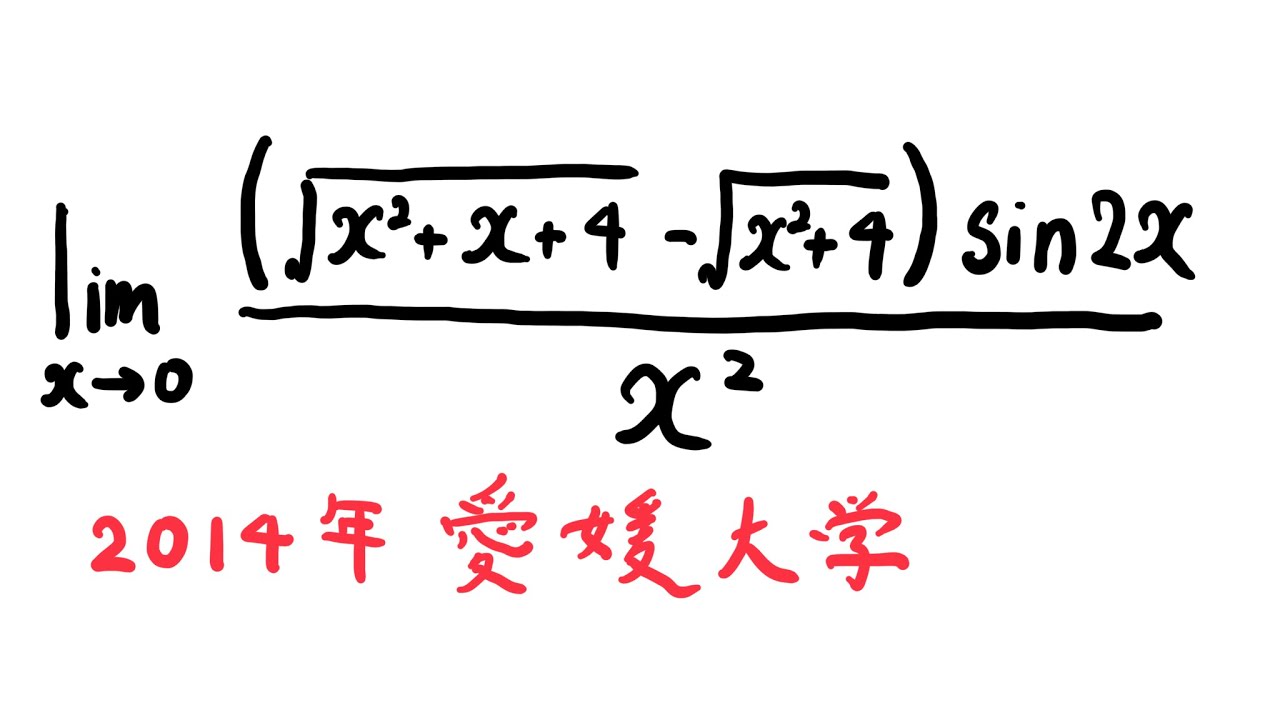
#愛媛大学2014#極限#ますただ
単元：
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#数学(高校生)#愛媛大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{(\sqrt{ x^2+x+4 }-\sqrt{ x^2+4 })\sin2x}{x^2}$
出典：2024年愛媛大学
この動画を見る
$\displaystyle \lim_{ x \to 0 } \displaystyle \frac{(\sqrt{ x^2+x+4 }-\sqrt{ x^2+4 })\sin2x}{x^2}$
出典：2024年愛媛大学
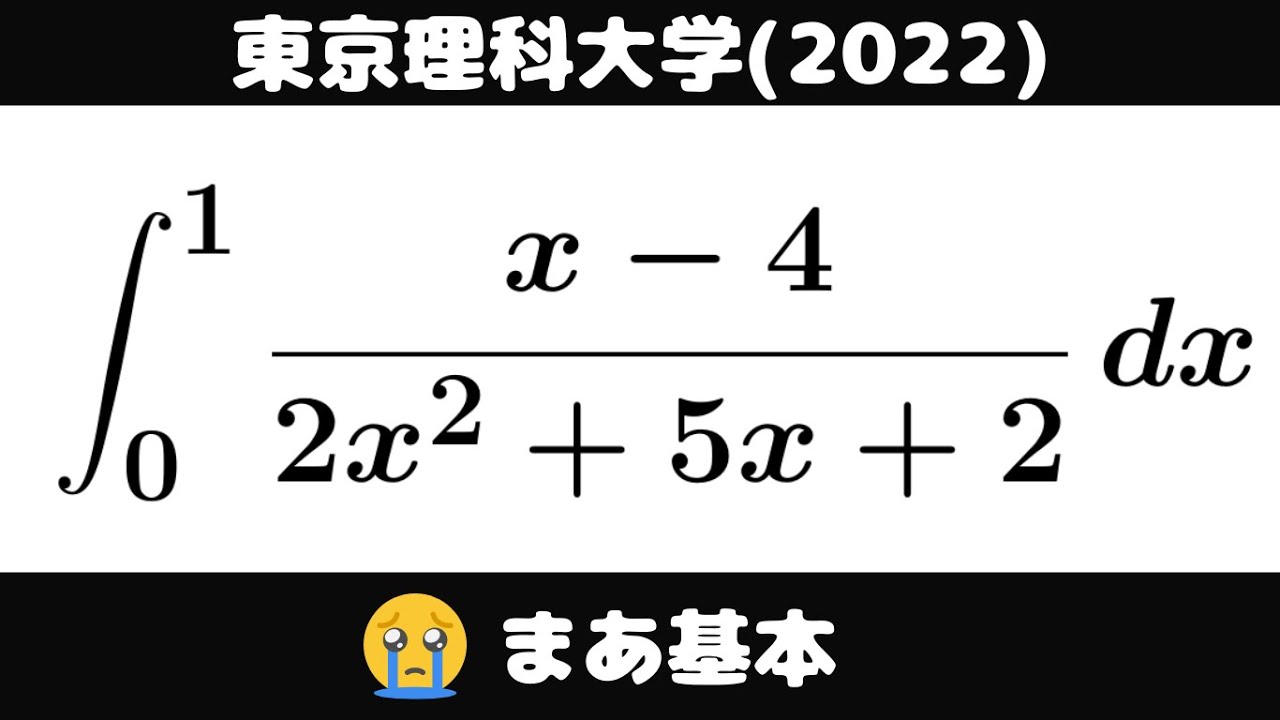
大学入試問題#884「ミスれん」 #東京理科大学(2022) #定積分
単元：
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#東京理科大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1} \displaystyle \frac{x-4}{2x^2+5x+2}$ $dx$
出典：2022年東京理科大学
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{x-4}{2x^2+5x+2}$ $dx$
出典：2022年東京理科大学
#小樽商科大学#不定積分#ますただ
単元：
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#小樽商科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+2}-\sqrt{ 2 }}$ $dx$
出典：小樽商科大学
この動画を見る
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 2x+2}-\sqrt{ 2 }}$ $dx$
出典：小樽商科大学
#山梨大学2013#定積分#ますただ
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#山梨大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-10}^{0} log(x+11)$ $dx$
出典：2013年山梨大学
この動画を見る
$\displaystyle \int_{-10}^{0} log(x+11)$ $dx$
出典：2013年山梨大学
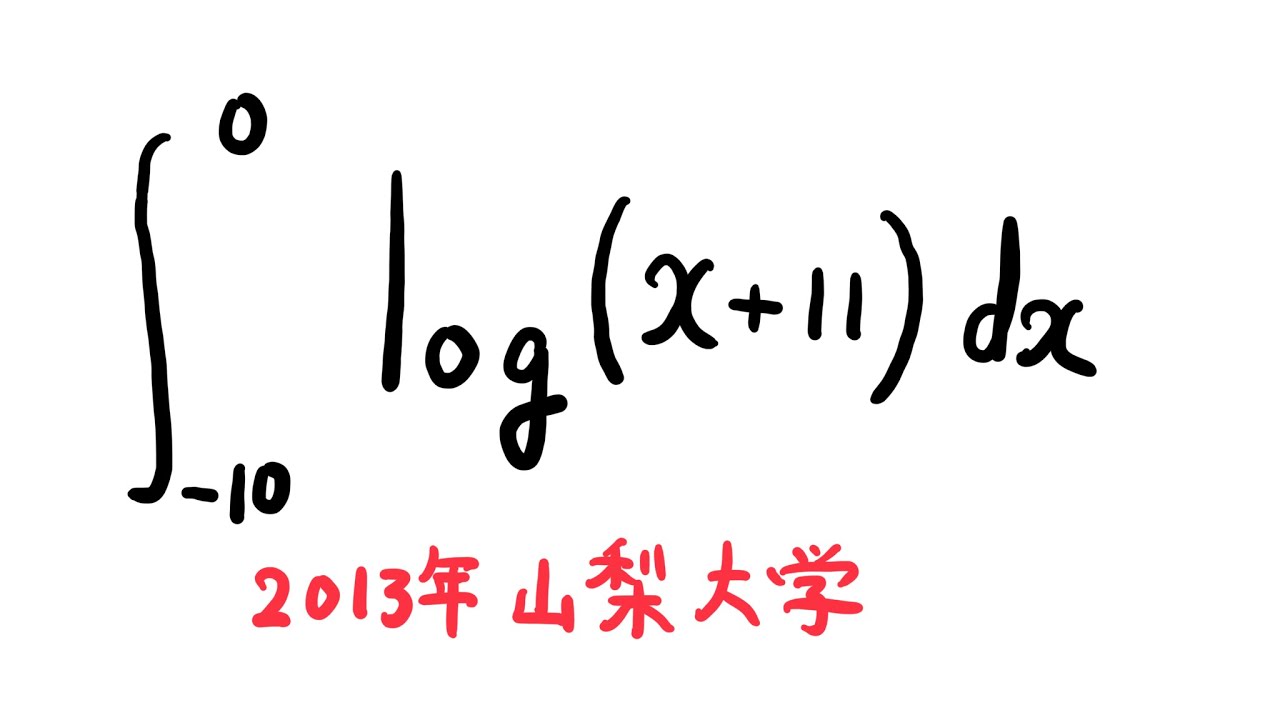
#玉川大学#不定積分#ますただ
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
以下の不定積分を解け
$\displaystyle \int e^{\sin x} \sin2x$ $dx$
出典：玉川大学
この動画を見る
以下の不定積分を解け
$\displaystyle \int e^{\sin x} \sin2x$ $dx$
出典：玉川大学
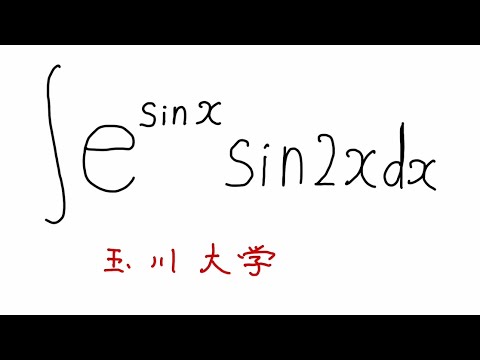
#信州大学 #不定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学
指導講師：
ますただ
問題文全文(内容文)：
以下の不定積分を解け
$\displaystyle \int e^x(e^x+1)^2 dx$
出典：信州大学
この動画を見る
以下の不定積分を解け
$\displaystyle \int e^x(e^x+1)^2 dx$
出典：信州大学
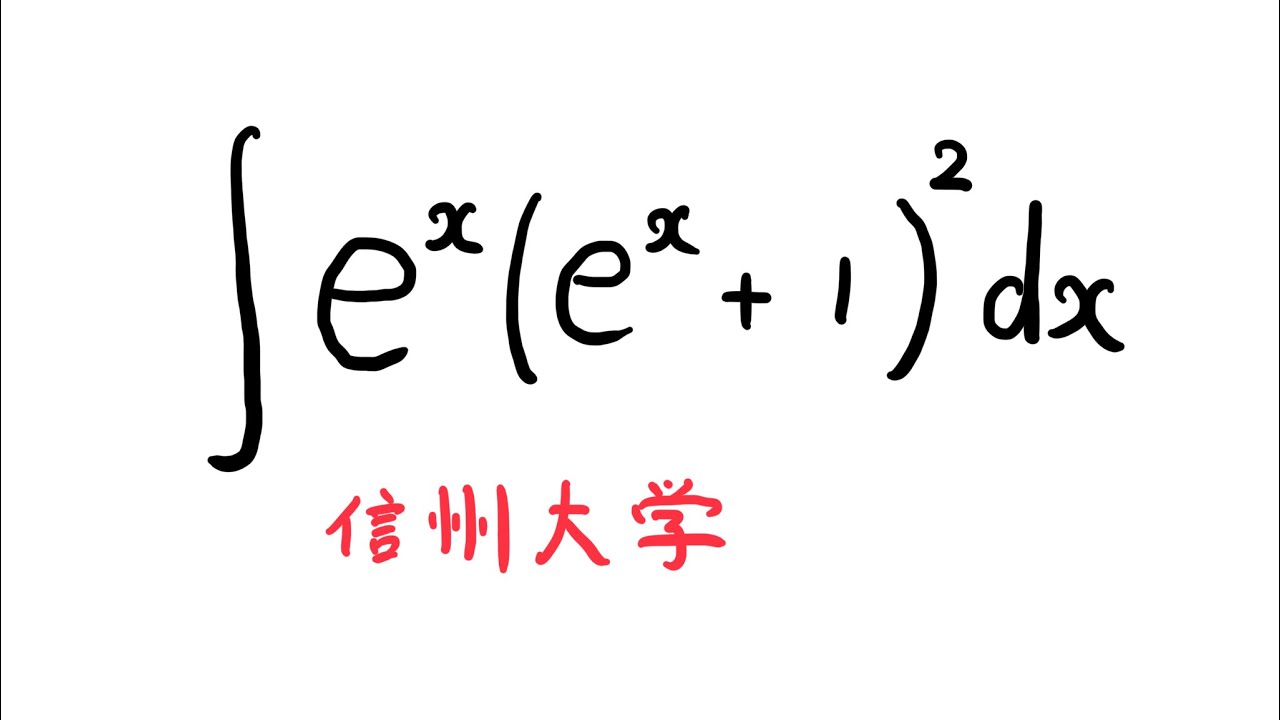
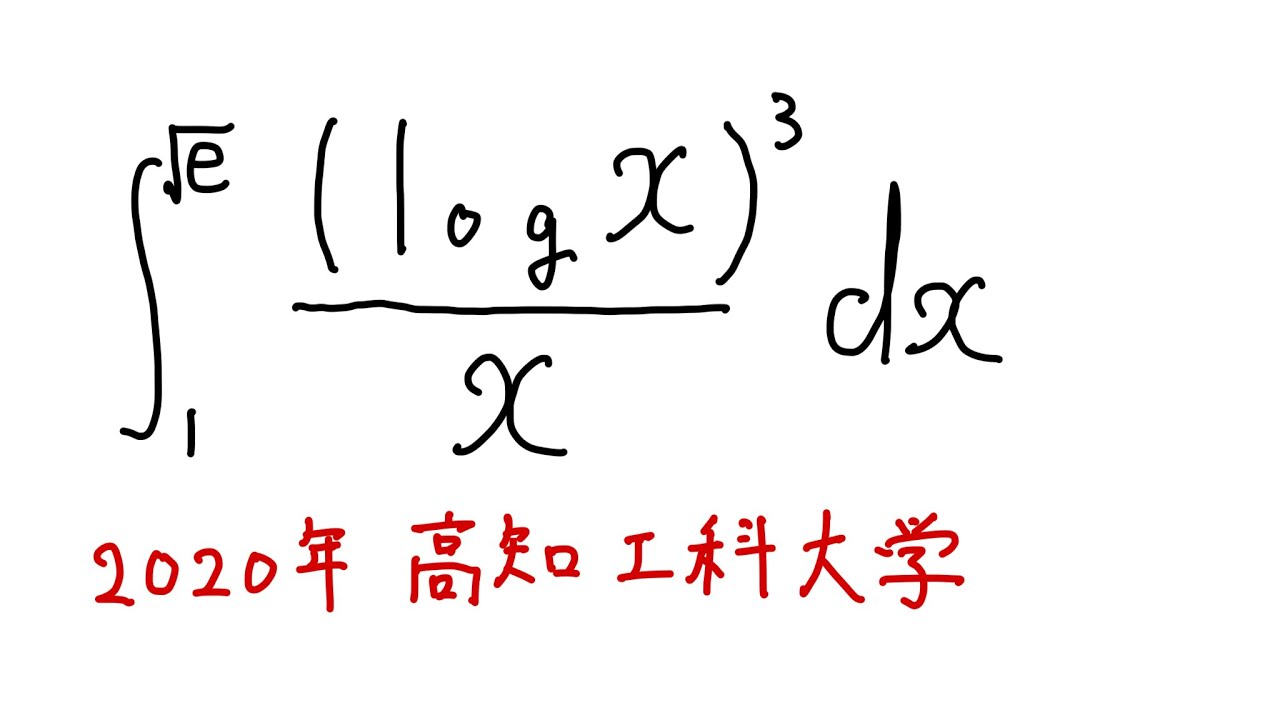
#高知工科大学2020 #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#高知工科大学
指導講師：
ますただ
問題文全文(内容文)：
以下の定積分を解け。
$\displaystyle \int_{1}^{\sqrt{ e }} \displaystyle \frac{(log x)^3}{x} dx$
出典：2020年高知工科大学
この動画を見る
以下の定積分を解け。
$\displaystyle \int_{1}^{\sqrt{ e }} \displaystyle \frac{(log x)^3}{x} dx$
出典：2020年高知工科大学
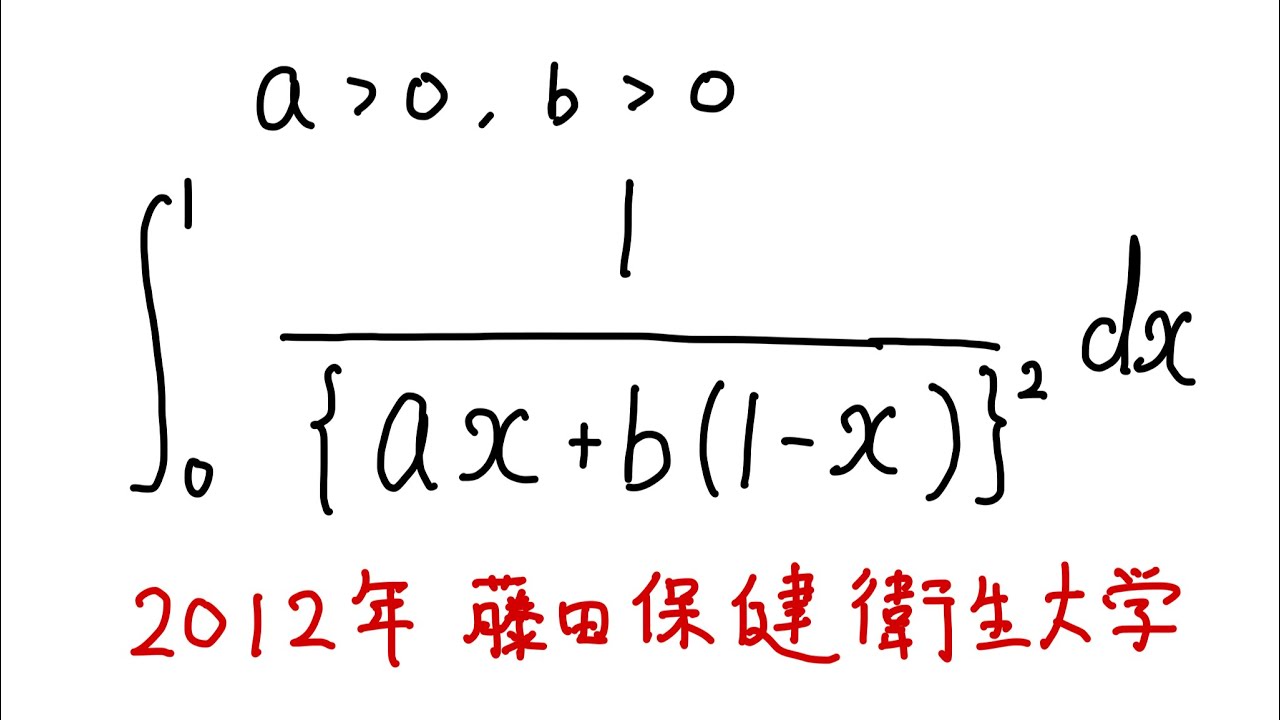
#藤田保健衛生大学2012 #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
以下の定積分を解け。
$a \gt 0,b \gt 0$
$\displaystyle \int_{0}^{1} \displaystyle \frac{1}{\{ax+b(1-x\}^2)} dx$
出典：2010年藤田保健衛生大学
この動画を見る
以下の定積分を解け。
$a \gt 0,b \gt 0$
$\displaystyle \int_{0}^{1} \displaystyle \frac{1}{\{ax+b(1-x\}^2)} dx$
出典：2010年藤田保健衛生大学
大学入試問題#873「コメント欄が賑わいそう」 #東京理科大学(2022) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{4} \displaystyle \frac{2}{(x+1)(x+2)(x+3)} dx$
出典：2022年東京理科大学 大学入試問題
この動画を見る
$\displaystyle \int_{0}^{4} \displaystyle \frac{2}{(x+1)(x+2)(x+3)} dx$
出典：2022年東京理科大学 大学入試問題
#自治医科大学2015 #極限
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#自治医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty } \{\sqrt{ (n+2)(n+3) }-\sqrt{ (n-2)(n-3) }\}$
出典：2015年自治医科大学
この動画を見る
$\displaystyle \lim_{ n \to \infty } \{\sqrt{ (n+2)(n+3) }-\sqrt{ (n-2)(n-3) }\}$
出典：2015年自治医科大学
#関西学院大学2012 #不定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#関西学院大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int x^m log$ $x$ $dx(m \neq -1)$
出典：2012年関西学院大学
この動画を見る
$\displaystyle \int x^m log$ $x$ $dx(m \neq -1)$
出典：2012年関西学院大学
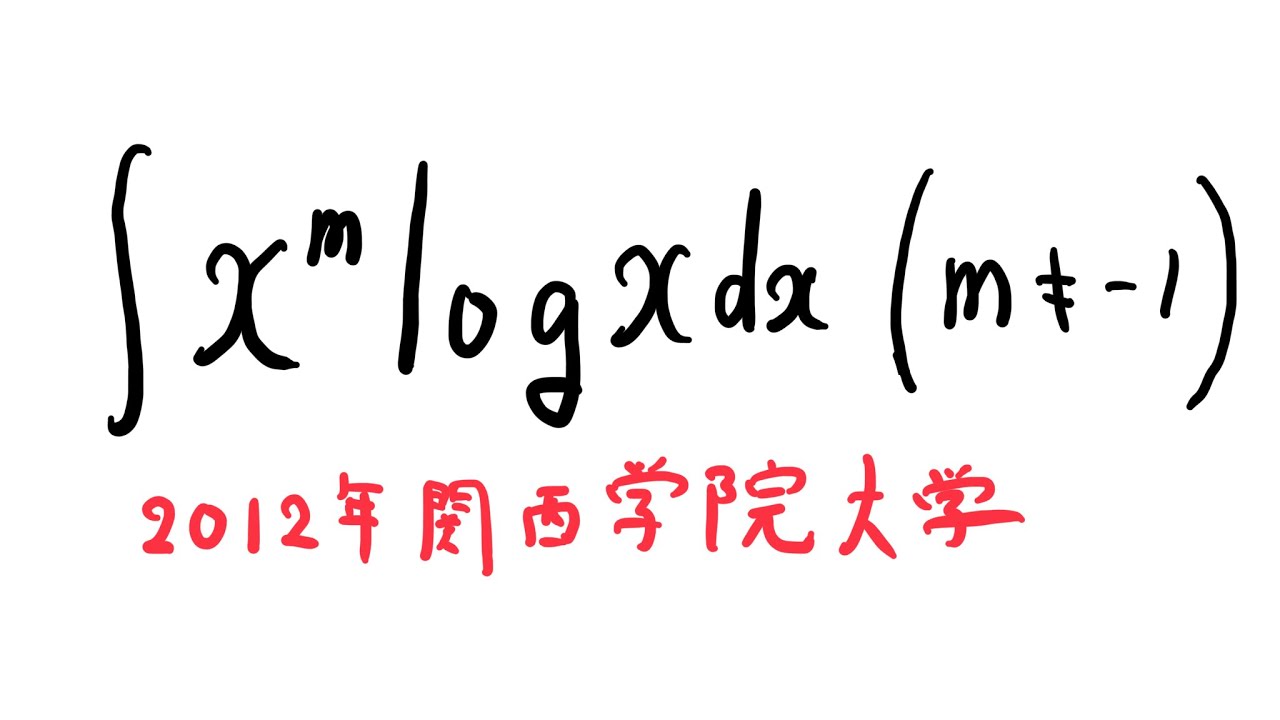
#関西大学2012 #三角関数
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#関西大学
指導講師：
ますただ
問題文全文(内容文)：
$x-y=\displaystyle \frac{\pi}{3}$のとき
$\displaystyle \frac{\sin x-\sin y}{\cos x+\cos y}$を求めよ。
出典：2012年関西大学
この動画を見る
$x-y=\displaystyle \frac{\pi}{3}$のとき
$\displaystyle \frac{\sin x-\sin y}{\cos x+\cos y}$を求めよ。
出典：2012年関西大学
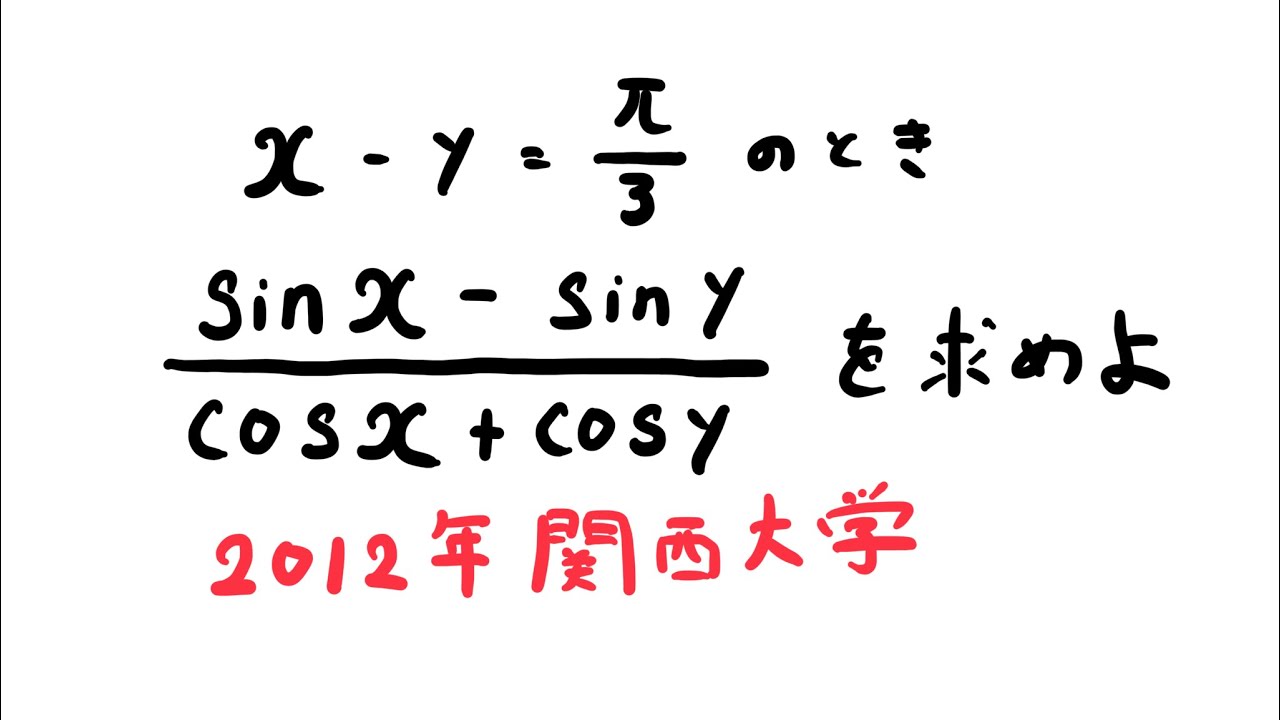
大学入試問題#872「受験生は一度は解くべき」 #東北大学医学部AO(2019) #極限
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$a$を実数とする。
次の極限を求めよ。
$\displaystyle \lim_{ n \to \infty } (1+a^{2n})^{\frac{1}{n}}$
出典：2019年東北大学医学部AO
この動画を見る
$a$を実数とする。
次の極限を求めよ。
$\displaystyle \lim_{ n \to \infty } (1+a^{2n})^{\frac{1}{n}}$
出典：2019年東北大学医学部AO
#青山学院大2019 #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#青山学院大学
指導講師：
ますただ
問題文全文(内容文)：
以下の不定積分を解け。
$\displaystyle \int \sin x \sin 2x$ $dx$
出典：2019年青山学院大学
この動画を見る
以下の不定積分を解け。
$\displaystyle \int \sin x \sin 2x$ $dx$
出典：2019年青山学院大学
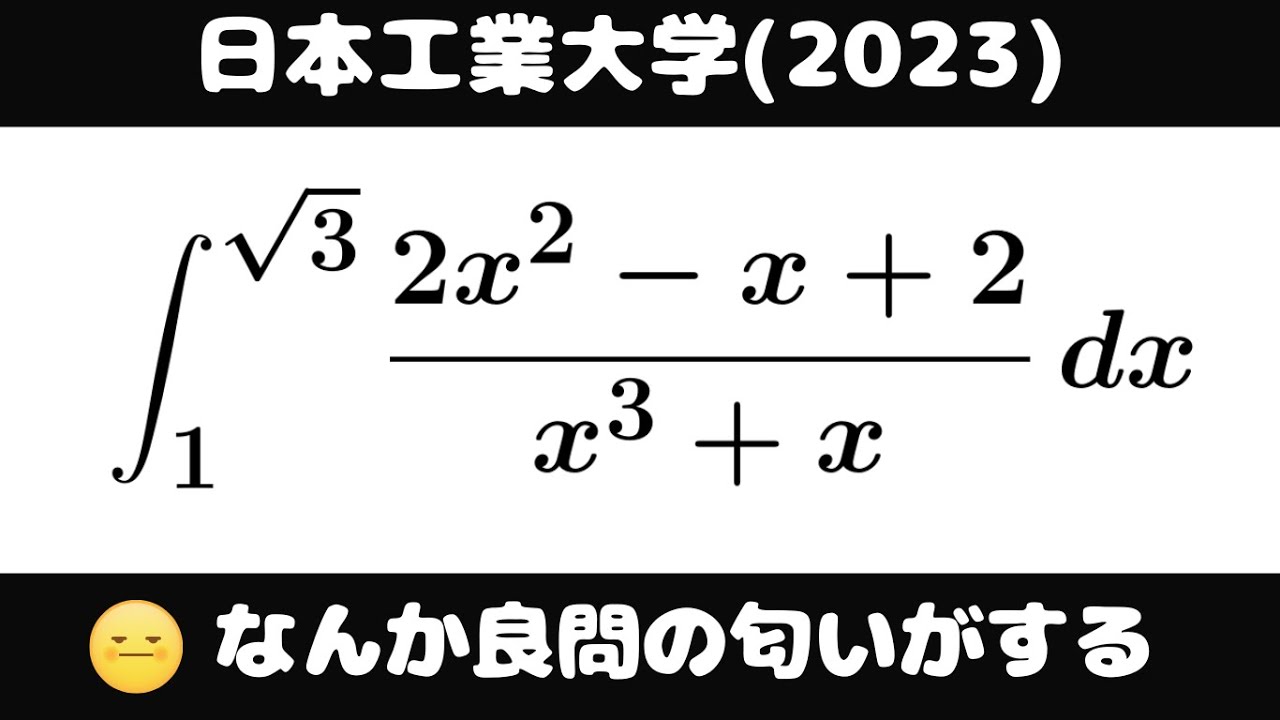
大学入試問題#871「初手が大事な基本問題」 #日本工業大学(2023) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{\sqrt{ 3 }} \displaystyle \frac{2x^2-x+2}{x^3+x} dx$
出典：2023年日本工業大学
この動画を見る
$\displaystyle \int_{1}^{\sqrt{ 3 }} \displaystyle \frac{2x^2-x+2}{x^3+x} dx$
出典：2023年日本工業大学
#自治医科大(2015) #定積分 #Shorts
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#自治医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \frac{35}{2}\displaystyle \int_{0}^{\frac{\pi}{2}} \sin^7x$ $dx$
出典：2015年自治医科大学
この動画を見る
$\displaystyle \frac{35}{2}\displaystyle \int_{0}^{\frac{\pi}{2}} \sin^7x$ $dx$
出典：2015年自治医科大学
#青山学院大学(2006) #定積分 #Shorts
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#青山学院大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{log3} (e^x+e^{2x}-2e^{-x}) dx$
出典：2006年青山学院大学
この動画を見る
$\displaystyle \int_{0}^{log3} (e^x+e^{2x}-2e^{-x}) dx$
出典：2006年青山学院大学
大学入試問題#870「基本問題」 #東北大学医学部AO(2019) #数列
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$S_n=2a_n+3n$を満たす数列$\{a_n\}$の一般項$a_n$を求めよ。
出典：2019年東北大学医学部AO
この動画を見る
$S_n=2a_n+3n$を満たす数列$\{a_n\}$の一般項$a_n$を求めよ。
出典：2019年東北大学医学部AO
大学入試問題#868「ヒントがあれば、どうってことない」 #埼玉医科大学(2010) #式変形
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#埼玉医科大学
指導講師：
ますただ
問題文全文(内容文)：
$a \leq b \leq c$とする。
$\sqrt{ 10+\sqrt{ 24 }+\sqrt{ 40 }+\sqrt{ 60 } }=\sqrt{ a }+\sqrt{ b }+\sqrt{ c }=$であるとき、$a,b,c$の値を求めよ。
出典：2010年埼玉医科大学
この動画を見る
$a \leq b \leq c$とする。
$\sqrt{ 10+\sqrt{ 24 }+\sqrt{ 40 }+\sqrt{ 60 } }=\sqrt{ a }+\sqrt{ b }+\sqrt{ c }=$であるとき、$a,b,c$の値を求めよ。
出典：2010年埼玉医科大学
#68数学検定1級1次「答えはめっちゃスッキリ」 #定積分
単元：
#数学検定・数学甲子園・数学オリンピック等#数学検定#数学検定1級
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-1}^{ 1 } \displaystyle \frac{x^4+2x^3+4x^2+6x+2}{x^3+2x^2+2x+4}$ $dx$
出典：数検1級1次
この動画を見る
$\displaystyle \int_{-1}^{ 1 } \displaystyle \frac{x^4+2x^3+4x^2+6x+2}{x^3+2x^2+2x+4}$ $dx$
出典：数検1級1次
大学入試問題#867「これは、過去1番の難問かも」 #産業医科大学(2012) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ 1-2\sin 2x+3\cos^2x }$ $dx$
出典：2012年産業医科大学
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sqrt{ 1-2\sin 2x+3\cos^2x }$ $dx$
出典：2012年産業医科大学
大学入試問題#866「まあ、なんとかなるわな」 #東京女子医科大学(2005) #式変形
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$(1-\sqrt[ 3 ]{ 2 }+\sqrt[ 3 ]{ 4 })^8$を計算せよ
出典：2005年東京女子医科大学
この動画を見る
$(1-\sqrt[ 3 ]{ 2 }+\sqrt[ 3 ]{ 4 })^8$を計算せよ
出典：2005年東京女子医科大学
大学入試問題#865「中学生の問題か！？」 #岩手医科大学(2008) #方程式
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\begin{eqnarray}
\left\{
\begin{array}{l}
(x-1)(y-1)=9 \\
x+y-\sqrt{ x^2+xy+y^2 }=1
\end{array}
\right.
\end{eqnarray}$を解け。
出典：2008年岩手医科大学
この動画を見る
$\begin{eqnarray}
\left\{
\begin{array}{l}
(x-1)(y-1)=9 \\
x+y-\sqrt{ x^2+xy+y^2 }=1
\end{array}
\right.
\end{eqnarray}$を解け。
出典：2008年岩手医科大学
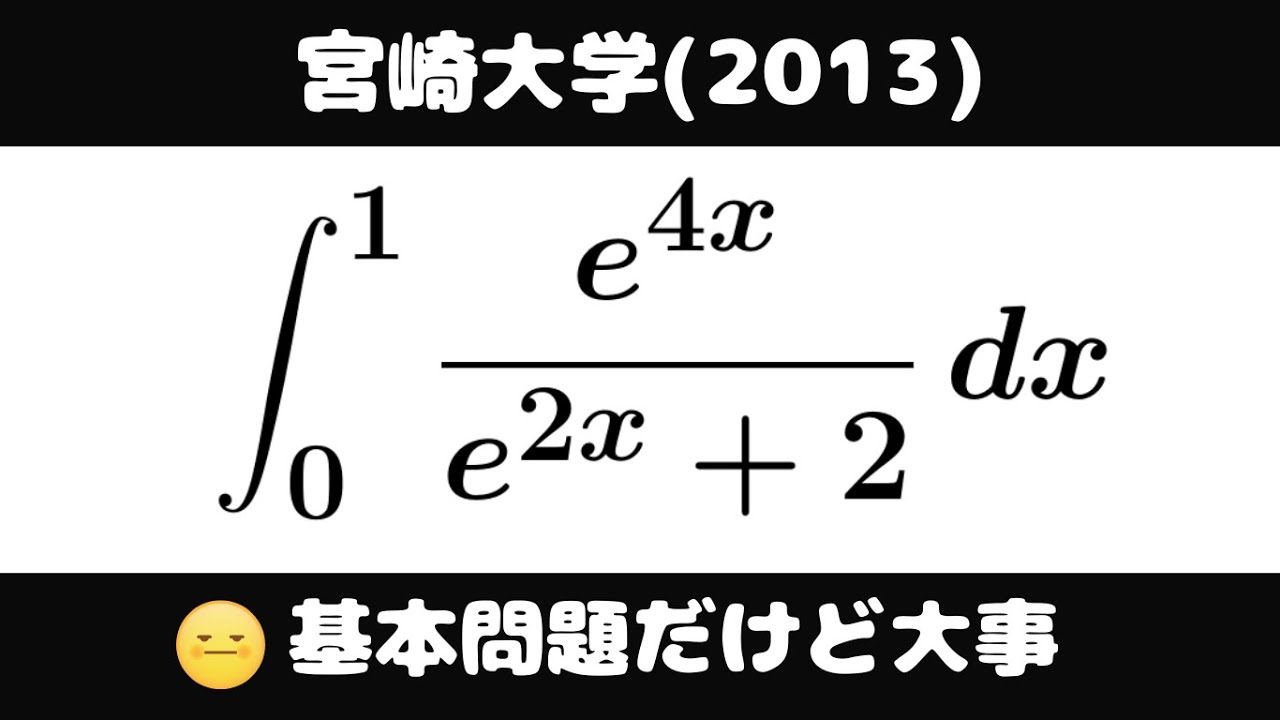
大学入試問題#864「基本に忠実に」 #宮崎大学(2013) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#宮崎大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1} \displaystyle \frac{e^{4x}}{e^{2x}+2} dx$
出典：2013年宮崎大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1} \displaystyle \frac{e^{4x}}{e^{2x}+2} dx$
出典：2013年宮崎大学 入試問題
#67数学検定1級1次「こんな問題で時間使いたくない」 #因数分解
単元：
#数学検定・数学甲子園・数学オリンピック等#数学検定#数学検定1級
指導講師：
ますただ
問題文全文(内容文)：
$xy(x^2-y^2)+yz(y^2-z^2)+zx(z^2-x^2)$を因数分解せよ
出典：数検1級1次
この動画を見る
$xy(x^2-y^2)+yz(y^2-z^2)+zx(z^2-x^2)$を因数分解せよ
出典：数検1級1次
#66数学検定1級1次過去問「怖いのは計算ミスのみ」 #式変形
単元：
#数学検定・数学甲子園・数学オリンピック等#数学検定#数学検定1級
指導講師：
ますただ
問題文全文(内容文)：
$(1-\sqrt[ 3 ]{ 2 }+\sqrt[ 3 ]{ 4 })^3$を簡単にせよ
出典：数検1級1次
この動画を見る
$(1-\sqrt[ 3 ]{ 2 }+\sqrt[ 3 ]{ 4 })^3$を簡単にせよ
出典：数検1級1次