 不定積分
不定積分
 不定積分
不定積分
大学入試問題#173 和歌山県立医科大学(2000) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#和歌山県立医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \cos^2x\ \sin^3x\ dx$を計算せよ。
出典:2000年和歌山県立医科大学 入試問題
この動画を見る
$\displaystyle \int \cos^2x\ \sin^3x\ dx$を計算せよ。
出典:2000年和歌山県立医科大学 入試問題
大学入試問題#170 東北大学(大正14年) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int log(log\ x)+\displaystyle \frac{1}{log\ x}\ dx$
出典:大正14年東北大学 入試問題
この動画を見る
$\displaystyle \int log(log\ x)+\displaystyle \frac{1}{log\ x}\ dx$
出典:大正14年東北大学 入試問題
大学入試問題#166 東京大学 改 (2022) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{4}}\cos\ x\ log(\cos\ x)dx$を求めよ。
出典:2022年東京大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}}\cos\ x\ log(\cos\ x)dx$を求めよ。
出典:2022年東京大学 入試問題
大学入試問題#142 広島市立大学(2014) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \cos\sqrt{ x }\ dx$を計算せよ。
出典:2014年広島市立大学 入試問題
この動画を見る
$\displaystyle \int \cos\sqrt{ x }\ dx$を計算せよ。
出典:2014年広島市立大学 入試問題
大学入試問題#134 京都工芸繊維大学(2018) 不定積分

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \{log(3+\cos^2\theta)\}\cos\theta d \theta$を計算せよ。
出典:2018年京都工芸繊維大学 入試問題
この動画を見る
$\displaystyle \int \{log(3+\cos^2\theta)\}\cos\theta d \theta$を計算せよ。
出典:2018年京都工芸繊維大学 入試問題
【数Ⅲ】微分法・積分法:<公式忘れても大丈夫!>三角関数の微積分 ~ぐるぐる回そう~

単元:
#微分とその応用#積分とその応用#色々な関数の導関数#不定積分#数学(高校生)#数Ⅲ
指導講師:
理数個別チャンネル
問題文全文(内容文):
三角形の重心における、頂点→重心:重心→中点の線分の比を導出する動画になります。
この動画を見る
三角形の重心における、頂点→重心:重心→中点の線分の比を導出する動画になります。
大学入試問題#126 慶應大学医学部(2005) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int (\displaystyle \frac{2}{x^3}+\displaystyle \frac{1}{x})\sin\ x\ dx$を計算せよ。
出典:2005年慶應義塾大学 入試問題
この動画を見る
$\displaystyle \int (\displaystyle \frac{2}{x^3}+\displaystyle \frac{1}{x})\sin\ x\ dx$を計算せよ。
出典:2005年慶應義塾大学 入試問題
大学入試問題#124 高知大学(2020) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#高知大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-\frac{7}{2}}^{\frac{9}{2}}\displaystyle \frac{2^x}{2^x+\sqrt{ 2 }}\ dx$を計算せよ。
出典:2020年高知大学 入試問題
この動画を見る
$\displaystyle \int_{-\frac{7}{2}}^{\frac{9}{2}}\displaystyle \frac{2^x}{2^x+\sqrt{ 2 }}\ dx$を計算せよ。
出典:2020年高知大学 入試問題
大学入試問題#112 琉球大学(1989) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#琉球大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$-1 \neq \alpha$:定数
$\displaystyle \int \displaystyle \frac{(log\ x)^\alpha}{x}\ log(\log\ x)dx$を計算せよ。
出典:1989年琉球大学 入試問題
この動画を見る
$-1 \neq \alpha$:定数
$\displaystyle \int \displaystyle \frac{(log\ x)^\alpha}{x}\ log(\log\ x)dx$を計算せよ。
出典:1989年琉球大学 入試問題
大学入試問題#98 千葉大学医学部(2018) 積分・極限
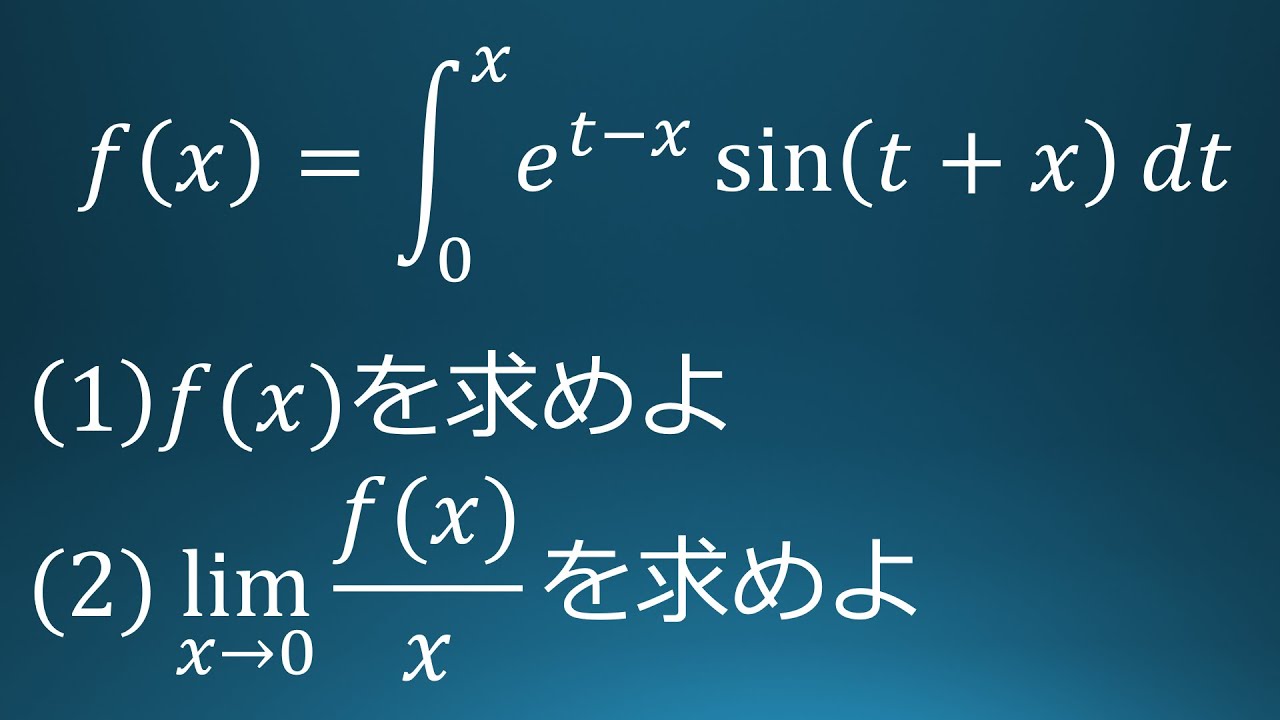
単元:
#大学入試過去問(数学)#関数と極限#積分とその応用#関数の極限#不定積分#定積分#学校別大学入試過去問解説(数学)#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
(1)
$f(x)=\displaystyle \int_{0}^{x}e^{t-x}\sin(t+x)dt$を求めよ。
(2)
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x}$を求めよ。
出典:2018年千葉大学 入試問題
この動画を見る
(1)
$f(x)=\displaystyle \int_{0}^{x}e^{t-x}\sin(t+x)dt$を求めよ。
(2)
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x}$を求めよ。
出典:2018年千葉大学 入試問題
大学入試問題#91 関西学院大学(2006) 不定積分
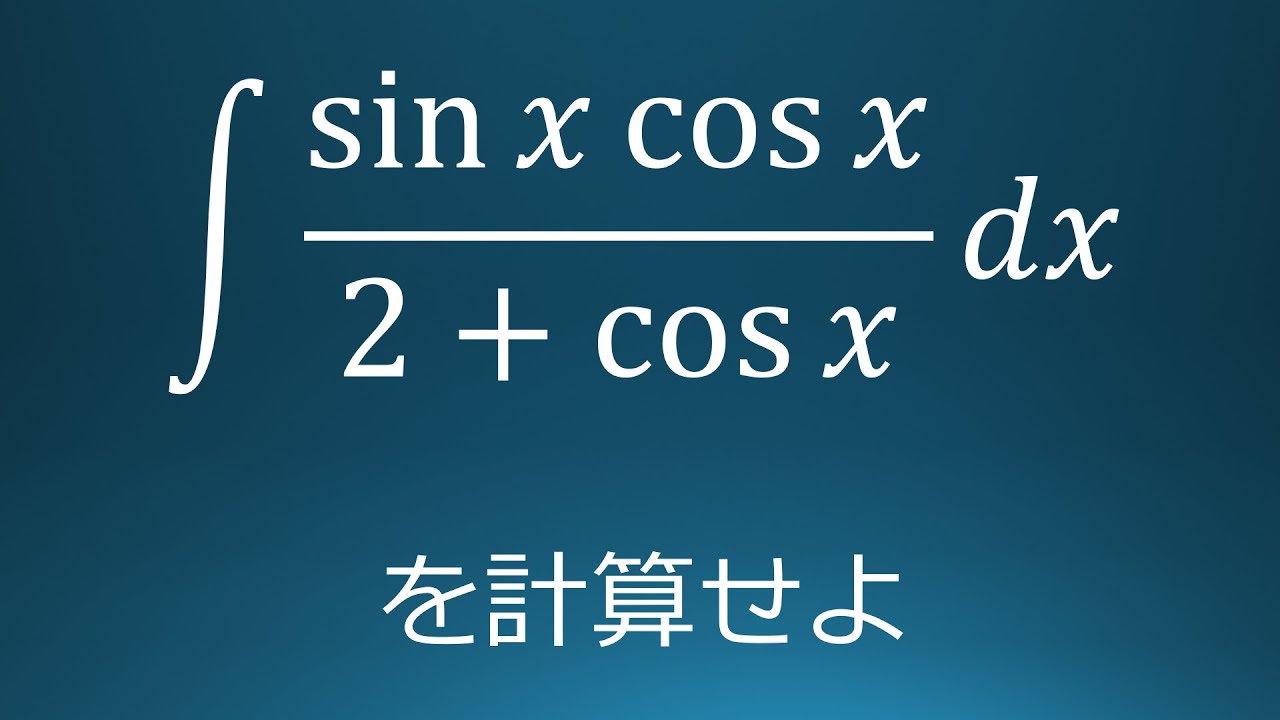
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#関西学院大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{\sin\ x\ \cos\ x}{2+\cos\ x}\ dx$を計算せよ。
出典:2006年関西学院大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{\sin\ x\ \cos\ x}{2+\cos\ x}\ dx$を計算せよ。
出典:2006年関西学院大学 入試問題
大学入試問題#89 信州大学(1988) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int (x^2+a^2)^{-\frac{3}{2}}dx$
$a \neq 0$を計算せよ。
出典:1988年信州大学 入試問題
この動画を見る
$\displaystyle \int (x^2+a^2)^{-\frac{3}{2}}dx$
$a \neq 0$を計算せよ。
出典:1988年信州大学 入試問題
大学入試問題#85 小樽商科大学(1988) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#小樽商科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \sin(log\ x)dx$を計算せよ。
出典:1988年小樽商科大学 入試問題
この動画を見る
$\displaystyle \int \sin(log\ x)dx$を計算せよ。
出典:1988年小樽商科大学 入試問題
大学入試問題#84 弘前大学(1986) 不定積分
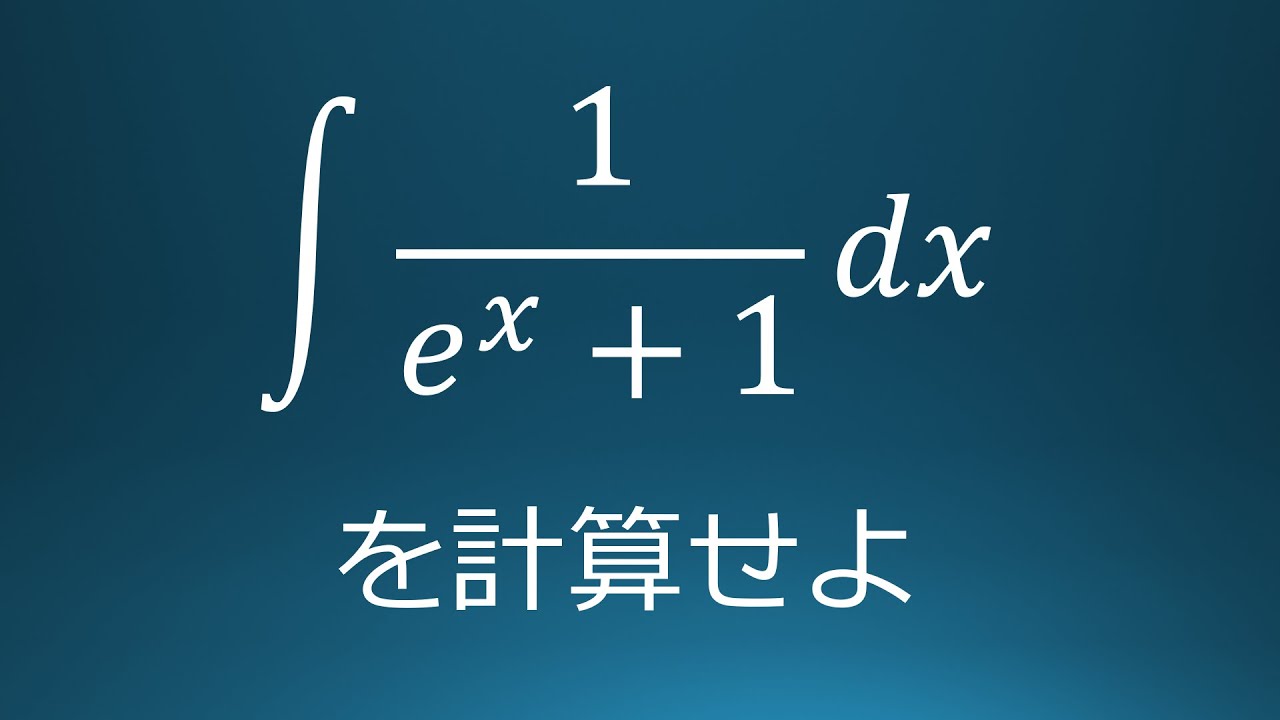
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#弘前大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{1}{e^x+1}\ dx$を計算せよ。
出典:1986年弘前大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{1}{e^x+1}\ dx$を計算せよ。
出典:1986年弘前大学 入試問題
大学入試問題#80 信州大学(2001) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ x+1 }+1}\ dx$を計算せよ。
出典:2001年信州大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ x+1 }+1}\ dx$を計算せよ。
出典:2001年信州大学 入試問題
#51 大学入試問題 新潟大学(2020) 定積分【King propertyっぽいけど・・・】
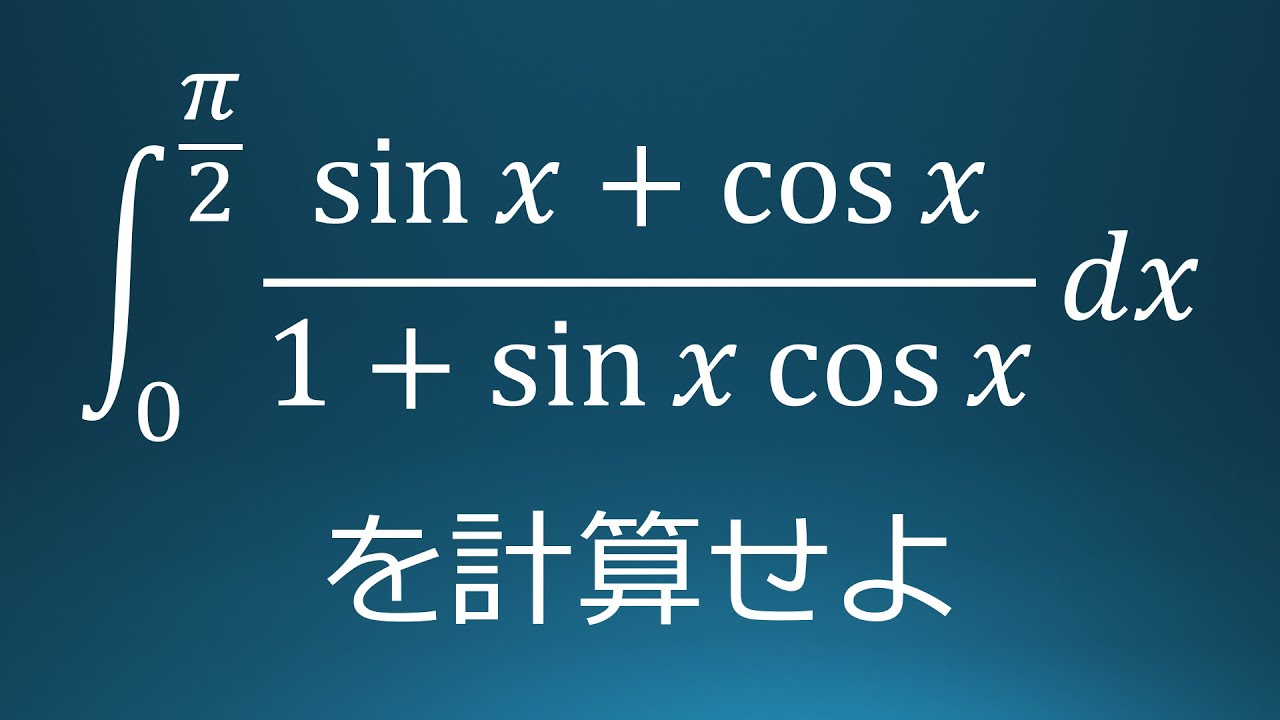
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#新潟大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x+\cos\ x}{1+\sin\ x\ \cos\ x}\ dx$を計算せよ。
出典:2020年新潟大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x+\cos\ x}{1+\sin\ x\ \cos\ x}\ dx$を計算せよ。
出典:2020年新潟大学 入試問題
#30 数検1級1次 過去問 複雑な定積分

単元:
#数学検定・数学甲子園・数学オリンピック等#積分とその応用#不定積分#定積分#数学検定#数学検定1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
定積分
$\displaystyle \int_{-1}^{1}\displaystyle \frac{x^4+2x^3+4x^2+6x+2}{x^3+2x^2+2x+4}\ dx$を計算せよ。
この動画を見る
定積分
$\displaystyle \int_{-1}^{1}\displaystyle \frac{x^4+2x^3+4x^2+6x+2}{x^3+2x^2+2x+4}\ dx$を計算せよ。
大学入試問題#42 慶應義塾大学(2021) 絶対値の定積分

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#慶應義塾大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$a:$実数
$f(x)=|x|+a$に対して$\displaystyle \int_{-5}^{5}|f(x)|dx$が最小となる$a$の値を求めよ。
出典:2021年慶應義塾大学 入試問題
この動画を見る
$a:$実数
$f(x)=|x|+a$に対して$\displaystyle \int_{-5}^{5}|f(x)|dx$が最小となる$a$の値を求めよ。
出典:2021年慶應義塾大学 入試問題
練習問題51 広島大学 改 不定積分

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int\ 2(x-1)e^{-x}\cos\ x\ dx$
$\displaystyle \int\ e^{-x}\cos\ x\ dx=\displaystyle \frac{e^{-x}}{2}(\sin\ x-\cos\ x)+c$
$\displaystyle \int\ e^{-x}\sin\ x\ dx=-\displaystyle \frac{e^{-x}}{2}(\sin\ x+\cos\ x)+c$
$c$は積分定数
出典:広島大学
この動画を見る
$\displaystyle \int\ 2(x-1)e^{-x}\cos\ x\ dx$
$\displaystyle \int\ e^{-x}\cos\ x\ dx=\displaystyle \frac{e^{-x}}{2}(\sin\ x-\cos\ x)+c$
$\displaystyle \int\ e^{-x}\sin\ x\ dx=-\displaystyle \frac{e^{-x}}{2}(\sin\ x+\cos\ x)+c$
$c$は積分定数
出典:広島大学
国際数学オリンピック 積和

単元:
#積分とその応用#不定積分#定積分#面積・体積・長さ・速度#数学(高校生)#数Ⅲ
指導講師:
鈴木貫太郎
問題文全文(内容文):
$\cos\dfrac{\pi}{7}-\cos\dfrac{2\pi}{7}+\cos\dfrac{3\pi}{7}=\dfrac{1}{2}$を示せ.
国際数学オリンピック
この動画を見る
$\cos\dfrac{\pi}{7}-\cos\dfrac{2\pi}{7}+\cos\dfrac{3\pi}{7}=\dfrac{1}{2}$を示せ.
国際数学オリンピック
練習問題1(数検準1級、教員採用試験 レベル)

単元:
#数学検定・数学甲子園・数学オリンピック等#積分とその応用#不定積分#その他#数学検定#数学検定準1級#数学(高校生)#数Ⅲ#教員採用試験
指導講師:
ますただ
問題文全文(内容文):
(1)$\int \frac{x}{cos^2x} dx$
(2)$\int \frac{x}{sin^2x} dx$
この動画を見る
(1)$\int \frac{x}{cos^2x} dx$
(2)$\int \frac{x}{sin^2x} dx$
円周率πが無理数であることの証明(数III)
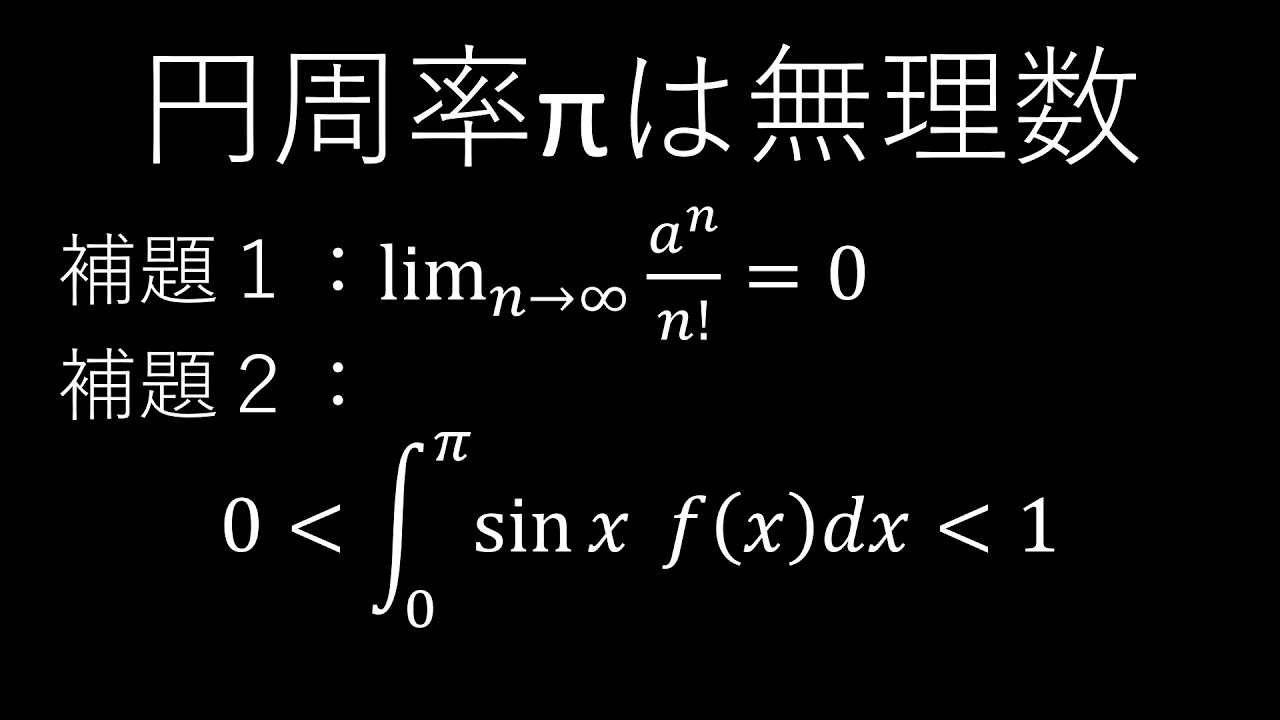
単元:
#関数と極限#積分とその応用#数列の極限#不定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
定理(1947,IvanNiren)
πは無理数である
補題1
${}^∀a \in \mathbb{R}$ , $\displaystyle \lim_{ n \to \infty } \frac{a^n}{n!}=0$ $(n \in \mathbb{N})$
補題2
$f(x)=\frac{1}{n!}p^nx^n(\pi - x)^n$ $(p,n \in \mathbb{N})$
nが十分大きいとき
$0 < \int_0^{\pi} f(x) dx < 1$
この動画を見る
定理(1947,IvanNiren)
πは無理数である
補題1
${}^∀a \in \mathbb{R}$ , $\displaystyle \lim_{ n \to \infty } \frac{a^n}{n!}=0$ $(n \in \mathbb{N})$
補題2
$f(x)=\frac{1}{n!}p^nx^n(\pi - x)^n$ $(p,n \in \mathbb{N})$
nが十分大きいとき
$0 < \int_0^{\pi} f(x) dx < 1$
20年5月数学検定準1級1次試験(積分)

単元:
#数学検定・数学甲子園・数学オリンピック等#積分とその応用#不定積分#定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
5⃣
(1)$\int \frac{dx}{sin2x}$
(2)$\int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \frac{dx}{sin2x}$
この動画を見る
5⃣
(1)$\int \frac{dx}{sin2x}$
(2)$\int_{\frac{\pi}{4}}^{\frac{\pi}{3}} \frac{dx}{sin2x}$
【高校数学】部分分数分解の分母に二乗があるパターン

単元:
#恒等式・等式・不等式の証明#数列とその和(等差・等比・階差・Σ)#積分とその応用#不定積分#数学(高校生)
指導講師:
受験メモ山本
問題文全文(内容文):
部分分数分解の分母に二乗がある場合の解説動画です
この動画を見る
部分分数分解の分母に二乗がある場合の解説動画です
【数Ⅲ-148】積分特訓③

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
数Ⅲ(積分特訓③)
①$\int\frac{1}{sinx}dx$
➁$\int\sqrt{x^2+1}\ dx$
この動画を見る
数Ⅲ(積分特訓③)
①$\int\frac{1}{sinx}dx$
➁$\int\sqrt{x^2+1}\ dx$
【数Ⅲ-147】積分特訓②

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
数Ⅲ(積分特訓➁)
①$\int\frac{1}{e^x-e^{-x}}dx$
➁$\int\frac{e^x-e^{-x}}{e^x+e^{-x}}dx$
③$\int\cos^5xdx$
この動画を見る
数Ⅲ(積分特訓➁)
①$\int\frac{1}{e^x-e^{-x}}dx$
➁$\int\frac{e^x-e^{-x}}{e^x+e^{-x}}dx$
③$\int\cos^5xdx$
【数Ⅲ-146】積分特訓①

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
④$\int \frac{2x+3}{\sqrt{x^2+3x-4}} dx$
⑤$\int x^2\log xdx$
⑥$\int\sin^2\frac{x}{2}dx$
この動画を見る
④$\int \frac{2x+3}{\sqrt{x^2+3x-4}} dx$
⑤$\int x^2\log xdx$
⑥$\int\sin^2\frac{x}{2}dx$
【数Ⅲ-145】指数関数・対数関数の積分

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
数Ⅲ(指数関数・対数関数の積分)
Q.次の不定積分を求めよ
①$\int \frac{1}{x(\log x)^2} dx$
➁$\int \frac{\log x}{x(\log x+1)^2} dx$
③$\int \frac{e^{3x}}{\sqrt{e^x+1}} dx$
この動画を見る
数Ⅲ(指数関数・対数関数の積分)
Q.次の不定積分を求めよ
①$\int \frac{1}{x(\log x)^2} dx$
➁$\int \frac{\log x}{x(\log x+1)^2} dx$
③$\int \frac{e^{3x}}{\sqrt{e^x+1}} dx$
【数Ⅲ-144】三角関数の積分②

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
数Ⅲ(三角関数の積分➁)
Q.次の不定積分を求めよ。
⑤$\int cos3xcos2xdx$
⑥$\int cos4xsin2xdx$
⑦$\int sinxsin2xdx$
⑧$\int sin3θ cosθdθ$
この動画を見る
数Ⅲ(三角関数の積分➁)
Q.次の不定積分を求めよ。
⑤$\int cos3xcos2xdx$
⑥$\int cos4xsin2xdx$
⑦$\int sinxsin2xdx$
⑧$\int sin3θ cosθdθ$
【数Ⅲ-143】三角関数の積分①

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
とある男が授業をしてみた
問題文全文(内容文):
数Ⅲ(三角関数の積分①)
Q.次の不定積分を求めよ
⑤$\int cos^2xdx$
⑥$\int sin^3xdx$
⑦$\int cosx sin^5xdx$
この動画を見る
数Ⅲ(三角関数の積分①)
Q.次の不定積分を求めよ
⑤$\int cos^2xdx$
⑥$\int sin^3xdx$
⑦$\int cosx sin^5xdx$
