 ますただ
ますただ
 ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
三重大学(2016) #Shorts

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#三重大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2016年三重大学
この動画を見る
出典:2016年三重大学
【数学ゴールデン】2巻と5巻で紹介された整数問題を解いてみた #数学ゴールデン #数学オリンピック #整数問題

単元:
#数A#整数の性質#約数・倍数・整数の割り算と余り・合同式#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
黒板に1以上100以下の整数が1つずつ書かれている。
黒板から整数
黒板に書かれている整数が1つだけになったとき、その整数は平方数でないことを示せ。
残り1つになった整数は平方数でない
この動画を見る
黒板に1以上100以下の整数が1つずつ書かれている。
黒板から整数
黒板に書かれている整数が1つだけになったとき、その整数は平方数でないことを示せ。
残り1つになった整数は平方数でない
兵庫医科大学(2021) #Shorts
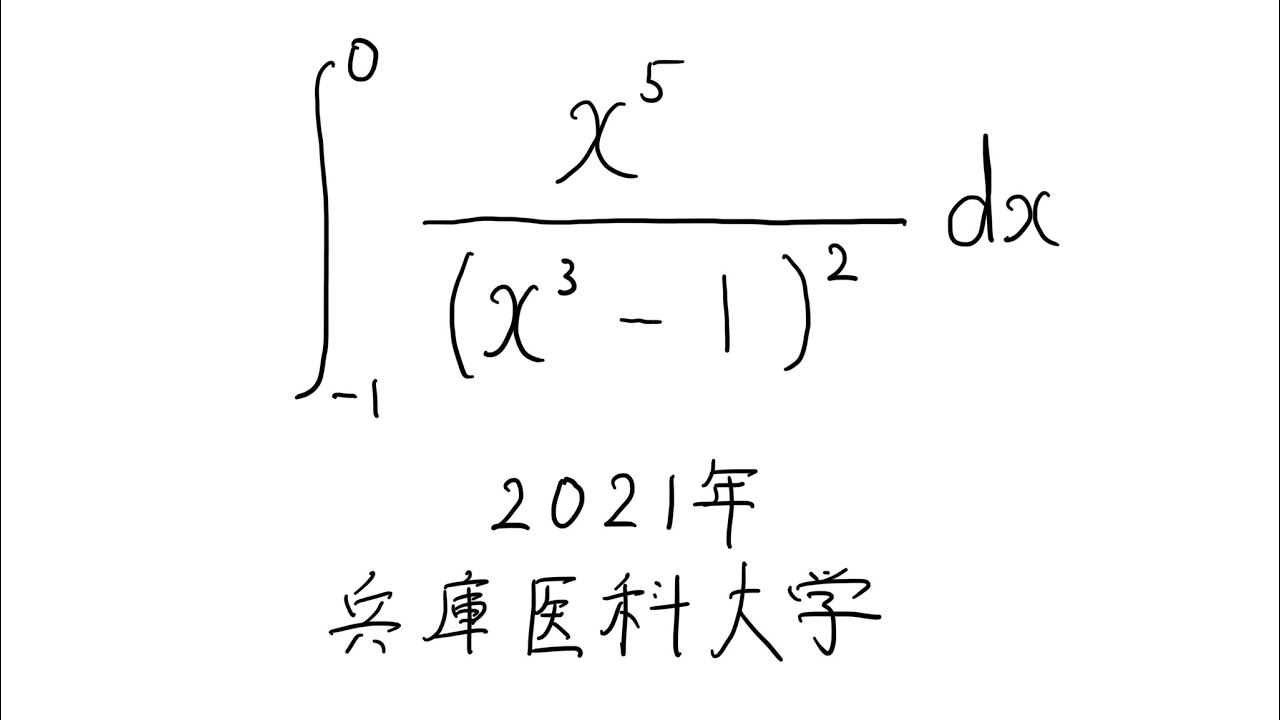
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#兵庫医科大学
指導講師:
ますただ
問題文全文(内容文):
出典:2021年兵庫医科大学 入試問題
この動画を見る
出典:2021年兵庫医科大学 入試問題
大学入試問題#615「ラッキー問題?」 東京工業大学(1976) #積分方程式
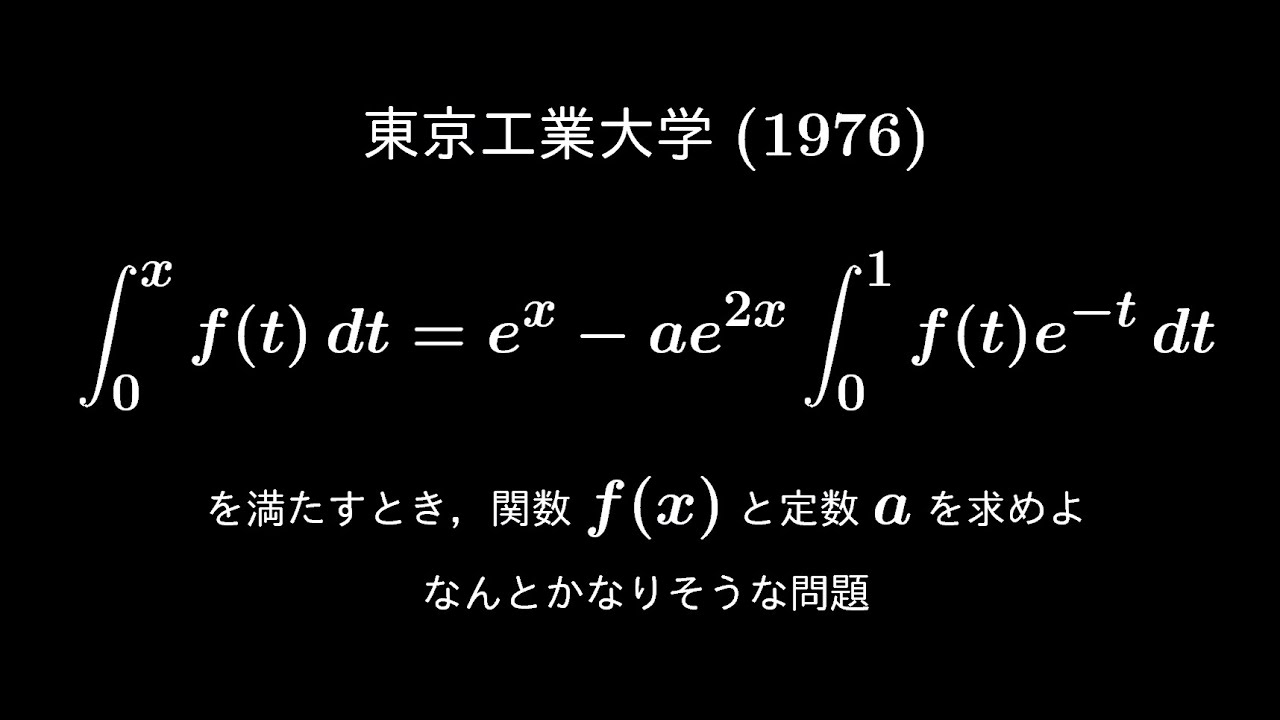
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京工業大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
関数
出典:1976年東京工業大学 入試問題
この動画を見る
関数
出典:1976年東京工業大学 入試問題
大学入試問題#614「これは、時間内で解くのは大変かもしれない」 立命館大学(2023) #曲線の長さ

単元:
#数Ⅱ#大学入試過去問(数学)#図形と方程式#学校別大学入試過去問解説(数学)#数学(高校生)#立命館大学
指導講師:
ますただ
問題文全文(内容文):
次の曲線の長さ
出典:2023年立命館大学 入試問題
この動画を見る
次の曲線の長さ
出典:2023年立命館大学 入試問題
大学入試問題#613「微分してたら、時間かかるだろうな~~」 慶應義塾大学(1996)

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:1996年慶應義塾大学 入試問題
この動画を見る
出典:1996年慶應義塾大学 入試問題
大学入試問題#612 早稲田大学(2021)

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
正の実数
出典:2021年早稲田大学 入試問題
この動画を見る
正の実数
出典:2021年早稲田大学 入試問題
大学入試問題#612「チャートにありそう」 慶応義塾大学(2011) #2次不等式

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
すべての実数
出典:2011年慶應義塾大学 入試問題
この動画を見る
すべての実数
出典:2011年慶應義塾大学 入試問題
大学入試問題#611「ストレートに解けそう」 千葉大学(2014) #定積分

福島県立医科大学(2005) #Shorts

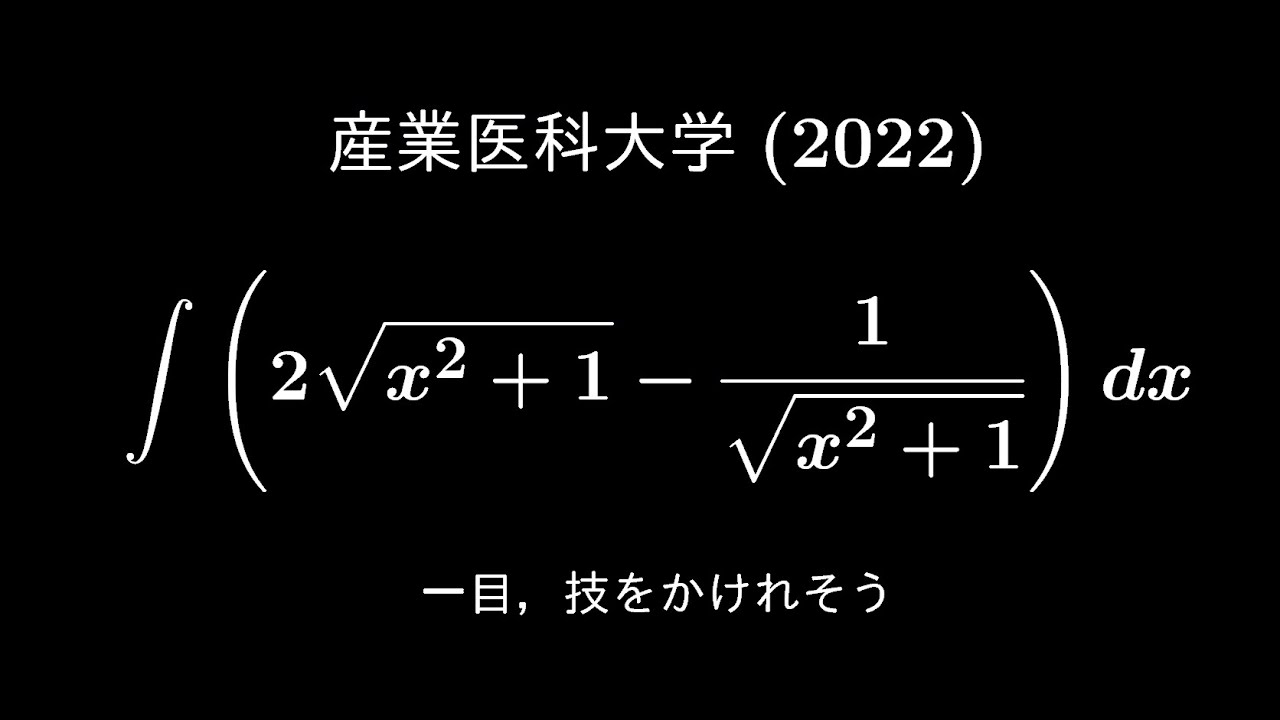
大学入試問題#610「初見だと涙がでるかも」 産業医科大学(2022) #不定積分
大学入試問題#609「落とすと落ちる良問」 早稲田大学(2023) #整数問題

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
出典:2023年早稲田大学 入試問題
この動画を見る
出典:2023年早稲田大学 入試問題
大学入試問題#608「絶対値・・・・」 横浜市立大学(2009) #定積分

単元:
#数Ⅰ#数Ⅱ#数と式#実数と平方根(循環小数・有理数・無理数・絶対値・平方根計算・2重根号)#三角関数#三角関数とグラフ#加法定理とその応用#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2009年横浜市立大学 入試問題
この動画を見る
出典:2009年横浜市立大学 入試問題
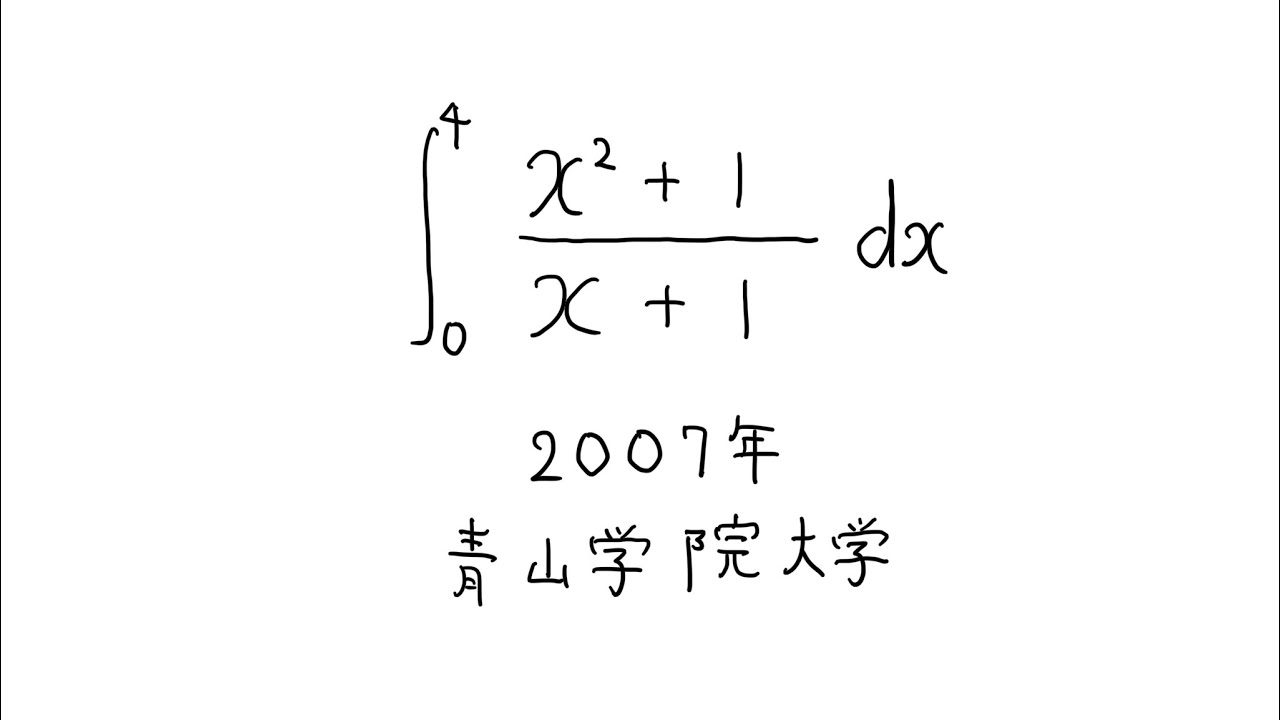
大学入試問題#607「やばい、忙しすぎる」 青山学院大学(2007) #定積分

単元:
#数Ⅱ#三角関数#三角関数とグラフ#微分とその応用#積分とその応用#微分法#定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2007年青山学院大学 入試問題
この動画を見る
出典:2007年青山学院大学 入試問題
大学入試問題#606「見るからに落とせない気がする」 福島大学(2012) #方程式

単元:
#数Ⅰ#2次関数#実数と平方根(循環小数・有理数・無理数・絶対値・平方根計算・2重根号)#2次方程式と2次不等式#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
出典:2012年福島大学 入試問題
この動画を見る
出典:2012年福島大学 入試問題
高校の範囲で解ける積分 By 英語orドイツ語シはBかHか さん #定積分

青山学院大学(2007年) #Shorts
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#青山学院大学
指導講師:
ますただ
問題文全文(内容文):
出典:2007年青山学院大学
この動画を見る
出典:2007年青山学院大学
大学入試問題#昭和大#604「nの計算丁寧に」 昭和大学医学部(2014) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#昭和大学
指導講師:
ますただ
問題文全文(内容文):
出典:2014年昭和大学 入試問題
この動画を見る
出典:2014年昭和大学 入試問題
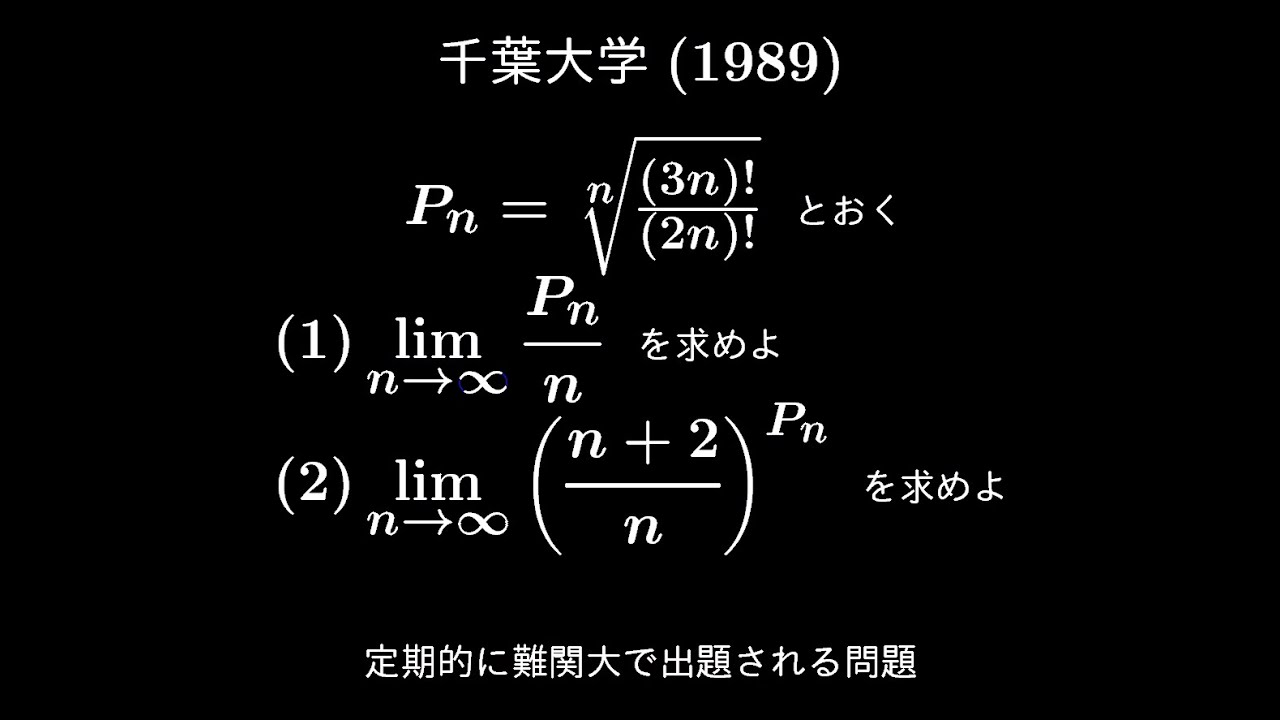
大学入試問題#603「もう飽きた?」 千葉大学(1989) #極限
単元:
#大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
(1)
(2)
出典:1989年千葉大学 入試問題
この動画を見る
(1)
(2)
出典:1989年千葉大学 入試問題
大学入試問題#601「これは落としたくないかも」 広島大学後期(2014) #定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#広島大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2014年広島大学後期 入試問題
この動画を見る
出典:2014年広島大学後期 入試問題
大学入試問題#600「合同式使ってみた」 山梨大学医学部(2014) #整式

単元:
#数Ⅱ#大学入試過去問(数学)#式と証明#複素数と方程式#整式の除法・分数式・二項定理#剰余の定理・因数定理・組み立て除法と高次方程式#学校別大学入試過去問解説(数学)#山梨大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
出典:2014年山梨大学 入試問題
この動画を見る
出典:2014年山梨大学 入試問題
大学入試問題#599「King-propertyは使ってません」 南山大学(2013) 定積分
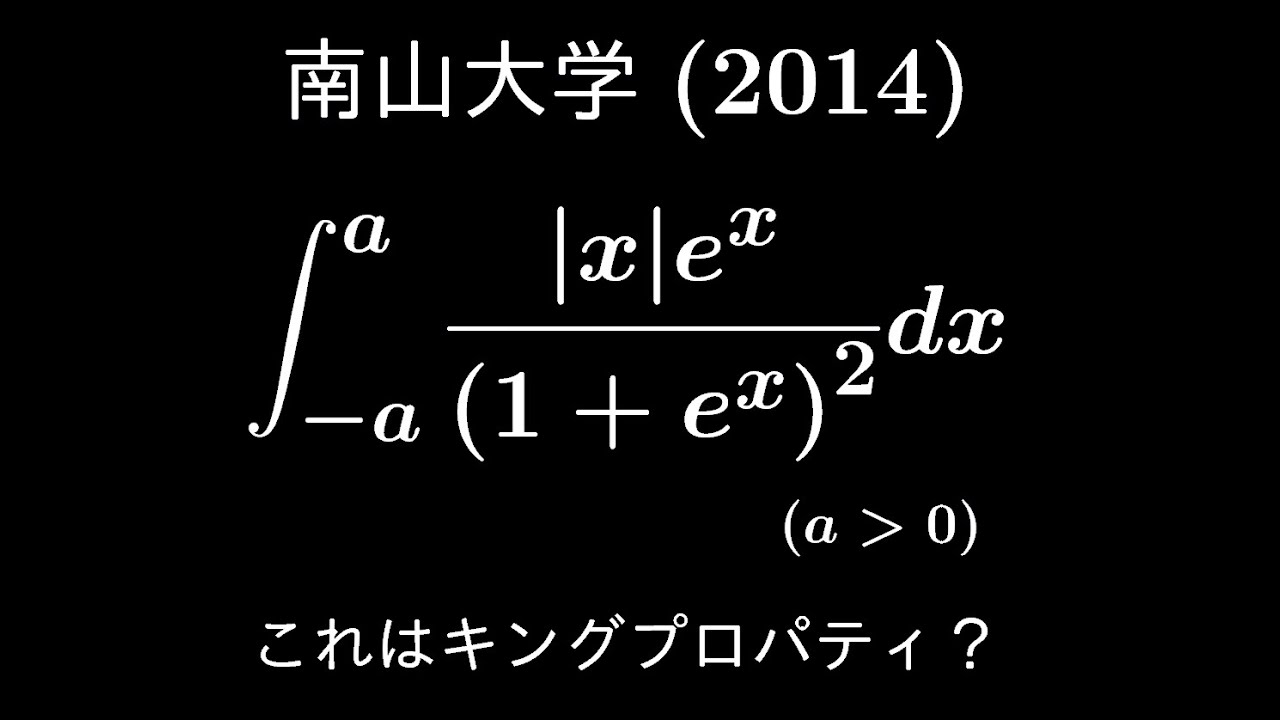
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#南山大学
指導講師:
ますただ
問題文全文(内容文):
出典:2013年南山大学 入試問題
この動画を見る
出典:2013年南山大学 入試問題
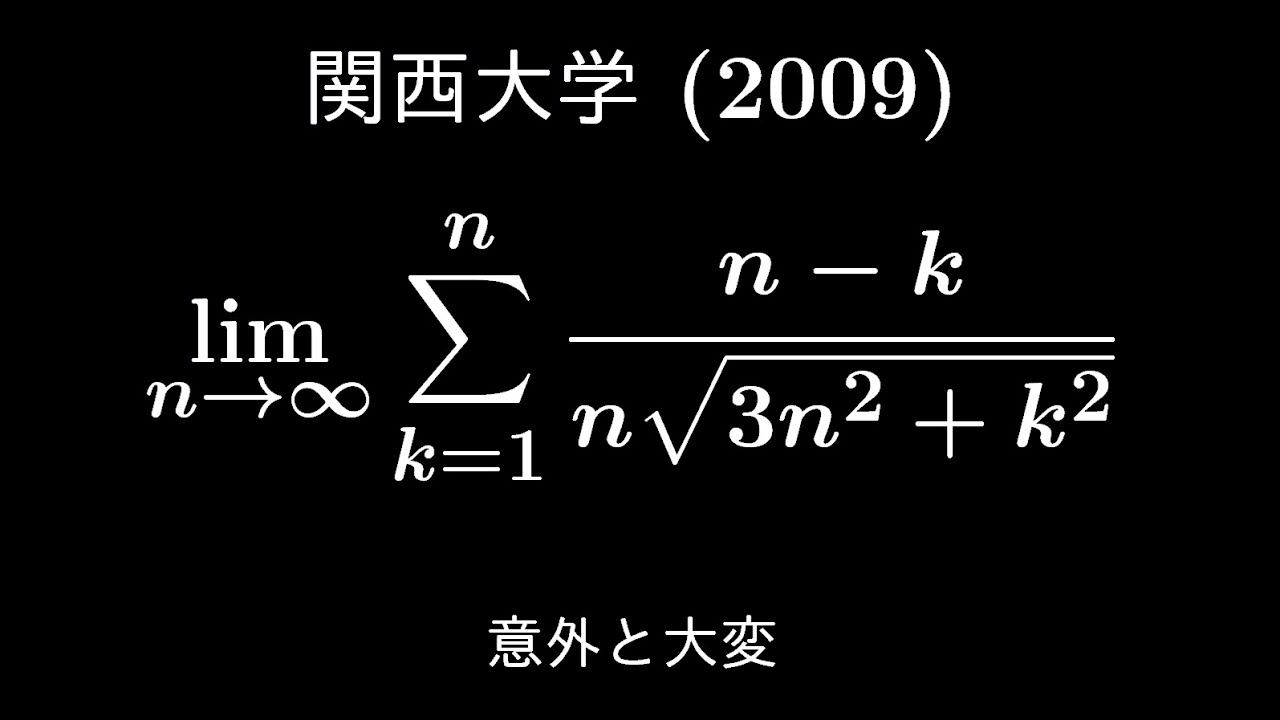
大学入試問題#598「計算が大変でした」 関西大学(2009) #区分求積法
単元:
#大学入試過去問(数学)#関数と極限#積分とその応用#数列の極限#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#関西大学
指導講師:
ますただ
問題文全文(内容文):
出典:2009年関西大学 入試問題
この動画を見る
出典:2009年関西大学 入試問題
大学入試問題#597「難しくはないと思う」 大阪教育大学(2014) #命題②

単元:
#数Ⅰ#大学入試過去問(数学)#数と式#集合と命題(集合・命題と条件・背理法)#学校別大学入試過去問解説(数学)#数学(高校生)#大阪教育大学
指導講師:
ますただ
問題文全文(内容文):
出典:2015年大阪教育大学 入試問題
この動画を見る
出典:2015年大阪教育大学 入試問題
電気通信大学2014年 #定積分 #Shorts

大学入試問題#596「√2のいとこ」 大阪教育大学(2014) #命題①

単元:
#数Ⅰ#大学入試過去問(数学)#数と式#集合と命題(集合・命題と条件・背理法)#学校別大学入試過去問解説(数学)#数学(高校生)#大阪教育大学
指導講師:
ますただ
問題文全文(内容文):
出典:2015年大阪教育大学 入試問題
この動画を見る
出典:2015年大阪教育大学 入試問題
電気通信大学2014年 #極限 #Shorts
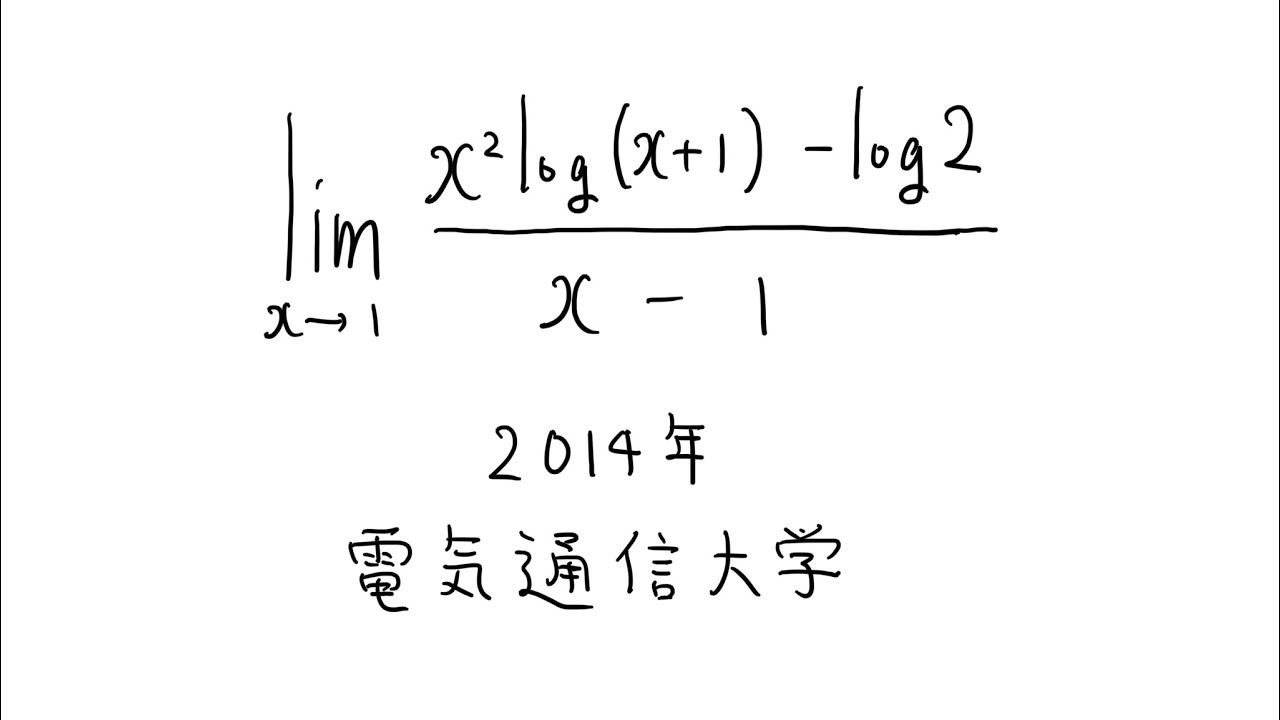
単元:
#大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師:
ますただ
問題文全文(内容文):
出典:2014年電気通信大学
この動画を見る
出典:2014年電気通信大学
大学入試問題#595「山口大学に初挑戦!」 山口大学(2014) #数列

単元:
#大学入試過去問(数学)#数列#漸化式#関数と極限#数列の極限#学校別大学入試過去問解説(数学)#数学(高校生)#山口大学#数B#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2014年山口大学 入試問題
この動画を見る
出典:2014年山口大学 入試問題
大学入試問題#594「やばいのは見た目だけ」 東京帝国大学(1926) #複素数

単元:
#大学入試過去問(数学)#複素数平面#複素数平面#学校別大学入試過去問解説(数学)#数学(高校生)#数C
指導講師:
ますただ
問題文全文(内容文):
出典:1926年東京帝国大学医学部 入試問題
この動画を見る
出典:1926年東京帝国大学医学部 入試問題
大学入試問題#593「計算ミスに気をつける」 福島大学(1987) #極限
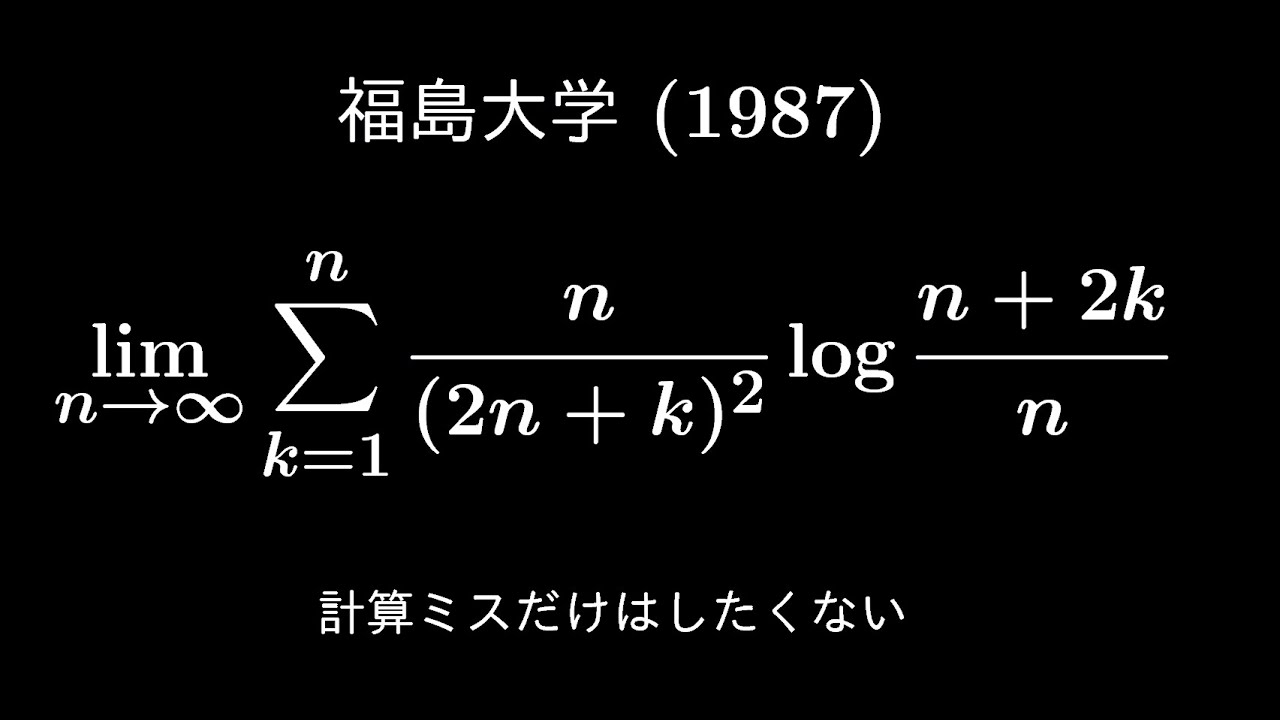
単元:
#大学入試過去問(数学)#積分とその応用#数列の極限#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#福島大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:1987年福島大学 入試問題
この動画を見る
出典:1987年福島大学 入試問題



