ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！
職業：数学者
職歴：高校非常勤（院生時代）、高専、大学 准教授
趣味：
①将棋 棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀 アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス 中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。
大学入試問題#180 秋田県立大学(2004) 定積分 ウォリス積分②
単元：
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1}(1-x^2)^4\ dx$
出典：2004年秋田県立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}(1-x^2)^4\ dx$
出典：2004年秋田県立大学 入試問題
大学入試問題#179 秋田県立大学(2004) 定積分 ウォリス積分①
単元：
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1}(1-x^2)^3 dx$
出典：2004年秋田県立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}(1-x^2)^3 dx$
出典：2004年秋田県立大学 入試問題
大学入試問題#178 東京大学昭和10年 定積分 King property
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\pi}\displaystyle \frac{x\ \sin\ x}{1+\cos^2x}\ dx$を計算せよ。
出典：昭和10年東京大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\pi}\displaystyle \frac{x\ \sin\ x}{1+\cos^2x}\ dx$を計算せよ。
出典：昭和10年東京大学 入試問題
大学入試問題#177 東京都市大学(2017) 定積分
単元：
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{3}\displaystyle \frac{x}{\sqrt{ x+1 }}\ dx$
出典：2017年東京都市大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{3}\displaystyle \frac{x}{\sqrt{ x+1 }}\ dx$
出典：2017年東京都市大学 入試問題
大学入試問題#176 日本医科大学(2019) 不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#日本医科大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \sqrt{ x^2+1 }\ dx$
出典：2019年日本医科大学 入試問題
この動画を見る
$\displaystyle \int \sqrt{ x^2+1 }\ dx$
出典：2019年日本医科大学 入試問題
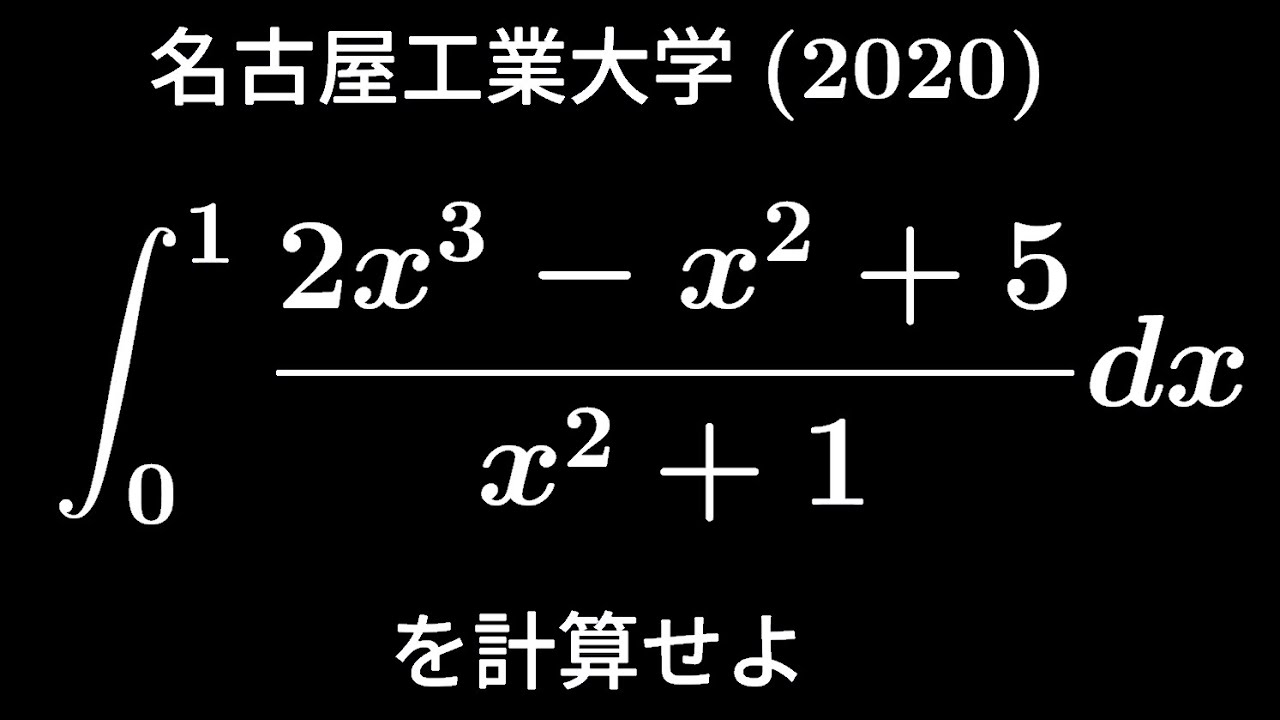
大学入試問題#175 名古屋工業大学2020 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#名古屋工業大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1}\displaystyle \frac{2x^3-x^2+5}{x^2+1}\ dx$を計算せよ。
出典：2020年名古屋工業大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}\displaystyle \frac{2x^3-x^2+5}{x^2+1}\ dx$を計算せよ。
出典：2020年名古屋工業大学 入試問題
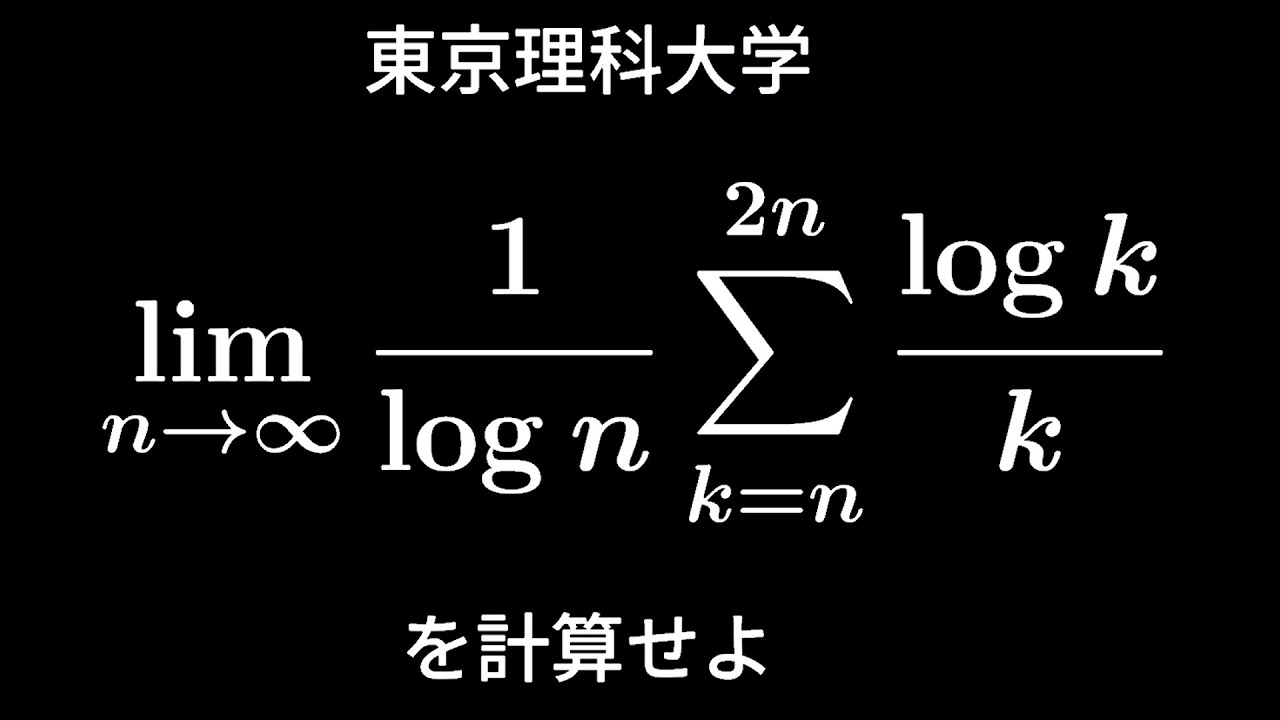
大学入試問題#174 東京理科大学 区分求積法
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty }\displaystyle \frac{1}{log\ n}\displaystyle \sum_{k=1}^{2n}\displaystyle \frac{log\ k}{k}$を求めよ。
出典：東京理科大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty }\displaystyle \frac{1}{log\ n}\displaystyle \sum_{k=1}^{2n}\displaystyle \frac{log\ k}{k}$を求めよ。
出典：東京理科大学 入試問題
大学入試問題#173 和歌山県立医科大学(2000) 不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#和歌山県立医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \cos^2x\ \sin^3x\ dx$を計算せよ。
出典：2000年和歌山県立医科大学 入試問題
この動画を見る
$\displaystyle \int \cos^2x\ \sin^3x\ dx$を計算せよ。
出典：2000年和歌山県立医科大学 入試問題
#1大学編入試験問題 電通大(2021) 重積分 変数変換
単元：
#積分とその応用#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$D:x^2+y^2 \leqq x$
$\displaystyle \int \displaystyle \int_{D}\ x\sqrt{ x }\ dx\ dy$を計算せよ。
出典：2021年電通大学編入試験
この動画を見る
$D:x^2+y^2 \leqq x$
$\displaystyle \int \displaystyle \int_{D}\ x\sqrt{ x }\ dx\ dy$を計算せよ。
出典：2021年電通大学編入試験
【概要欄必読】大学入試問題#172 東京都市大学 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\sqrt{ 3 }}{2}}(x+4x^3)\sqrt{ 1+4x^2 }\ dx$
出典：東京都市大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\sqrt{ 3 }}{2}}(x+4x^3)\sqrt{ 1+4x^2 }\ dx$
出典：東京都市大学 入試問題
大学入試問題#171 横浜国立大学 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{4}}\cos3x・\sin2x・\tan\ x\ dx$を求めよ。
出典：横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}}\cos3x・\sin2x・\tan\ x\ dx$を求めよ。
出典：横浜国立大学 入試問題
大学入試問題#170 東北大学(大正14年) 不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int log(log\ x)+\displaystyle \frac{1}{log\ x}\ dx$
出典：大正14年東北大学 入試問題
この動画を見る
$\displaystyle \int log(log\ x)+\displaystyle \frac{1}{log\ x}\ dx$
出典：大正14年東北大学 入試問題
大学入試問題#169 愛知教育大学(2013) 区分求積法
単元：
#大学入試過去問(数学)#関数と極限#積分とその応用#数列の極限#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#愛知教育大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty }\displaystyle \sum_{k=1}^n\displaystyle \frac{1}{n+k}(log(n+k)-log\ n)$を求めよ。
出典：2013年愛知教育大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty }\displaystyle \sum_{k=1}^n\displaystyle \frac{1}{n+k}(log(n+k)-log\ n)$を求めよ。
出典：2013年愛知教育大学 入試問題
大学入試問題#168 広島市立大学(2020) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{2}x\sqrt{ 2-x }\ dx$を求めよ。
出典：2020年広島市立大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{2}x\sqrt{ 2-x }\ dx$を求めよ。
出典：2020年広島市立大学 入試問題
【概要欄に問題掲載】大学入試問題#167 岡山県立大学2020 数列
単元：
#大学入試過去問(数学)#数列#数列とその和（等差・等比・階差・Σ）#学校別大学入試過去問解説(数学)#数学(高校生)#数B#岡山県立大学
指導講師：
ますただ
問題文全文(内容文)：
$S_n=2a_n-n^2$のとき
一般項$a_n$を求めよ。
出典：2020年岡山県立大学 入試問題
この動画を見る
$S_n=2a_n-n^2$のとき
一般項$a_n$を求めよ。
出典：2020年岡山県立大学 入試問題
大学入試問題#166 東京大学 改 (2022) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{4}}\cos\ x\ log(\cos\ x)dx$を求めよ。
出典：2022年東京大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}}\cos\ x\ log(\cos\ x)dx$を求めよ。
出典：2022年東京大学 入試問題
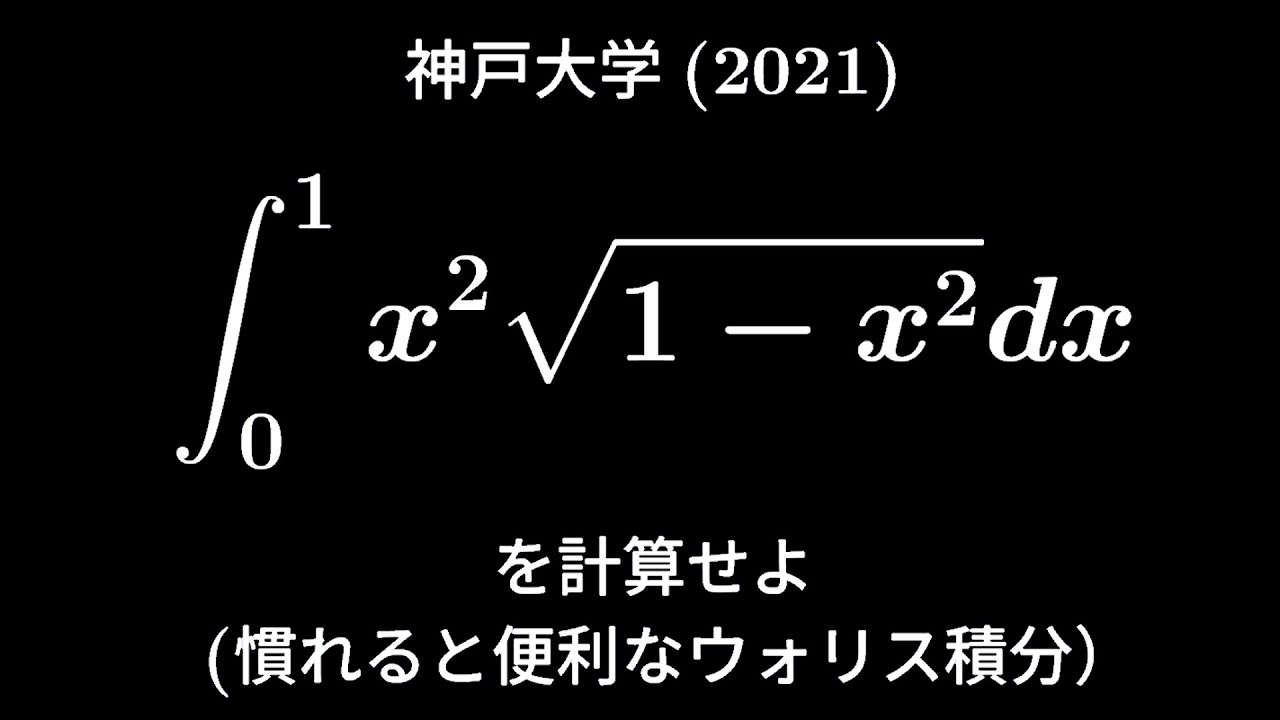
大学入試問題#165 神戸大学(2021) ウォリス積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#神戸大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1}x^2\sqrt{ 1-x^2 }\ dx$を求めよ。
（ウォリス積分）
出典：2021年神戸大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}x^2\sqrt{ 1-x^2 }\ dx$を求めよ。
（ウォリス積分）
出典：2021年神戸大学 入試問題
大学入試問題#164 防衛医科大学(2020) 指数の計算
単元：
#数Ⅱ#大学入試過去問(数学)#指数関数と対数関数#指数関数#学校別大学入試過去問解説(数学)#数学(高校生)#防衛医科大学
指導講師：
ますただ
問題文全文(内容文)：
$1 \leqq m,n$実数
$m^{\frac{n}{3}}+m^{-\frac{n}{3}}=\displaystyle \frac{3\sqrt{ 2 }}{2}$のとき
$mm^n-m^{-n}$の値を求めよ。
出典：2020年防衛医科大学 入試問題
この動画を見る
$1 \leqq m,n$実数
$m^{\frac{n}{3}}+m^{-\frac{n}{3}}=\displaystyle \frac{3\sqrt{ 2 }}{2}$のとき
$mm^n-m^{-n}$の値を求めよ。
出典：2020年防衛医科大学 入試問題
大学入試問題#163 信州大学(2004) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{2}\displaystyle \frac{log\ x}{x^3}\ dx$
出典：2004年信州大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{2}\displaystyle \frac{log\ x}{x^3}\ dx$
出典：2004年信州大学 入試問題
大学入試問題#161 大阪市立大学(1999) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#大阪市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{2}}(\displaystyle \frac{x\ \sin\ x}{1+\cos\ x}+\displaystyle \frac{x\ \cos\ x}{1+\sin\ x})dx$を計算せよ。
出典：1999年大阪市立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}}(\displaystyle \frac{x\ \sin\ x}{1+\cos\ x}+\displaystyle \frac{x\ \cos\ x}{1+\sin\ x})dx$を計算せよ。
出典：1999年大阪市立大学 入試問題
大学入試問題#160 名古屋市立大学(2020) 三角関数
単元：
#数Ⅱ#大学入試過去問(数学)#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#数学(高校生)#名古屋市立大学
指導講師：
ますただ
問題文全文(内容文)：
$0 \leqq \theta \leqq \pi$
$\cos4\theta=\cos2\theta$をみたす$\theta$をすべて求めよ。
出典：2020年名古屋市立大学 入試問題
この動画を見る
$0 \leqq \theta \leqq \pi$
$\cos4\theta=\cos2\theta$をみたす$\theta$をすべて求めよ。
出典：2020年名古屋市立大学 入試問題
大学入試問題#159 横浜国立大学 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#横浜国立大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{4}\displaystyle \frac{dx}{\sqrt{ 3-\sqrt{ x } }}$を計算せよ
出典：横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{4}\displaystyle \frac{dx}{\sqrt{ 3-\sqrt{ x } }}$を計算せよ
出典：横浜国立大学 入試問題
ハルハル様の作成問題 手筋連発
単元：
#数Ⅱ#式と証明#整数の性質#約数・倍数・整数の割り算と余り・合同式#恒等式・等式・不等式の証明#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$x$についての方程式
$x^3+x^2-x-5=0$の最小の実数解を$\alpha$とする。
$\alpha^5$の整数部分を求めよ。
この動画を見る
$x$についての方程式
$x^3+x^2-x-5=0$の最小の実数解を$\alpha$とする。
$\alpha^5$の整数部分を求めよ。
大学入試問題#158 名古屋市立大学(2020) ２項展開の応用
単元：
#数Ⅱ#大学入試過去問(数学)#式と証明#整式の除法・分数式・二項定理#学校別大学入試過去問解説(数学)#数学(高校生)#名古屋市立大学
指導講師：
ますただ
問題文全文(内容文)：
$(x+2y)^2(x+2y+3z)^4$を展開した時
$x^4y^2,x^3y^2z$の係数をそれぞれ求めよ。
出典：2020年名古屋市立大学 入試問題
この動画を見る
$(x+2y)^2(x+2y+3z)^4$を展開した時
$x^4y^2,x^3y^2z$の係数をそれぞれ求めよ。
出典：2020年名古屋市立大学 入試問題
大学入試問題#157 旭川医科大学(2014) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#旭川医科大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{1}log(1+x^2)dx$を求めよ。
出典：2014年旭川医科大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}log(1+x^2)dx$を求めよ。
出典：2014年旭川医科大学 入試問題
20和歌山県教員採用試験 数列、整数問題
単元：
#数列#数列とその和（等差・等比・階差・Σ）#その他#数学(高校生)#数B#教員採用試験
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \frac{15}{8},\displaystyle \frac{165}{11},\displaystyle \frac{315}{14},\displaystyle \frac{465}{17},・・・$の一般項$a_n$が自然数となるもののうち最大となるときの$n$を求めよ。
出典：2020年教育採用試験和歌山
この動画を見る
$\displaystyle \frac{15}{8},\displaystyle \frac{165}{11},\displaystyle \frac{315}{14},\displaystyle \frac{465}{17},・・・$の一般項$a_n$が自然数となるもののうち最大となるときの$n$を求めよ。
出典：2020年教育採用試験和歌山
大学入試問題#156 昭和大学(2019) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#昭和大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{1}^{2}\displaystyle \frac{dx}{x^2-2x+2}$を求めよ。
出典：2019年昭和大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{2}\displaystyle \frac{dx}{x^2-2x+2}$を求めよ。
出典：2019年昭和大学 入試問題
大学入試問題#155 琉球大学(1987) 極限
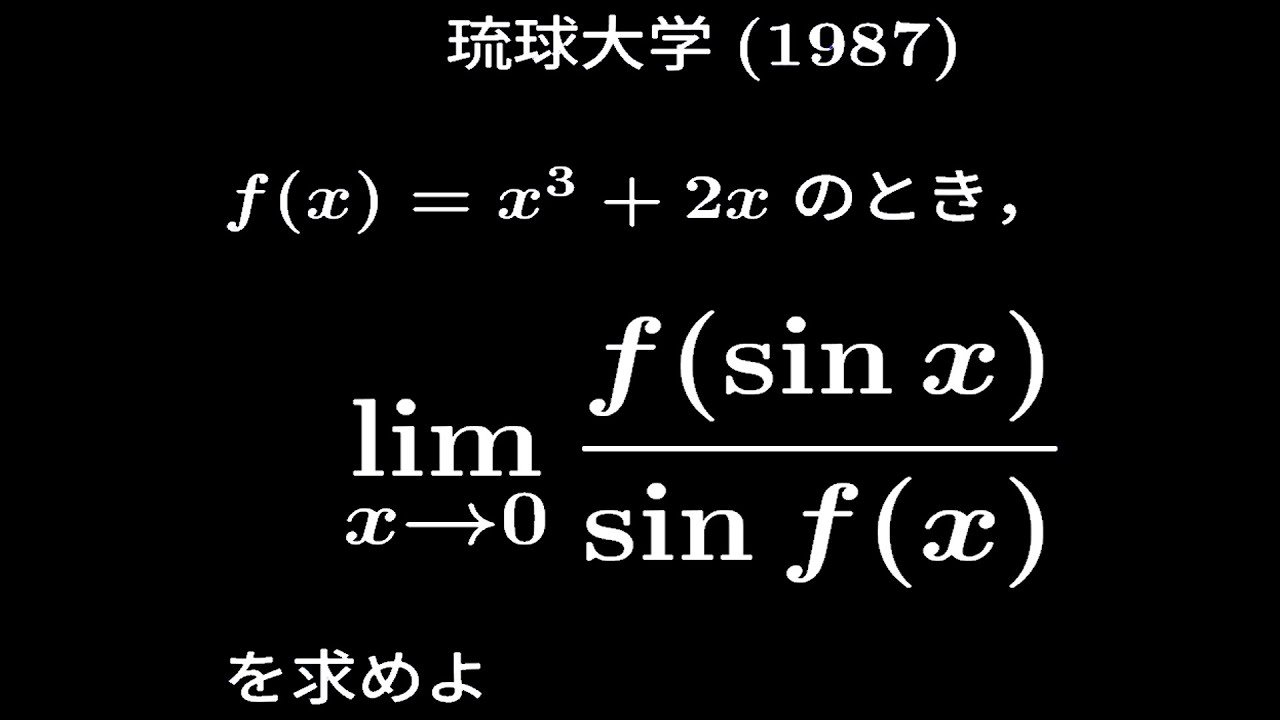
単元：
#大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#琉球大学#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
$f(x)=x^3+2x$のとき
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(\sin\ x)}{\sin\ f(x)}$を求めよ。
出典：1987年琉球大学 入試問題
この動画を見る
$f(x)=x^3+2x$のとき
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(\sin\ x)}{\sin\ f(x)}$を求めよ。
出典：1987年琉球大学 入試問題
大学入試問題#154 横浜市立大学医学部(2017) 定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#横浜市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x}{\cos\ 2x}\ dx$を求めよ。
出典：2017年横浜市立大学医学部 入試問題
この動画を見る
$\displaystyle \int_{\frac{\pi}{3}}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x}{\cos\ 2x}\ dx$を求めよ。
出典：2017年横浜市立大学医学部 入試問題
大学入試問題#153 東京医科大学(2017) 微積の応用
単元：
#大学入試過去問(数学)#微分とその応用#積分とその応用#微分法#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学#東京医科大学
指導講師：
ますただ
問題文全文(内容文)：
$x \gt 0$
$f(x)=\displaystyle \int_{1}^{x}\displaystyle \frac{x+4t}{\sqrt{ 3x^4+t^4 }}\ dt$において$f'(x)$を求めよ。
出典：2017年東京医科大学 入試問題
この動画を見る
$x \gt 0$
$f(x)=\displaystyle \int_{1}^{x}\displaystyle \frac{x+4t}{\sqrt{ 3x^4+t^4 }}\ dt$において$f'(x)$を求めよ。
出典：2017年東京医科大学 入試問題