 不定積分
不定積分
 不定積分
不定積分
大学入試問題#439「国立大学らしい綺麗な問題」 群馬大学(2015) #微分方程式
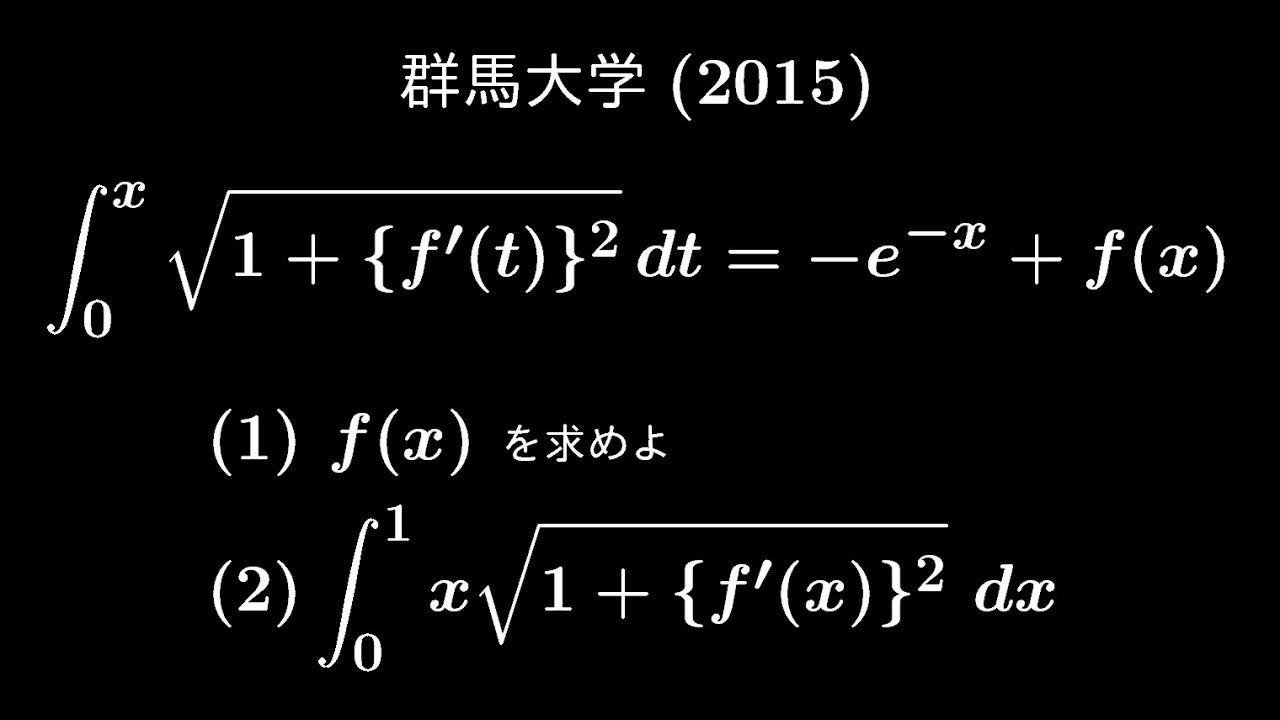
単元:
#大学入試過去問(数学)#微分とその応用#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#群馬大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{x} \sqrt{ 1+\{f'(t)\}^2 }dt=-e^{-x}+f(x)$
(1)
$f(x)$を求めよ。
(2)
$\displaystyle \int_{0}^{1} x\sqrt{ 1+\{f'(x)\}^2 }\ dx$
出典:2015年群馬大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{x} \sqrt{ 1+\{f'(t)\}^2 }dt=-e^{-x}+f(x)$
(1)
$f(x)$を求めよ。
(2)
$\displaystyle \int_{0}^{1} x\sqrt{ 1+\{f'(x)\}^2 }\ dx$
出典:2015年群馬大学 入試問題
【数Ⅲ】部分積分【公式不要!微分して被積分関数になるものを作り出せ】

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
めいちゃんねる
問題文全文(内容文):
$ (1)\displaystyle \int x\cos x dxを求めよ.$
$ (2)\displaystyle \int (2x+1)\sin 3x dxを求めよ.$
$ (3)\displaystyle \int \log x dx,\displaystyle \int x\log x dx,\displaystyle \int \log(2x+1)dxを求めよ.$
$ (4)\displaystyle \int_{0}^{\pi} x^2\sin x dxを求めよ.$
$ (5)\displaystyle \int_{0}^{\pi} e^x \sin x dxを求めよ.$
この動画を見る
$ (1)\displaystyle \int x\cos x dxを求めよ.$
$ (2)\displaystyle \int (2x+1)\sin 3x dxを求めよ.$
$ (3)\displaystyle \int \log x dx,\displaystyle \int x\log x dx,\displaystyle \int \log(2x+1)dxを求めよ.$
$ (4)\displaystyle \int_{0}^{\pi} x^2\sin x dxを求めよ.$
$ (5)\displaystyle \int_{0}^{\pi} e^x \sin x dxを求めよ.$
大学入試問題#435「基本的な性質が盛り沢山の良問!!」 信州大学(2014) #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{3\sin\theta-\sin3\theta}{1+\cos\theta}d\theta$
出典:2014年信州大学理学部後期 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{3\sin\theta-\sin3\theta}{1+\cos\theta}d\theta$
出典:2014年信州大学理学部後期 入試問題
大学入試問題#394「積サーで紹介されてたから解いてみた」 東京大学(大正時代) #不定積分

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int (log(log\ x)+\displaystyle \frac{1}{log\ x})dx$
出典:大正時代東京大学 入試問題
この動画を見る
$\displaystyle \int (log(log\ x)+\displaystyle \frac{1}{log\ x})dx$
出典:大正時代東京大学 入試問題
大学入試問題#393「サクッと式変形」 #東京都市大学(2011) #定積分

単元:
#積分とその応用#不定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{2} \displaystyle \frac{x^3-x^2-2}{x^2+2} dx$
出典:2011年東京都市大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{2} \displaystyle \frac{x^3-x^2-2}{x^2+2} dx$
出典:2011年東京都市大学 入試問題
大学入試問題#388「大学名に再生回数を託してみた」 #福島県立医科大学2009 #部分積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#福島県立医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{\tan^2x}{\cos^2x} dx$
出典:2009年福島県立医科大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{\tan^2x}{\cos^2x} dx$
出典:2009年福島県立医科大学 入試問題
大学入試問題#387「覚えておきたい計算方法」 #北里大学医学部2011 #定積分

単元:
#積分とその応用#不定積分#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{2}^{4} \displaystyle \frac{dx}{(x-1)^2(x+2)}$
出典:2011年北里大学医学部 入試問題
この動画を見る
$\displaystyle \int_{2}^{4} \displaystyle \frac{dx}{(x-1)^2(x+2)}$
出典:2011年北里大学医学部 入試問題
福田の1.5倍速演習〜合格する重要問題009〜九州大学2015年度理系数学第2問〜関数の増減と区分求積

単元:
#大学入試過去問(数学)#微分とその応用#積分とその応用#関数の変化(グラフ・最大最小・方程式・不等式)#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#九州大学#数Ⅲ
指導講師:
福田次郎
問題文全文(内容文):
以下の問いに答えよ。
(1)関数$\ y=\frac{1}{x(\log x)^2}$は$x \gt 1$において単調に減少することを示せ。
(2)不定積分$\ \int\frac{1}{x(\log x)^2}dx$ を求めよ。
(3)nを3以上の整数とするとき、不等式
$\sum_{k=3}^n\frac{1}{k(\log k)^2} \lt \frac{1}{\log 2}$
が成り立つことを示せ。
2015九州大学理系過去問
この動画を見る
以下の問いに答えよ。
(1)関数$\ y=\frac{1}{x(\log x)^2}$は$x \gt 1$において単調に減少することを示せ。
(2)不定積分$\ \int\frac{1}{x(\log x)^2}dx$ を求めよ。
(3)nを3以上の整数とするとき、不等式
$\sum_{k=3}^n\frac{1}{k(\log k)^2} \lt \frac{1}{\log 2}$
が成り立つことを示せ。
2015九州大学理系過去問
大学入試問題#373「結局いつもの唐揚げ定食」 横浜国立大学2012 #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$a \gt 0$
$\displaystyle \int x^2\cos(a\ log\ x)dx$
出典:2012年横浜国立大学 入試問題
この動画を見る
$a \gt 0$
$\displaystyle \int x^2\cos(a\ log\ x)dx$
出典:2012年横浜国立大学 入試問題
大学入試問題#368「よくみる積分」 防衛医科大学校2014 #不定積分
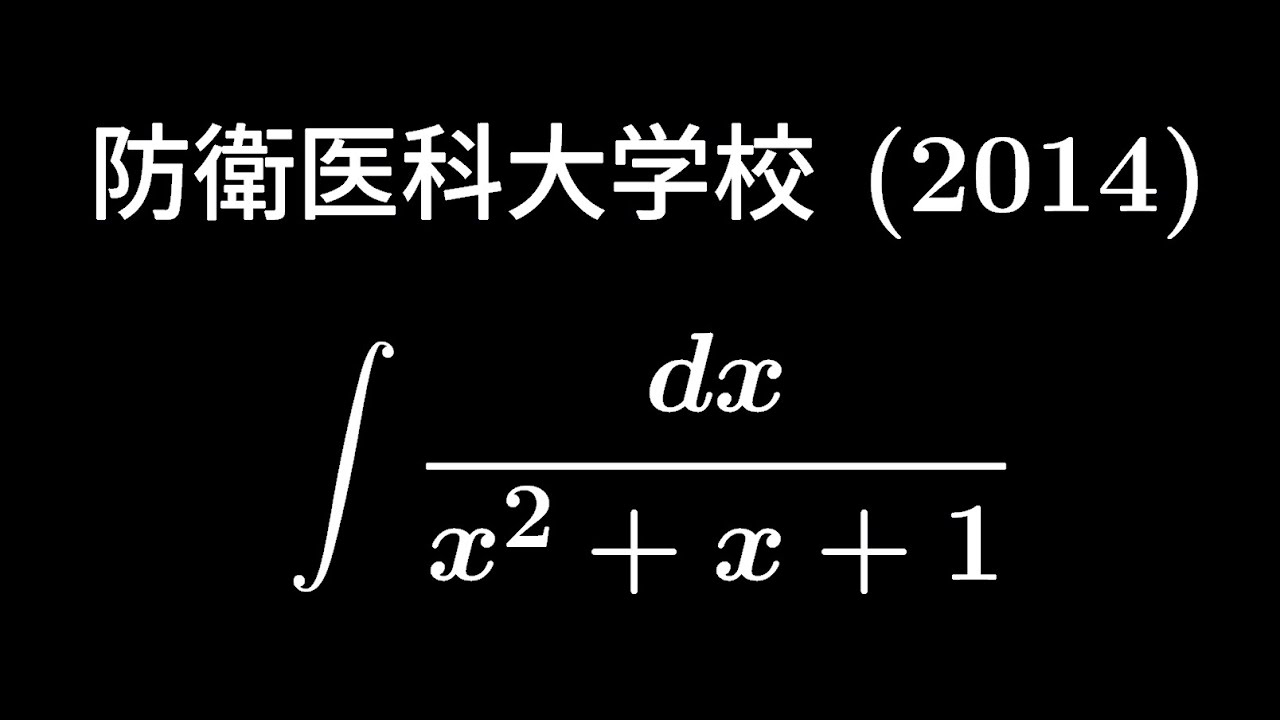
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#防衛医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{dx}{x^2+x+1}$
出典:2014年防衛医科大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{dx}{x^2+x+1}$
出典:2014年防衛医科大学 入試問題
大学入試問題#364「計算が大変でした」 岩手大学2014 #不定積分
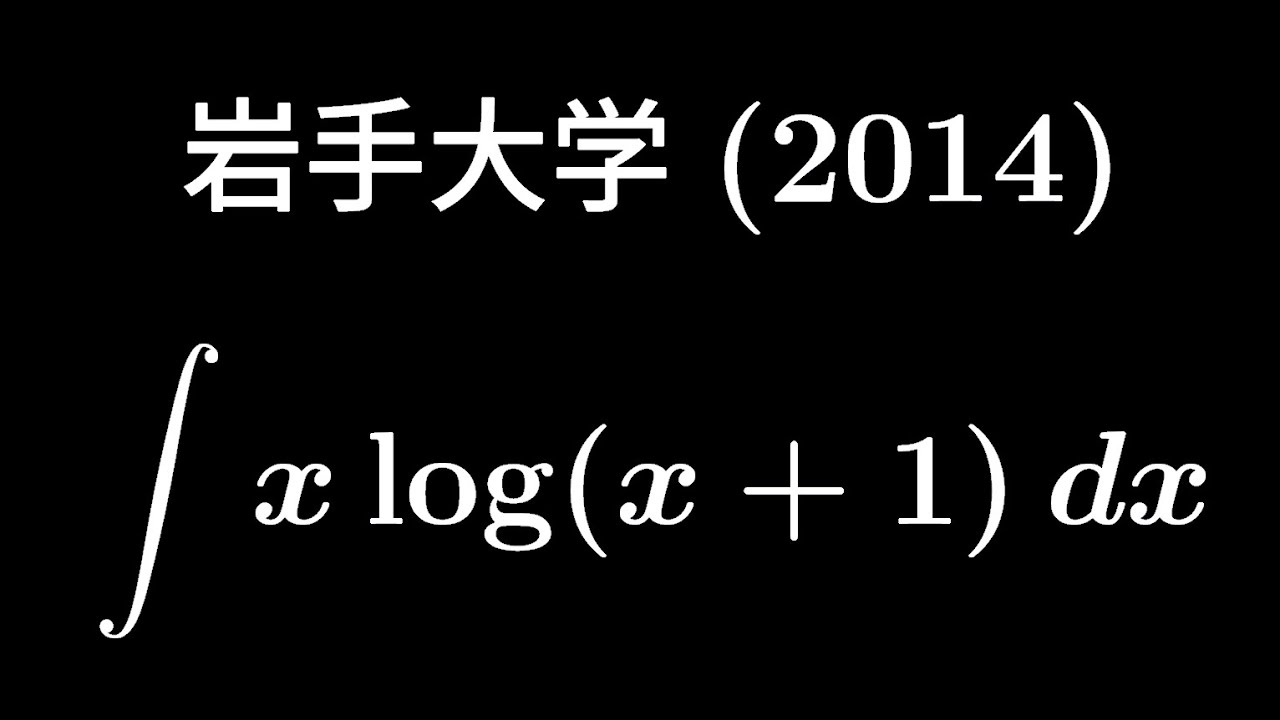
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#岩手大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int x\ log(x+1)dx$
出典:2014年岩手大学 入試問題
この動画を見る
$\displaystyle \int x\ log(x+1)dx$
出典:2014年岩手大学 入試問題
大学入試問題#352「よく出題されそうな綺麗な問題」 京都工芸繊維大学(2011) #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int (\displaystyle \frac{log\ x}{x})^2 dx$
出典:2011年京都工芸繊維大学 入試問題
この動画を見る
$\displaystyle \int (\displaystyle \frac{log\ x}{x})^2 dx$
出典:2011年京都工芸繊維大学 入試問題
大学入試問題#341「部分積分の心を・・・」 立教大学 #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#立教大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int (\sin\ x+x\ \cos\ x)log\ x\ dx$
出典:立教大学 入試問題
この動画を見る
$\displaystyle \int (\sin\ x+x\ \cos\ x)log\ x\ dx$
出典:立教大学 入試問題
【最後の足し算で計算ミスしてます。】大学入試問題#334 広島市立大学(2011) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{x^2+1}{x+1}dx$
出典:2011年広島市立大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{x^2+1}{x+1}dx$
出典:2011年広島市立大学 入試問題
大学入試問題#332 Instagram #不定積分
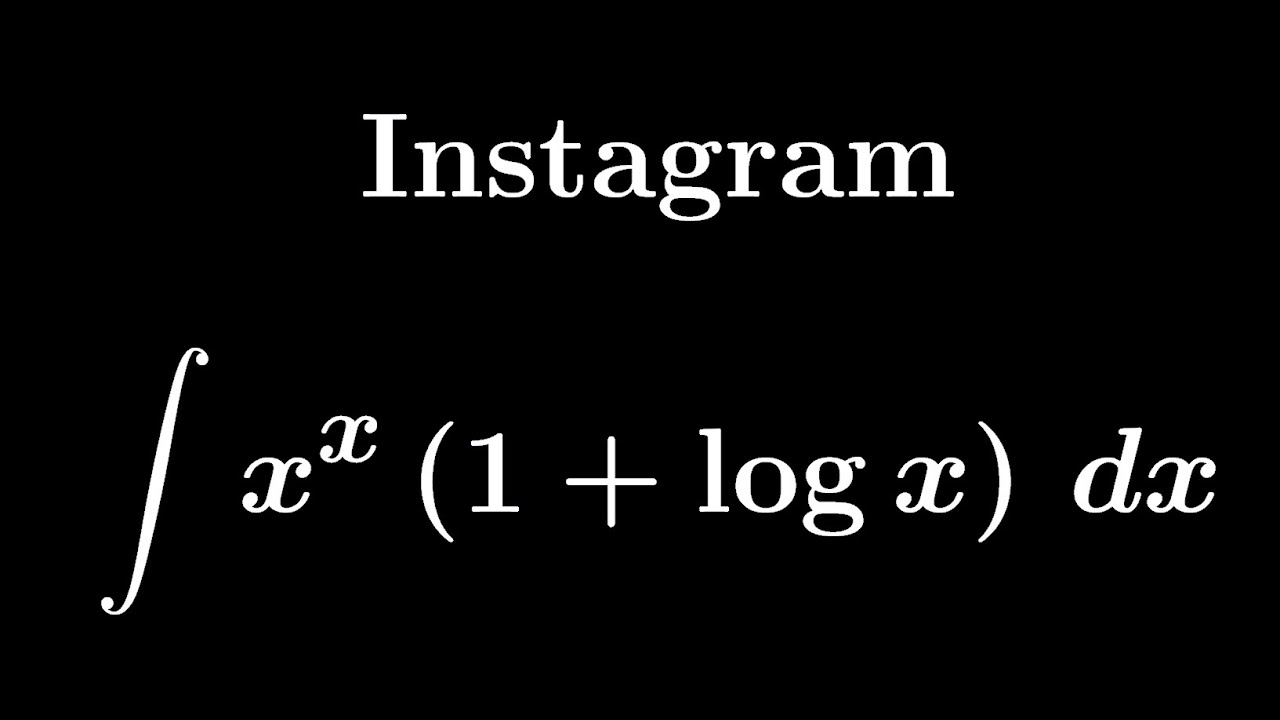
【概要欄必読】大学入試問題#326 Instagram #不定積分
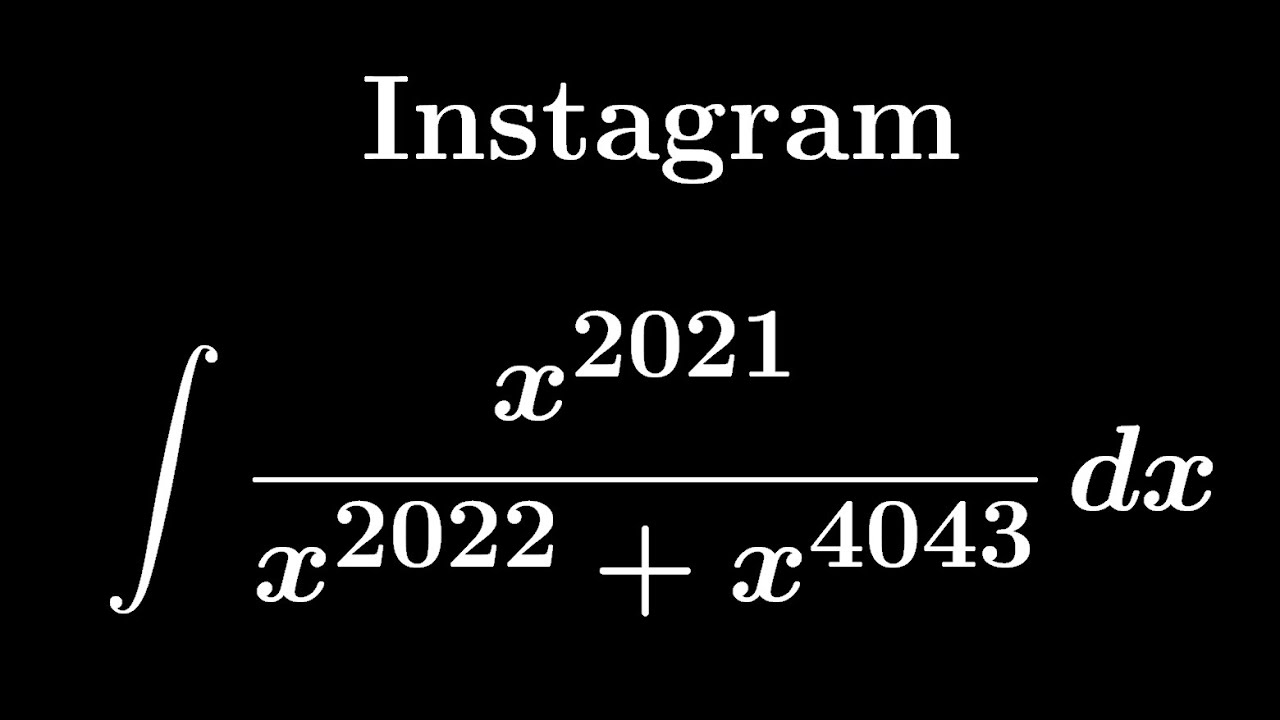
単元:
#不定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{x^{2021}}{x^{2022}+x^{4043}}dx$
この動画を見る
$\displaystyle \int \displaystyle \frac{x^{2021}}{x^{2022}+x^{4043}}dx$
大学入試問題#319 電気通信大学(2010) #定積分 #極限

単元:
#大学入試過去問(数学)#関数と極限#積分とその応用#関数の極限#不定積分#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ a \to \infty }\displaystyle \int_{0}^{a}\displaystyle \frac{1}{1+e^x}dx$
出典:2010年電気通信大学 入試問題
この動画を見る
$\displaystyle \lim_{ a \to \infty }\displaystyle \int_{0}^{a}\displaystyle \frac{1}{1+e^x}dx$
出典:2010年電気通信大学 入試問題
大学入試問題#236 茨城大学(2012) 改 #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#茨城大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{1}{x^2}(1+\displaystyle \frac{2}{x})^4dx$を計算せよ。
出典:2012年茨城大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{1}{x^2}(1+\displaystyle \frac{2}{x})^4dx$を計算せよ。
出典:2012年茨城大学 入試問題
大学入試問題#231 電気通信大学(2012) #不定積分

単元:
#大学入試過去問(数学)#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#電気通信大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \sin(log\ x)dx$を計算せよ。
出典:2012年電気通信大学 入試問題
この動画を見る
$\displaystyle \int \sin(log\ x)dx$を計算せよ。
出典:2012年電気通信大学 入試問題
大学入試問題#226 信州大学(2012) #不定積分
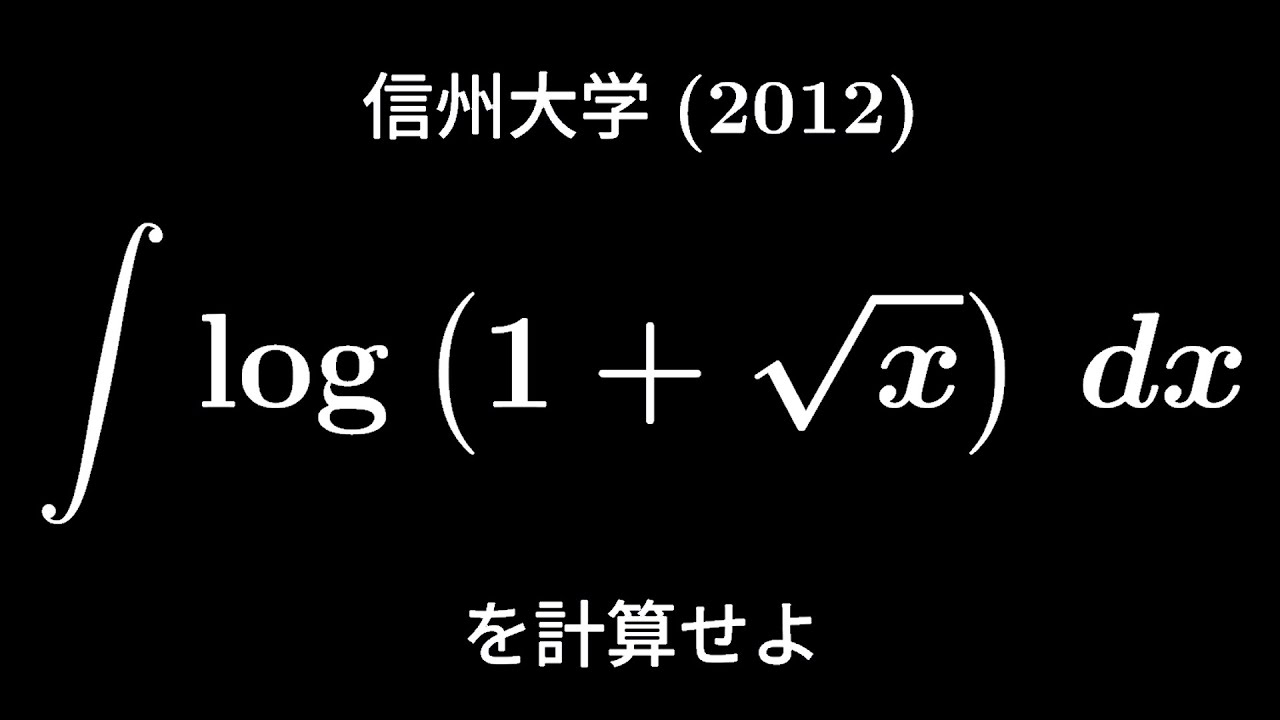
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int log(1+\sqrt{ x })dx$を計算せよ
出典:2012年信州大学 入試問題
この動画を見る
$\displaystyle \int log(1+\sqrt{ x })dx$を計算せよ
出典:2012年信州大学 入試問題
大学入試問題#224 防衛医科大学(2015) #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#防衛医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{dx}{x^2+x+1}$を計算せよ
出典:2015年防衛医科大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{dx}{x^2+x+1}$を計算せよ
出典:2015年防衛医科大学 入試問題
大学入試問題#222 広島市立大学2015 #不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{\cos\ x}{\cos^2x+2\sin\ x-2}dx$を計算せよ
出典:2015年広島市立大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{\cos\ x}{\cos^2x+2\sin\ x-2}dx$を計算せよ
出典:2015年広島市立大学 入試問題
大学入試問題#210 宮崎大学(2018) 不定積分
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#宮崎大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
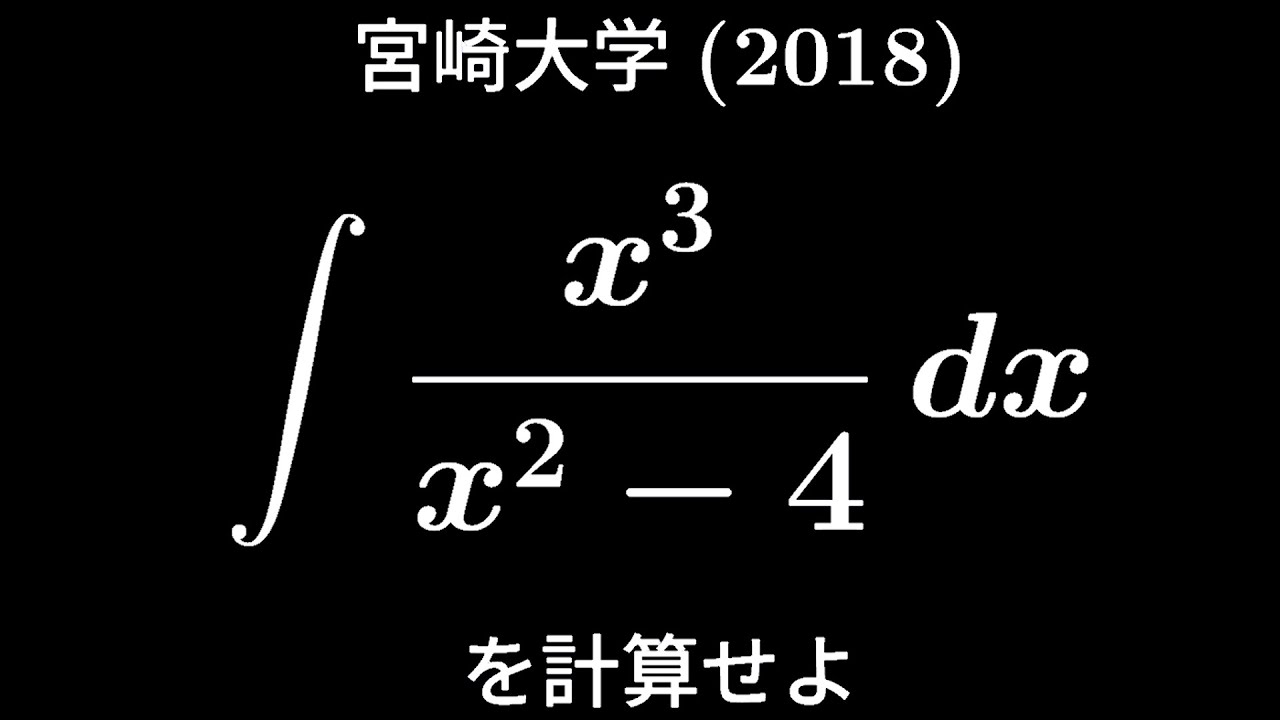
$\displaystyle \int \displaystyle \frac{x^3}{x^2-4}\ dx$を計算せよ。
出典:2018年宮崎大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{x^3}{x^2-4}\ dx$を計算せよ。
出典:2018年宮崎大学 入試問題
大学入試問題#207 埼玉大学(2006) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#埼玉大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int -4\tan\ x\ log(\cos^2x)dx$を計算せよ。
出典:2006年埼玉大学 入試問題
この動画を見る
$\displaystyle \int -4\tan\ x\ log(\cos^2x)dx$を計算せよ。
出典:2006年埼玉大学 入試問題
大学入試問題#206 東京農工大学(2020) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京農工大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{1}{e^x+2}\ dx$
出典:2020年東京農業工業大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{1}{e^x+2}\ dx$
出典:2020年東京農業工業大学 入試問題
大学入試問題#194 横浜国立大学 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \sqrt{ 1-e^{-2x} }\ dx$を計算せよ。
出典:横浜国立大学 入試問題
この動画を見る
$\displaystyle \int \sqrt{ 1-e^{-2x} }\ dx$を計算せよ。
出典:横浜国立大学 入試問題
大学入試問題#192 東京大学(昭和9年) 不定積分 3手1組の好手順
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
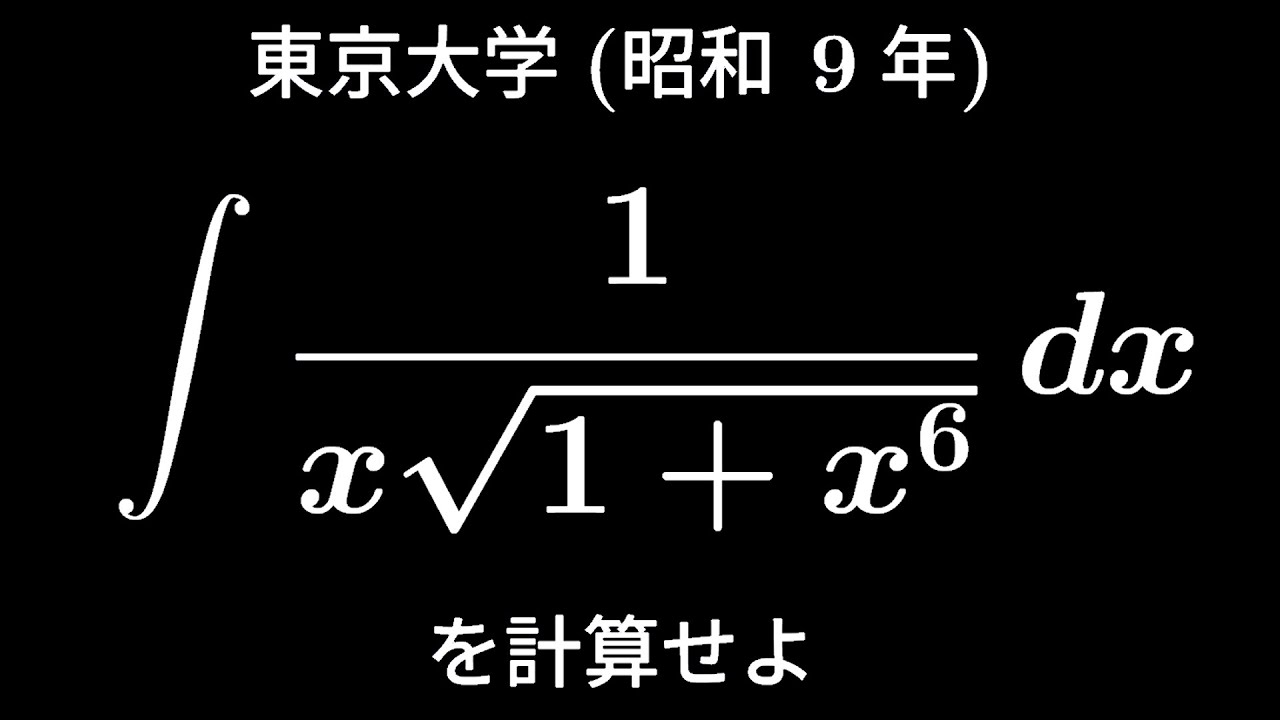
$\displaystyle \int \displaystyle \frac{1}{x\sqrt{ 1+x^6 }}\ dx$を計算せよ。
出典:昭和9年東京大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{1}{x\sqrt{ 1+x^6 }}\ dx$を計算せよ。
出典:昭和9年東京大学 入試問題
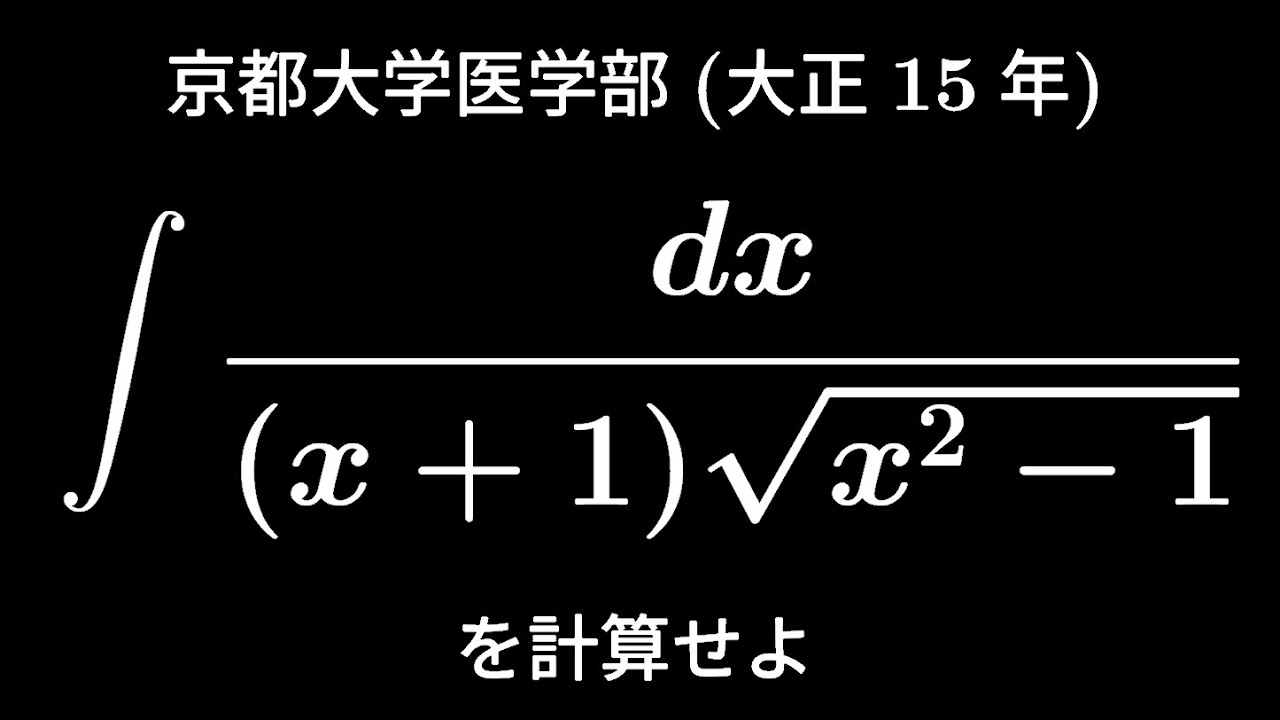
大学入試問題#186 京都大学医学部(大正15年) 不定積分 たぶん難問
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{dx}{(x+1)\sqrt{ x^2-1 }}$を計算せよ。
出典:大正15年京都大学医学部 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{dx}{(x+1)\sqrt{ x^2-1 }}$を計算せよ。
出典:大正15年京都大学医学部 入試問題
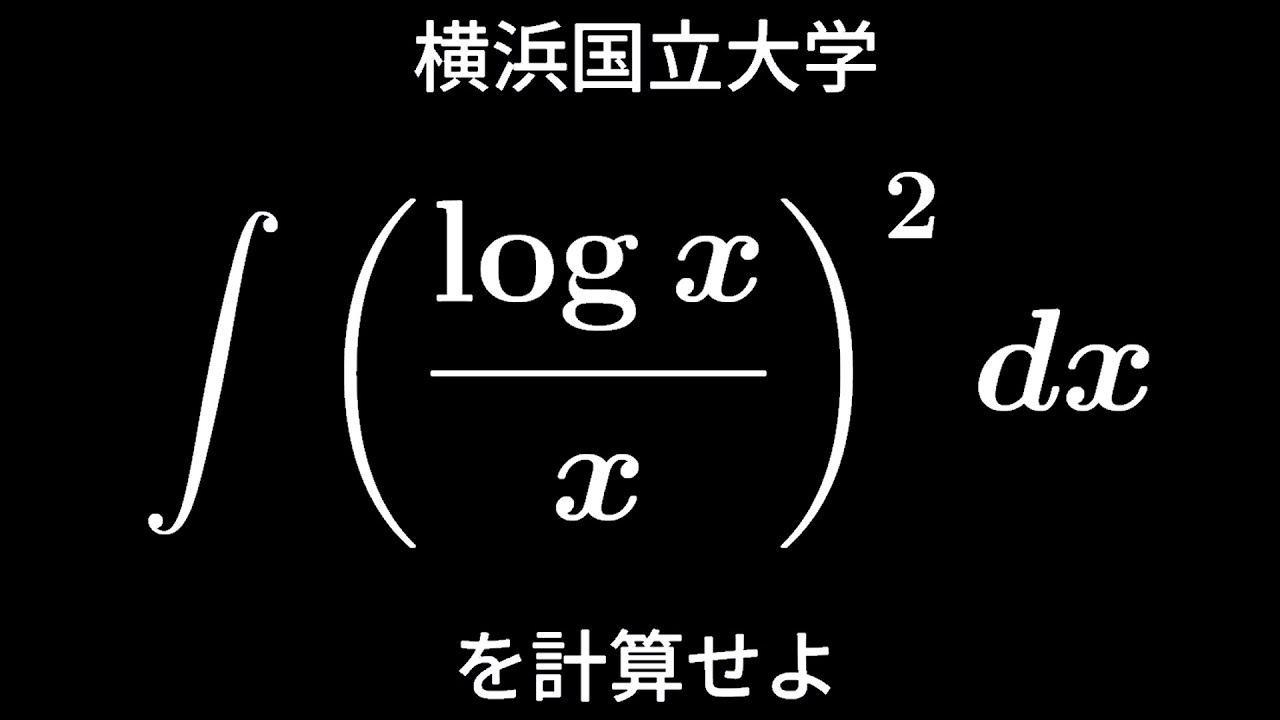
大学入試問題#182 横浜国立大学 不定積分
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int (\displaystyle \frac{log\ x}{x})^2dx$を計算せよ
出典:横浜国立大学 入試問題
この動画を見る
$\displaystyle \int (\displaystyle \frac{log\ x}{x})^2dx$を計算せよ
出典:横浜国立大学 入試問題
大学入試問題#176 日本医科大学(2019) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#日本医科大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \sqrt{ x^2+1 }\ dx$
出典:2019年日本医科大学 入試問題
この動画を見る
$\displaystyle \int \sqrt{ x^2+1 }\ dx$
出典:2019年日本医科大学 入試問題
