 微分法と積分法
微分法と積分法
 微分法と積分法
微分法と積分法
#高専_7#定積分#元高専教員
単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
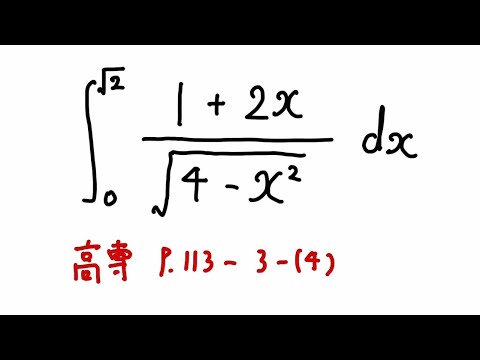
問題文全文(内容文):
以下の定積分を解け。
$\displaystyle \int_{0}^{\sqrt{ 2 }} \displaystyle \frac{1+2x}{\sqrt{ 4-x^2 }} dx$
この動画を見る
以下の定積分を解け。
$\displaystyle \int_{0}^{\sqrt{ 2 }} \displaystyle \frac{1+2x}{\sqrt{ 4-x^2 }} dx$
#千葉大学2018#不定積分#数学者
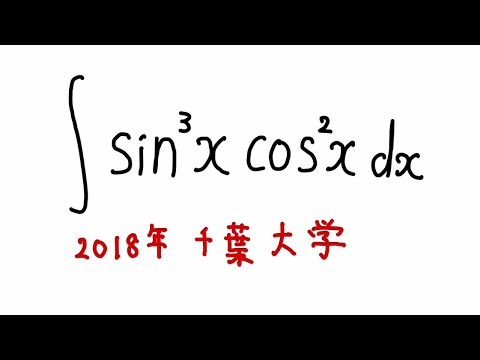
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
以下の不定積分を解け。
$\displaystyle \int \sin^3x \cos^2x$ $dx$
出典:2018年千葉大学
この動画を見る
以下の不定積分を解け。
$\displaystyle \int \sin^3x \cos^2x$ $dx$
出典:2018年千葉大学
#高専_6#不定積分#元高専教員

単元:
#数Ⅱ#微分法と積分法#積分とその応用#不定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
以下の不定積分を解け。
$\displaystyle \int (3x+1)\cos2x$ $dx$
この動画を見る
以下の不定積分を解け。
$\displaystyle \int (3x+1)\cos2x$ $dx$
#千葉大学2022#極限#ますただ

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#千葉大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ n \to \infty } (\sqrt{ n^2+n }-n)$
出典:2022年千葉大学
この動画を見る
$\displaystyle \lim_{ n \to \infty } (\sqrt{ n^2+n }-n)$
出典:2022年千葉大学
大学入試問題#898「教科書例題」 #千葉大学(2024)

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
次の等式を満たす$x \gt 0$で定義された関数$f(x)$と定数$a$の値を求めよ。
ただし、$a \gt 0$とする。
$\displaystyle \int_{a}^{x} f(t) dt=x+\displaystyle \frac{1}{2}log$ $x-1$
出典:2024年千葉大学
この動画を見る
次の等式を満たす$x \gt 0$で定義された関数$f(x)$と定数$a$の値を求めよ。
ただし、$a \gt 0$とする。
$\displaystyle \int_{a}^{x} f(t) dt=x+\displaystyle \frac{1}{2}log$ $x-1$
出典:2024年千葉大学
#高専数学_5#不定積分#元高専教員

単元:
#数Ⅱ#微分法と積分法#積分とその応用#不定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
下記の不定積分を解け。
$\displaystyle \int x log (x+1)$ $dx$
この動画を見る
下記の不定積分を解け。
$\displaystyle \int x log (x+1)$ $dx$
#千葉大学2020#不定積分#ますただ
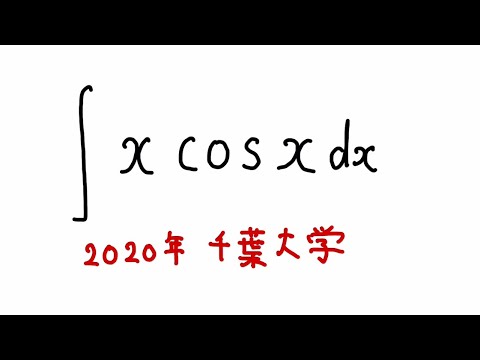
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int x\cos x$ $dx$
出典:2024年千葉大学
この動画を見る
$\displaystyle \int x\cos x$ $dx$
出典:2024年千葉大学
#高専_4#不定積分#元高専教員

単元:
#数Ⅱ#微分法と積分法#積分とその応用#不定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
以下の不定積分を解け。
$\displaystyle \int \displaystyle \frac{(log t)^2}{t} dt$
この動画を見る
以下の不定積分を解け。
$\displaystyle \int \displaystyle \frac{(log t)^2}{t} dt$
#高専_3#定積分#元高専教員

単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-1}^{1} (e^x-e^{-x})^2(e^x+e^{-x}) dx$
この動画を見る
$\displaystyle \int_{-1}^{1} (e^x-e^{-x})^2(e^x+e^{-x}) dx$
大学入試問題#896「難関大学ではたまにでる?」 #北海道大学(2024)

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#数学(高校生)#北海道大学
指導講師:
ますただ
問題文全文(内容文):
関数$f_1(x),f_2(x),f_3(x),…$を次の関係式で定める。
$f_1(x)=3x$
$f_{n+1}(x)=(n+2)x^{n+1}+(\displaystyle \int_{0}^{1} f_n(t) dt)x$
関数$f_n(x)$を$x$と$n$の式で表せ。$(n=1,2,3,…)$
出典:2024年北海道大学
この動画を見る
関数$f_1(x),f_2(x),f_3(x),…$を次の関係式で定める。
$f_1(x)=3x$
$f_{n+1}(x)=(n+2)x^{n+1}+(\displaystyle \int_{0}^{1} f_n(t) dt)x$
関数$f_n(x)$を$x$と$n$の式で表せ。$(n=1,2,3,…)$
出典:2024年北海道大学
#高専_2#定積分

単元:
#数Ⅱ#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int (1-\sin^3x)\cos x$ $dx$
この動画を見る
$\displaystyle \int (1-\sin^3x)\cos x$ $dx$
微分法と積分法 数Ⅱ定積分:1/6公式の使い方【烈's study!がていねいに解説】

単元:
#数Ⅱ#微分法と積分法#不定積分・定積分#数学(高校生)
教材:
#TK数学#TK数学問題集2(幾何編)#中高教材
指導講師:
理数個別チャンネル
問題文全文(内容文):
$\displaystyle \int_{α}^{ β } (x-α)(x-β)dx=-\dfrac{1}{6}(β-α)^3$を用いて、次の定積分を求めよ。
(1)$\displaystyle \int_{-1}^{ 2 } (x^2-x-2)dx$
(2)$\displaystyle \int_{1-\sqrt{2} }^{1+\sqrt{2}} (x^2-2x-1)dx$
(3)$\displaystyle \int_{3}^{ 4 } (14x-24-2x^2)dx$
この動画を見る
$\displaystyle \int_{α}^{ β } (x-α)(x-β)dx=-\dfrac{1}{6}(β-α)^3$を用いて、次の定積分を求めよ。
(1)$\displaystyle \int_{-1}^{ 2 } (x^2-x-2)dx$
(2)$\displaystyle \int_{1-\sqrt{2} }^{1+\sqrt{2}} (x^2-2x-1)dx$
(3)$\displaystyle \int_{3}^{ 4 } (14x-24-2x^2)dx$
#高専#不定積分-1
単元:
#数Ⅱ#微分法と積分法#積分とその応用#不定積分#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
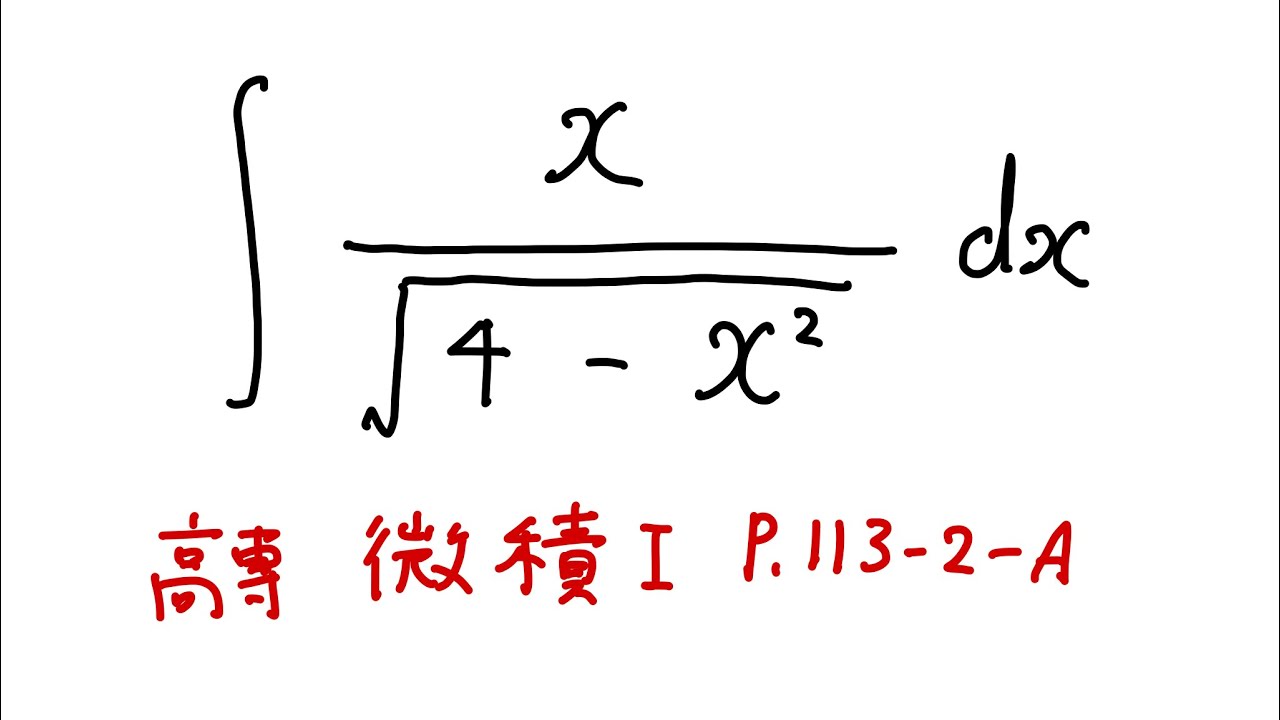
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 4-x^2 }} dx$
この動画を見る
$\displaystyle \int \displaystyle \frac{x}{\sqrt{ 4-x^2 }} dx$
#千葉大学2024#定積分
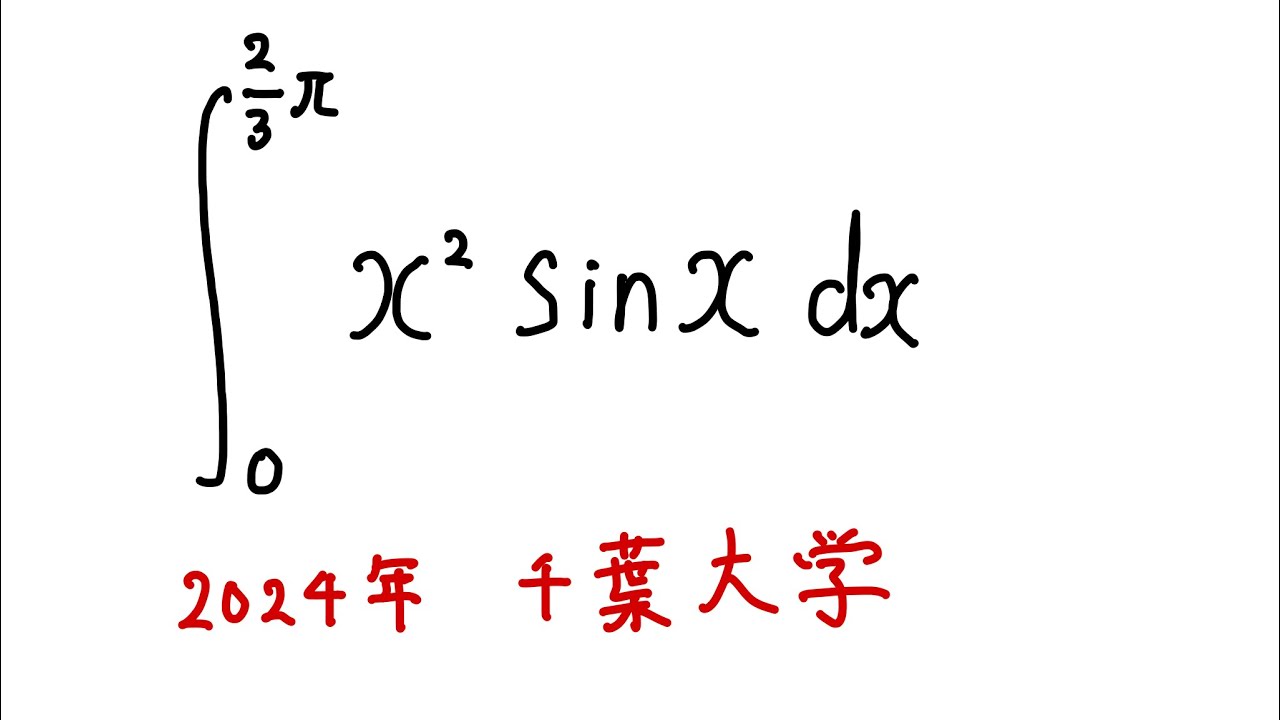
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
以下の定積分を解け。
$\displaystyle \int_{0}^{\frac{2}{3}\pi} x^2\sin x$ $dx$
出典:2024年千葉大学
この動画を見る
以下の定積分を解け。
$\displaystyle \int_{0}^{\frac{2}{3}\pi} x^2\sin x$ $dx$
出典:2024年千葉大学
#千葉大学2023#定積分#ますただ
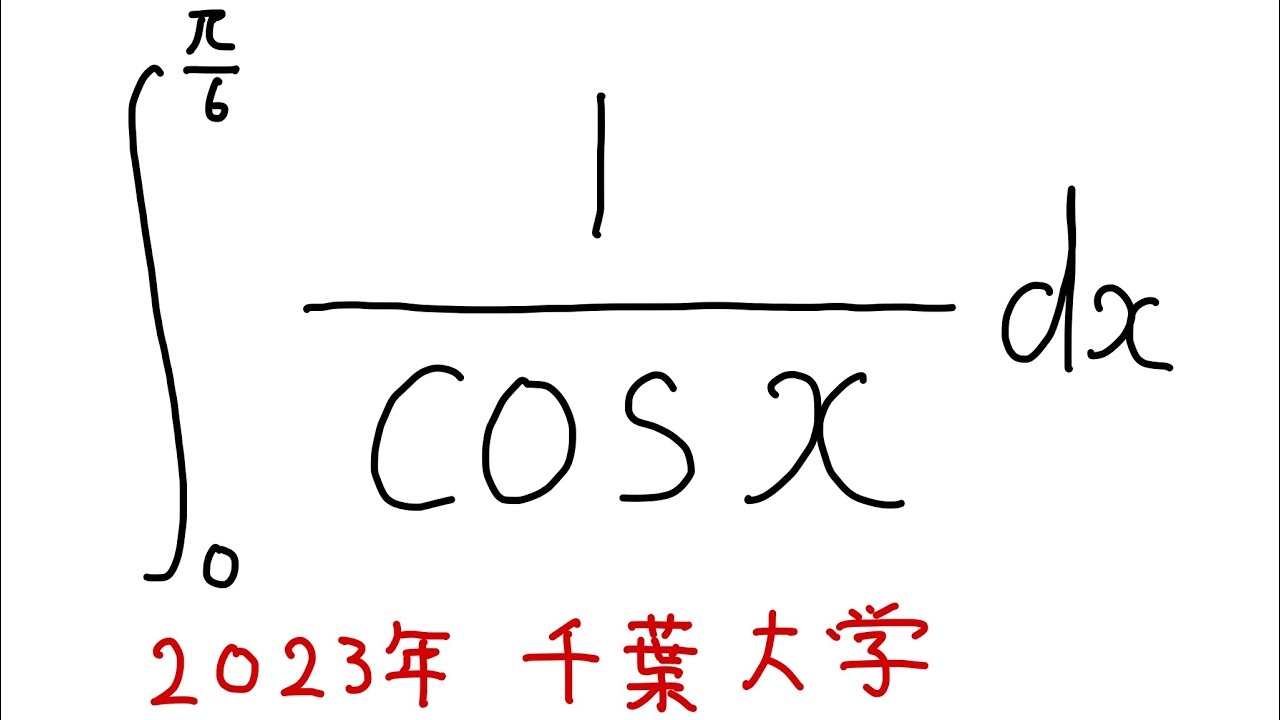
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
以下の定積分を解け。
$\displaystyle \int_{0}^{\frac{\pi}{6}} \displaystyle \frac{1}{\cos x} dx$
出典:2023年千葉大学
この動画を見る
以下の定積分を解け。
$\displaystyle \int_{0}^{\frac{\pi}{6}} \displaystyle \frac{1}{\cos x} dx$
出典:2023年千葉大学
#千葉大学2023#定積分
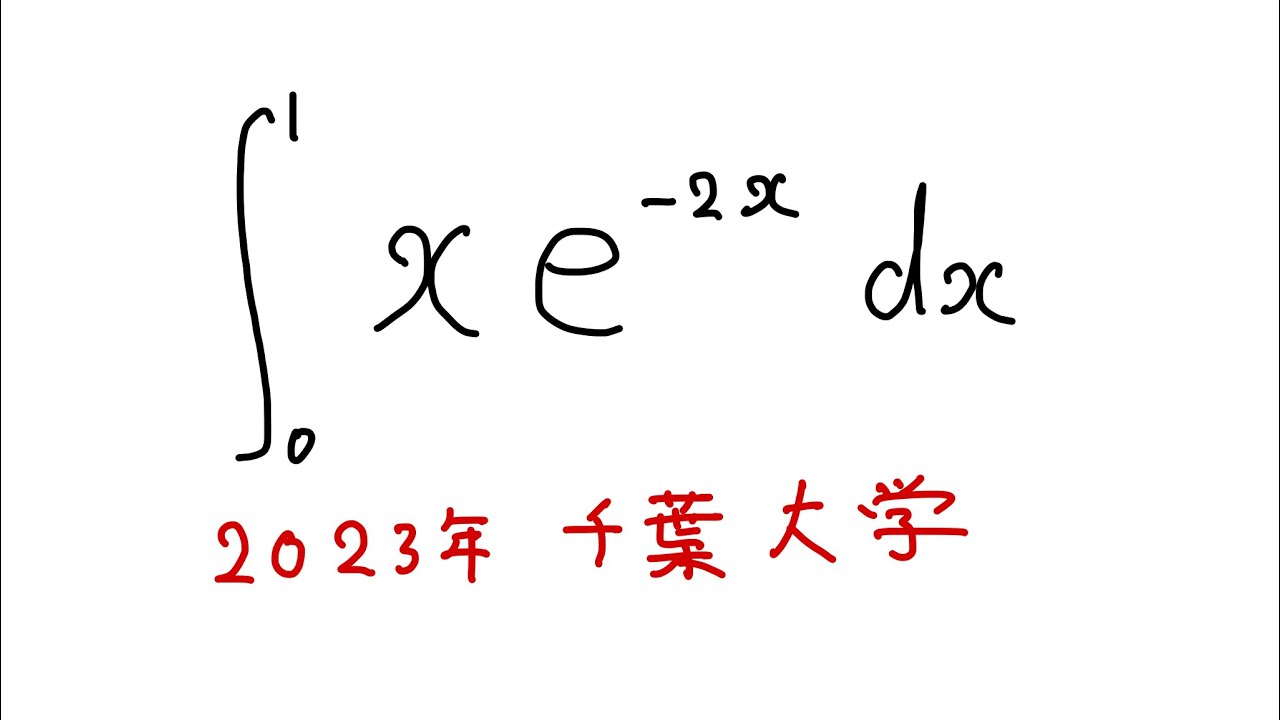
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
下記の定積分を解け
$\displaystyle \int_{0}^{1} xe^{-2x} dx$
出典:2023年千葉大学
この動画を見る
下記の定積分を解け
$\displaystyle \int_{0}^{1} xe^{-2x} dx$
出典:2023年千葉大学
#広島市立大学#不定積分#ますただ
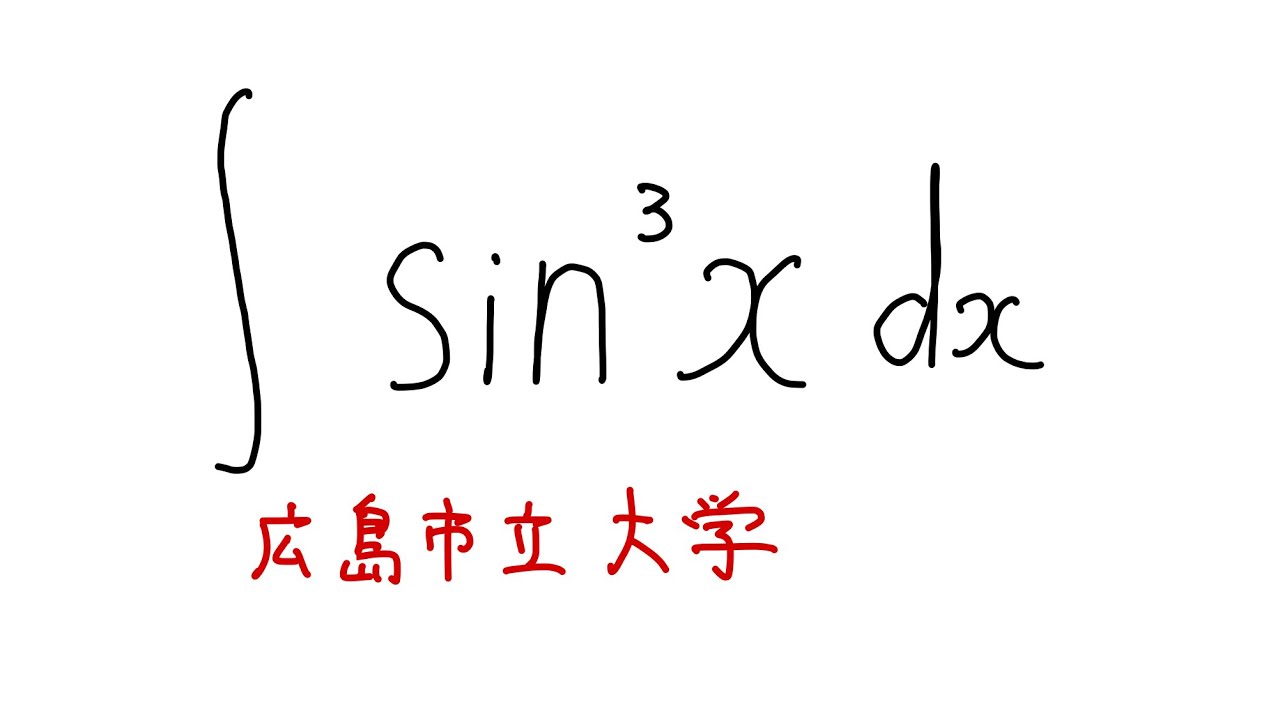
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \sin^3 x$ $dx$
広島市立大過去問
この動画を見る
$\displaystyle \int \sin^3 x$ $dx$
広島市立大過去問
大学入試問題#893「難易度クソ高め」 #信州大学(2015)
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学
指導講師:
ますただ
問題文全文(内容文):
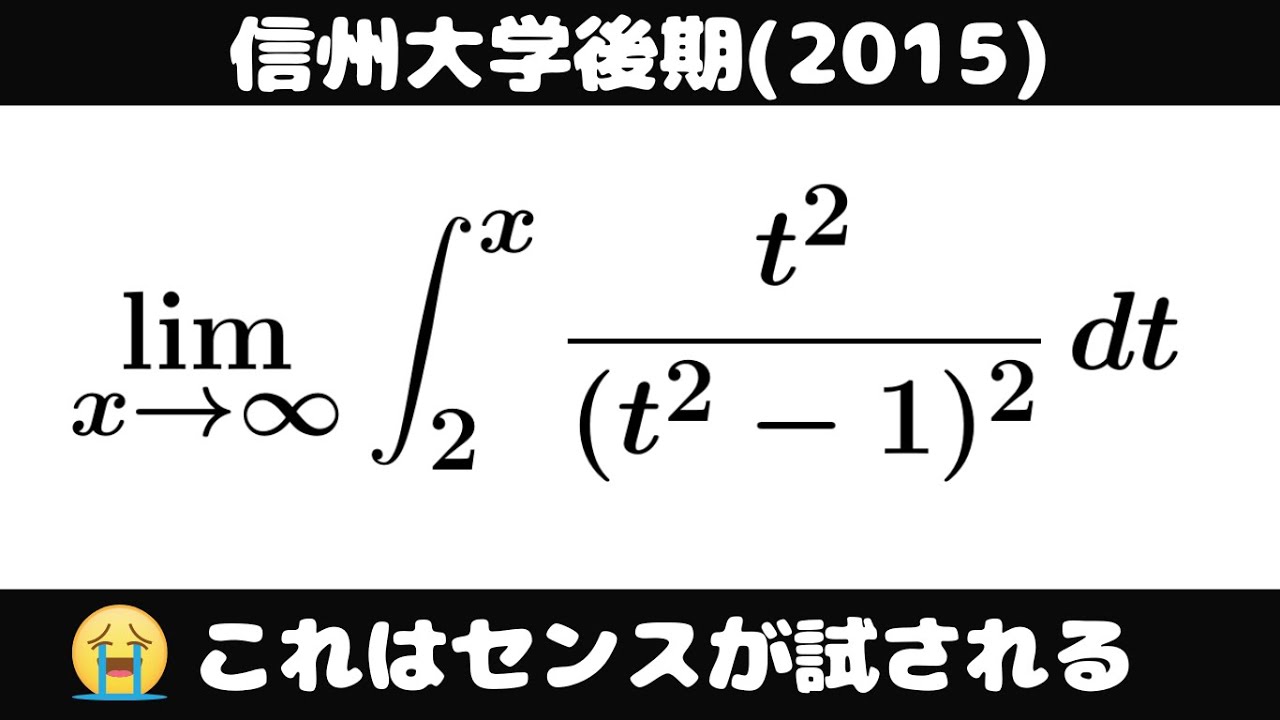
$\displaystyle \lim_{ n \to \infty } \displaystyle \int_{2}^{x} \displaystyle \frac{t^2}{(t^2-1)^2}dt$
出典:2015年信州大学後期
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \int_{2}^{x} \displaystyle \frac{t^2}{(t^2-1)^2}dt$
出典:2015年信州大学後期
#電気通信大学2015#区分求積法#ますただ
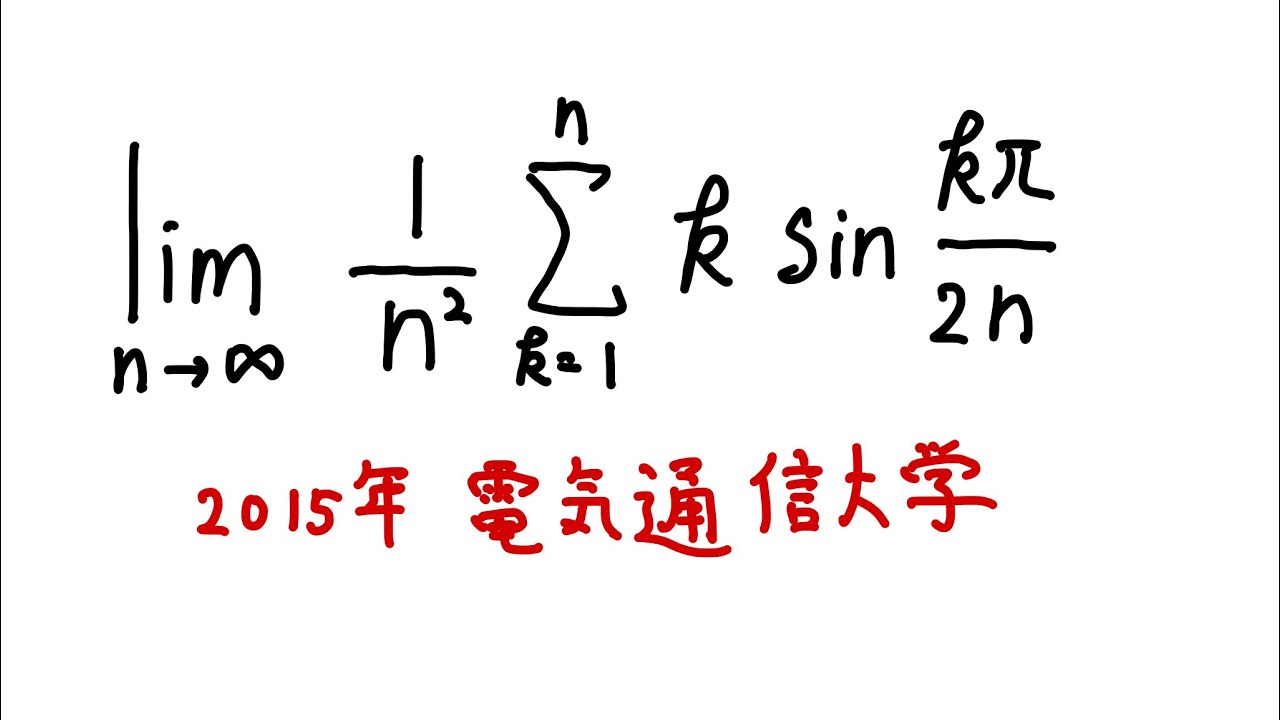
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \lim_{ n \to \infty } \displaystyle \frac{1}{n^2}\displaystyle \sum_{k=1}^n k \sin\displaystyle \frac{k\pi}{2n}$
出典:2015年電気通信大学
この動画を見る
$\displaystyle \lim_{ n \to \infty } \displaystyle \frac{1}{n^2}\displaystyle \sum_{k=1}^n k \sin\displaystyle \frac{k\pi}{2n}$
出典:2015年電気通信大学
#富山大学推薦2019#定積分
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#富山大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
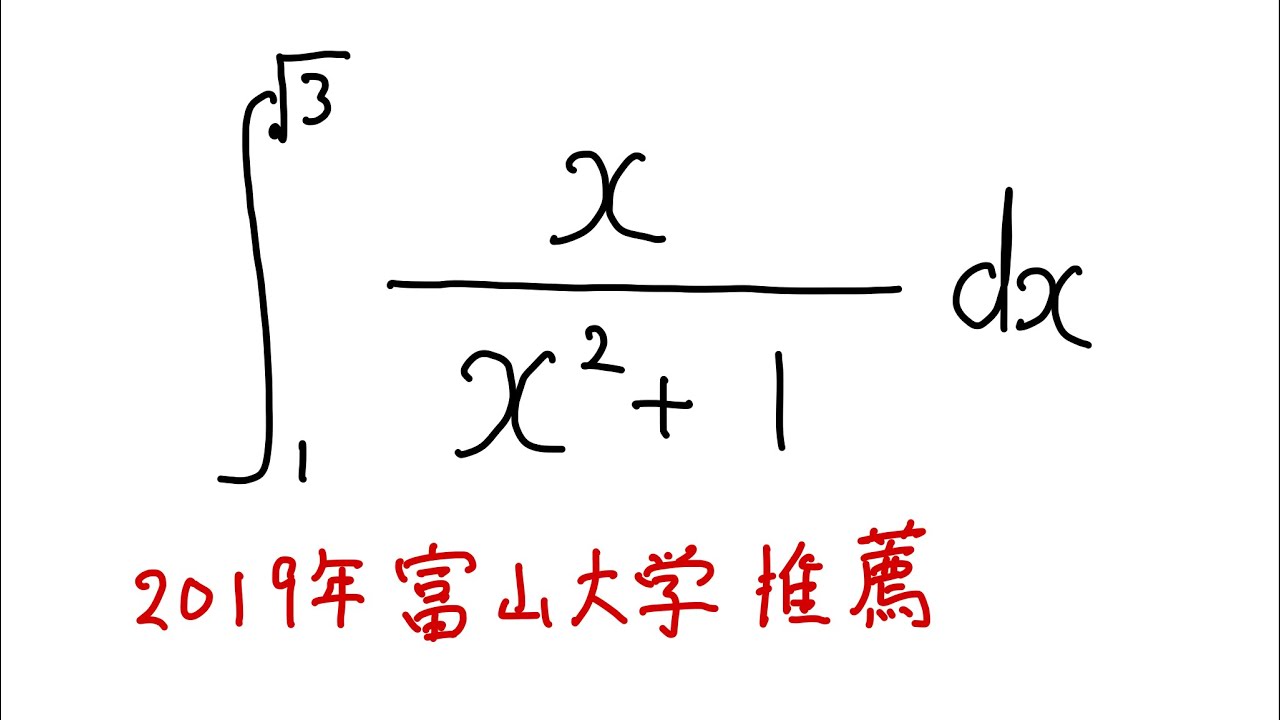
$\displaystyle \int_{1}^{\sqrt{ 3 }} \displaystyle \frac{x}{x^2+1} dx$
出典:2019年富山大学推薦
この動画を見る
$\displaystyle \int_{1}^{\sqrt{ 3 }} \displaystyle \frac{x}{x^2+1} dx$
出典:2019年富山大学推薦
大学入試問題#892「数学はやっぱ根性」 #京都工芸繊維大学(2023)

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
$0 \leq \theta \leq \displaystyle \frac{\pi}{4}$とする
$f(\theta)=\displaystyle \int_{0}^{\frac{\pi}{4}} \displaystyle \frac{|\sin\theta-\sin x|}{\cos^2x} dx$
出典:2023年京都工芸繊維大学
この動画を見る
$0 \leq \theta \leq \displaystyle \frac{\pi}{4}$とする
$f(\theta)=\displaystyle \int_{0}^{\frac{\pi}{4}} \displaystyle \frac{|\sin\theta-\sin x|}{\cos^2x} dx$
出典:2023年京都工芸繊維大学
#広島市立大学2014#不定積分#ますただ
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
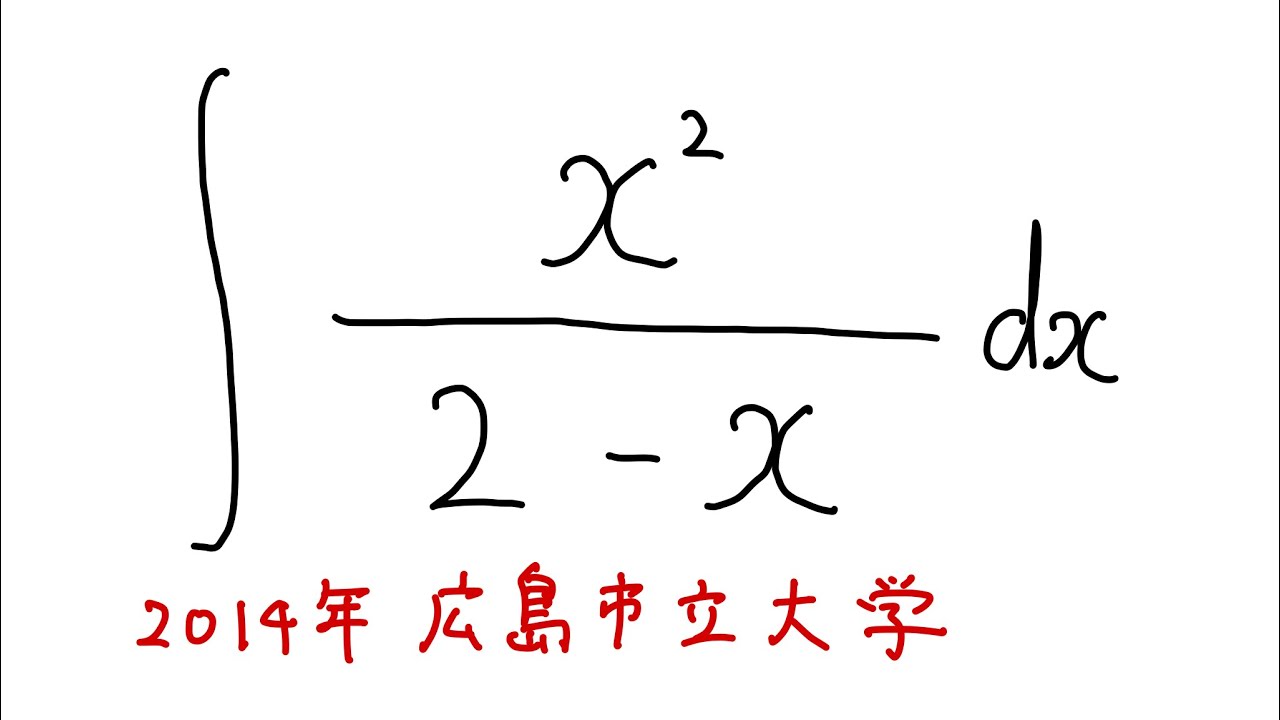
$\displaystyle \int \displaystyle \frac{x^2}{2-x} dx$
出典:2014年広島市立大学
この動画を見る
$\displaystyle \int \displaystyle \frac{x^2}{2-x} dx$
出典:2014年広島市立大学
#電気通信大学2015#定積分#ますただ
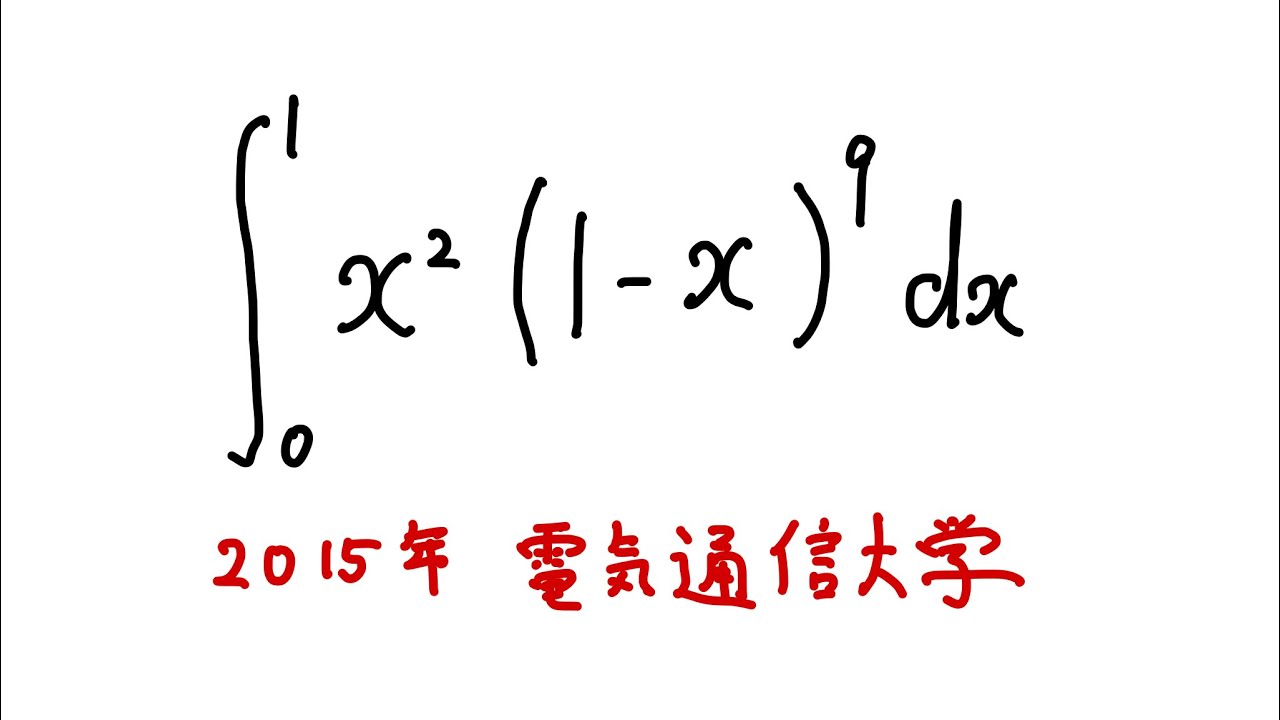
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1} x^2(1-x)^9 dx$
出典:2015年電気通信大学
この動画を見る
$\displaystyle \int_{0}^{1} x^2(1-x)^9 dx$
出典:2015年電気通信大学
大学入試問題#891「まだこのタイプの問題残ってた」 #信州大学(2023) #キングプロパティ

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#信州大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-\pi}^{ \pi } \displaystyle \frac{1}{1+e^{-2\sin x}} dx$
出典:2023年信州大学
この動画を見る
$\displaystyle \int_{-\pi}^{ \pi } \displaystyle \frac{1}{1+e^{-2\sin x}} dx$
出典:2023年信州大学
#富山大学薬学部2018#不定積分
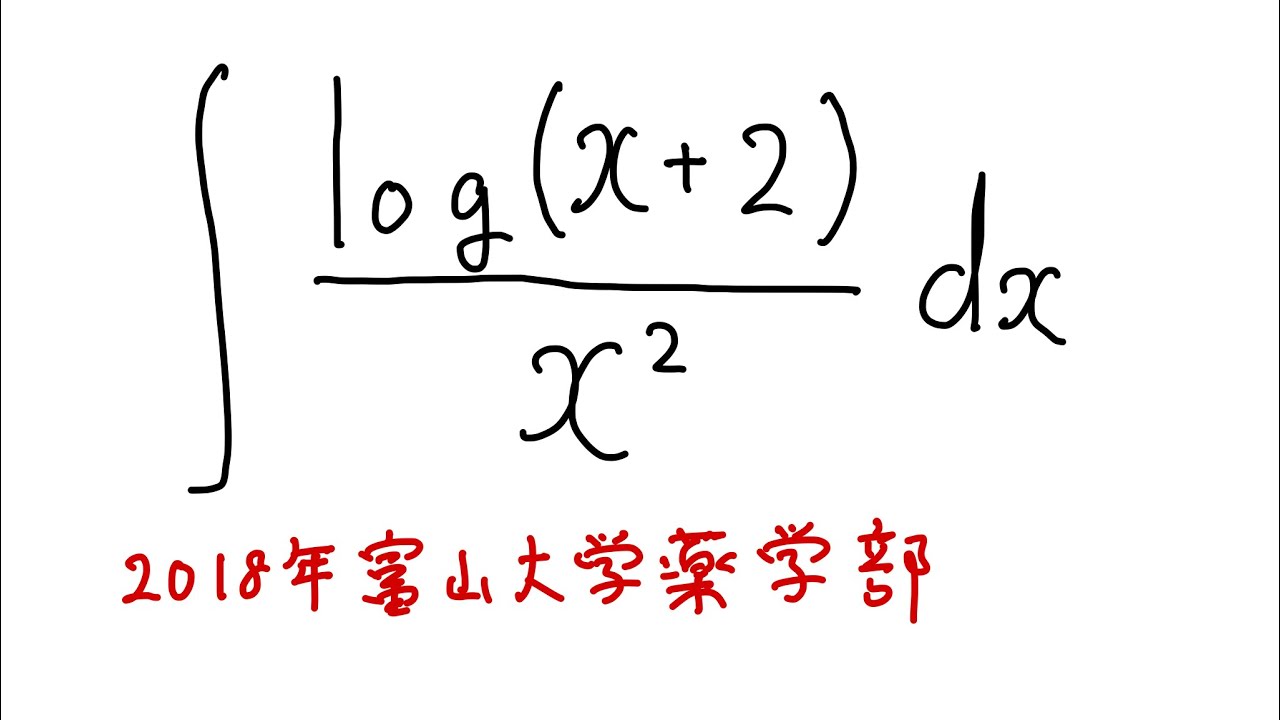
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#富山大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{log(x+2)}{x^2} dx$
出典:2018年富山大学薬学部
この動画を見る
$\displaystyle \int \displaystyle \frac{log(x+2)}{x^2} dx$
出典:2018年富山大学薬学部
#数検準1級1次_4#不定積分
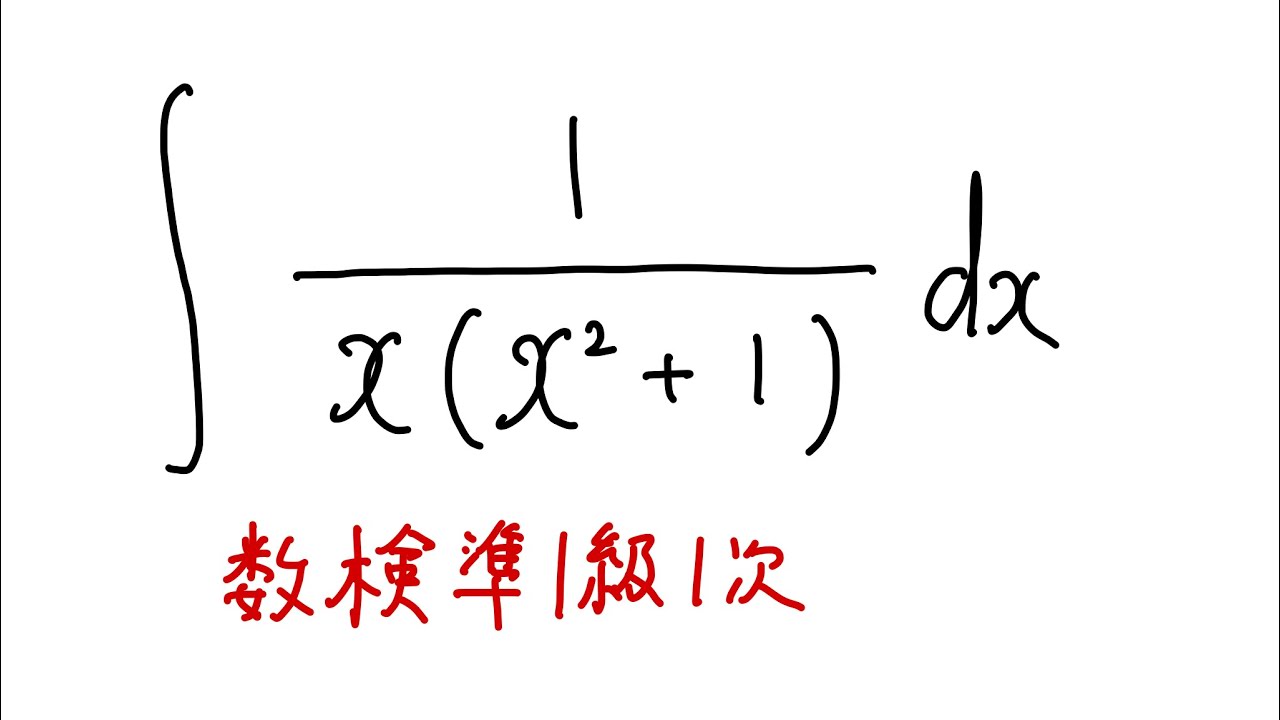
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#不定積分#不定積分・定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{1}{x(x^2+1)} dx$
出典:数検準1級1次
この動画を見る
$\displaystyle \int \displaystyle \frac{1}{x(x^2+1)} dx$
出典:数検準1級1次
大学入試問題#890「苦手な受験生多そう」 #富山大学(2019)

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#平均変化率・極限・導関数#学校別大学入試過去問解説(数学)#数学(高校生)#富山大学
指導講師:
ますただ
問題文全文(内容文):
$f(x)=x\sqrt{ x+1 }$を導関数の定義に従って微分せよ。
出典:2019年富山大学推薦
この動画を見る
$f(x)=x\sqrt{ x+1 }$を導関数の定義に従って微分せよ。
出典:2019年富山大学推薦
#藤田医科大学2023#定積分
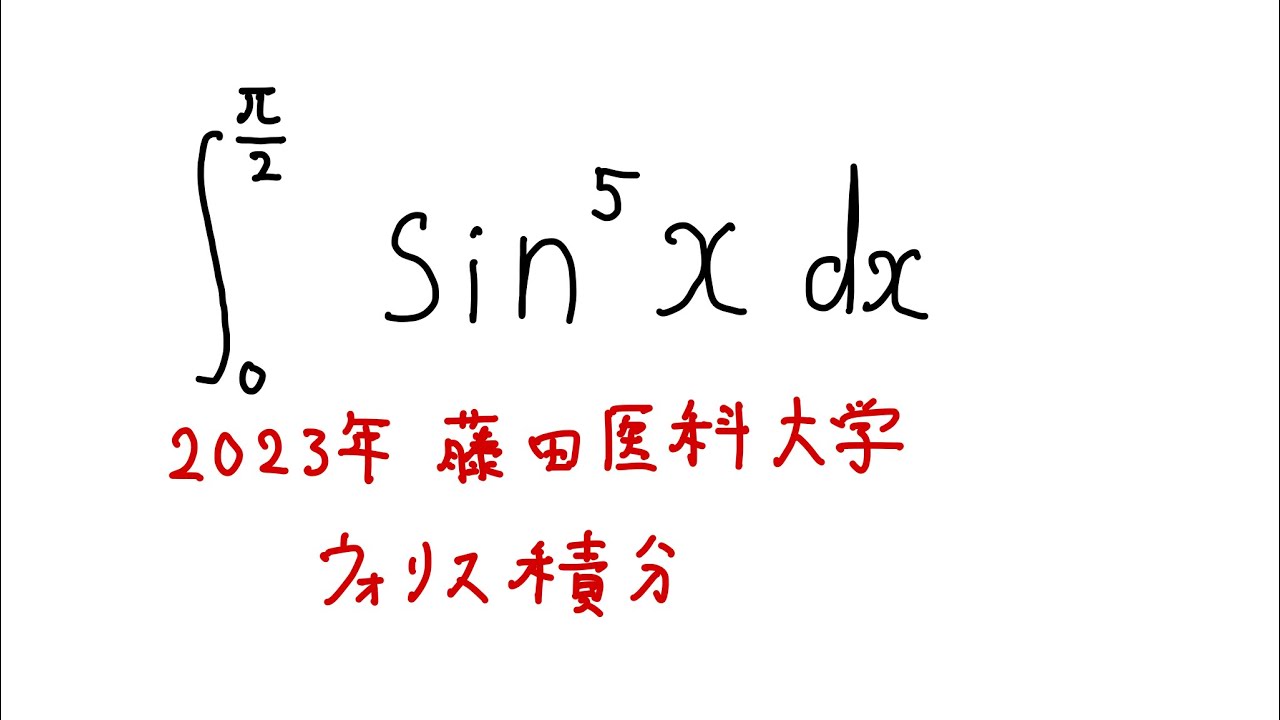
単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#積分とその応用#定積分#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#藤田医科大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sin^5x$ $dx$
出典:2023年藤田医科大学
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}} \sin^5x$ $dx$
出典:2023年藤田医科大学
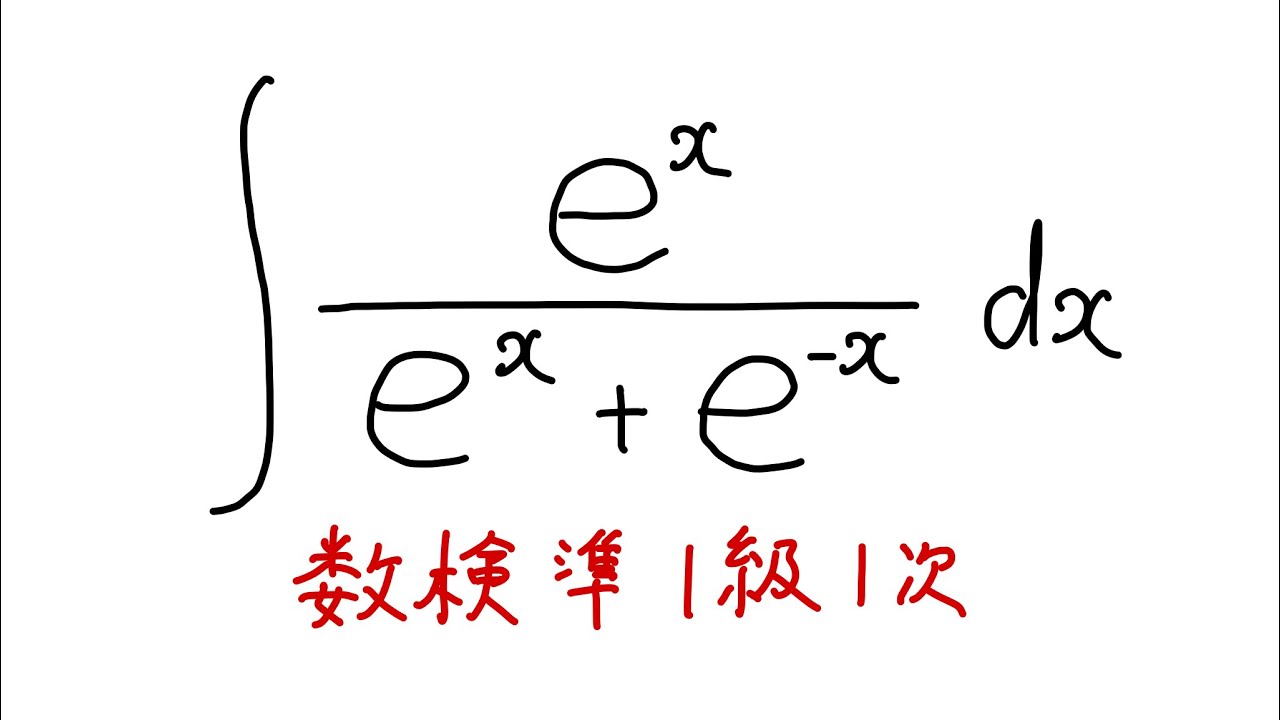
#数検準1級1次_2 #不定積分
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#不定積分#不定積分・定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int \displaystyle \frac{e^x}{e^x+e^{-x}} dx$
出典:数検準1級1次
この動画を見る
$\displaystyle \int \displaystyle \frac{e^x}{e^x+e^{-x}} dx$
出典:数検準1級1次
大学入試問題#889「丁寧に計算するのみ」 #富山大学(2019)

単元:
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#富山大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{2\pi} (\cos^2x+x^2\sin^2x) dx$
出典:2019年富山大学推薦
この動画を見る
$\displaystyle \int_{0}^{2\pi} (\cos^2x+x^2\sin^2x) dx$
出典:2019年富山大学推薦
