 定積分
定積分
 定積分
定積分
大学入試問題#119 横浜国立大学(2020) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{\frac{\pi}{6}}^{\frac{\pi}{4}}\displaystyle \frac{log(\sin\ x)}{\tan\ x}\ dx$を計算せよ。
出典:2020年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{\frac{\pi}{6}}^{\frac{\pi}{4}}\displaystyle \frac{log(\sin\ x)}{\tan\ x}\ dx$を計算せよ。
出典:2020年横浜国立大学 入試問題
大学入試問題#116 岡山県立大学(2009) 定積分②
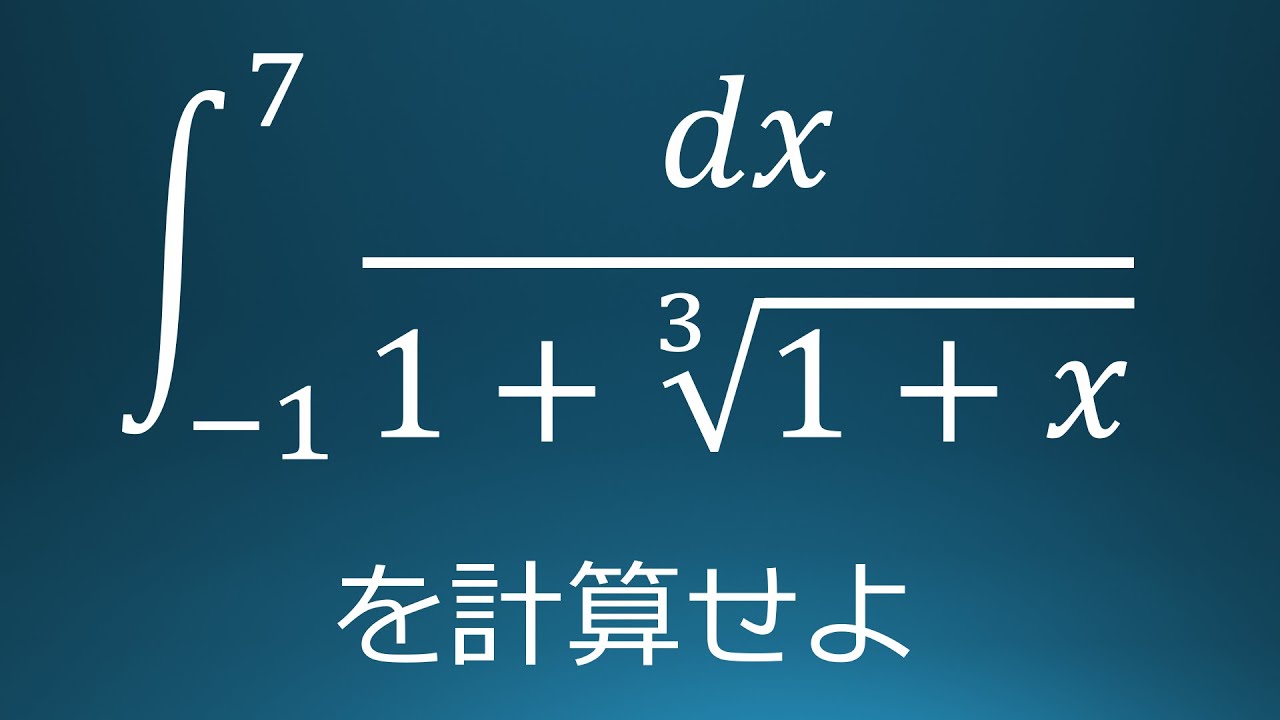
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#岡山県立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-1}^{7}\displaystyle \frac{dx}{1+\sqrt[ 3 ]{ 1+x }}$を計算せよ。
出典:2009年岡山県立大学 入試問題
この動画を見る
$\displaystyle \int_{-1}^{7}\displaystyle \frac{dx}{1+\sqrt[ 3 ]{ 1+x }}$を計算せよ。
出典:2009年岡山県立大学 入試問題
大学入試問題#114 岡山県立大学(2009) 定積分
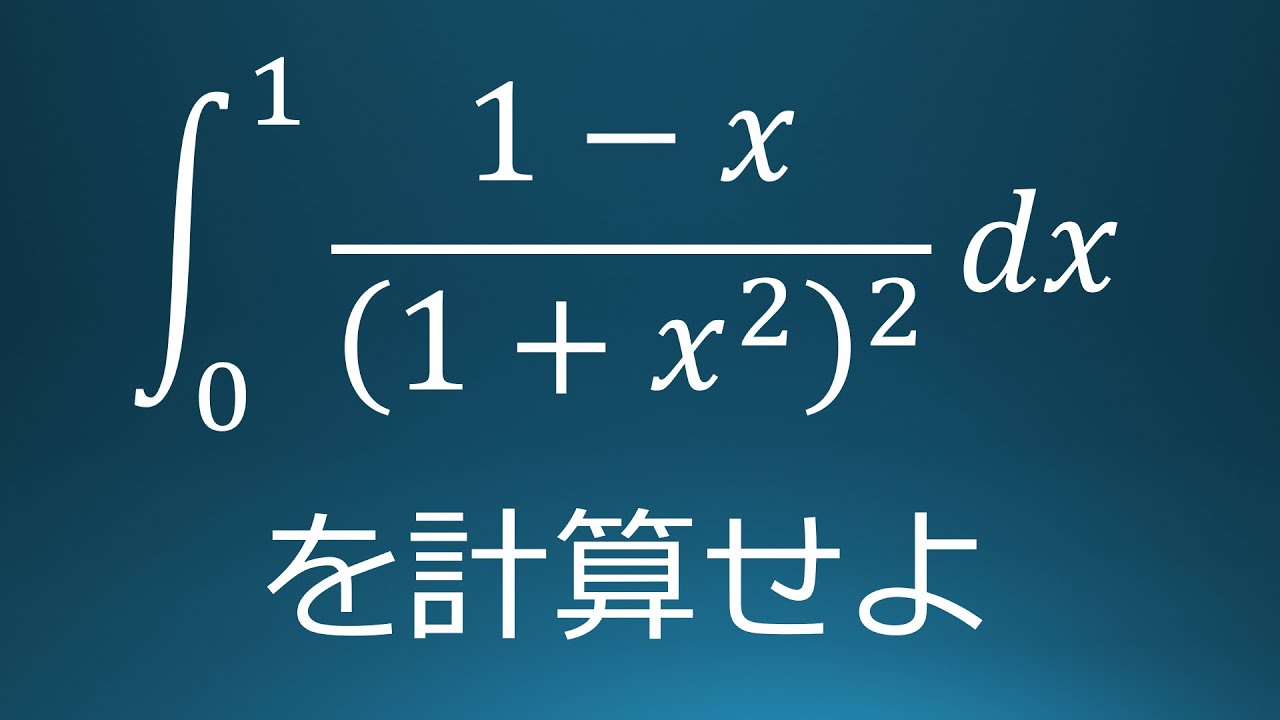
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#岡山県立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}\displaystyle \frac{1-x}{(1+x^2)^2}\ dx$を計算せよ。
出典:2009年岡山県立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}\displaystyle \frac{1-x}{(1+x^2)^2}\ dx$を計算せよ。
出典:2009年岡山県立大学 入試問題
大学入試問題#110 産業医科大学(2019) 定積分②

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}}\ \tan^4x\ dx$を計算せよ。
出典:2019年産業医科大学 入試問題
この動画を見る
$\displaystyle \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}}\ \tan^4x\ dx$を計算せよ。
出典:2019年産業医科大学 入試問題
大学入試問題#108 弘前大学(2018) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#弘前大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$a \gt 0$
$\displaystyle \int_{0}^{1}\displaystyle \frac{dx}{(e^{2x}+a)(e^{-2x}+a)}\ $を計算せよ。
出典:2018年弘前大学 入試問題
この動画を見る
$a \gt 0$
$\displaystyle \int_{0}^{1}\displaystyle \frac{dx}{(e^{2x}+a)(e^{-2x}+a)}\ $を計算せよ。
出典:2018年弘前大学 入試問題
大学入試問題#107 産業医科大学(2019) 定積分①

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#産業医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{99}^{101}\sqrt{ (x-99)(101-x) }\ dx\ $を計算せよ。
出典:2019年産業医科大学 入試問題
この動画を見る
$\displaystyle \int_{99}^{101}\sqrt{ (x-99)(101-x) }\ dx\ $を計算せよ。
出典:2019年産業医科大学 入試問題
大学入試問題#101 東海大学医学部(2017) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東海大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}x^3(1-x^2)^8dx$を計算せよ。
出典:2017年東海大学医学部 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}x^3(1-x^2)^8dx$を計算せよ。
出典:2017年東海大学医学部 入試問題
大学入試問題#98 千葉大学医学部(2018) 積分・極限
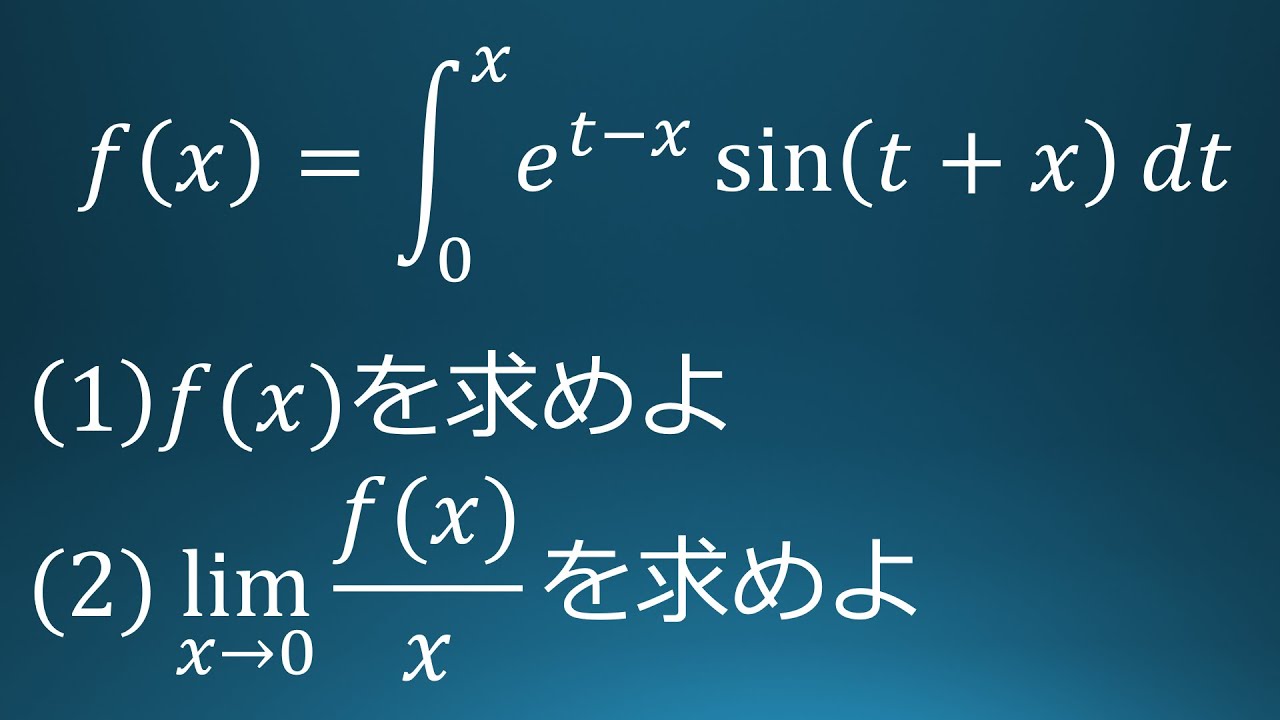
単元:
#大学入試過去問(数学)#関数と極限#積分とその応用#関数の極限#不定積分#定積分#学校別大学入試過去問解説(数学)#千葉大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
(1)
$f(x)=\displaystyle \int_{0}^{x}e^{t-x}\sin(t+x)dt$を求めよ。
(2)
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x}$を求めよ。
出典:2018年千葉大学 入試問題
この動画を見る
(1)
$f(x)=\displaystyle \int_{0}^{x}e^{t-x}\sin(t+x)dt$を求めよ。
(2)
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x}$を求めよ。
出典:2018年千葉大学 入試問題
大学入試問題#96 横浜国立大学(2015) 定積分
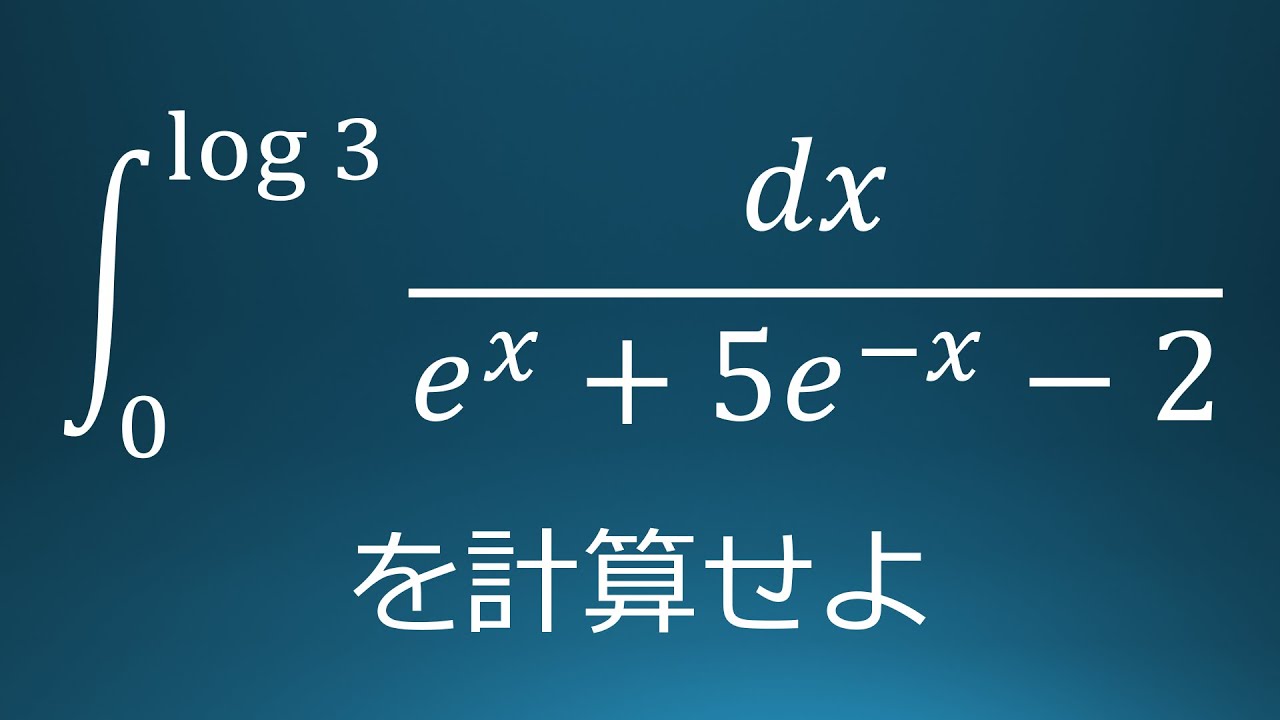
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{log\ 3}\displaystyle \frac{dx}{e^x+5e^{-x}-2}$を求めよ。
出典:2015横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{log\ 3}\displaystyle \frac{dx}{e^x+5e^{-x}-2}$を求めよ。
出典:2015横浜国立大学 入試問題
大学入試問題#95 横浜市立大学医学部(2013) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#横浜市立大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sqrt{ 2 }}{\sin\ x+\cos\ x}\ dx$を求めよ。
出典:2013年横浜市立大学医学部 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sqrt{ 2 }}{\sin\ x+\cos\ x}\ dx$を求めよ。
出典:2013年横浜市立大学医学部 入試問題
大学入試問題#94 横浜国立大学(2007) 定積分
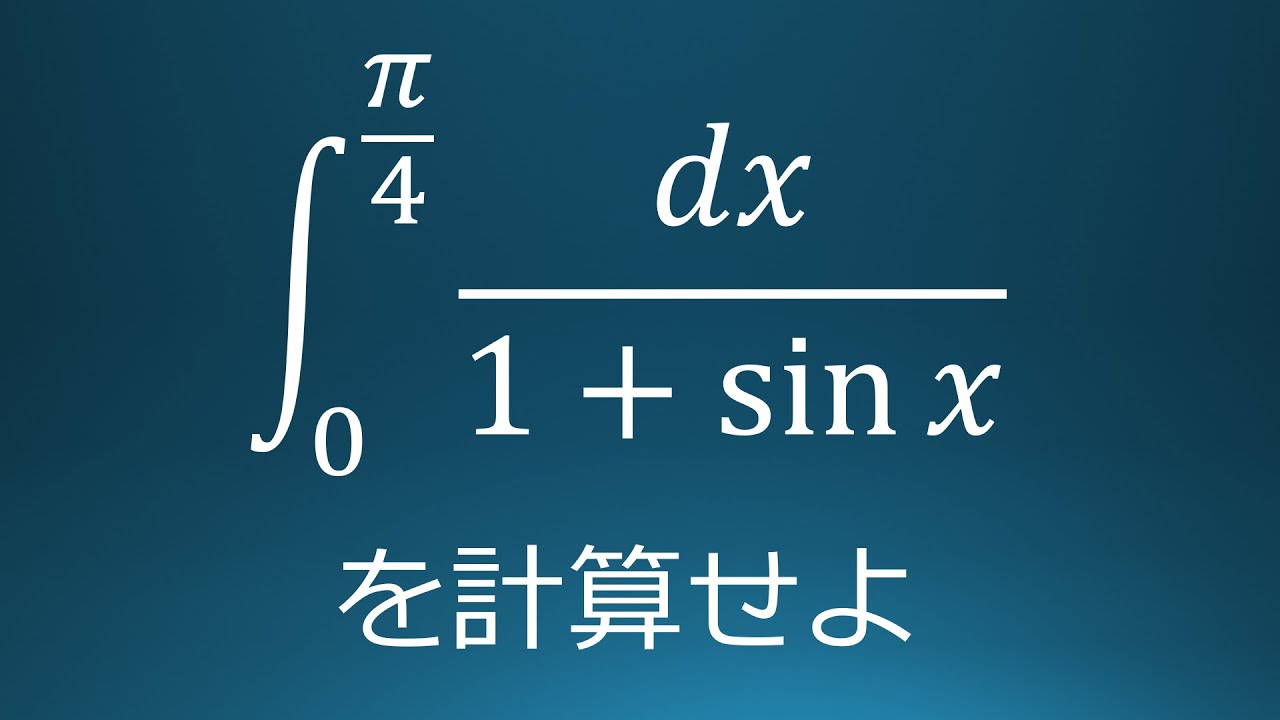
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{4}}\displaystyle \frac{dx}{1+\sin\ x}$を計算せよ。
出典:2007年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}}\displaystyle \frac{dx}{1+\sin\ x}$を計算せよ。
出典:2007年横浜国立大学 入試問題
大学入試問題#92 東京医科大学(2015) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京医科大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}(x\sqrt{ 1-x^2 })^3 dx$を計算せよ。
出典:2015年東京医科大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}(x\sqrt{ 1-x^2 })^3 dx$を計算せよ。
出典:2015年東京医科大学 入試問題
大学入試問題#82 神戸大学(2012) 複雑な置換積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#神戸大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x-\cos\ x}{1+\cos\ x}\ dx$
出典:2012年神戸大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x-\cos\ x}{1+\cos\ x}\ dx$
出典:2012年神戸大学 入試問題
大学入試問題#78 横浜国立大学(2006) 置換積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{4}}\displaystyle \frac{dx}{\sin^2x+3\cos^2x}$を計算せよ。
出典:2006年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{4}}\displaystyle \frac{dx}{\sin^2x+3\cos^2x}$を計算せよ。
出典:2006年横浜国立大学 入試問題
大学入試問題#75 横浜国立大学(2006) 部分積分
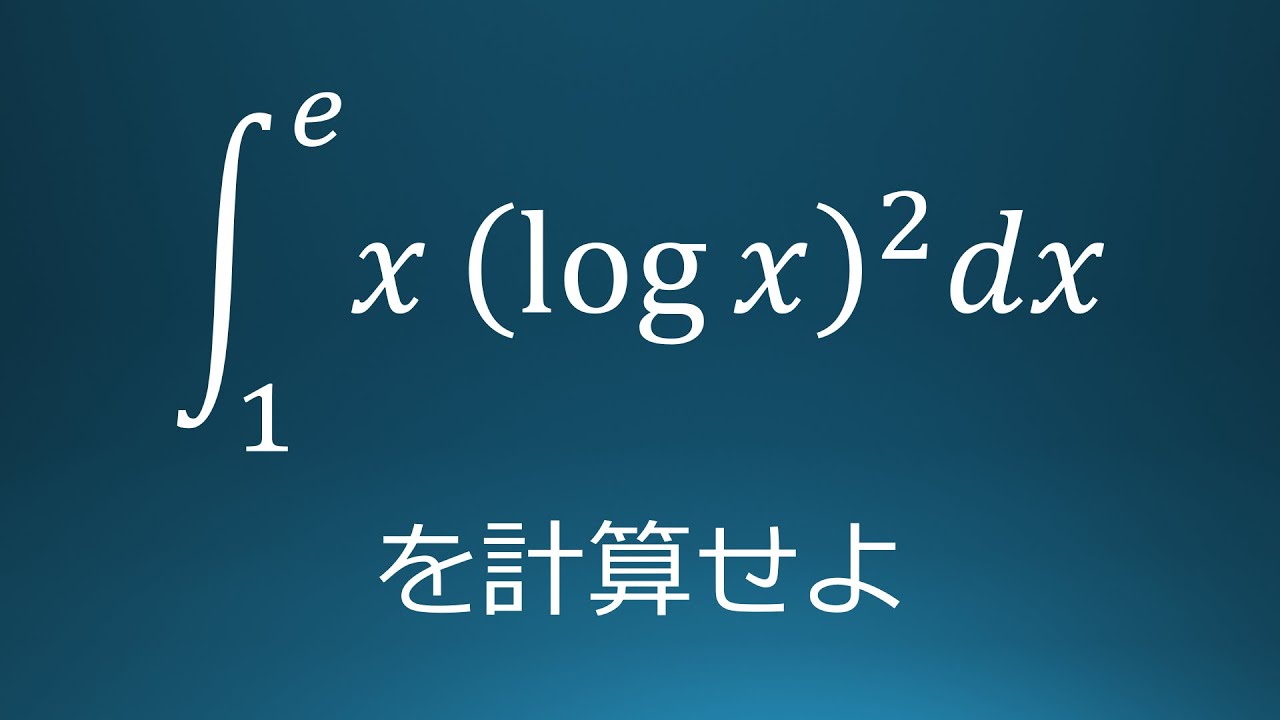
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{e}x(log\ x)^2dx$を計算せよ。
出典:2006年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{e}x(log\ x)^2dx$を計算せよ。
出典:2006年横浜国立大学 入試問題
大学入試問題#72 福岡教育大学(2009) 置換積分②

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{log\ 7}(\displaystyle \frac{e^x}{1+e^x})^3dx$を計算せよ。
出典:2009年福岡教育大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{log\ 7}(\displaystyle \frac{e^x}{1+e^x})^3dx$を計算せよ。
出典:2009年福岡教育大学 入試問題
大学入試問題#71 横浜国立大学(2005) 置換積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{log\ 3}{2}}\ \displaystyle \frac{e^x+1}{e^{2x}+1}\ dx$
出典:2005年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{log\ 3}{2}}\ \displaystyle \frac{e^x+1}{e^{2x}+1}\ dx$
出典:2005年横浜国立大学 入試問題
大学入試問題#70 鳥取大学医学部(2012) 微積の応用

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#鳥取大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$a,b$:実数
$0 \lt a \lt 2$
$\displaystyle \int_{a}^{x}f(x-t)f(t)dt=\cos(ax)-b$
(1)$a,b$の値を求めよ。
(2)$f(x)$を求めよ
(3)$f(x)$が最大値をとるときの$x$の値を求めよ。
出典:2012年鳥取大学医学部 入試問題
この動画を見る
$a,b$:実数
$0 \lt a \lt 2$
$\displaystyle \int_{a}^{x}f(x-t)f(t)dt=\cos(ax)-b$
(1)$a,b$の値を求めよ。
(2)$f(x)$を求めよ
(3)$f(x)$が最大値をとるときの$x$の値を求めよ。
出典:2012年鳥取大学医学部 入試問題
大学入試問題#68 京都大学(2012) 部分積分
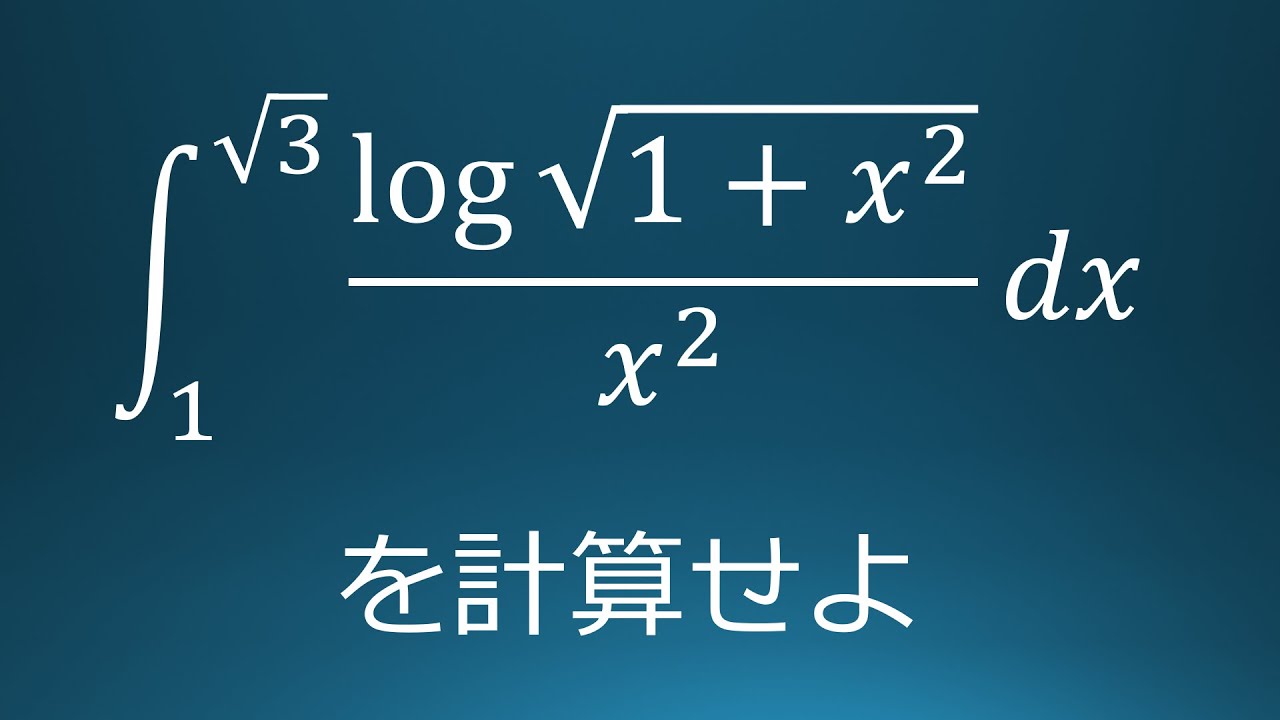
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{\sqrt{ 3 }}\displaystyle \frac{log\sqrt{ 1+x^2 }}{x^2}\ dx$
出典:2012年京都大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{\sqrt{ 3 }}\displaystyle \frac{log\sqrt{ 1+x^2 }}{x^2}\ dx$
出典:2012年京都大学 入試問題
大学入試問題#67 福岡教育大学(2009) 置換積分①

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{log7}(\displaystyle \frac{e^x}{1+e^x})^2dx$を計算せよ。
出典:2009年福岡教育大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{log7}(\displaystyle \frac{e^x}{1+e^x})^2dx$を計算せよ。
出典:2009年福岡教育大学 入試問題
大学入試問題#66 横浜国立大学(2003) 置換積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}\displaystyle \frac{x+1}{(x^2+x^1)^2}\ dx$を計算せよ。
出典:2003年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}\displaystyle \frac{x+1}{(x^2+x^1)^2}\ dx$を計算せよ。
出典:2003年横浜国立大学 入試問題
大学入試問題#65 同志社大学(2010) 複雑な部分積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#同志社大学
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{1}\sqrt{ 4x^2+1 }\ dx$を計算せよ。
出典:2010年同志社大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{1}\sqrt{ 4x^2+1 }\ dx$を計算せよ。
出典:2010年同志社大学 入試問題
大学入試問題#64 早稲田大学(1987) 置換積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{2}\displaystyle \frac{dx}{(x^2+1)\sqrt{ x^2+1 }}$を計算せよ。
出典:1987年早稲田大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{2}\displaystyle \frac{dx}{(x^2+1)\sqrt{ x^2+1 }}$を計算せよ。
出典:1987年早稲田大学 入試問題
大学入試問題#63 京都大学(2011) 気合で置換積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{1}{2}}(x+1)\sqrt{ 1-2x^2 }\ dx$を計算せよ。
出典:2011年京都大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{1}{2}}(x+1)\sqrt{ 1-2x^2 }\ dx$を計算せよ。
出典:2011年京都大学 入試問題
大学入試問題#62 横浜国立大学(2003) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{1}^{e}5^{log\ x}dx$を計算せよ。
出典:2003年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{1}^{e}5^{log\ x}dx$を計算せよ。
出典:2003年横浜国立大学 入試問題
【数Ⅲ】積分法:sin^8 xの積分をスマートに解く
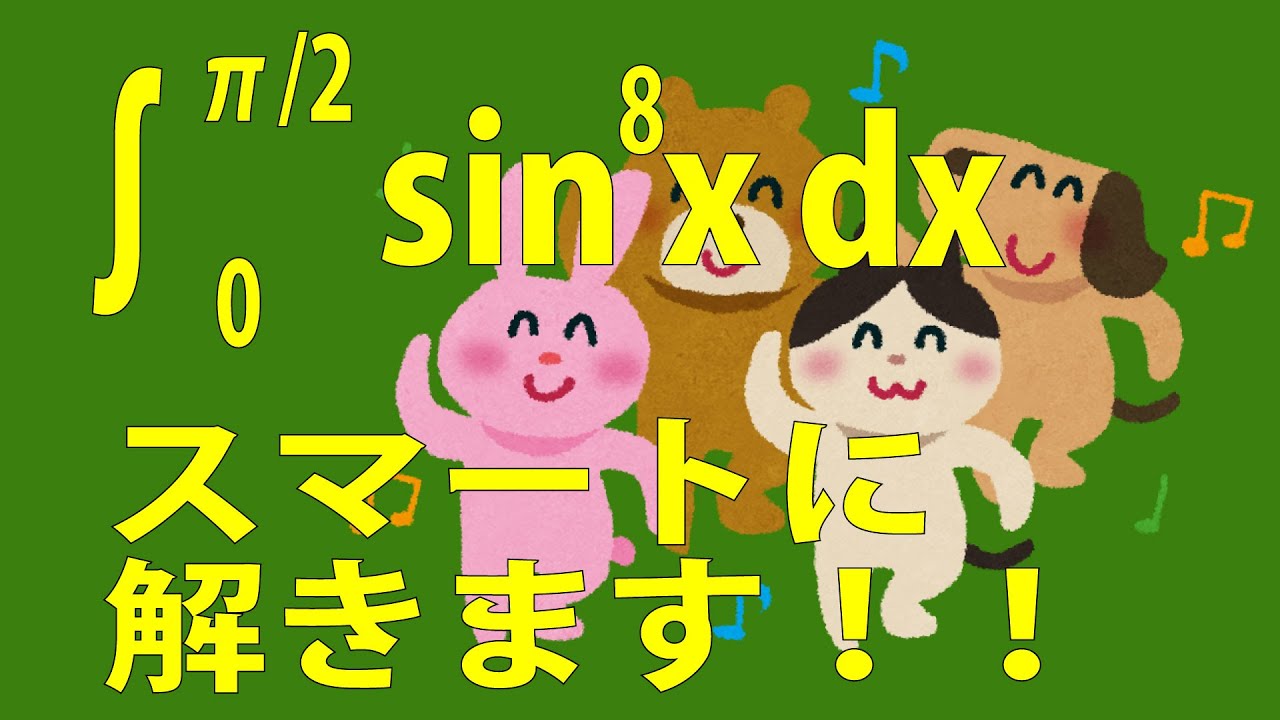
単元:
#積分とその応用#定積分#数学(高校生)#数Ⅲ
指導講師:
理数個別チャンネル
問題文全文(内容文):
$sin^8 x$の0から$\dfrac{\pi}{2}$の範囲の積分を求めよ
この動画を見る
$sin^8 x$の0から$\dfrac{\pi}{2}$の範囲の積分を求めよ
#51 大学入試問題 新潟大学(2020) 定積分【King propertyっぽいけど・・・】
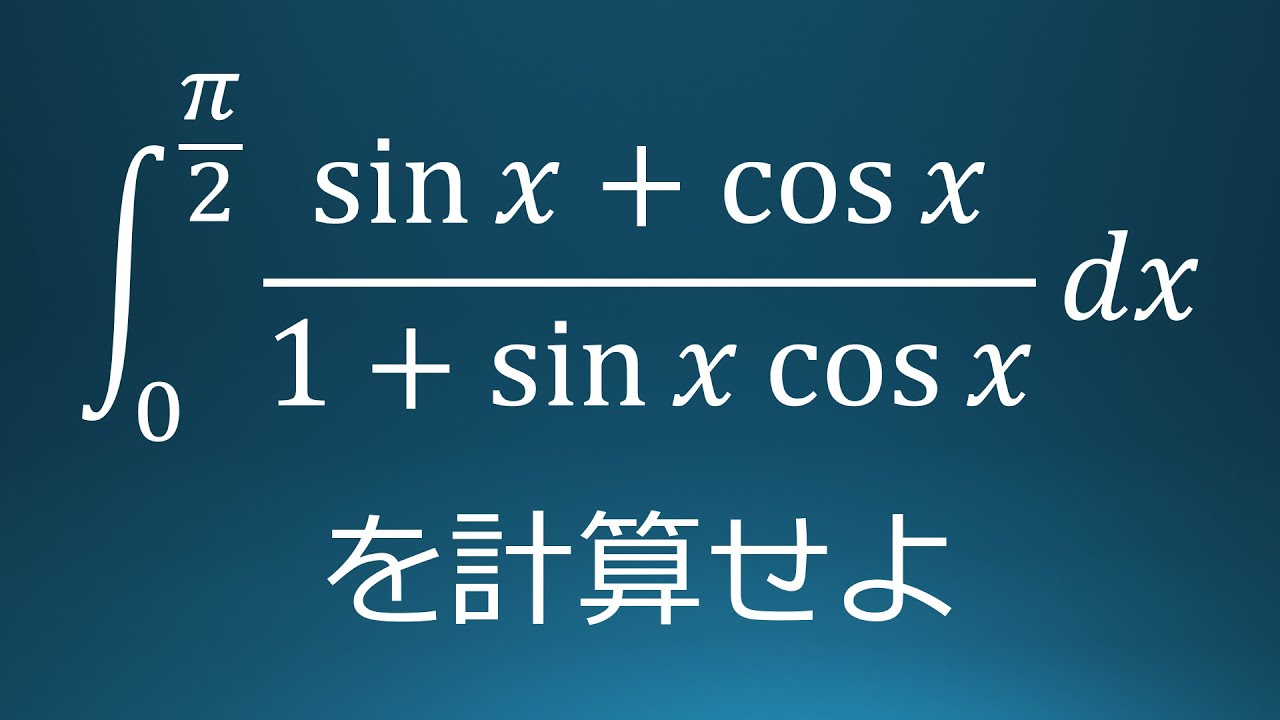
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#新潟大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x+\cos\ x}{1+\sin\ x\ \cos\ x}\ dx$を計算せよ。
出典:2020年新潟大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{\sin\ x+\cos\ x}{1+\sin\ x\ \cos\ x}\ dx$を計算せよ。
出典:2020年新潟大学 入試問題
#37 数検1級1次 過去問 重積分
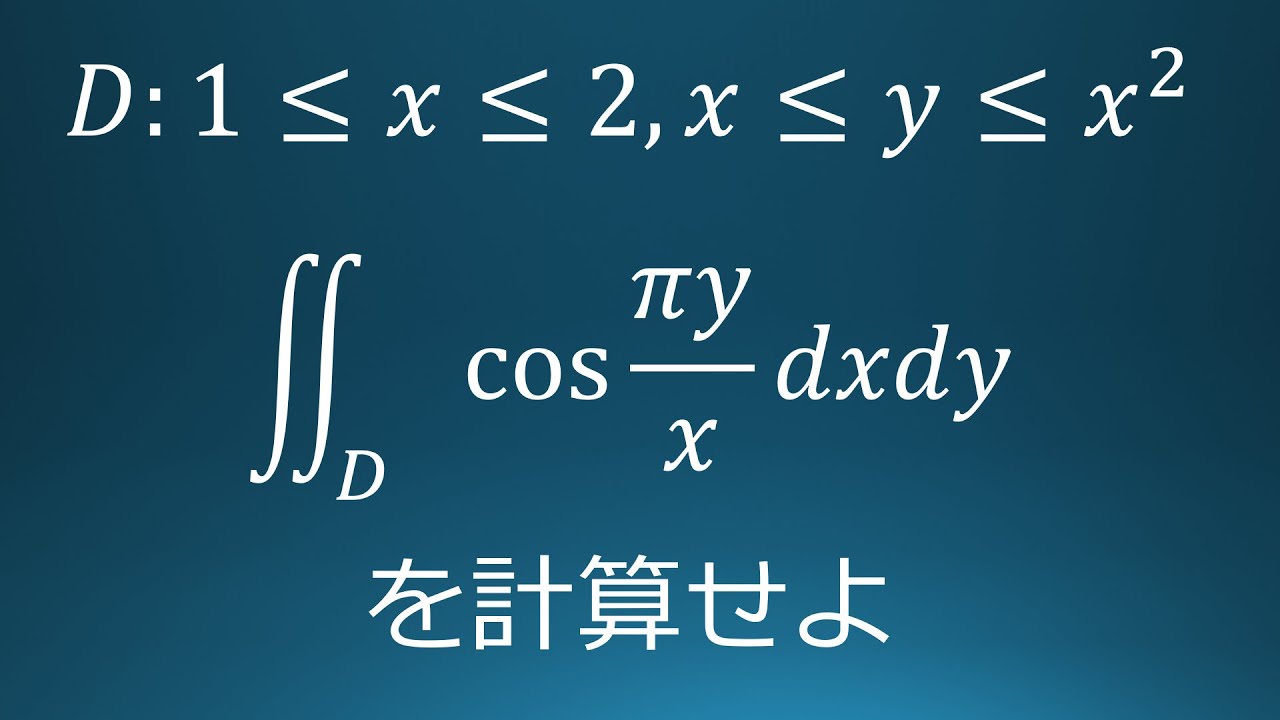
単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$D:1 \leqq x \leqq 2,x \leqq y \leqq x^2$
$\displaystyle \int \displaystyle \int \cos\displaystyle \frac{\pi y}{x}\ dxdy$を計算せよ。
この動画を見る
$D:1 \leqq x \leqq 2,x \leqq y \leqq x^2$
$\displaystyle \int \displaystyle \int \cos\displaystyle \frac{\pi y}{x}\ dxdy$を計算せよ。
#36 数検1級1次 過去問 積分

単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{-1}^{1}\sqrt{ \displaystyle \frac{1+x}{1-x} }\ dx$を計算せよ。
この動画を見る
$\displaystyle \int_{-1}^{1}\sqrt{ \displaystyle \frac{1+x}{1-x} }\ dx$を計算せよ。
#34 数検1級1次 過去問 積分

単元:
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#微分法と積分法#積分とその応用#定積分#不定積分・定積分#数学検定#数学検定1級#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
$\displaystyle \int_{0}^{\infty}\displaystyle \frac{1}{(x^2+1)^4}\ dx$を計算せよ。
この動画を見る
$\displaystyle \int_{0}^{\infty}\displaystyle \frac{1}{(x^2+1)^4}\ dx$を計算せよ。
