ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！
職業：数学者
職歴：高校非常勤（院生時代）、高専、大学 准教授
趣味：
①将棋 棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀 アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス 中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。
関西医科大学 #極限 #Shorts
単元：
#大学入試過去問(数学)#関数と極限#関数の極限#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#関西医科大学
指導講師：
ますただ
問題文全文(内容文)：
出典：関西医科大学
この動画を見る
出典：関西医科大学
大学入試問題#594「解法が見えると計算に萎えそう」 南山大学(2019) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#南山大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2019年南山大学 入試問題
この動画を見る
出典：2019年南山大学 入試問題
大学入試問題#593「カップラーメン食べながらでも解いて」 関西大学(2011) #三角関数
単元：
#数Ⅱ#大学入試過去問(数学)#三角関数#三角関数とグラフ#学校別大学入試過去問解説(数学)#数学(高校生)#関西大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2011年関西大学 入試問題
この動画を見る
出典：2011年関西大学 入試問題
大学入試問題#592「カップラーメンができる前には解きたい」 北海学園大学(2019) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：2019年北海学園大学 入試問題
この動画を見る
出典：2019年北海学園大学 入試問題
大学入試問題#591「技をかけたくなる積分」 茨城大学(2021) #定積分
単元：
#数Ⅱ#大学入試過去問(数学)#微分法と積分法#学校別大学入試過去問解説(数学)#不定積分・定積分#数学(高校生)#茨城大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2021年茨城大学 入試問題
この動画を見る
出典：2021年茨城大学 入試問題
大学入試問題#590「見た目以上に難しめ」 横浜市立大学(2020) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#横浜市立大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2020年横浜市立大学医理学部 入試問題
この動画を見る
出典：2020年横浜市立大学医理学部 入試問題
会津大学2014 #Shorts #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：2014年会津大学
この動画を見る
出典：2014年会津大学
この積分は難問「もはや積分偏差値70over」 By 英語orドイツ語シはBかHか さん
会津大学2014 #定積分 #shorts
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：2019年会津大学
この動画を見る
出典：2019年会津大学
大学入試問題#589「一度は解いておきたい良問」 奈良女子大学(2004) #数列
単元：
#大学入試過去問(数学)#数列#数列とその和（等差・等比・階差・Σ）#関数と極限#数列の極限#学校別大学入試過去問解説(数学)#奈良女子大学#数学(高校生)#数B#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：2004年奈良女子大学 入試問題
この動画を見る
出典：2004年奈良女子大学 入試問題
大学入試問題#588「なんか似た問題解いたことある。」 横浜市立大学(2020) #方程式
単元：
#数Ⅱ#大学入試過去問(数学)#複素数と方程式#剰余の定理・因数定理・組み立て除法と高次方程式#学校別大学入試過去問解説(数学)#数学(高校生)#横浜市立大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2020年横浜市立大学医学部 入試問題
この動画を見る
出典：2020年横浜市立大学医学部 入試問題
大学入試問題#587「たぶん基本問題」 広島市立大学(2013) #不定積分
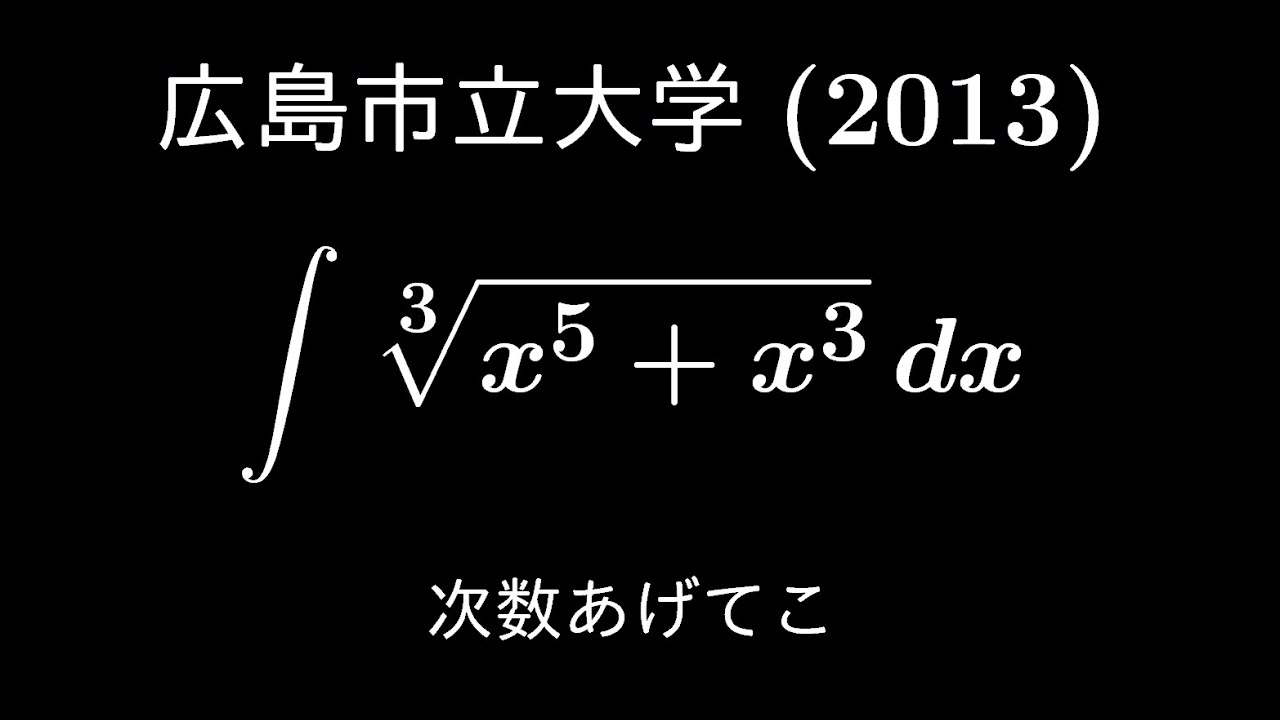
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2013年広島市立大学 入試問題
この動画を見る
出典：2013年広島市立大学 入試問題
大学入試問題#587「落とせない問題」 京都大学(1960) #方程式
単元：
#数Ⅱ#大学入試過去問(数学)#複素数と方程式#剰余の定理・因数定理・組み立て除法と高次方程式#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
出典：1960年京都大学 入試問題
この動画を見る
出典：1960年京都大学 入試問題
広島市立大学 2016 #不定積分 #Shorts
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2016年広島市立大学
この動画を見る
出典：2016年広島市立大学
大学入試問題#586「見た目が美しい」 広島市立大学(2009) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2009年広島市立大学 入試問題
この動画を見る
出典：2009年広島市立大学 入試問題
大学入試問題#585「気付けば暗算」 同志社大学(2004) #極限
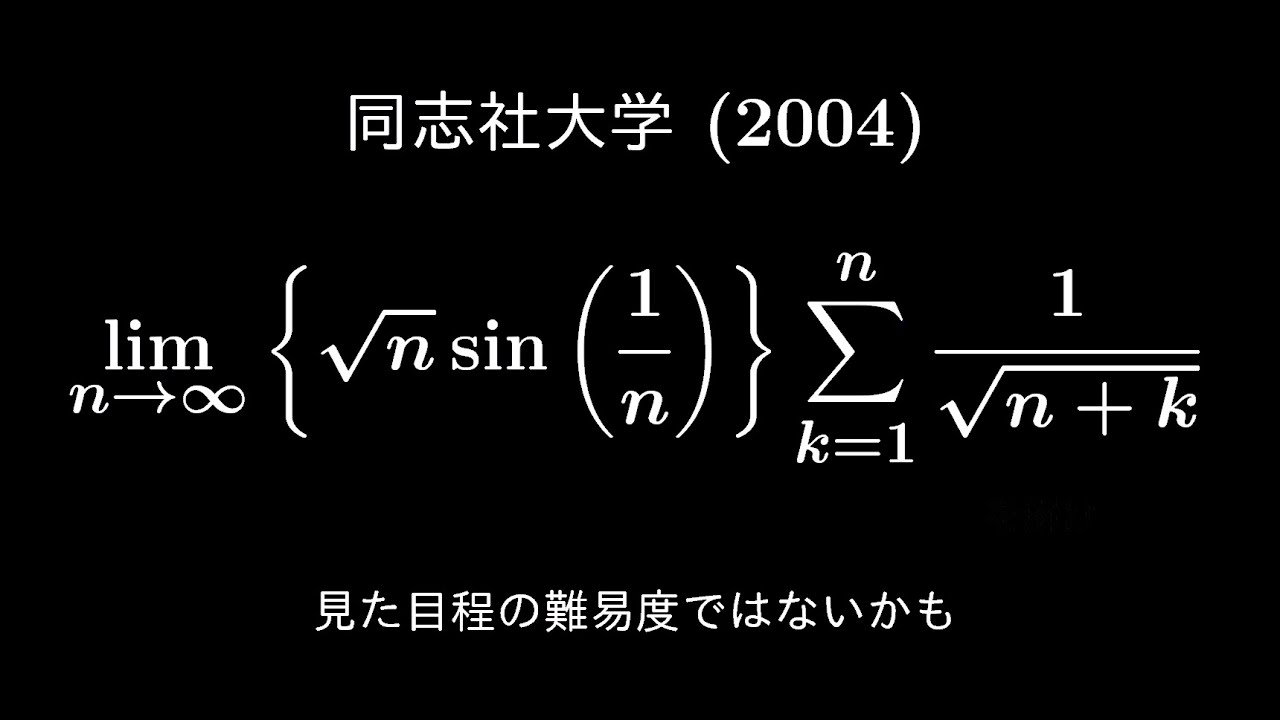
単元：
#大学入試過去問(数学)#関数と極限#積分とその応用#関数の極限#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#同志社大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2004年同志社大学 入試問題
この動画を見る
出典：2004年同志社大学 入試問題
大学入試問題#584「これは落としたくない！！」 京都帝国大学(1937) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：1937年京都帝国大学 入試問題
この動画を見る
出典：1937年京都帝国大学 入試問題
広島市立大学(2009) #不定積分 #Shorts
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2009年広島市立大学
この動画を見る
出典：2009年広島市立大学
#58数検1級1次「ぱっと見はちょろそうだけど・・・」 #方程式
単元：
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#複素数と方程式#剰余の定理・因数定理・組み立て除法と高次方程式#数学検定#数学検定1級#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
方程式
出典：数検1級1次
この動画を見る
方程式
出典：数検1級1次
大学入試問題#582「ガチンコでぶつかると危険」 東京帝国大学(1946) 不定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：1946年東京帝国大学 入試問題
この動画を見る
出典：1946年東京帝国大学 入試問題
#57数検準1級1次「ほぼ1本道」 #極限
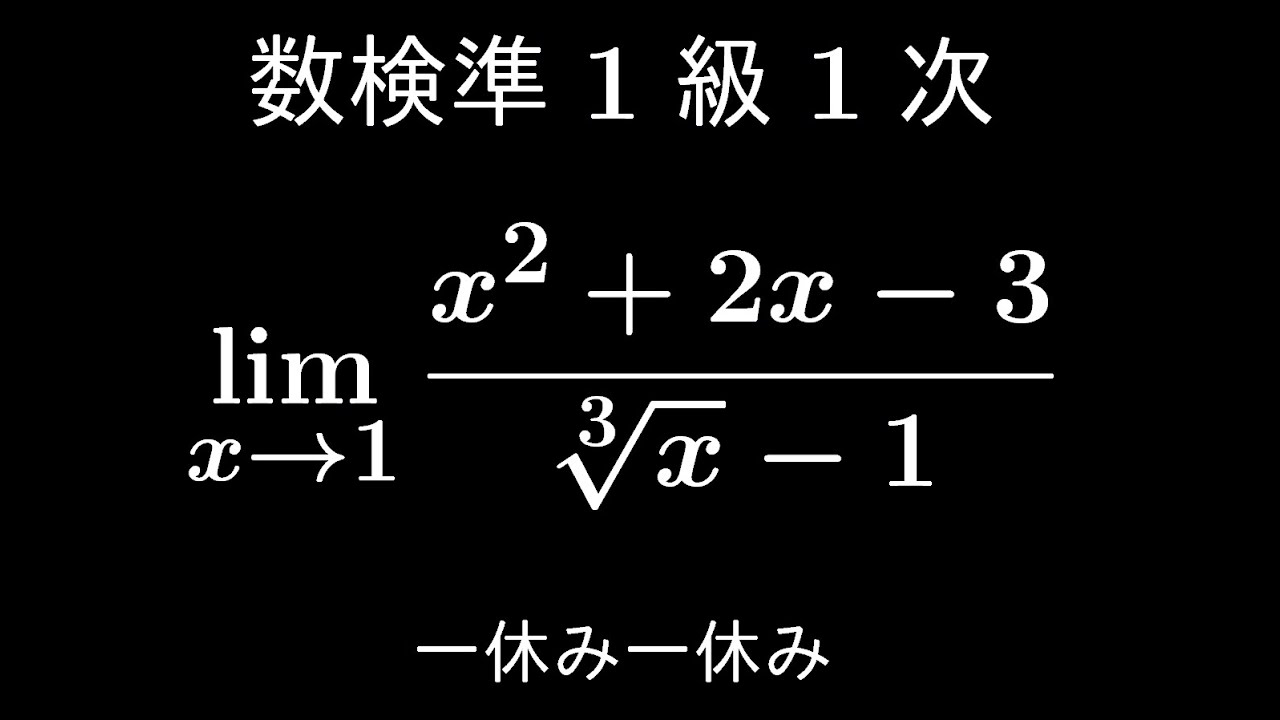
単元：
#数学検定・数学甲子園・数学オリンピック等#関数と極限#関数の極限#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：数検準1級1次
この動画を見る
出典：数検準1級1次
東邦大学医学部(2011) #Shorts #King_property #キングプロパティ
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東邦大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2011年東邦大学医学部 入試問題
この動画を見る
出典：2011年東邦大学医学部 入試問題
#57数検準1級1次 #定積分
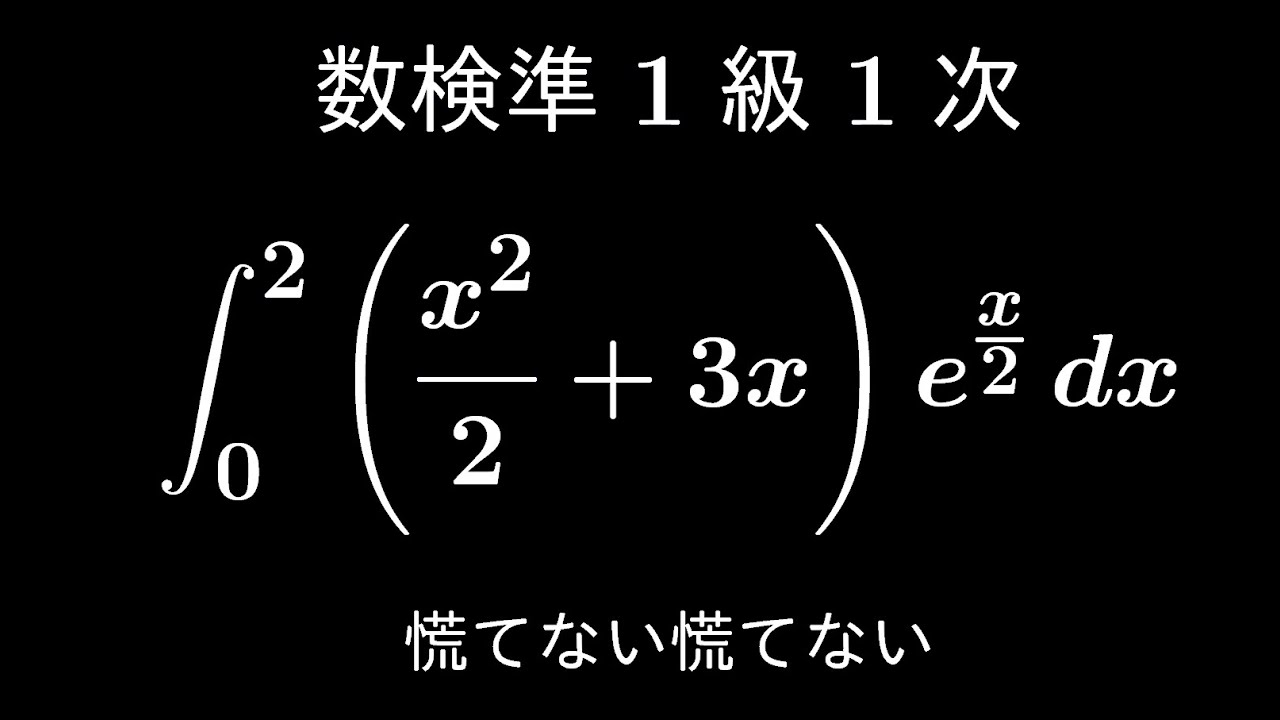
単元：
#数学検定・数学甲子園・数学オリンピック等#積分とその応用#定積分#数学検定#数学検定準1級#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：数検準1級1次
この動画を見る
出典：数検準1級1次
大学入試問題#581「最後まで落ち着いて！」 東京帝国大学(1940) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：1940年東京帝国大学 入試問題
この動画を見る
出典：1940年東京帝国大学 入試問題
#56数検１級１次 過去問 #4次方程式
単元：
#数Ⅱ#数学検定・数学甲子園・数学オリンピック等#複素数と方程式#剰余の定理・因数定理・組み立て除法と高次方程式#数学検定#数学検定1級#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
方程式
出典：数検1級1次
この動画を見る
方程式
出典：数検1級1次
大学入試問題#580「これは落としたくない」 東邦大学医学部(2017) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東邦大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2017年東邦大学医学部 入試問題
この動画を見る
出典：2017年東邦大学医学部 入試問題
大学入試問題#579「技のかけ方は好みでしょうか」 京都帝国大学(1939) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：1939年京都帝国大学 入試問題
この動画を見る
出典：1939年京都帝国大学 入試問題
大学入試問題#578「定数aにかまっていられない」 京都帝国大学(1939) #定積分
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：1939年京都帝国大学 入試問題
この動画を見る
出典：1939年京都帝国大学 入試問題
東邦大学医学部医学科(2015) #Shorts #King_property #キングプロパティ
単元：
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東邦大学
指導講師：
ますただ
問題文全文(内容文)：
出典：2015年東邦大学医学部医学科
この動画を見る
出典：2015年東邦大学医学部医学科
大学入試問題#576「逆に閃かないと苦戦」 京都帝国大学(1938) #不定積分
単元：
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師：
ますただ
問題文全文(内容文)：
出典：1938年京都帝国大学 入試問題
この動画を見る
出典：1938年京都帝国大学 入試問題