加法定理とその応用

【数学II】加法定理の証明の仕方を理解して覚える動画！

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：カサニマロ【べんとう・ふきのとうの授業動画】

問題文全文(内容文)：
【数学II】加法定理の証明についての説明動画です

この動画を見る　

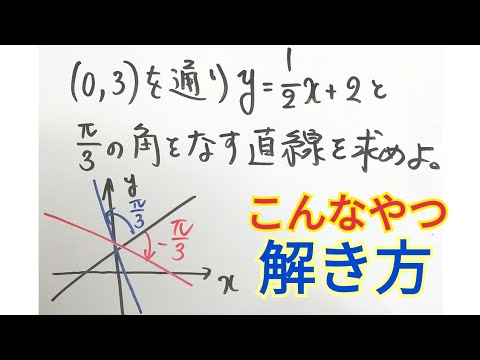
【数学II】tanθの加法定理と直線の方程式

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：カサニマロ【べんとう・ふきのとうの授業動画】

問題文全文(内容文)：
【数学II】tanθの加法定理と直線の方程式の解説動画です
-----------------
(0,3)を通り、直線

y = \frac{1}{2} x + 2

と

\frac{π}{3}

の角をなす直線の方程式を求めよ。

この動画を見る　

九州大　良問再投稿　合成公式

単元： #数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#九州大学

指導講師：鈴木貫太郎

問題文全文(内容文)：

\sin 10^{\circ}

は

8 x^{3} - 6 x + 1 = 0

の解であることを示し、他の2解も求めよ

出典：1975年九州大学　過去問

この動画を見る　

東大卒のもっちゃんと数学Vol.7 加法定理を証明しよう（東大過去問）

単元： #数Ⅱ#大学入試過去問(数学)#図形と計量#三角比（三角比・拡張・相互関係・単位円）#三角比への応用（正弦・余弦・面積）#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：
加法定理を証明　解説動画です

\cos (α + β) = \cos α \cos β - \sin α \sin β

この動画を見る　

【数Ⅱ】三角関数：関数y=sin²x-cos²x+2√3xsinxcosx(0≦x＜2π)の最大値・最小値及び、そのときのxの値を求めよ。

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：理数個別チャンネル

問題文全文(内容文)：
関数

y = \sin^{2} x - \cos^{2} x + 2 \sqrt{3} x \sin x \cos x (0 ≦ x < 2 π)

の最大値・最小値及び、そのときのxの値を求めよ。

この動画を見る　

弘前大　３倍角　５倍角 Mathematics Japanese university entrance exam

単元： #数Ⅱ#大学入試過去問(数学)#複素数平面#三角関数#加法定理とその応用#複素数平面#学校別大学入試過去問解説(数学)#数学(高校生)#弘前大学#数C

指導講師：鈴木貫太郎

問題文全文(内容文)：
（1）

\sin 3 x

を

\sin x

で表せ

（2）

\sin x + \cos x = 4 \sin x \cos^{2} x

を満たす

x

を求めよ

出典：1986年弘前大学　過去問

この動画を見る　

京都大　5倍角高校数学 Mathematics Japanese university entrance exam Kyoto University

単元： #大学入試過去問(数学)#三角関数#恒等式・等式・不等式の証明#加法定理とその応用#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：
96年　京都大学過去問
(1)

\cos 5 θ = f (\cos θ)

をみたす多項式

f (x)

をもとめよ。

(2)

\cos \frac{π}{10} \cos \frac{3 π}{10} \cos \frac{7 π}{10} \cos \frac{9 π}{10} = \frac{5}{16}

を示せ。

この動画を見る　

【高校数学】一緒に解こう三角関数の合成 4-15【数学Ⅱ】

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
(1) 0≦x<2πのとき、次の方程式を解け。
　　sin x-

\sqrt{3}

cos x=1

(2)次の関数の最大値と最小値、およびそのときのxの値を求めよ。
　 y=sin x+cos x(0≦x≦2π)

この動画を見る　

【高校数学】加法定理③～三角関数の合成～ 4-14【数学Ⅱ】

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
【三角関数の合成】

a sinθ+b cosθ=

\sqrt{a ² + b ²}

sin(θ+α)(=r sin(θ+α))
ただし、sinα=

\frac{b}{\sqrt{a ² + b ²}}

,cos α=

\frac{a}{\sqrt{a ² + b ²}}

,r=

\sqrt{a ² + b ²}

である。

(1) 三角関数を合成せよ
sinθ+

\sqrt{3}

cosθ

(2) 0≦x<2πのとき、次の方程式を解け
sin x-

\sqrt{3}

cosx=1

この動画を見る　

【高校数学】加法定理②～２倍角・半角の公式～ 4-13【数学Ⅱ】

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
加法定理２倍角・半角の公式の説明動画です

この動画を見る　

【高校数学】加法定理①～語呂合わせで覚える加法定理～ 4-12【数学Ⅱ】

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
加法定理の説明動画です

この動画を見る　

京都大三角比 Mathematics Japanese university entrance exam Kyoto University

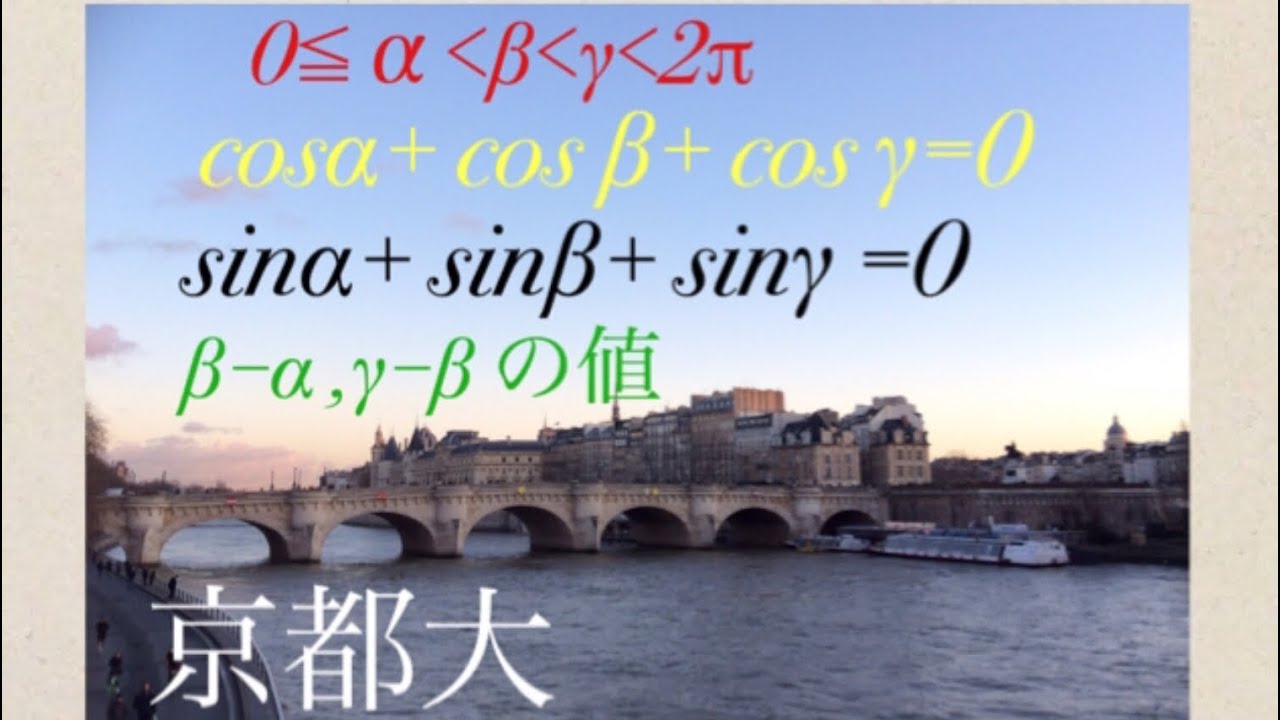
単元： #数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：
京都大学過去問題

0 ≦ α ＜ β ＜ γ ＜ 2 π

c o s α + c o s β + c o s γ = 0

s i n α + s i n β + s i n γ = 0

である
β-α、γ-βの値を求めよ。

この動画を見る　

【高校数学】三角関数⑨～今までの応用～ 4-11【数学Ⅱ】

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：【楽しい授業動画】あきとんとん

問題文全文(内容文)：
(1) 0≦θ<2πのとき、次の関数の最大値と最小値を求めよ。
　　そのときのθの値を求めよ。
　　y=cos²θ-4sinθ+2

(2) 0≦θ<2πのとき、次の方程式を満たすθの値を求めよ。
　　2sin²θ-5cosθ+5=0

この動画を見る　

北海道大　式の最大値 Mathematics Japanese university entrance exam

単元： #数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#北海道大学

指導講師：鈴木貫太郎

問題文全文(内容文)：
北海道大学過去問題
x,y実数

x^{2} + y^{2} = 1

を満たす

\sqrt{3} x^{2} + 2 x y - \sqrt{3} y^{2}

の最大値と、そのときのx,yの値

この動画を見る　

京都府立医・長崎大　三角関数　高校数学 Mathematics Japanese university entrance exam

単元： #数Ⅱ#大学入試過去問(数学)#三角関数#三角関数とグラフ#加法定理とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#長崎大学#京都府立医科大学

指導講師：鈴木貫太郎

問題文全文(内容文)：
京都府立医科大学

s i n x + s i n 2 x + s i n 3 x = c o s x + c o s 2 x

+ c o s 3 x

を解け

長崎大学過去問題

0 ≦ x ≦ π

cos2x+4asinx+a-2=0
相異2実根をもつaの範囲

この動画を見る　

札幌医大　三角方程式　高校数学 Mathematics Japanese university entrance exam

単元： #三角関数とグラフ#加法定理とその応用

指導講師：鈴木貫太郎

問題文全文(内容文)：
札幌医科大学過去問題
xに関する方程式

c o s 2 x + a c o s x + b = 0

この方程式

0 ≦ x < 2 π

の範囲で2個の異なる実数解を持つためのa,bに関する条件

この動画を見る　

放物線　光は1点に集る

単元： #数Ⅰ#数Ⅱ#２次関数#三角関数#微分法と積分法#加法定理とその応用#接線と増減表・最大値・最小値#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：

y = x

に

y

軸ｔ平行に入った光はある一点を必ず通ることを示せ.

この動画を見る　

広島大　円の方程式　三角比　高校数学 Japanese university entrance exam questions

単元： #数Ⅱ#大学入試過去問(数学)#図形と方程式#三角関数#円と方程式#加法定理とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#広島大学

指導講師：鈴木貫太郎

問題文全文(内容文)：
広島大学過去問題
2つの円

x^{2} + y^{2} + (2 \sqrt{2} s i n θ) x - \frac{\sqrt{17}}{2} y + s i n^{2} θ +

\frac{17}{16} = 0

x^{2} + y^{2} = \frac{9}{16} (0^{\circ} < θ < 180^{\circ})

が共有点をもたないようなθの範囲を求めよ。

この動画を見る　

九州大学　三倍角　高校数学 Japanese university entrance exam questions

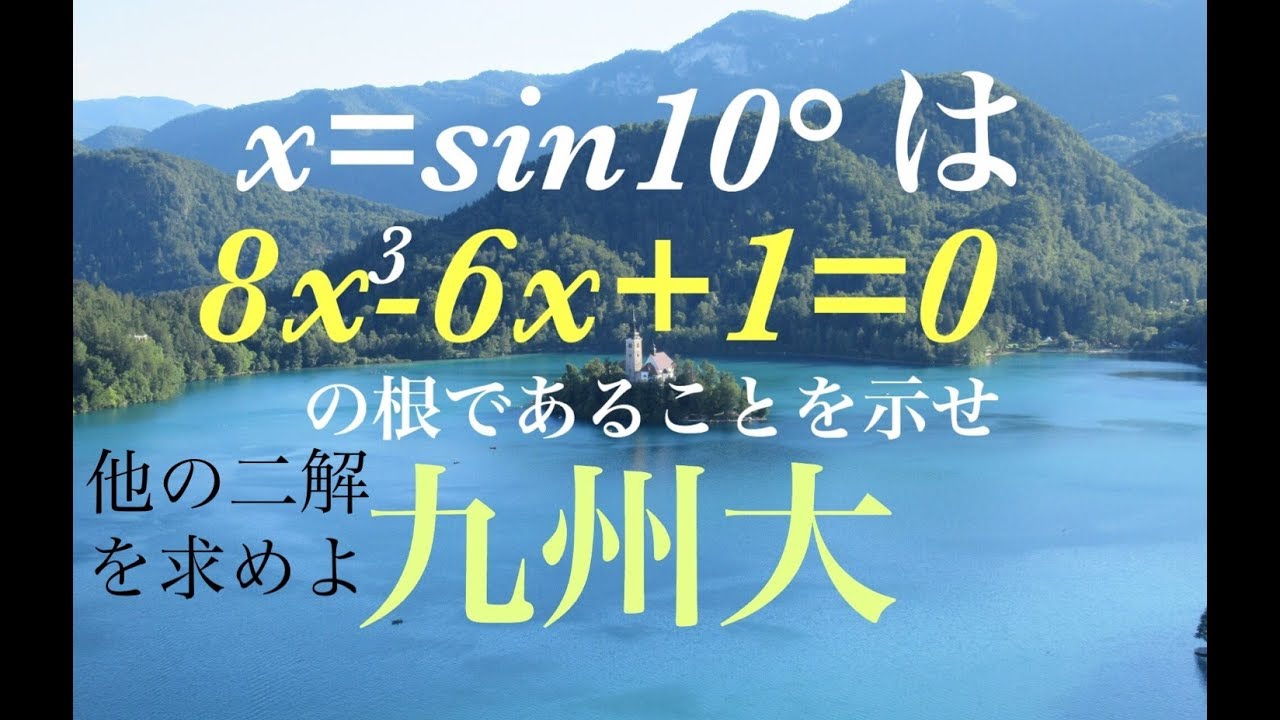
単元： #数Ⅱ#大学入試過去問(数学)#三角関数#加法定理とその応用#学校別大学入試過去問解説(数学)#数学(高校生)#九州大学

指導講師：鈴木貫太郎

問題文全文(内容文)：
九州大学過去問題
(1)

\sin 10^{\circ}

は3次方程式

8 x^{3} - 6 x + 1 = 0

の解であることを示せ。
(2)他の2解を求めよ。

この動画を見る　

横浜市立(医)　正二十面体　面のなす角　高校数学 Japanese university entrance exam questions

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：
'94横浜市立大学過去問題
(1)正五角形ABCDEの一辺を1としたときのAD=ACの長さ
(2)正二十面体のとなり合う面のなす角をθとしたときのcosθの値

この動画を見る　

福田の一夜漬け数学〜多変数関数、１文字固定その２（受験編）

単元： #数Ⅱ#式と証明#三角関数#恒等式・等式・不等式の証明#加法定理とその応用#数学(高校生)

指導講師：福田次郎

問題文全文(内容文)：

△ A B C

において次の不等式を示せ。
(1)

\cos A + \cos B + \cos C ≦ \frac{3}{2}

(2)

\cos A \cos B \cos C ≦ \frac{1}{8}

この動画を見る　

組立除法、三角関数の合成、視聴者からの質問への返答

単元： #複素数と方程式#三角関数#剰余の定理・因数定理・組み立て除法と高次方程式#加法定理とその応用#数学(高校生)

指導講師：鈴木貫太郎

問題文全文(内容文)：
組立除法、三角関数の合成、視聴者からの質問への返答です.

\begin{array}{r} x - α a x^{3} + b x^{2} + c x + d \end{array}

この動画を見る　

【数学】３分で和積公式が馬鹿でもわかる考え方

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：カサニマロ【べんとう・ふきのとうの授業動画】

問題文全文(内容文)：
【数学】３分で和積公式解説動画です

この動画を見る　

【数学】４分で積和公式が馬鹿でもわかる考え方

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：カサニマロ【べんとう・ふきのとうの授業動画】

問題文全文(内容文)：
【数学】４分で積和公式解説動画です

この動画を見る　

【高校数学】　数Ⅱ－１２０　三角関数の合成③

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎

0 ≦ x < 2 π

のとき、次の不等式を解こう。

①

\sin x - \sqrt{3} \cos x > - 1

②

\sqrt{3} \sin x - \cos x ≦ \sqrt{2}

この動画を見る　

【高校数学】　数Ⅱ－１１９　三角関数の合成②

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎

0 ≦ x < 2 π

のとき、次の方程式を解こう。

①

\sqrt{3} \sin x - \cos x = \sqrt{3}

②

2 (\sin x + \cos x) - \sqrt{6}

この動画を見る　

【高校数学】　数Ⅱ－１１８　三角関数の合成①

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：
◎次の式を

r s i n (θ + α)

の形に変形しよう。ただし、

r > 0, - π < α < π

とする。

①

\sqrt{3} \sin θ + \cos θ

②

\sqrt{2} \sin θ - \sqrt{6} \cos θ

③

3 \sin θ + 4 \cos θ

この動画を見る　

【高校数学】　数Ⅱ－１１７　和と積の公式②・和(差)→積編

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：

\sin A + \sin B =

①＿＿＿＿＿＿＿＿＿＿＿＿

\cos A + \cos B =

②＿＿＿＿＿＿＿＿＿＿＿＿

\sin A - \sin B =

③＿＿＿＿＿＿＿＿＿＿＿＿

\cos A - \cos B =

④＿＿＿＿＿＿＿＿＿＿＿＿

◎次の値を求めよう。

⑤

\sin 105 ° + \sin 15 °

⑥

\cos 75 ° - \sin 15 °

⑦

\cos 75 ° + \cos 15 °

この動画を見る　

【高校数学】　数Ⅱ－１１５　三角関数を含む方程式・不等式⑧

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：

0 ≦ x < 2 π

のとき、次の不等式を解こう。

①

\cos 2 x ≦ 3 \sin x - 1

②

\sin 2 x > \sin x

この動画を見る　

【高校数学】　数Ⅱ－１１４　三角関数を含む方程式・不等式⑦

単元： #数Ⅱ#三角関数#加法定理とその応用#数学(高校生)

指導講師：とある男が授業をしてみた

問題文全文(内容文)：

0 ≦ x < 2 π

のとき、次の方程式を書こう。

①

2 \cos 2 x + 1 = 4 \sin x

②

\sin 2 x = \cos x

この動画を見る　

<img src="https://kaiketsu-db.net/wp-content/uploads/2021/11/112-book-morph-outline.gif" alt="" data-eio="l"> 加法定理とその応用

【数学II】加法定理の証明の仕方を理解して覚える動画！

【数学II】tanθの加法定理と直線の方程式

九州大 良問再投稿 合成公式

東大卒のもっちゃんと数学Vol.7 加法定理を証明しよう（東大過去問）

【数Ⅱ】三角関数：関数y=sin²x-cos²x+2√3xsinxcosx(0≦x＜2π)の最大値・最小値及び、そのときのxの値を求めよ。

弘前大 ３倍角 ５倍角 Mathematics Japanese university entrance exam

京都大 5倍角 高校数学 Mathematics Japanese university entrance exam Kyoto University

【高校数学】一緒に解こう三角関数の合成 4-15【数学Ⅱ】

【高校数学】加法定理③～三角関数の合成～ 4-14【数学Ⅱ】

【高校数学】加法定理②～２倍角・半角の公式～ 4-13【数学Ⅱ】

【高校数学】加法定理①～語呂合わせで覚える加法定理～ 4-12【数学Ⅱ】

京都大 三角比 Mathematics Japanese university entrance exam Kyoto University

【高校数学】三角関数⑨～今までの応用～ 4-11【数学Ⅱ】

北海道大 式の最大値 Mathematics Japanese university entrance exam

京都府立医・長崎大 三角関数 高校数学 Mathematics Japanese university entrance exam

札幌医大 三角方程式 高校数学 Mathematics Japanese university entrance exam

放物線 光は1点に集る

広島大 円の方程式 三角比 高校数学 Japanese university entrance exam questions

九州大学 三倍角 高校数学 Japanese university entrance exam questions

横浜市立(医) 正二十面体 面のなす角 高校数学 Japanese university entrance exam questions

福田の一夜漬け数学〜多変数関数、１文字固定その２（受験編）

組立除法、三角関数の合成、視聴者からの質問への返答

【数学】３分で和積公式が馬鹿でもわかる考え方

【数学】４分で積和公式が馬鹿でもわかる考え方

【高校数学】 数Ⅱ－１２０ 三角関数の合成③

【高校数学】 数Ⅱ－１１９ 三角関数の合成②

【高校数学】 数Ⅱ－１１８ 三角関数の合成①

【高校数学】 数Ⅱ－１１７ 和と積の公式②・和(差)→積編

【高校数学】 数Ⅱ－１１５ 三角関数を含む方程式・不等式⑧

【高校数学】 数Ⅱ－１１４ 三角関数を含む方程式・不等式⑦

九州大　良問再投稿　合成公式

弘前大　３倍角　５倍角 Mathematics Japanese university entrance exam

京都大　5倍角高校数学 Mathematics Japanese university entrance exam Kyoto University

京都大三角比 Mathematics Japanese university entrance exam Kyoto University

北海道大　式の最大値 Mathematics Japanese university entrance exam

京都府立医・長崎大　三角関数　高校数学 Mathematics Japanese university entrance exam

札幌医大　三角方程式　高校数学 Mathematics Japanese university entrance exam

放物線　光は1点に集る

広島大　円の方程式　三角比　高校数学 Japanese university entrance exam questions

九州大学　三倍角　高校数学 Japanese university entrance exam questions

横浜市立(医)　正二十面体　面のなす角　高校数学 Japanese university entrance exam questions

【高校数学】　数Ⅱ－１２０　三角関数の合成③

【高校数学】　数Ⅱ－１１９　三角関数の合成②

【高校数学】　数Ⅱ－１１８　三角関数の合成①

【高校数学】　数Ⅱ－１１７　和と積の公式②・和(差)→積編

【高校数学】　数Ⅱ－１１５　三角関数を含む方程式・不等式⑧

【高校数学】　数Ⅱ－１１４　三角関数を含む方程式・不等式⑦