 ますただ
ますただ
 ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
大学入試問題#213 広島市立大学(2015) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#広島市立大学
指導講師:
ますただ
問題文全文(内容文):
出典:2015年広島市立大学 入試問題
この動画を見る
出典:2015年広島市立大学 入試問題
大学入試問題#212 滋賀県立大学(2017) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#滋賀県立大学
指導講師:
ますただ
問題文全文(内容文):
出典:2017年滋賀県立大学 入試問題
この動画を見る
出典:2017年滋賀県立大学 入試問題
大学入試問題#211 宮崎大学(2018) 定積分
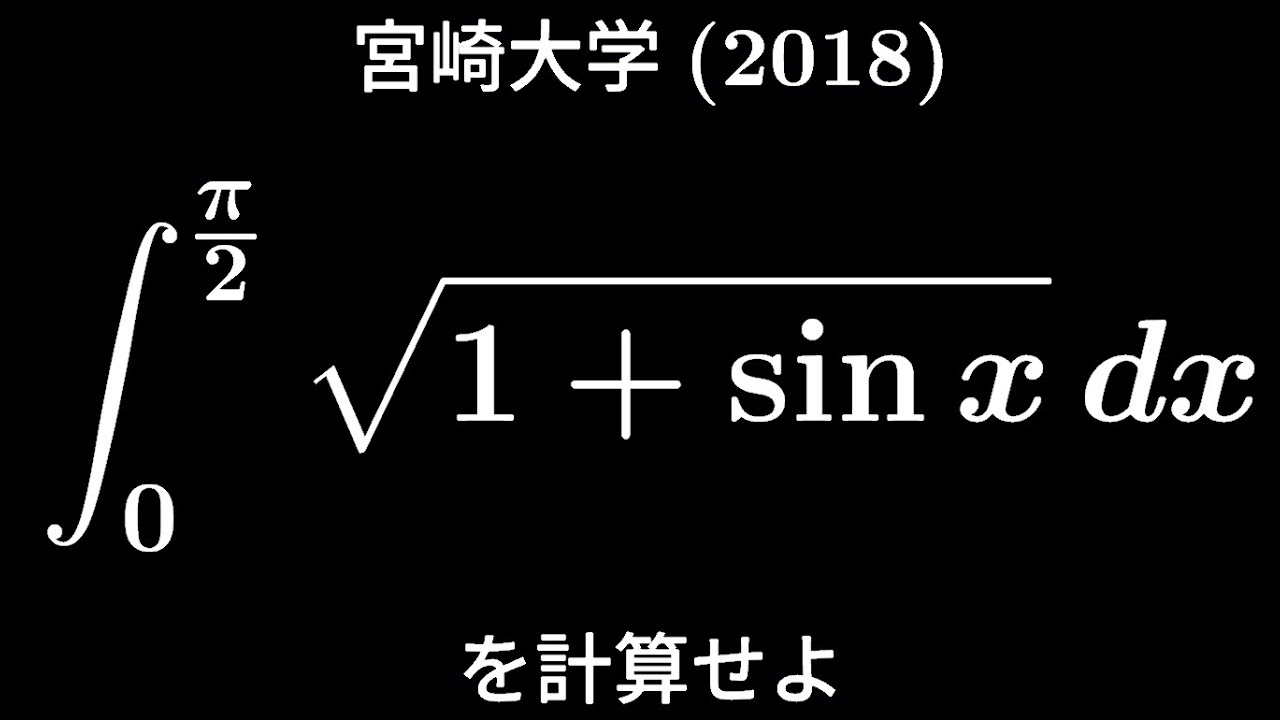
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#宮崎大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2018年宮崎大学 入試問題
この動画を見る
出典:2018年宮崎大学 入試問題
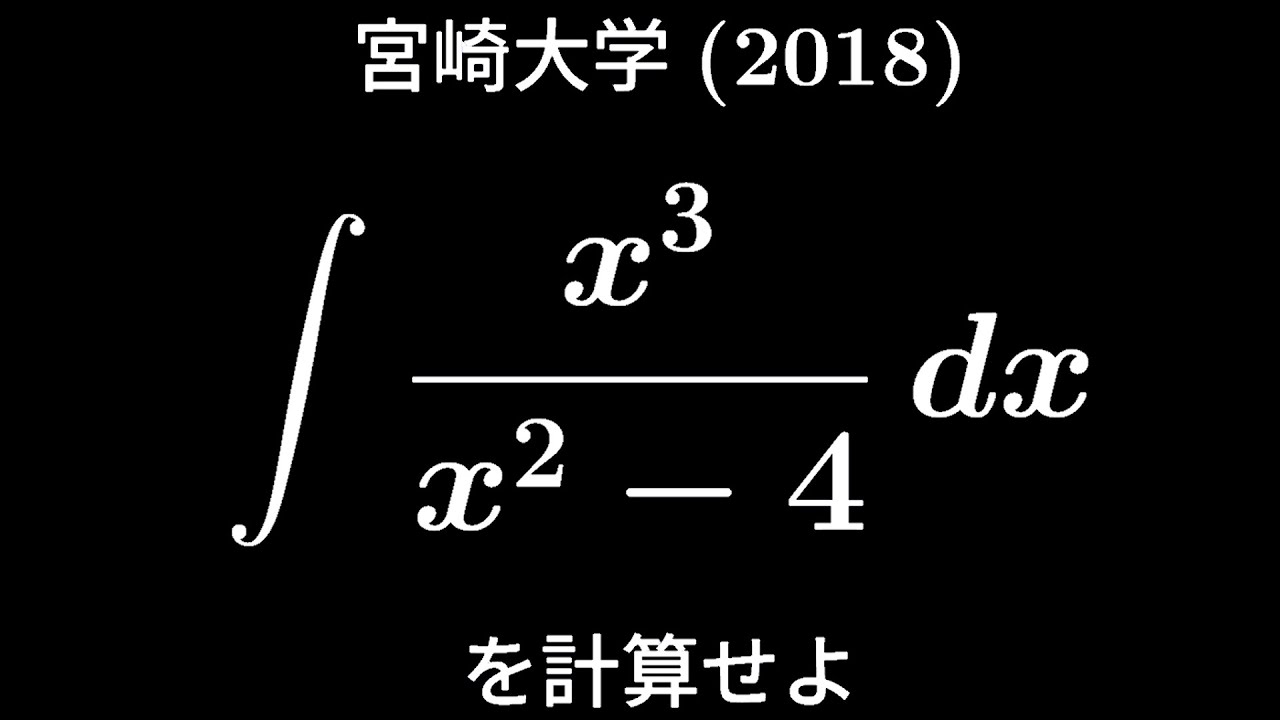
大学入試問題#210 宮崎大学(2018) 不定積分
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#宮崎大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2018年宮崎大学 入試問題
この動画を見る
出典:2018年宮崎大学 入試問題
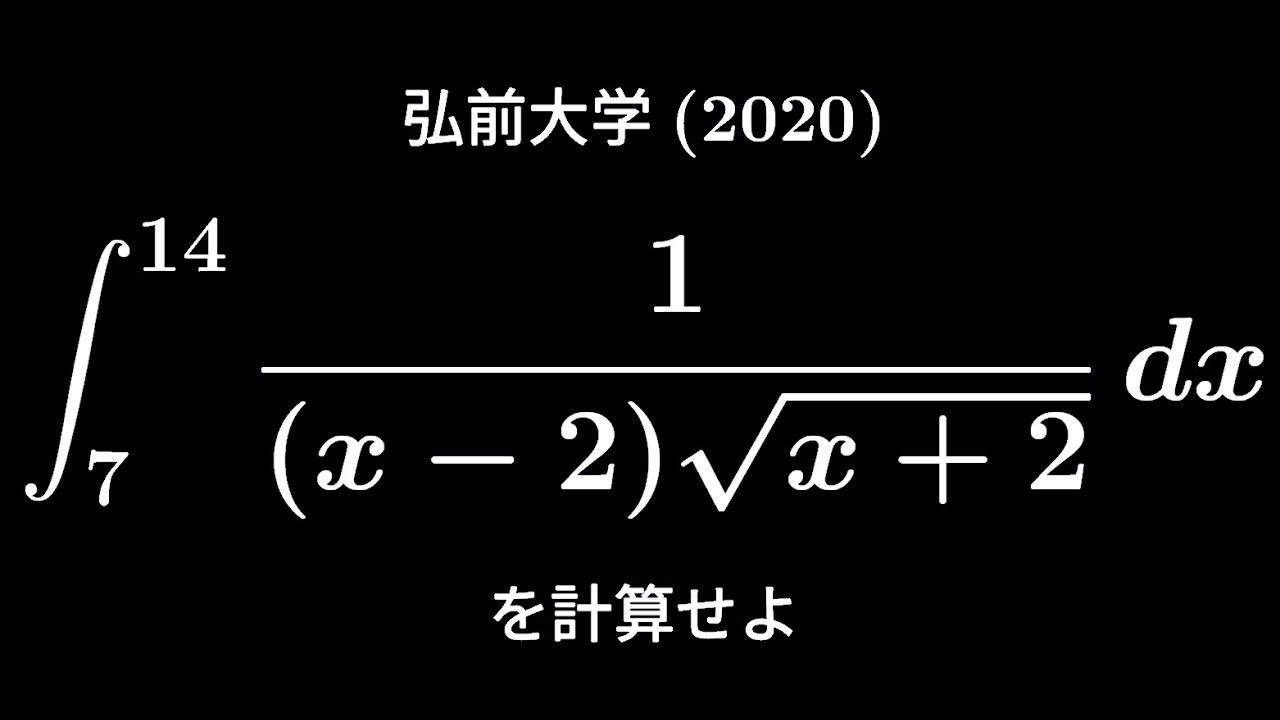
大学入試問題#209 弘前大学(2020) 定積分
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#弘前大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2020年広前大学 入試問題
この動画を見る
出典:2020年広前大学 入試問題
大学入試問題#208 信州大学(2021) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2021年信州大学 入試問題
この動画を見る
出典:2021年信州大学 入試問題
大学入試問題#207 埼玉大学(2006) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#埼玉大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2006年埼玉大学 入試問題
この動画を見る
出典:2006年埼玉大学 入試問題
大学入試問題#206 東京農工大学(2020) 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東京農工大学
指導講師:
ますただ
問題文全文(内容文):
出典:2020年東京農業工業大学 入試問題
この動画を見る
出典:2020年東京農業工業大学 入試問題
【誘導:概要欄】大学入試問題#205 大阪教育大学(2022) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#大阪教育大学
指導講師:
ますただ
問題文全文(内容文):
(1)
(2)
出典:2022年大阪教育大学 入試問題
この動画を見る
(1)
(2)
出典:2022年大阪教育大学 入試問題
大学入試問題#204 東京大学(改) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:東京大学 入試問題
この動画を見る
出典:東京大学 入試問題
大学入試問題#203 琉球大学(2020) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#琉球大学#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2020年琉球大学 入試問題
この動画を見る
出典:2020年琉球大学 入試問題
大学入試問題#202 横浜国立大学 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:横浜国立大学 入試問題
この動画を見る
出典:横浜国立大学 入試問題
大学入試問題#201 山梨大学(2021) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#山梨大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2021年山梨大学 入試問題
この動画を見る
出典:2021年山梨大学 入試問題
大学入試問題#200 大阪教育大学2022 定積分 King property

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#大阪教育大学
指導講師:
ますただ
問題文全文(内容文):
出典:2022年大阪教育大学 入試問題
この動画を見る
出典:2022年大阪教育大学 入試問題
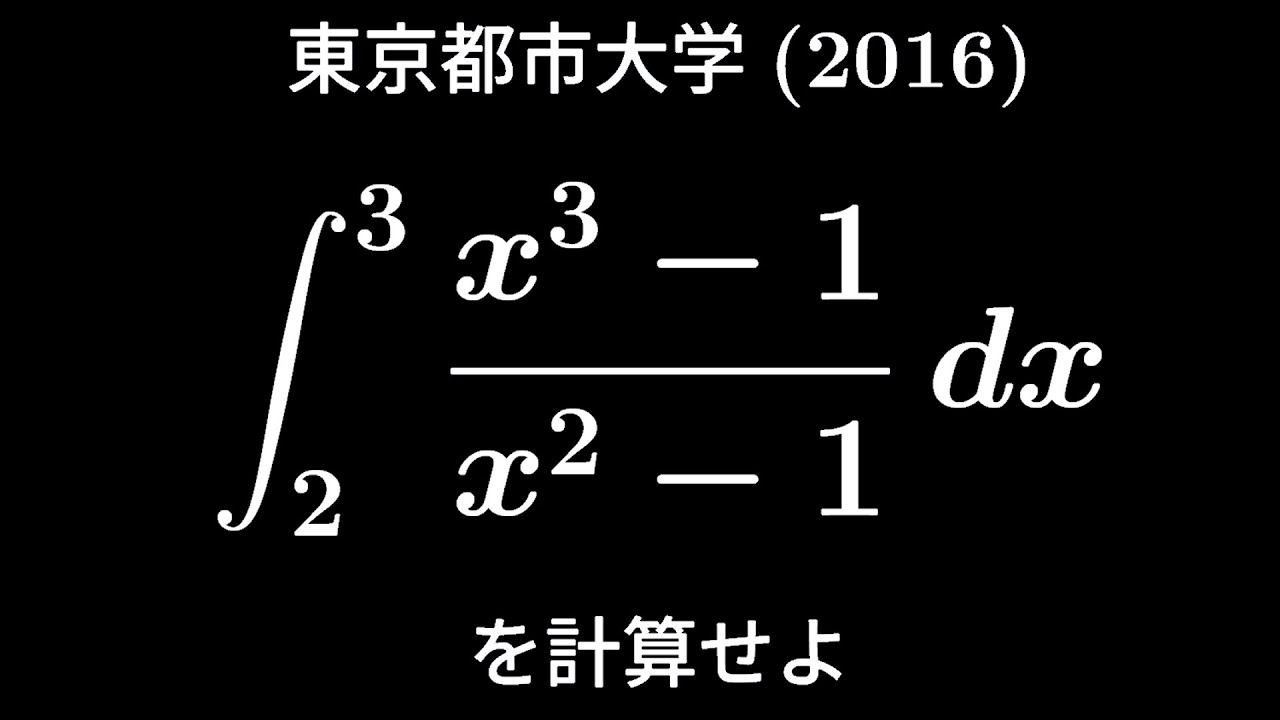
大学入試問題#199 東京都市大学(2016) 定積分
ハルハル様の作成問題② 複雑な方程式の解

大学入試問題#198 東京理科大学2010 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#東京理科大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2010年東京理科大学 入試問題
この動画を見る
出典:2010年東京理科大学 入試問題
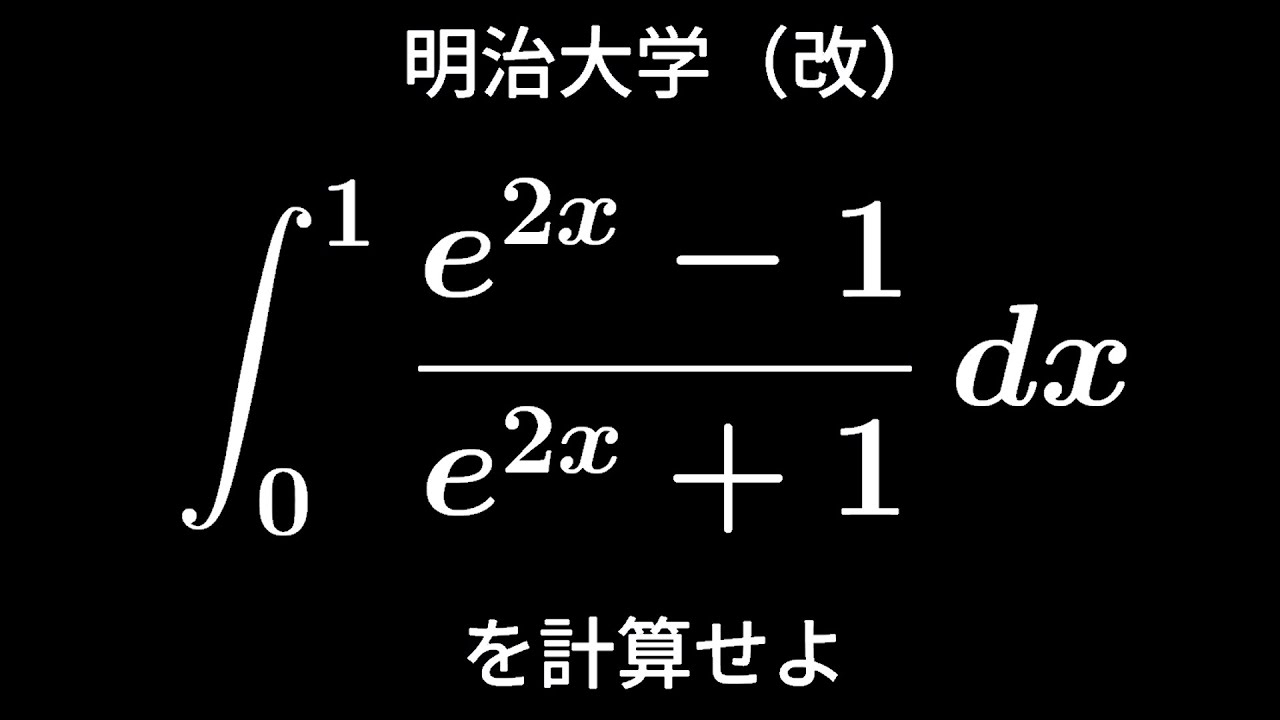
大学入試問題#197 明治大学(改) 定積分
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#明治大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:明治大学 入試問題
この動画を見る
出典:明治大学 入試問題
大学入試問題#196 神戸薬科大学2020 定積分

大学入試問題#195 兵庫医科大学 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#兵庫医科大学
指導講師:
ますただ
問題文全文(内容文):
出典:兵庫医科大学 入試問題
この動画を見る
出典:兵庫医科大学 入試問題
大学入試問題#194 横浜国立大学 不定積分

単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:横浜国立大学 入試問題
この動画を見る
出典:横浜国立大学 入試問題
大学入試問題#193 東海大学医学部 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#東海大学
指導講師:
ますただ
問題文全文(内容文):
出典:東海大学医学部 入試問題
この動画を見る
出典:東海大学医学部 入試問題
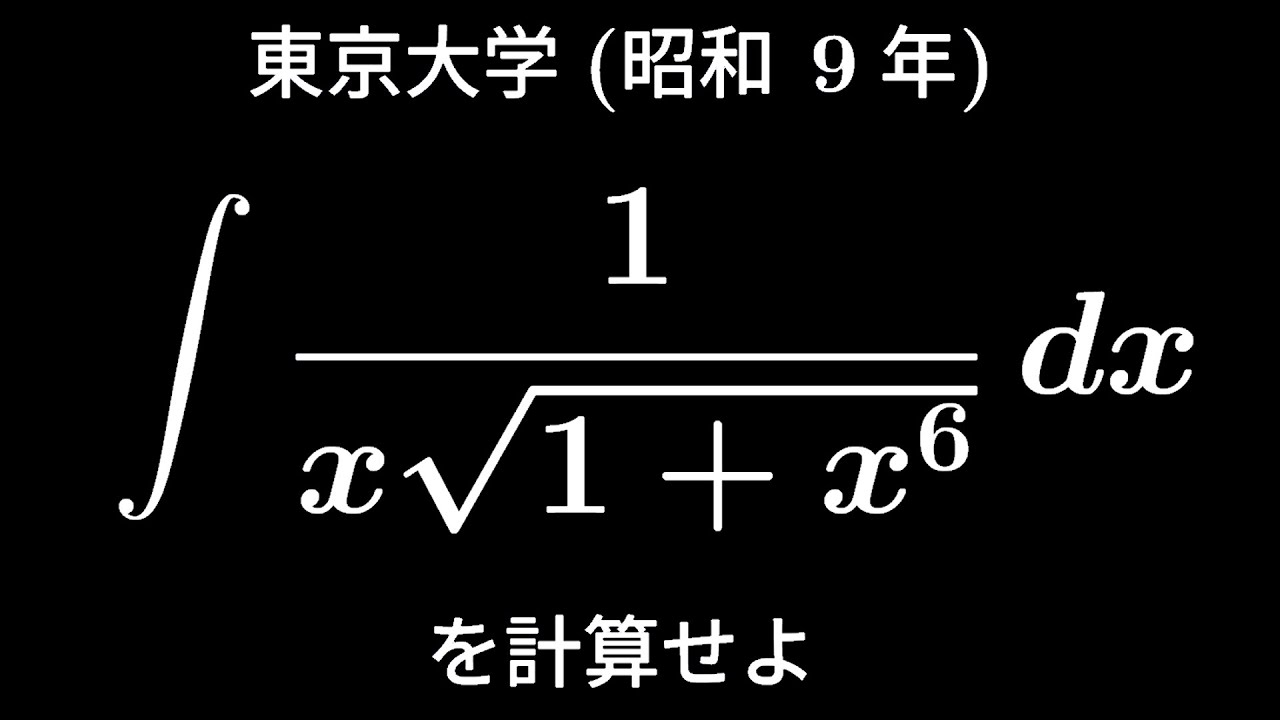
大学入試問題#192 東京大学(昭和9年) 不定積分 3手1組の好手順
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#東京大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:昭和9年東京大学 入試問題
この動画を見る
出典:昭和9年東京大学 入試問題
大学入試問題#191 岡山県立大学(2013) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#岡山県立大学
指導講師:
ますただ
問題文全文(内容文):
出典:2013年岡山県立大学 入試問題
この動画を見る
出典:2013年岡山県立大学 入試問題
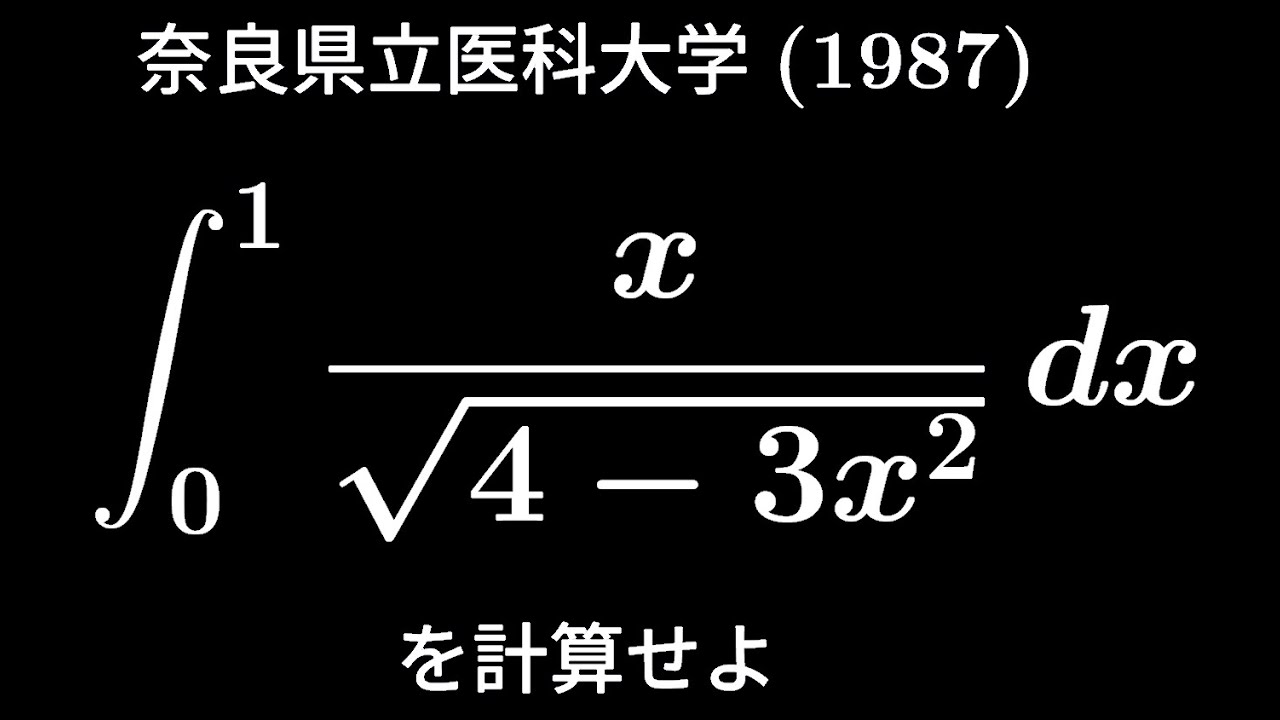
大学入試問題#190 奈良県立医科大学(1987) 定積分
単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#数学(高校生)#数Ⅲ#奈良県立医科大学
指導講師:
ますただ
問題文全文(内容文):
出典:1987年奈良県立医科大学 入試問題
この動画を見る
出典:1987年奈良県立医科大学 入試問題
大学入試問題#189 早稲田大学(2005) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2005年早稲田大学 入試問題
この動画を見る
出典:2005年早稲田大学 入試問題
大学入試問題#188 会津大学(2021) 定積分

大学入試問題#187 慶應義塾大学(2006) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2006年慶應義塾大学 入試問題
この動画を見る
出典:2006年慶應義塾大学 入試問題
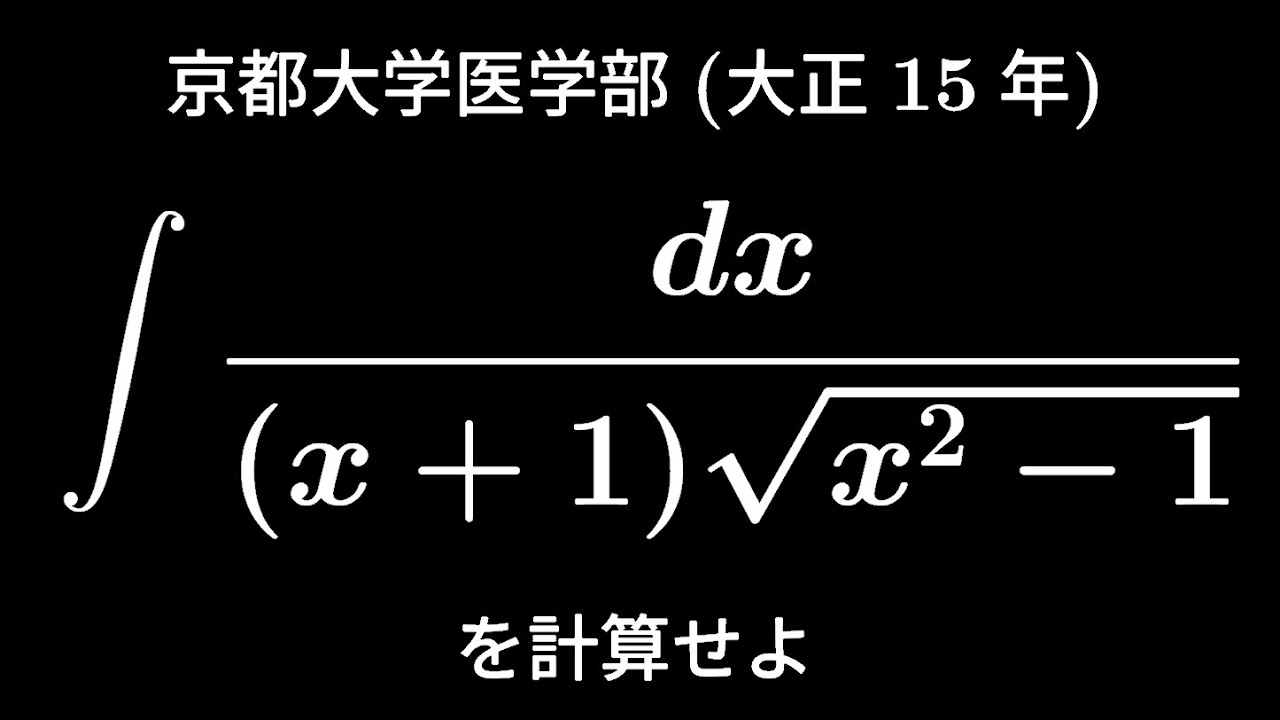
大学入試問題#186 京都大学医学部(大正15年) 不定積分 たぶん難問
単元:
#大学入試過去問(数学)#積分とその応用#不定積分#学校別大学入試過去問解説(数学)#京都大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:大正15年京都大学医学部 入試問題
この動画を見る
出典:大正15年京都大学医学部 入試問題
大学入試問題#185 大阪府立大学(2010) 定積分

単元:
#大学入試過去問(数学)#積分とその応用#定積分#学校別大学入試過去問解説(数学)#大阪府立大学#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
出典:2010年大阪府立大学 入試問題
この動画を見る
出典:2010年大阪府立大学 入試問題



