ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
大学入試問題をベースに数学の動画を作成しています。大学過去問を多数解説しています。
頻繁に更新していますので是非ご視聴ください！
職業：数学者
職歴：高校非常勤（院生時代）、高専、大学 准教授
趣味：
①将棋 棋力は将棋ウォーズ４段、将棋倶楽部２４はR２０００前後（小学校５，６年のころに頑張ってました）
②麻雀 アカウントは消えましたが、無課金で天鵬で７段まで上がりました。
③ソフトテニス 中学から大学まで、１０年間部活でやっていました。大学時代は４年になってもリーグにでていました。
【誘導は概要欄のリンクから】大学入試問題#294 東北大学工学部AO II期(2021) #整数問題
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#東北大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
等差数列
$a,a+1,a+2,・・・,a+n$の和が1000となるような自然数の組$(a,n)$をすべて求めよ。
出典：2021年東北大学工学部AOⅡ期 入試問題
この動画を見る
等差数列
$a,a+1,a+2,・・・,a+n$の和が1000となるような自然数の組$(a,n)$をすべて求めよ。
出典：2021年東北大学工学部AOⅡ期 入試問題
大学入試問題#293 横浜国立大学後期(2010) #定積分 #King property
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\pi}\displaystyle \frac{x\ \sin^3x}{\sin^2x+8}dx$
出典：2010年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\pi}\displaystyle \frac{x\ \sin^3x}{\sin^2x+8}dx$
出典：2010年横浜国立大学 入試問題
AkiyaMathさんと学ぶ積分計算Level 3
単元：
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-\frac{1}{2}}^{\frac{2}{3}}\displaystyle \frac{dx}{\sqrt[ 3 ]{ x^3-3x+2 }}$
この動画を見る
$\displaystyle \int_{-\frac{1}{2}}^{\frac{2}{3}}\displaystyle \frac{dx}{\sqrt[ 3 ]{ x^3-3x+2 }}$
大学入試問題#292 山梨大学医学部後期(2010) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#山梨大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\sqrt{ \frac{\pi}{2} }}x^3\sin(x^2)dx$
出典：2010年山梨大学医学部 入試問題
この動画を見る
$\displaystyle \int_{0}^{\sqrt{ \frac{\pi}{2} }}x^3\sin(x^2)dx$
出典：2010年山梨大学医学部 入試問題
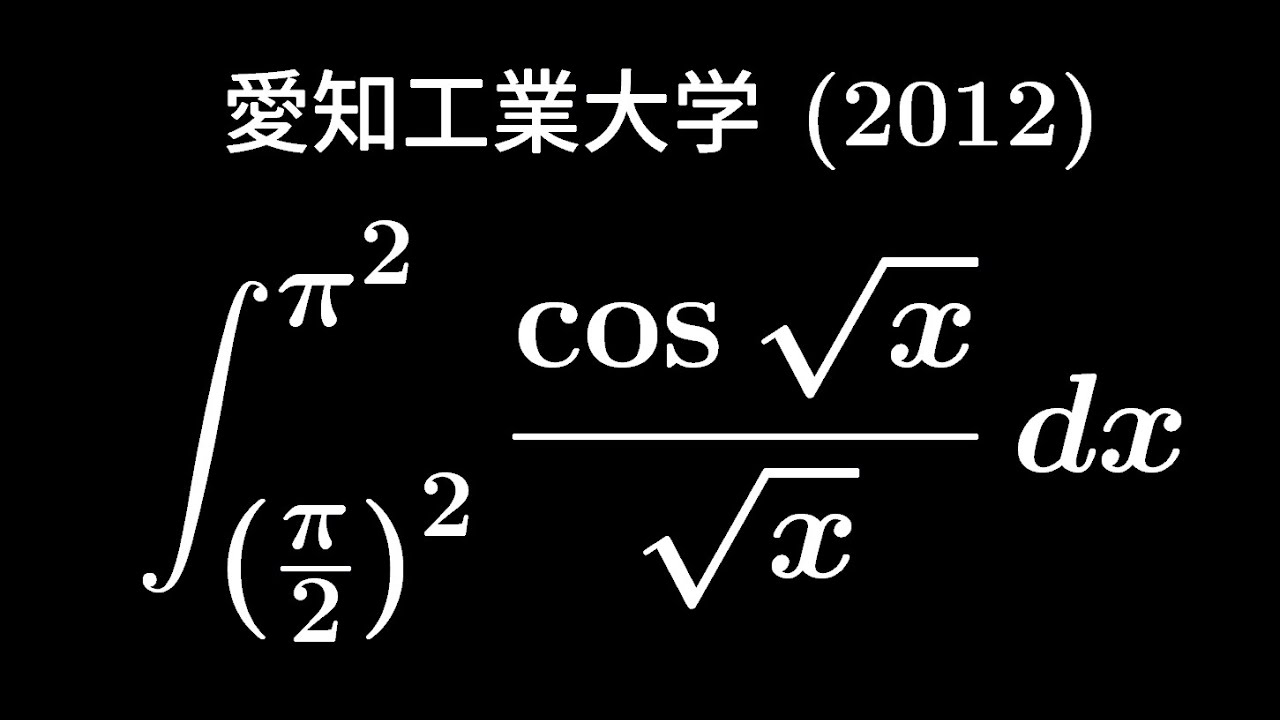
大学入試問題#291 愛知工業大学(2012) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#愛知工業大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{(\frac{\pi}{2})^2}^{\pi^2}\displaystyle \frac{\cos\sqrt{ x }}{\sqrt{ x }}dx$
出典：2012年愛知工業大学 入試問題
この動画を見る
$\displaystyle \int_{(\frac{\pi}{2})^2}^{\pi^2}\displaystyle \frac{\cos\sqrt{ x }}{\sqrt{ x }}dx$
出典：2012年愛知工業大学 入試問題
大学入試問題#290 広島市立大学2010 #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#広島市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\pi}\displaystyle \frac{\sin\ x}{\sqrt{ 5+4\cos\ x }}dx$
出典：2010年広島市立大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\pi}\displaystyle \frac{\sin\ x}{\sqrt{ 5+4\cos\ x }}dx$
出典：2010年広島市立大学 入試問題
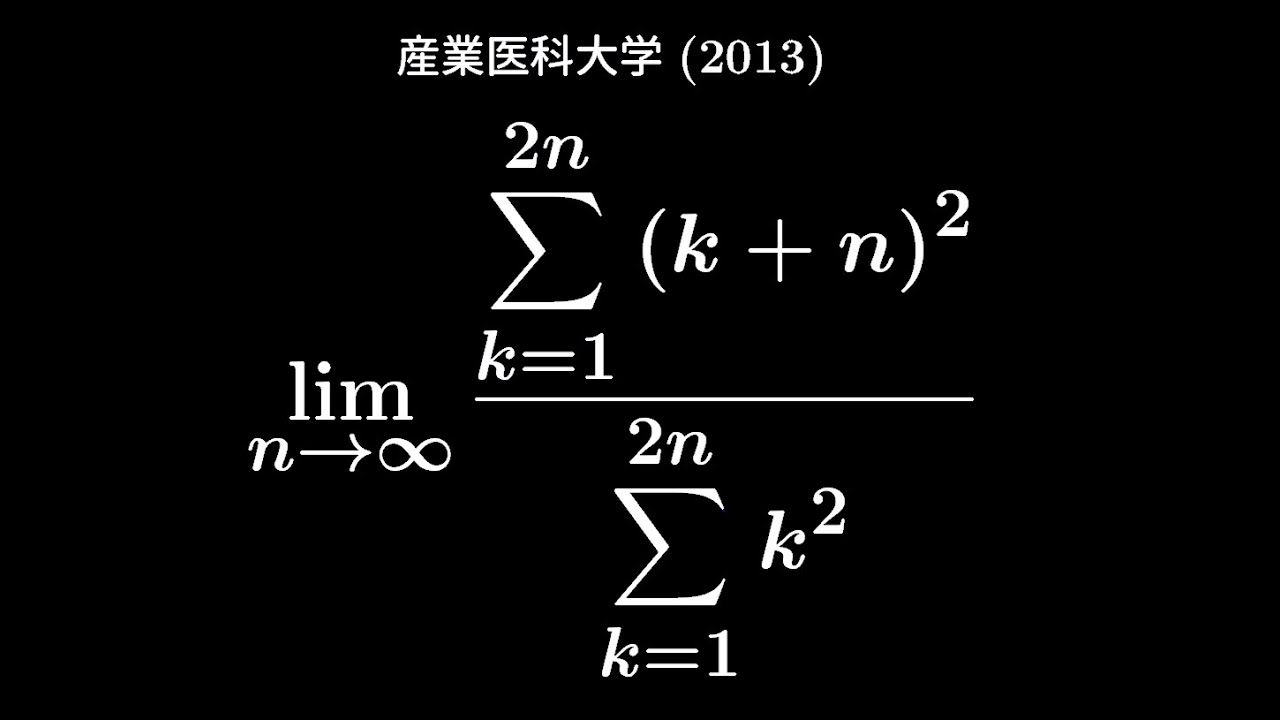
大学入試問題#290 産業医科大学(2013) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{d\theta}{\sqrt{ 2 }\cos(\theta-\displaystyle \frac{\pi}{4})}$
出典：2013年産業医科大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{2}}\displaystyle \frac{d\theta}{\sqrt{ 2 }\cos(\theta-\displaystyle \frac{\pi}{4})}$
出典：2013年産業医科大学 入試問題
大学入試問題#288 高知大学(2019) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#高知大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-\frac{7}{2}}^{\frac{9}{2}}\displaystyle \frac{2^x}{2^x+\sqrt{ 2 }}dx$
出典：2019年高知大学 入試問題
この動画を見る
$\displaystyle \int_{-\frac{7}{2}}^{\frac{9}{2}}\displaystyle \frac{2^x}{2^x+\sqrt{ 2 }}dx$
出典：2019年高知大学 入試問題
大学入試問題#287 同志社大学(2013) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#同志社大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{6}}\cos^6\theta\ d\ \theta$
出典：2013年同志社大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{6}}\cos^6\theta\ d\ \theta$
出典：2013年同志社大学 入試問題
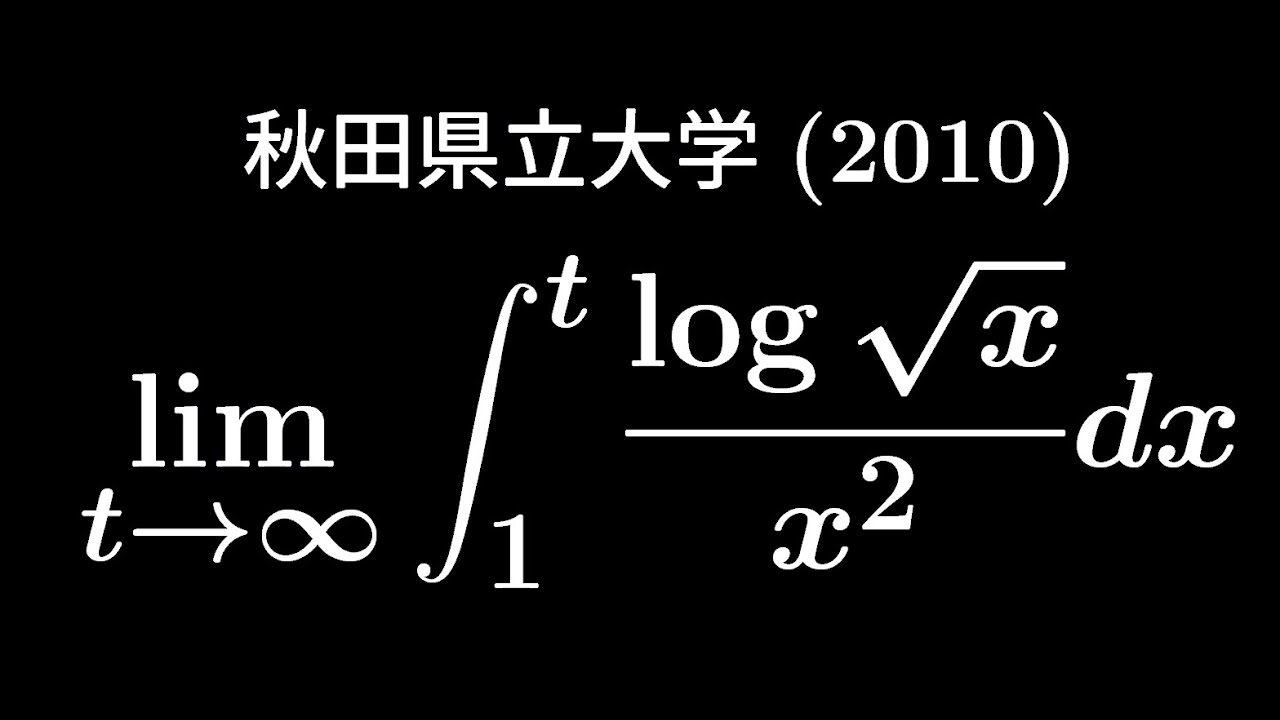
大学入試問題#286 秋田県立大学(2010) #定積分 #極限
単元：
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ t \to \infty }\displaystyle \int_{1}^{t}\displaystyle \frac{log\sqrt{ x }}{x^2}dx$
出典：2010年秋田県立大学 入試問題
この動画を見る
$\displaystyle \lim_{ t \to \infty }\displaystyle \int_{1}^{t}\displaystyle \frac{log\sqrt{ x }}{x^2}dx$
出典：2010年秋田県立大学 入試問題
大学入試問題#285 早稲田大学(2013) #解と係数の関係
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$k$：実数
直線$l:y-2=k(x-1)$
放物線$C:y=x^2$
で囲まれる図形の面積の最小値とそのときの$k$の値を求めよ。
出典：2013年早稲田大学 入試問題
この動画を見る
$k$：実数
直線$l:y-2=k(x-1)$
放物線$C:y=x^2$
で囲まれる図形の面積の最小値とそのときの$k$の値を求めよ。
出典：2013年早稲田大学 入試問題
大学入試問題#284 同志社大学(2013) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#同志社大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\frac{\pi}{6}}\cos^4\theta\ d\theta$
出典：2013年同志社大学 入試問題
この動画を見る
$\displaystyle \int_{0}^{\frac{\pi}{6}}\cos^4\theta\ d\theta$
出典：2013年同志社大学 入試問題
大学入試問題#283 早稲田大学(2013) #整数問題
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$5 \leqq p$：素数
$p^3$を$p-4$で割った余りが4のとき$p$の値を求めよ。
出典：2013年早稲田大学 入試問題
この動画を見る
$5 \leqq p$：素数
$p^3$を$p-4$で割った余りが4のとき$p$の値を求めよ。
出典：2013年早稲田大学 入試問題
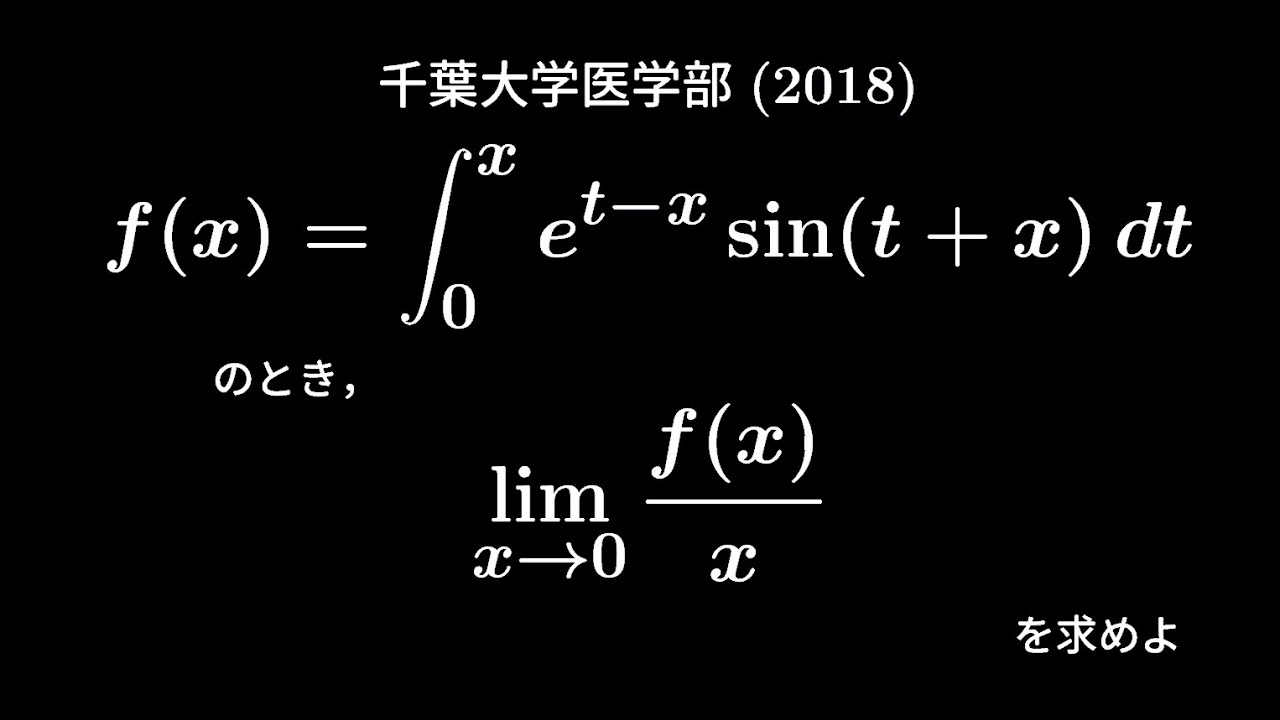
大学入試問題#282 千葉大学医学部(2018) #定積分 #極限
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#千葉大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$f(x)=\displaystyle \int_{0}^{x}e^{t-x}\sin(t+x)dt$のとき
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x}$を求めよ。
出典：2018年千葉大学医学部 入試問題
この動画を見る
$f(x)=\displaystyle \int_{0}^{x}e^{t-x}\sin(t+x)dt$のとき
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x}$を求めよ。
出典：2018年千葉大学医学部 入試問題
大学入試問題#255 早稲田大学(2011) #整数問題
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#早稲田大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$a,b$：整数
$2a+3b=42$をみたすとき$ab$の最大値を求めよ。
出典：2011年早稲田大学 入試問題
この動画を見る
$a,b$：整数
$2a+3b=42$をみたすとき$ab$の最大値を求めよ。
出典：2011年早稲田大学 入試問題
大学入試問題#281 首都大学東京(2019) #整数問題 #完全数
単元：
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師：
ますただ
問題文全文(内容文)：
$2 \leqq m$：自然数
$2^m-1$：素数
$2^{m-1}(2^m-1)$：完全数
出典：2019年首都大学東京 入試問題
この動画を見る
$2 \leqq m$：自然数
$2^m-1$：素数
$2^{m-1}(2^m-1)$：完全数
出典：2019年首都大学東京 入試問題
ハルハル様の作成問題④ #整数問題 【難】
単元：
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師：
ますただ
問題文全文(内容文)：
$(x^3+1)^3+(x^2+1)^2=2^y$を満たす整数の組$(x,y)$をすべて求めよ。
この動画を見る
$(x^3+1)^3+(x^2+1)^2=2^y$を満たす整数の組$(x,y)$をすべて求めよ。
大学入試問題#279 電気通信大学(2012) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#電気通信大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{0}^{\cos\frac{\pi}{7}}\displaystyle \frac{dx}{\sqrt{ 1-x^2 }}$
出典：2012年電気通信大学後期 入試問題
この動画を見る
$\displaystyle \int_{0}^{\cos\frac{\pi}{7}}\displaystyle \frac{dx}{\sqrt{ 1-x^2 }}$
出典：2012年電気通信大学後期 入試問題
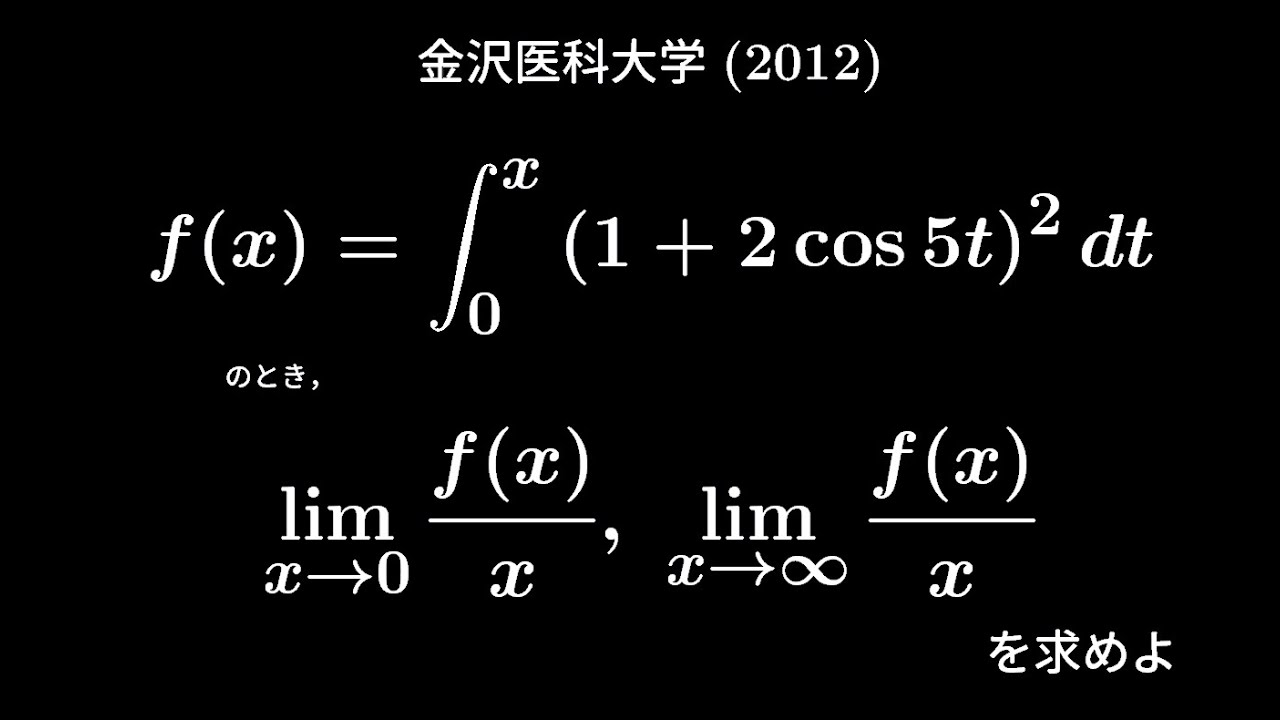
大学入試問題#278 金沢医科大学(2012) #定積分 #極限
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#金沢医科大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$f(x)=\displaystyle \int_{0}^{x}(1+2\cos5t)^2dt$のとき
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x},\displaystyle \lim_{ x \to \infty }\displaystyle \frac{f(x)}{x}$を求めよ。
出典：2012年金沢医科大学 入試問題
この動画を見る
$f(x)=\displaystyle \int_{0}^{x}(1+2\cos5t)^2dt$のとき
$\displaystyle \lim_{ x \to 0 }\displaystyle \frac{f(x)}{x},\displaystyle \lim_{ x \to \infty }\displaystyle \frac{f(x)}{x}$を求めよ。
出典：2012年金沢医科大学 入試問題
大学入試問題#277 横浜国立大学後期(2012) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int_{-1}^{1}(1-x^2)e^{-2x}dx$を求めよ
出典：2010年横浜国立大学 入試問題
この動画を見る
$\displaystyle \int_{-1}^{1}(1-x^2)e^{-2x}dx$を求めよ
出典：2010年横浜国立大学 入試問題
大学入試問題#276 信州大学医学部後期 改 (2012) #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#信州大学
指導講師：
ますただ
問題文全文(内容文)：
$0 \lt a \lt 1$
$\displaystyle \int_{0}^{\sqrt{ 1-a^2 }}x\ log(x^2+a^2)dx$
出典：2012年信州大学医学部後期 入試問題
この動画を見る
$0 \lt a \lt 1$
$\displaystyle \int_{0}^{\sqrt{ 1-a^2 }}x\ log(x^2+a^2)dx$
出典：2012年信州大学医学部後期 入試問題
大学入試問題#275 産業医科大学(2013) #区分求積法
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#産業医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty }\displaystyle \frac{\displaystyle \sum_{k=1}^{2n} (k+n)^2}{\displaystyle \sum_{k=1}^{2n} k^2}$
出典：2013年産業医科大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty }\displaystyle \frac{\displaystyle \sum_{k=1}^{2n} (k+n)^2}{\displaystyle \sum_{k=1}^{2n} k^2}$
出典：2013年産業医科大学 入試問題
大学入試問題#274 横浜国立大学後期2012 #区分求積法 #極限
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#横浜国立大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty }\{\displaystyle \frac{(2n)!}{n!n^n}\}^{\frac{1}{n}}$を求めよ
出典：2010年横浜国立大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty }\{\displaystyle \frac{(2n)!}{n!n^n}\}^{\frac{1}{n}}$を求めよ
出典：2010年横浜国立大学 入試問題
大学入試問題#273 日本大学(2010) #微分 #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#日本大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
$0 \leqq x \leqq \pi$
$f(x)=e^{-\frac{3}{4}\sin^2x}\sin2x$
$x=\alpha$で$f(x)$は最大値をとる
（1）$\sin\alpha$の値
（2）$\displaystyle \int_{0}^{\alpha}f(x)dx$
出典：2013年日本大学 入試問題
この動画を見る
$0 \leqq x \leqq \pi$
$f(x)=e^{-\frac{3}{4}\sin^2x}\sin2x$
$x=\alpha$で$f(x)$は最大値をとる
（1）$\sin\alpha$の値
（2）$\displaystyle \int_{0}^{\alpha}f(x)dx$
出典：2013年日本大学 入試問題
大学入試問題#272 慶應義塾大学(2010) #y軸回転体 #定積分 #バームクーヘン積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#慶應義塾大学#数学(高校生)
指導講師：
ますただ
問題文全文(内容文)：
曲線$y=\displaystyle \frac{x}{\sqrt{ 1-x^2 }}$
$x$軸、$x=\displaystyle \frac{1}{2}$で囲まれた部分を$y$軸中心に回転した体積$V$を求めよ。
出典：2010年慶應義塾大学 入試問題
この動画を見る
曲線$y=\displaystyle \frac{x}{\sqrt{ 1-x^2 }}$
$x$軸、$x=\displaystyle \frac{1}{2}$で囲まれた部分を$y$軸中心に回転した体積$V$を求めよ。
出典：2010年慶應義塾大学 入試問題
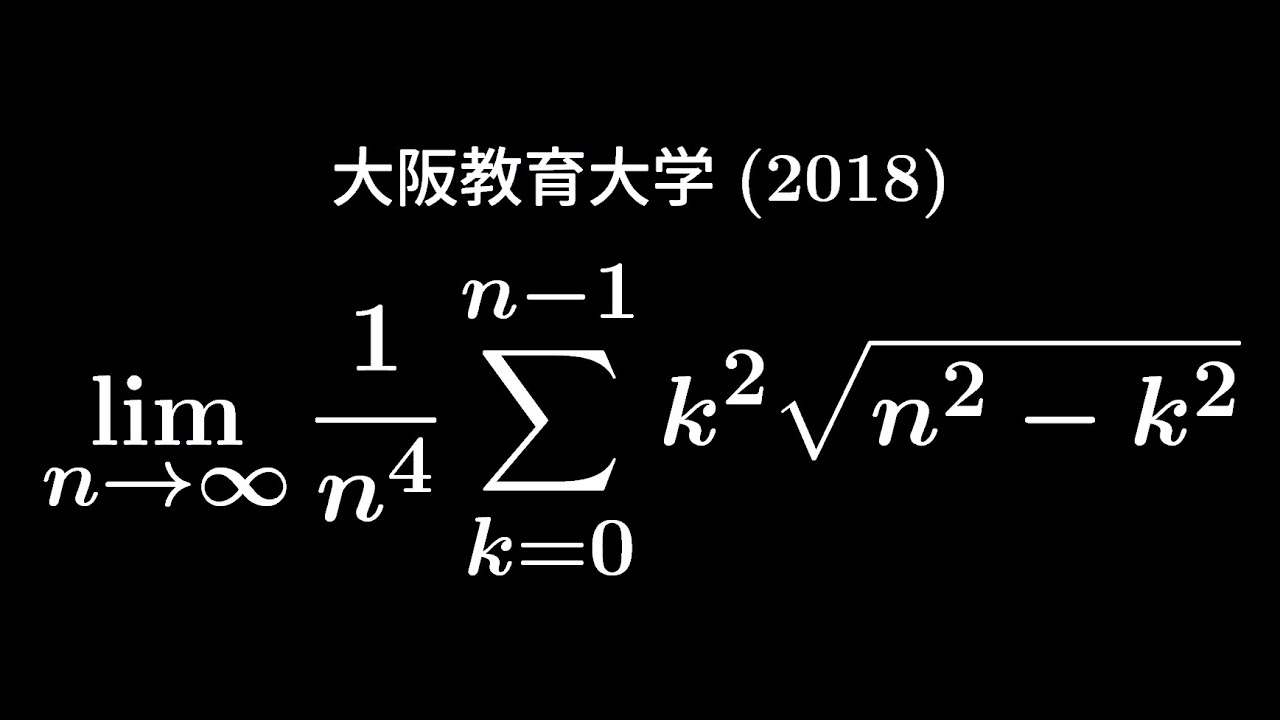
大学入試問題#271 大阪教育大学2018 #区分求積法 #ウォリス積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#大阪教育大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ n \to \infty }\displaystyle \frac{1}{n^4}\displaystyle \sum_{k=0}^{n-1}k^2 \sqrt{ n^2-k^2 }$を求めよ。
出典：2018年大阪教育大学 入試問題
この動画を見る
$\displaystyle \lim_{ n \to \infty }\displaystyle \frac{1}{n^4}\displaystyle \sum_{k=0}^{n-1}k^2 \sqrt{ n^2-k^2 }$を求めよ。
出典：2018年大阪教育大学 入試問題
大学入試問題#270 岡山県立大学(2010) #不定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#岡山県立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{x}{1+\cos\ 2x}\ dx$
出典：2010年岡山県立大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{x}{1+\cos\ 2x}\ dx$
出典：2010年岡山県立大学 入試問題
大学入試問題#269 横浜市立大学医学部(2010) #極限 #定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#横浜市立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \lim_{ R \to \infty }\displaystyle \int_{1}^{R^2}\displaystyle \frac{e^{-\sqrt{ x }}}{2}dx$
出典：2010年横浜市立大学 医学部 入試問題
この動画を見る
$\displaystyle \lim_{ R \to \infty }\displaystyle \int_{1}^{R^2}\displaystyle \frac{e^{-\sqrt{ x }}}{2}dx$
出典：2010年横浜市立大学 医学部 入試問題
大学入試問題 岡山県立大学2010 #不定積分
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#岡山県立大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{\sin\ x}{1+\cos\ 2x}dx$
出典：2010年岡山県立大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{\sin\ x}{1+\cos\ 2x}dx$
出典：2010年岡山県立大学 入試問題
大学入試問題#267 奈良県立医科大学 改 (2011) #不定積分 【難】
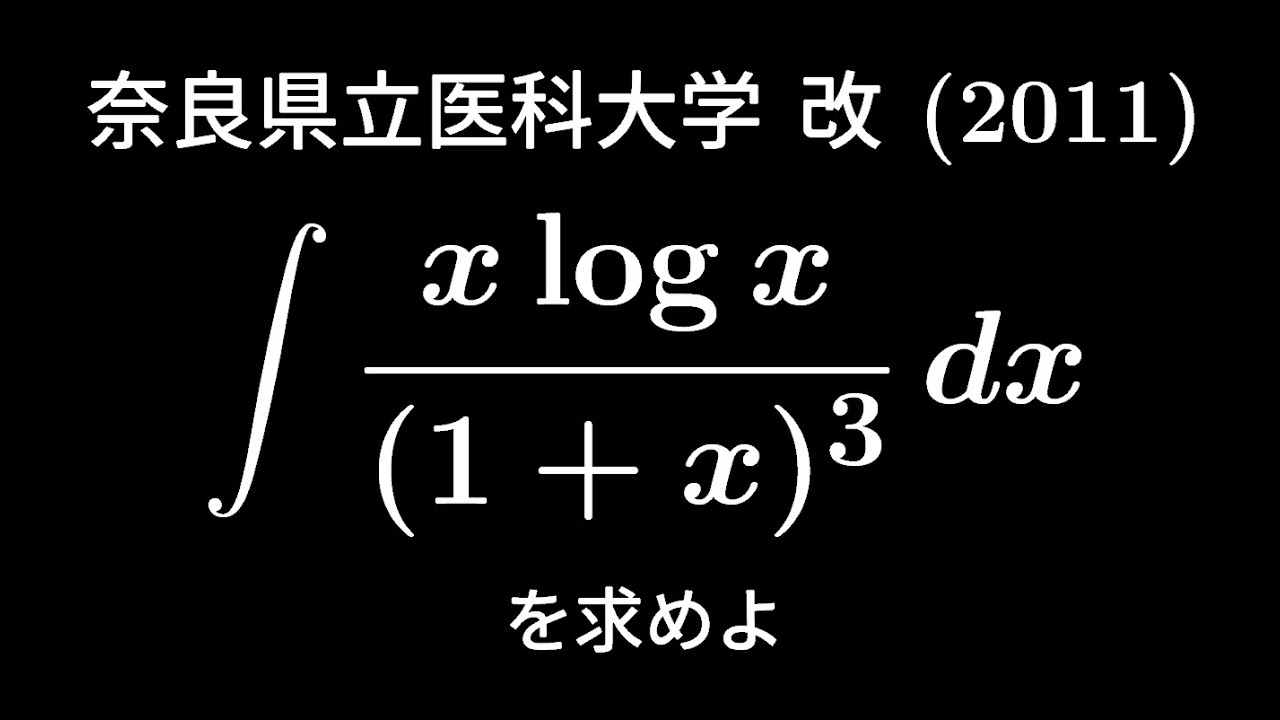
単元：
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#奈良県立医科大学
指導講師：
ますただ
問題文全文(内容文)：
$\displaystyle \int \displaystyle \frac{x\ log\ x}{(1+x)^3}dx$を計算せよ
出典：2011年奈良県立医科大学 入試問題
この動画を見る
$\displaystyle \int \displaystyle \frac{x\ log\ x}{(1+x)^3}dx$を計算せよ
出典：2011年奈良県立医科大学 入試問題