 ますただ
ますただ
 ますただ
ますただ
※下の画像部分をクリックすると、先生の紹介ページにリンクします。
重積分⑥-1【曲面・平面で囲まれた体積】(高専数学 微積II,数検1級1次解析対応)

単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#高専(高等専門学校)
指導講師:
ますただ
問題文全文(内容文):
曲面
この動画を見る
曲面
10京都府教員採用試験(数学:2番 極限値)
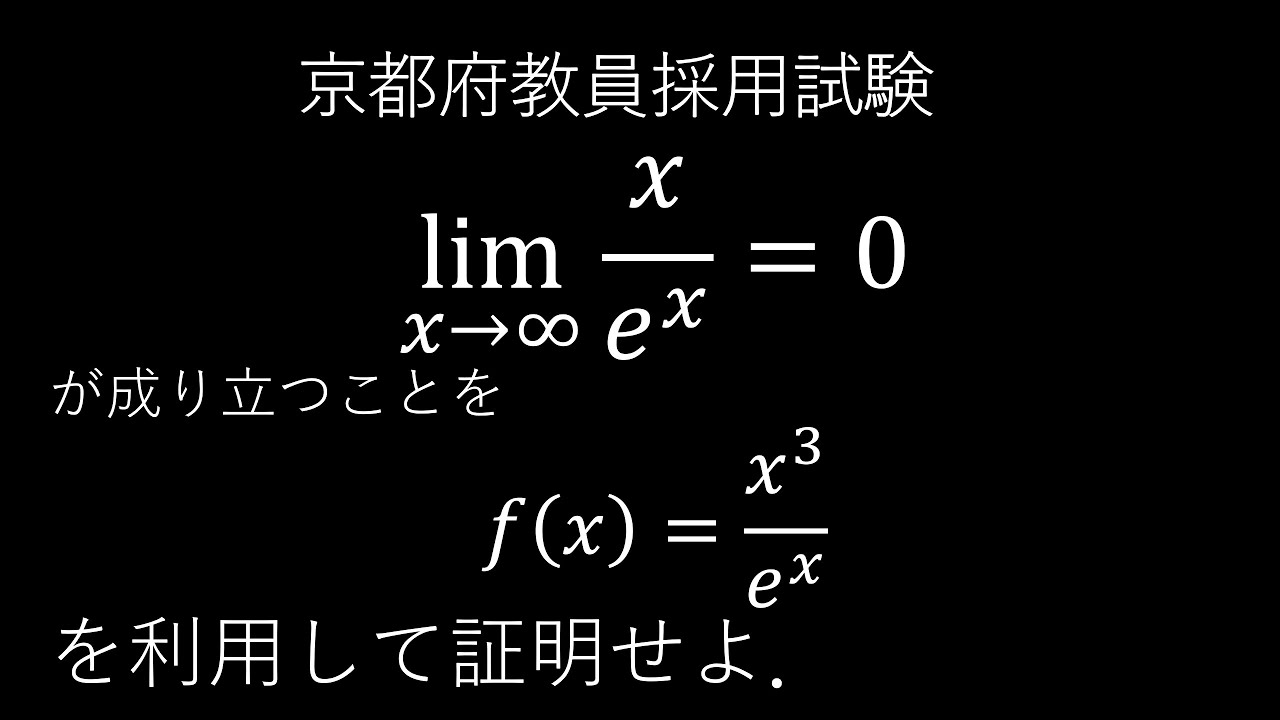
重積分⑤【積分順序の変更(応用)】(高専数学 微積II,数検1級1次対応)
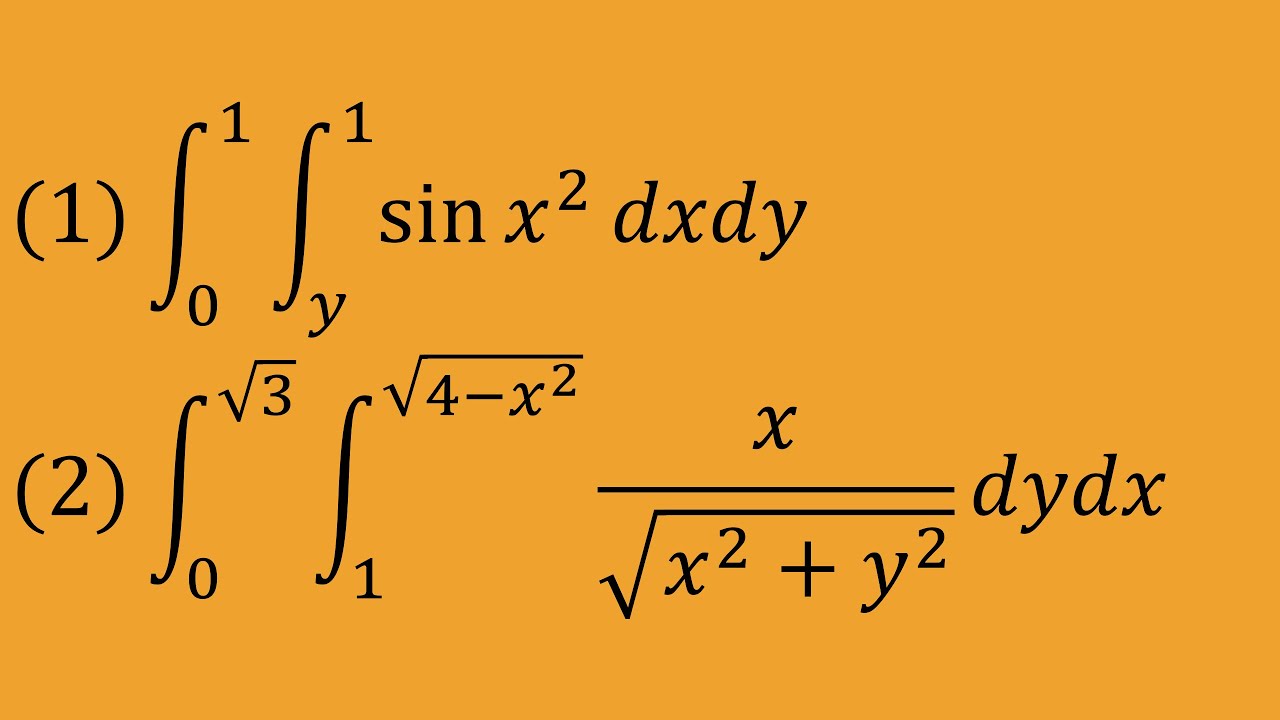
17愛知県教員採用試験(数学:10-(2) x軸回転体)

重積分④【積分順序の変更】(高専数学 微積II,数学検定1級解析)
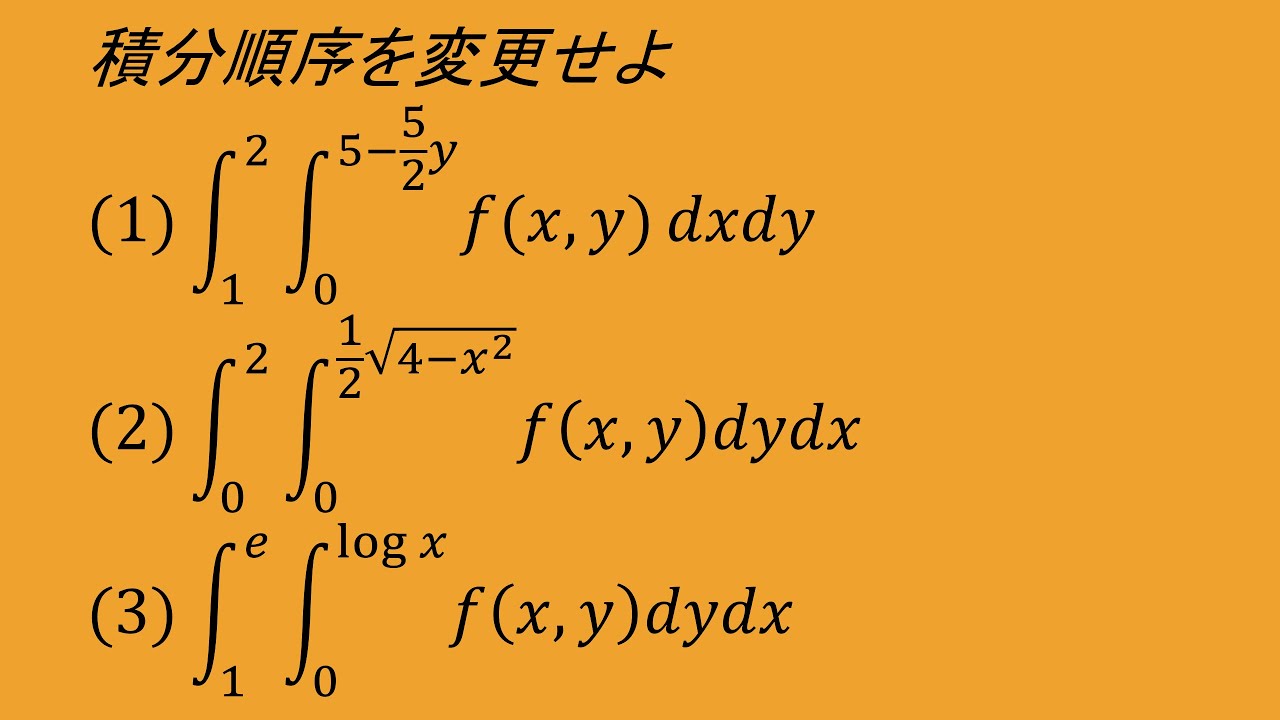
単元:
#大学入試過去問(数学)#学校別大学入試過去問解説(数学)#数学(高校生)#高専(高等専門学校)
指導講師:
ますただ
問題文全文(内容文):
積分順序の変更
(1)
(2)
(3)
この動画を見る
積分順序の変更
(1)
(2)
(3)
10東京都教員採用試験(数学:1-(1) 解と係数の関係)

単元:
#数Ⅱ#複素数と方程式#解と判別式・解と係数の関係#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):

この動画を見る

重積分③【積分領域の工夫】(高専数学 微積II,数学検定1級解析)
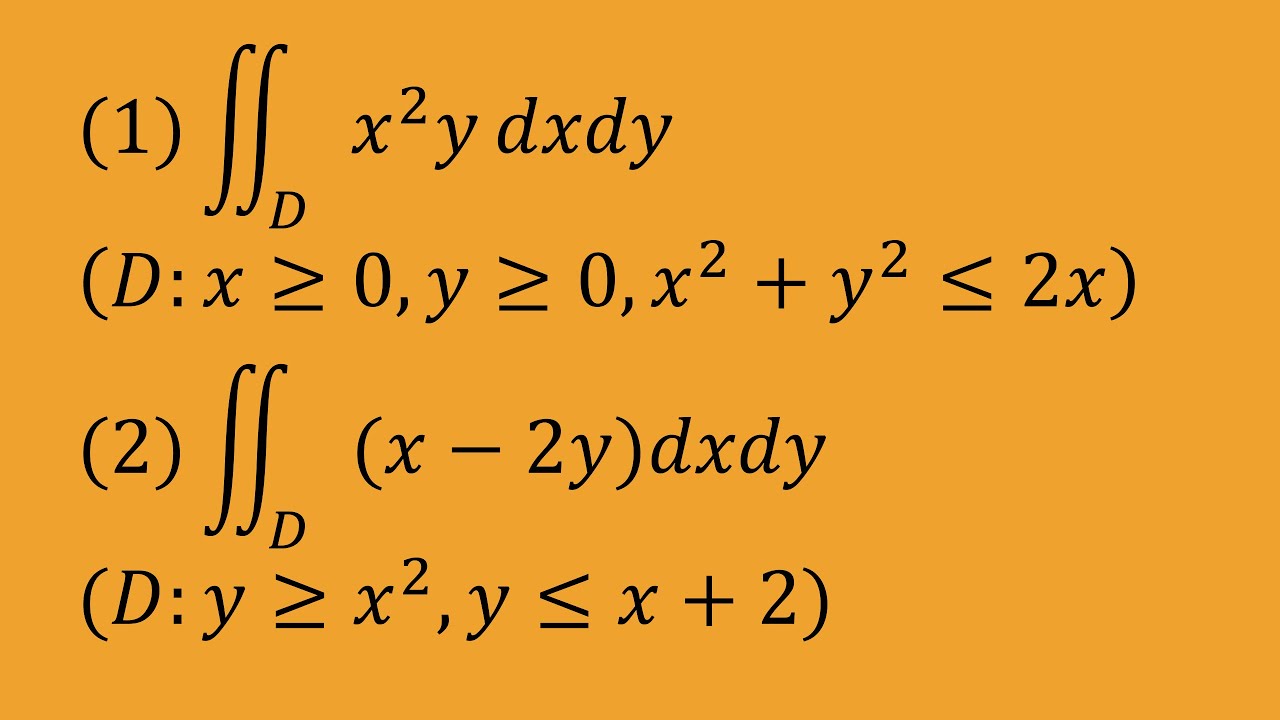
重積分②(高専数学 微積II,数学検定1級解析)
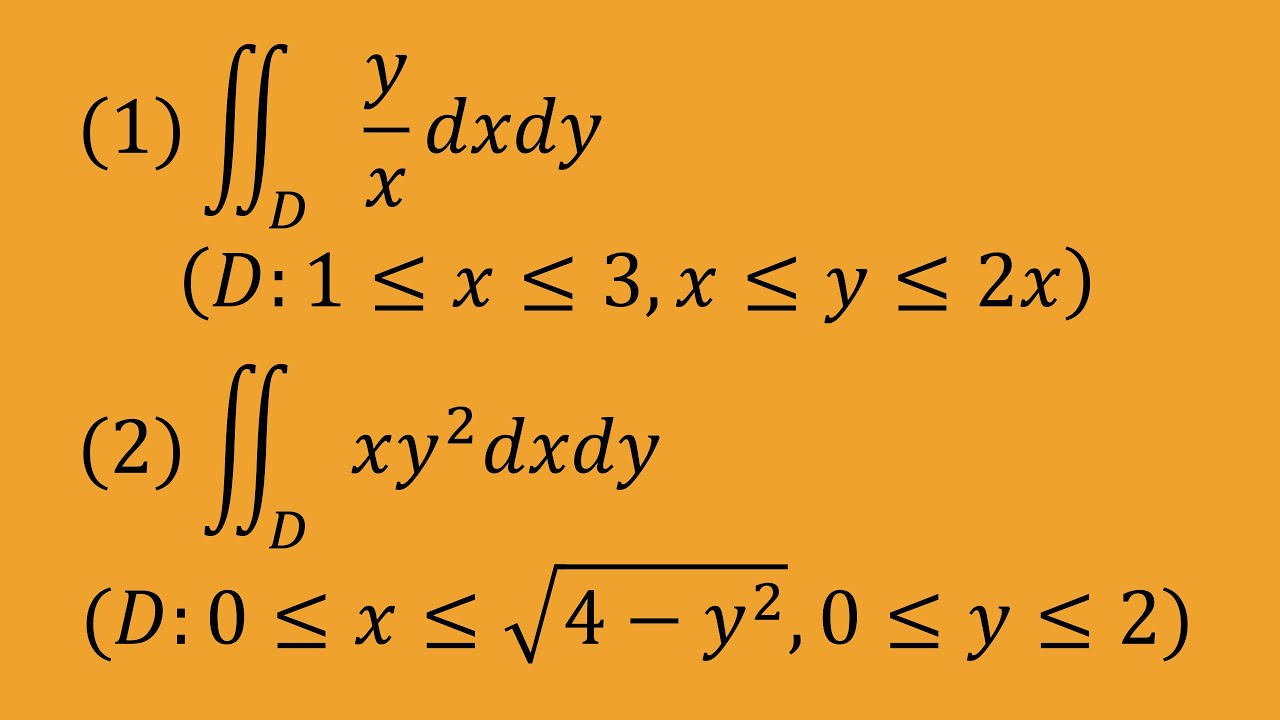
単元:
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師:
ますただ
問題文全文(内容文):
重積分(累次積分)
ex1
この動画を見る
重積分(累次積分)
ex1
重積分①(高専数学 微積II,数学検定1級解析)
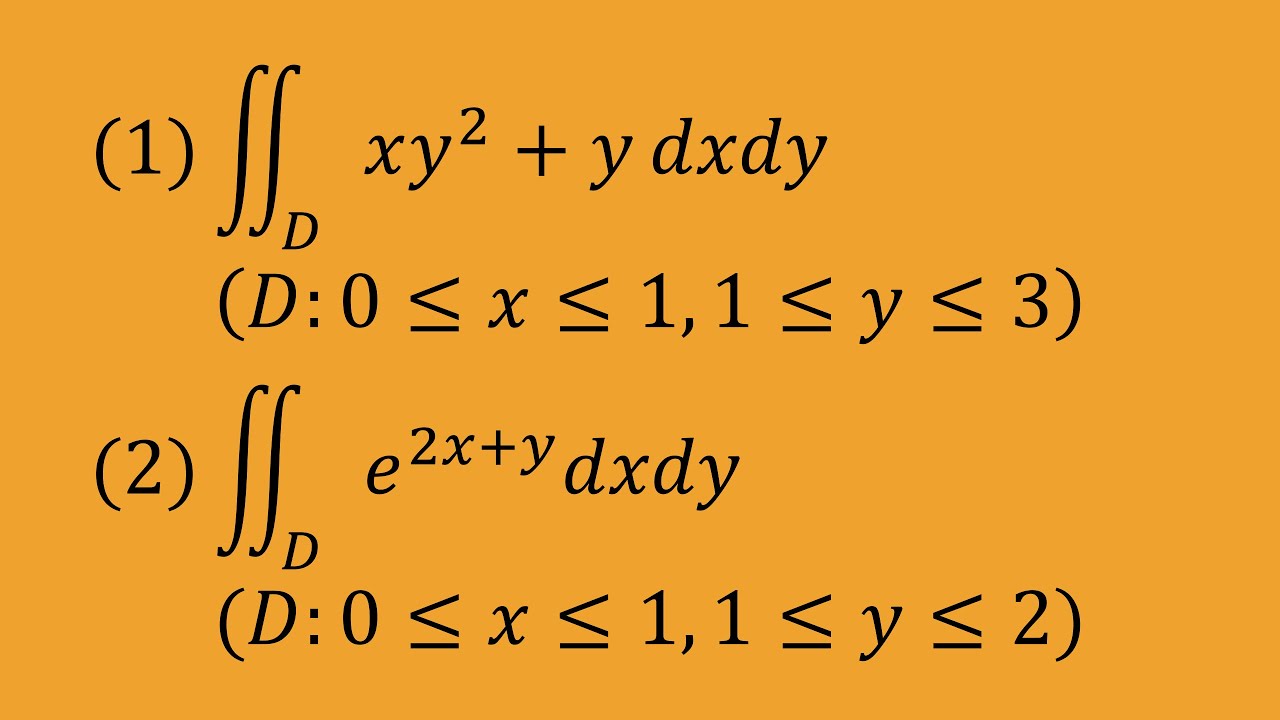
単元:
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師:
ますただ
問題文全文(内容文):
重積分(累次積分)
ex1
この動画を見る
重積分(累次積分)
ex1
14京都府教員採用試験(数学:5番 三角関数)

08東京都教員採用試験(数学:1-(1) 相加平均・相乗平均)

単元:
#数Ⅱ#式と証明#恒等式・等式・不等式の証明#数学(高校生)
指導講師:
ますただ
問題文全文(内容文):
 (1) x,y,zは正の数
(1) x,y,zは正の数
x+2y+4z=2のとき
xyzの最大値を求めよ。
この動画を見る
 (1) x,y,zは正の数
(1) x,y,zは正の数x+2y+4z=2のとき
xyzの最大値を求めよ。
14奈良県教員採用試験(数学:2-(6) y軸回転体・バームクーヘン積分 )

単元:
#積分とその応用#面積・体積・長さ・速度#その他#数学(高校生)#数Ⅲ#教員採用試験
指導講師:
ますただ
問題文全文(内容文):
 -(6)
-(6)
この動画を見る
 -(6)
-(6)16愛知県教員採用試験(数学:5番 対数,相加平均・相乗平均)

11神奈川県教員採用試験(数学:11番 重積分)

18奈良県教員採用試験(数学:1番 ベクトル)
単元:
#平面上のベクトル#平面上のベクトルと内積#ベクトルと平面図形、ベクトル方程式#その他#数学(高校生)#数C#教員採用試験
指導講師:
ますただ
問題文全文(内容文):
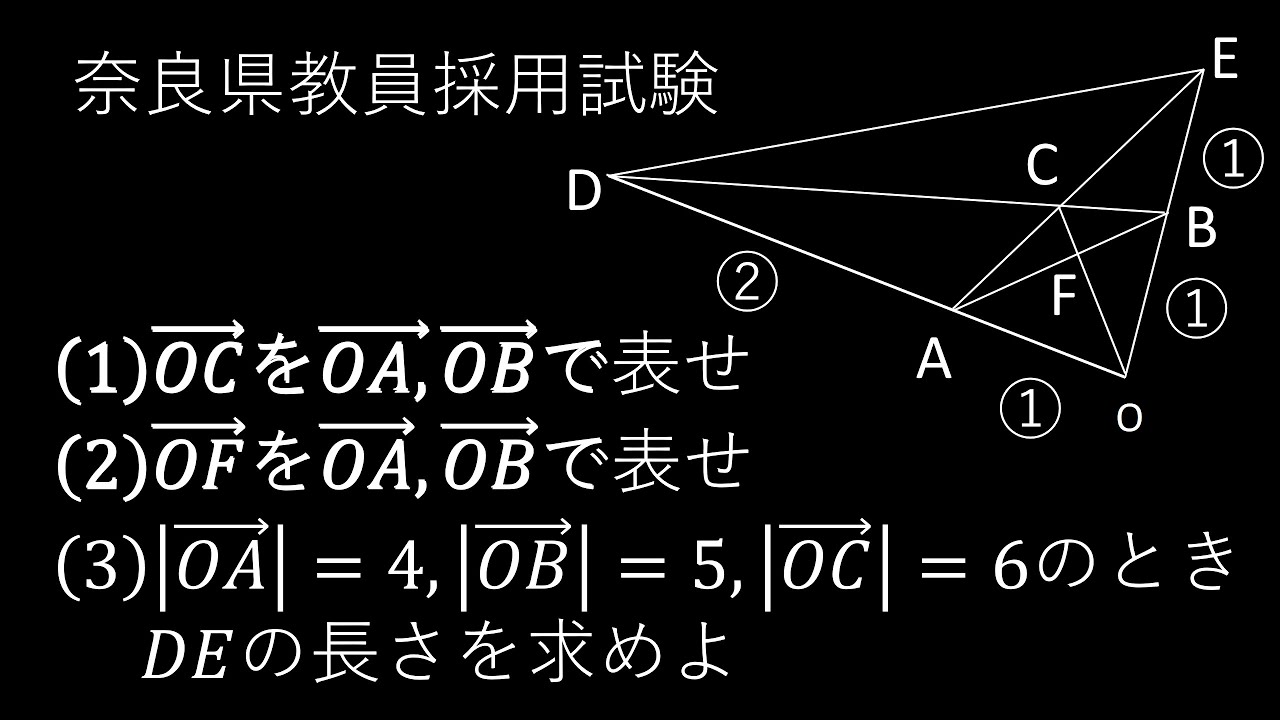
 一直線上にないO、A、B
一直線上にないO、A、B
BDとAEの交点をC
(1)
(2)OCとABの交点をF
AF:FBを求めよ。
(3)
この動画を見る
 一直線上にないO、A、B
一直線上にないO、A、BBDとAEの交点をC
(1)
(2)OCとABの交点をF
AF:FBを求めよ。
(3)
15京都府教員採用試験(数学:3番 微分)

15神奈川県教員採用試験(数学:10番 定積分)

13神奈川県教員採用試験(数学:9番 数列の極限値)

13神奈川県教員採用試験(数学:11番 曲線の長さ)

13神奈川県教員採用試験(数学:8番 行列)

13神奈川県教員採用試験(数学:4番 整式の割り算)

円周率πが無理数であることの証明(数III)
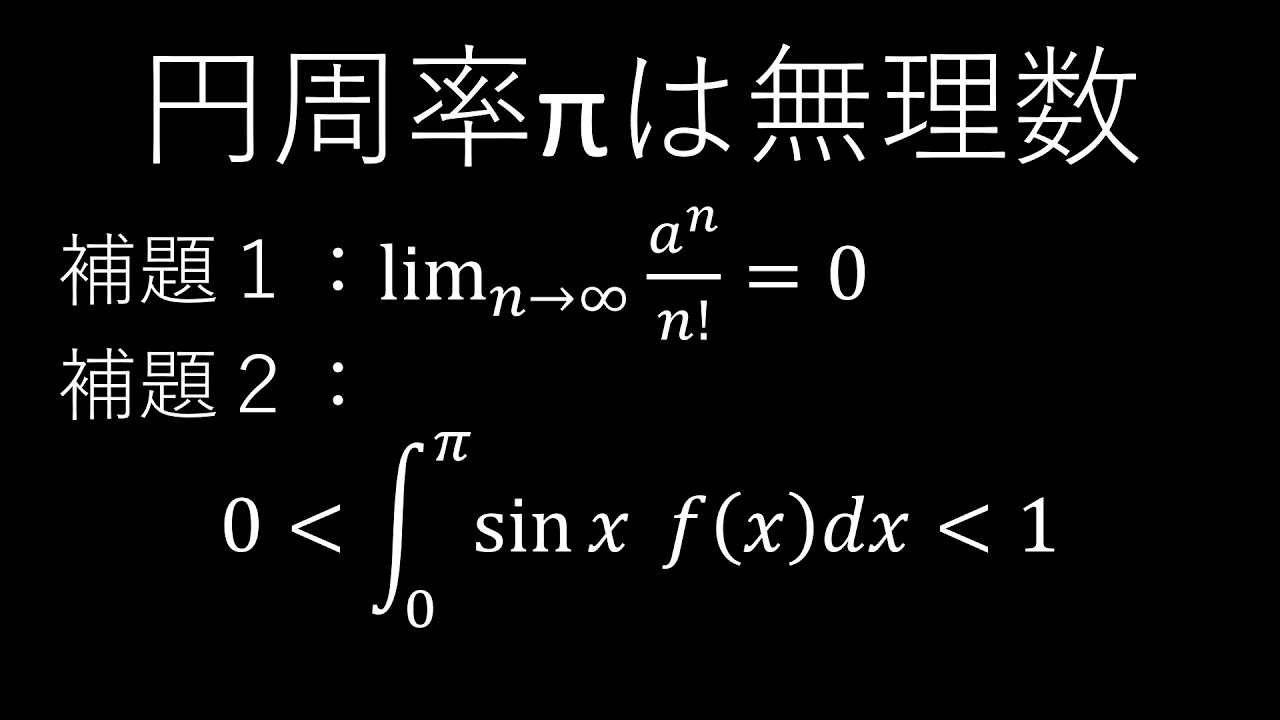
単元:
#関数と極限#積分とその応用#数列の極限#不定積分#数学(高校生)#数Ⅲ
指導講師:
ますただ
問題文全文(内容文):
定理(1947,IvanNiren)
πは無理数である
補題1
補題2
nが十分大きいとき
この動画を見る
定理(1947,IvanNiren)
πは無理数である
補題1
補題2
nが十分大きいとき
13兵庫県教員採用試験(数学:2番 微分)

10兵庫県教員採用試験(数学:2番 整数問題)

単元:
#数A#整数の性質#その他#数学(高校生)#教員採用試験
指導講師:
ますただ
問題文全文(内容文):

(1)A(10)を求めよ。
(2)A(n)=3をみたすnの個数
(3)
この動画を見る

(1)A(10)を求めよ。
(2)A(n)=3をみたすnの個数
(3)
15兵庫県教員採用試験(数学:3番 微積)

単元:
#数Ⅱ#微分法と積分法#その他#面積、体積#数学(高校生)#教員採用試験
指導講師:
ますただ
問題文全文(内容文):

Cは極大値16をもつ。C上の点(1,f(1))の接線lとCで囲まれた面積Sを求めよ。
この動画を見る

Cは極大値16をもつ。C上の点(1,f(1))の接線lとCで囲まれた面積Sを求めよ。
19奈良県教員採用試験(数学:2番 三角関数)

単元:
#数Ⅱ#三角関数#三角関数とグラフ#接線と増減表・最大値・最小値#その他#数学(高校生)#教員採用試験
指導講師:
ますただ
問題文全文(内容文):

この動画を見る

08大阪府教員採用試験(数学:1番 整数問題)

13大阪府教員採用試験(数学:1-1番 対数の整数問題)

単元:
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師:
ますただ
問題文全文(内容文):
 (1) 10<x<100
(1) 10<x<100
この動画を見る
 (1) 10<x<100
(1) 10<x<10010兵庫県教員採用試験(数学:2番 円と直線)

単元:
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師:
ますただ
問題文全文(内容文):

は2点P,Qで交わる。
(1)
(2)△PQRが最大となる円C上の点Rの座標を求めよ。
*図は動画内参照
この動画を見る

は2点P,Qで交わる。
(1)
(2)△PQRが最大となる円C上の点Rの座標を求めよ。
*図は動画内参照
11兵庫県教員採用試験(数学:1-4番 対数)

単元:
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
Warning: usort() expects parameter 1 to be array, bool given in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 269
Warning: Invalid argument supplied for foreach() in /home/kaiketsudb/kaiketsu-db.net/public_html/wp-content/themes/lightning-child-sample/taxonomy-teacher.php on line 270
指導講師:
ますただ
問題文全文(内容文):
 (4)
(4)
の最大値、最小値を求めよ。
この動画を見る
 (4)
(4)の最大値、最小値を求めよ。







